A study reports the accompanying data on discharge amount ( ), flow area ( ), and slope of the water surface (b, in m/m) obtained at a number of floodplain stations. The study proposed a multiplicative power model .
a. Use an appropriate transformation to make the model linear and then estimate the regression parameters for the transformed model. Finally, estimate
(the parameters of the original model). What would be your prediction of discharge amount when flow area is 10 and slope is .01?
b. Without actually doing any analysis, how would you fit a multiplicative exponential model 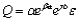
?
c. After the transformation to linearity in part (a), a 95% CI for the value of the transformed regression function when a = 3.3 and b = .0046 was obtained from computer output as (.217, 1.755). Obtain a 95% CI for
when a = 3.3 and b = .0046.
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q67: In the accompanying table, we give
Q68: Wear resistance of certain nuclear reactor
Q69: Let y = sales at a
Q70: In each of the following cases,
Q71: A multiple regression model with four
Q72: Answer the following questions.
a. Could a linear
Q73: Cardiorespiratory fitness is widely recognized as
Q74: A study reported data on y-tensile
Q75: The following data resulted from an
Q76: Consider the following data on mass
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents