Three factories each dump waste water containing three different types of pollutants into a river. State regulations require the factories to treat their waste in order to reduce pollution levels. The table shows the possible percent reduction of each pollutant at each site and the cost per ton to process the waste. 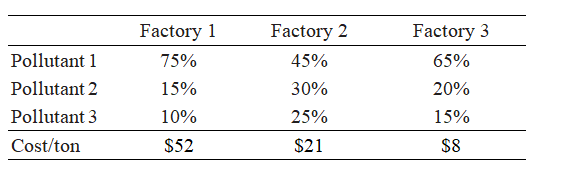
If the state requires a reduction of at least 65 tons per day of pollutant 1, at least 40 tons per day of pollutant 2, and at least 20 tons per day of pollutant 3, find the number of tons of waste that must be treated each day at each site so that the state's requirements are satisfied and the treatment costs are minimized. Find the minimum cost.
A) The minimum cost is $3,190 per day when Factory #2 and Factory #3 each process 110 tons and Factory #1 does not process any.
B) The minimum cost is $2,920 per day when Factory #1 and Factory #2 each process 40 tons and Factory #3 does not process any.
C) The minimum cost is $1,600 per day when Factory #3 process 200 tons, Factory #1 a and Factory #2 do not process any.
D) The minimum cost is $1,540 per day when Factory #1 and Factory #2 each process 20 tons and Factory #3 produces 10 tons.
E) The minimum cost is $2,490 per day when Factory #2 and Factory #3 each process 50 tons and Factory #1 produces 20 tons.
Correct Answer:
Verified
Q77: Suppose a primal minimization problem and its
Q78: Form the matrix associated with the given
Q79: Write the dual maximization problem. Be sure
Q80: Solve the following minimization problem by solving
Q81: Two factories produce three different types of
Q83: A primal maximization problem is given. Solve
Q84: Write the dual maximization problem.
Minimize
Q87: A minimization problem is given. Solve its
Q95: CDF Appliances has assembly plants in Atlanta
Q100: Nekita Corporation assembles cell phones and camera
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents