A triangle is inscribed in a semicircle of diameter 6R. Show that the smallest possible value for the area of the shaded region is . 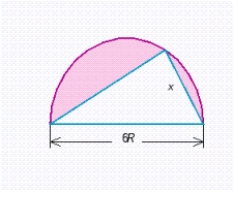 Hint: The area of the shaded region is a minimum when the area of the triangle is a maximum. Find the value of x that maximizes the square of the area of the triangle. This will be the same x that maximizes the area of the triangle.
Hint: The area of the shaded region is a minimum when the area of the triangle is a maximum. Find the value of x that maximizes the square of the area of the triangle. This will be the same x that maximizes the area of the triangle.
A) The hint tells us that the area of the region is a minimum when the area of the triangle is a maximum. We first find the value of x that maximizes the square of the area of the triangle. The area of the triangle is equal to .
The square of the area of the triangle is equal to , and the substitution will transform this expression into the quadratic function
Since we want to find the maximum value of t, we will substitute the value into the equation. Solving for t gives us the following minimum area of the shaded region: .
B) The hint tells us that the area of the region is a minimum when the area of the triangle is a maximum. We first find the value of x that maximizes the square of the area of the triangle. The area of the triangle is equal to .
The square of the area of the triangle is equal to The substitution will transform this expression into the quadratic function
Since the graph of equation (1) will be a parabola opening downward, the input t that yields a maximum value for this function is
Substituting the value into the equation gives us and consequently (The negative root can be rejected since the side of a triangle can't be negative) . With this value of x, we can calculate the minimum area of the shaded region. The minimum area of the shaded region is equal to
Substituting the value in the equation (2) gives us that the minimum value of the shaded region is equal to .
C) The hint tells us that the area of the region is a minimum when the area of the triangle is a maximum. We first find the value of x that maximizes the square of the area of the triangle. The square of the area of the triangle is equal to .
The substitution will transform this into the quadratic function
Since the graph of equation (1) will be a parabola opening downward, the input t that yields a maximum value for this function is
Substituting the value into the equation gives us and consequently . With this value of x, we can calculate the minimum area of the shaded region. The minimum area of the shaded region is equal to
Substituting the value into the equation (2) , we find that the minimum value of the shaded region is equal to .
D) The hint tells us that the area of the region is a minimum when the area of the triangle is a maximum. We first find the value of x that maximizes the square of the area of the triangle.
The square of the area of the triangle is equal to , which is a quadratic function. The graph of this function will be a parabola opening downward, so we can write the maximum value of this function as:
We can then write as and calculate the minimum area of the shaded region. Substituting this value into the area equation, we find its minimum area: .
E) The hint tells us that the area of the region is a minimum when the area of the triangle is a maximum. We first find the value of x that maximizes the square of the area of the triangle. The square of the area of the triangle is equal to .
The substitution will transform this into the quadratic function
Since the graph of equation (1) will be a parabola opening downward, the input t that yields a maximum value for this function is
Substituting the value into the equation gives us and consequently . With this value of x, we can calculate the minimum area of the shaded region. The minimum area of the shaded region is equal to
Substituting the value into the equation (2) , we find that the minimum value of the shaded region is equal to .
Correct Answer:
Verified
Q1: Find all fixed points of the
Q2: Suppose that the revenue generated by
Q3: Sketch the graph of the function
Q4: Graph the quadratic function. Specify the
Q5: Sketch the graph of the function and
Q7: Plot the following points: (6, 2), (7,
Q8: Sketch the graph of the function and
Q9: What is the largest possible area for
Q10: Find the linear function satisfying the
Q11: For the following figure, express the
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents