Cheater? A group of curious college students decide to test the integrity of their fellow collegians. In order to see if students will cheat, when given an opportunity, they decide to use chocolately M&M's. They tell each student that a discerning palette will be able to tell the difference in flavor between and red and a yellow candy. The blindfolded subjects are given two piles of candy to test. But the experimenter turns his back so that the subject thinks that they have a window of opportunity to take a quick peak. Unbeknownst to the subjects, there is another helper who is hidden and secretly watching to see who cheats. Here is their data.
a. What is the probability that a subject cheated?
b. If a subject was a male, what are the chances that they cheated?
c. Using your answers to (a) and (b), does it appear that cheating and gender are
independent?
d. A statistics student in the group decides she wants to run a Chi-square test for independence. Why would this not be an advisable choice?
e. An argument begins. The girls are suggesting that the guys cheated more than girls; and
that this difference is larger than can be explained by chance variation. Of course, the guys insist that with a small sample size like this, anything could happen. Fortunately, a randomization machine is discovered. The 18 observations are randomly placed into the 4 categories randomly. This procedure is repeated 1000 times and the number of male
cheaters is counted each time. A graph is below. What does this graph tell you about the claims of the two groups? 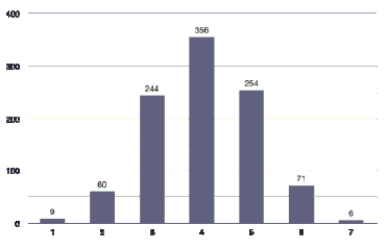
Correct Answer:
Verified
b. 6/8 = 75%
c. Since the ...
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q2: Poverty In a study of how
Q3: Suppose that after the study described in
Q4: For the scenario described below, simply name
Q5: College admissions According to information from a
Q6: Peanut M&Ms According to the Mars Candy
Q8: A sports analyst was interested in
Q9: Suppose you were asked to analyze
Q10: A college admissions counselor was interested
Q11: Height and weight Is the height
Q12: For the scenario described below, simply name
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents