Solved

Formulate but Do Not Solve the Following Exercise as a Linear

Question 118

Multiple Choice

Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit?


A) Maximize: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to: Subject to: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to:
B) Maximize: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to: Subject to: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to:
C) Maximize: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to: Subject to: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to:
D) Maximize: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: Subject to: B) Maximize: Subject to: C) Maximize: Subject to: D) Maximize: Subject to: Subject to: Formulate but do not solve the following exercise as a linear programming problem. A farmer has 140 acres of land suitable for cultivating crops A and B. The cost of cultivating crop A is $30/acre, whereas that of crop B is $80/acre. The farmer has a maximum of $5,700 available for land cultivation. Each acre of crop A requires 30 labor-hours, and each acre of crop B requires 35 labor-hours. The farmer has a maximum of 4,800 labor-hours available. If she expects to make a profit of $130/acre on crop A and $230/acre on crop B, how many acres of each crop should she plant in order to maximize her profit? A) Maximize: 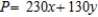Subject to: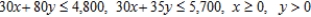 B) Maximize: 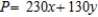Subject to: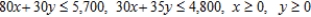 C) Maximize: 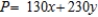Subject to: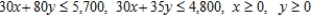 D) Maximize: 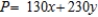Subject to: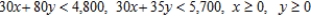

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q113: Custom Office Furniture Company is introducing a

Q114: Formulate but do not solve the following

Q115: Formulate but do not solve the following

Q116: AntiFam, a hunger-relief organization, has earmarked between

Q117: Acoustical Company manufactures a CD storage cabinet

Q119: Formulate but do not solve the following

Q120: Formulate but do not solve the following

Q121: Kane Manufacturing has a division that produces

Q122: Solve the linear programming problem by the

Q123: National Business Machines manufactures two models of

Unlock this Answer For Free Now!

View this answer and more for free by performing one of the following actions

qr-code

Scan the QR code to install the App and get 2 free unlocks

upload documents

Unlock quizzes for free by uploading documents