Tracksaws, Inc Formulation:
Let X = Number of Tractors Produced Per Period

Tracksaws, Inc. makes tractors and lawn mowers. The firm makes a profit of $30 on each tractor and $30 on each lawn mower, and they sell all they can produce. The time requirements in the machine shop, fabrication, and tractor assembly are given in the table.
$$\begin{array} { l l l l } & \text { Machine Shop } & \text { Fabrication } & \text { Assembly } \\\hline \text { Tractor } & 2 \text { hours } & 2 \text { hours } & 1 \text { hour } \\\text { Lawn Mower } & 1 \text { hour } & 3 \text { hours } & 0 \text { hour } \\\text { Hrs. Available } & 60 \text { hours } & 120 \text { hours } & 45 \text { hours } \\\hline\end{array}$$ Formulation:
Let x = number of tractors produced per period
y = number of lawn mowers produced per period


$\begin{array} { l } \text { MAX 30x+30y } \\\qquad\text { subject to } 2 x + y \quad \leq 60 \\\qquad 2 x + 3 y \quad \leq 120 \\\qquad x \leq 45\end{array}$

The graphical solution is shown below.

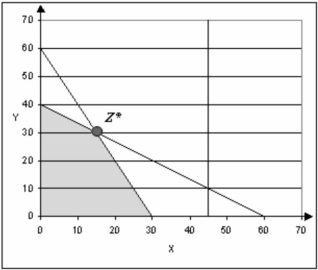
-What is the maximum amount a manager would be willing to pay for one additional hour of machining time?