Solved

If the Variance of U Is Quadratic in X,then It

Question 29

Multiple Choice

If the variance of u is quadratic in X,then it can be expressed as


A) var(ui|Xi) = If the variance of u is quadratic in X,then it can be expressed as A) var(ui|Xi) = B) var(ui|Xi) = θ0 + θ1 C) var(ui|Xi) = θ0 + θ1 D) var(ui|Xi) =
B) var(ui|Xi) = θ0 + θ1 If the variance of u is quadratic in X,then it can be expressed as A) var(ui|Xi) = B) var(ui|Xi) = θ0 + θ1 C) var(ui|Xi) = θ0 + θ1 D) var(ui|Xi) =
C) var(ui|Xi) = θ0 + θ1 If the variance of u is quadratic in X,then it can be expressed as A) var(ui|Xi) = B) var(ui|Xi) = θ0 + θ1 C) var(ui|Xi) = θ0 + θ1 D) var(ui|Xi) =
D) var(ui|Xi) = If the variance of u is quadratic in X,then it can be expressed as A) var(ui|Xi) = 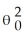B) var(ui|Xi) = θ0 + θ1 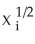C) var(ui|Xi) = θ0 + θ1 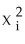D) var(ui|Xi) =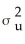

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q21: The advantage of using heteroskedasticity-robust standard errors

Q22: In practice, you may want to use

Q25: Homoskedasticity means that
A)var(ui|Xi)= Homoskedasticity means</p></div><svg width=

Q27: (Requires Appendix material)If X and Y are

Q29: What does the Gauss-Markov theorem prove? Without

Q31: One of the earlier textbooks in econometrics,

Q32: Suppose that the conditional variance is var(ui|Xi)=

Q34: In practice, the most difficult aspect of

Q34: In order to use the t-statistic for

Q39: "I am an applied econometrician and therefore

Unlock this Answer For Free Now!

View this answer and more for free by performing one of the following actions

qr-code

Scan the QR code to install the App and get 2 free unlocks

upload documents

Unlock quizzes for free by uploading documents