The mean of the population ( 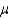 ) is 200 on a test that measures math skills of middle school students.The variance
) is 200 on a test that measures math skills of middle school students.The variance 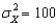 .The test scores for the students in Mr.Petris's class at Suburban Middle School are given below.Use a rejection region with a probability of 5% only in the upper tail.What should you conclude?
.The test scores for the students in Mr.Petris's class at Suburban Middle School are given below.Use a rejection region with a probability of 5% only in the upper tail.What should you conclude? 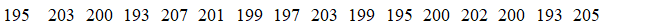
A) Since the z-value falls within the region of rejection,we should conclude this sample mean likely represents some other population.
B) Since the z-value does not fall within the region of rejection,we should not conclude this sample mean represents some other population.
C) Since the z-value falls within the region of rejection,we should not conclude this sample mean represents some other population.
D) Since the z-value does not fall within the region of rejection,we should conclude this sample mean likely represents some other population.
Correct Answer:
Verified
Q45: Suppose you drew a random sample from
Q46: A cognitive psychologist tested whether people
Q47: When +1.645 is used as the critical
Q48: A study about the college aptitude of
Q49: Suppose you drew a random sample from
Q51: How can we determine the representativeness of
Q52: When a z-score is not in the
Q53: If we decide not to reject the
Q54: In a test of physical fitness,a group
Q55: The introductory biology class at State University
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents