The partial mega stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. 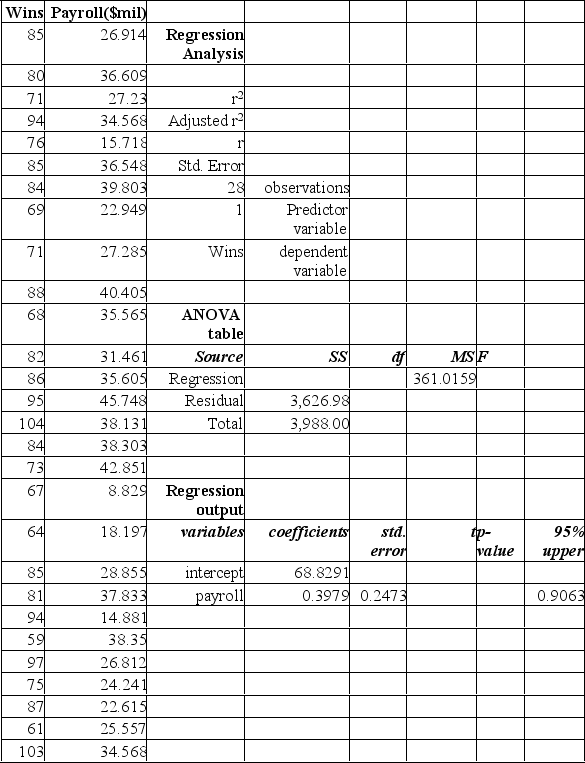 Refer to the printout above. The regression equation is:
Refer to the printout above. The regression equation is:
A) 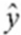 = 0.379 + 68.8291x
= 0.379 + 68.8291x
B) 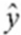 = 68.8291 + 0.3979x
= 68.8291 + 0.3979x
C) 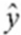 = 0.2473 + 0.3979x
= 0.2473 + 0.3979x
D) 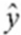 = 68.8291 + 0.2473x
= 68.8291 + 0.2473x
E) 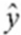 = 0.2473 + 68.8291x
= 0.2473 + 68.8291x
Correct Answer:
Verified
Q53: i. The purpose of correlation analysis is
Q54: Data is collected from 20 sales people
Q55: Information was collected from employee records to
Q56: Data is collected from 20 sales people
Q57: i. A coefficient of correlation close to
Q59: A sales manager for an advertising agency
Q60: The partial Mega Stat output below is
Q61: i. A coefficient of correlation close to
Q62: i. If the value of r is
Q63: i. What is the range of values
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents