Find the critical point(s) of the function. Then use the second derivative test to classify the nature of each point, if possible. Finally, determine the relative extrema of the function. 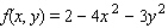
A) (0, 0) ; relative maximum value: f(0, 0) = 2
B) (2, 4) ; relative maximum value: f(0, 0) = 3
C) (3, 0) ; relative maximum value: f(0, 0) = 4
Correct Answer:
Verified
Q135: Find the critical point(s) of the function.
Q136: Find the critical point(s) of the function.
Q137: An auxiliary electric power station will serve
Q138: An open rectangular box having a volume
Q139: Find the critical point(s) of the function.
Q141: Determine whether the statement is true or
Q142: A building in the shape of a
Q143: An auxiliary electric power station will serve
Q144: Find the first partial derivatives of the
Q145: Find the critical point(s) of the function.
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents