Multiple Choice
Let 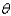 be the angle between equal sides of an isosceles triangle and let x be the length of these sides. x is increasing at
be the angle between equal sides of an isosceles triangle and let x be the length of these sides. x is increasing at 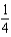 meter per hour and
meter per hour and 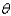 is increasing at
is increasing at 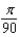 radian per hour. Find the rate of increase of the area when
radian per hour. Find the rate of increase of the area when 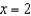 and
and 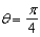 . Round your answer to three decimal places.
. Round your answer to three decimal places.
A) 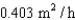
B) 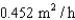
C) 
D) 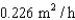
E) 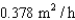
Correct Answer:
Verified
Related Questions

