Solved

Let and Let S Be the Surface Bounded by

Question 2

Multiple Choice

Let Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E) and let S be the surface bounded by Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E) and Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E) . Verify the Divergence Theorem by evaluating Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E) as a surface integral and as a triple integral. Round your answer to two decimal places.


A) Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E)
B) Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E)
C) Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E)
D) Let and let S be the surface bounded by and . Verify the Divergence Theorem by evaluating as a surface integral and as a triple integral. Round your answer to two decimal places. A) B) C) D) E)
E) Let 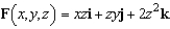and let S be the surface bounded by 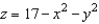and 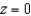. Verify the Divergence Theorem by evaluating 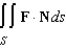as a surface integral and as a triple integral. Round your answer to two decimal places. A) 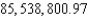B) 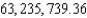C) 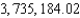D) 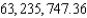E)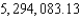

Correct Answer:

verifed

Verified

Related Questions

Q1: Evaluate Evaluate ,</p></div><svg width=

Q3: Evaluate Evaluate where</p></div><svg width=

Q4: Use Divergence Theorem to evaluate

Q5: Use Divergence Theorem to evaluate

Q6: Let Let and</p></div><svg width=

Q7: Use the Divergence Theorem to evaluate

Q8: Use Divergence Theorem to evaluate

Q9: Use the Divergence Theorem to evaluate

Q10: Let Let and</p></div><svg width=

Q11: Use Divergence Theorem to evaluate

Unlock this Answer For Free Now!

View this answer and more for free by performing one of the following actions

qr-code

Scan the QR code to install the App and get 2 free unlocks

upload documents

Unlock quizzes for free by uploading documents