Graph the parametric equations using the given range for the parameter . Begin with the standard viewing rectangle and then make adjustments, as necessary, so that the graph utilizes as much of the viewing screen as possible. For example, in graphing the circle given by and it would be natural to choose a viewing rectangle extending from -1 to 1 in both the - and -directions. Graph the parametric equations on a graphing utility. Sketch the result. and , (one-quarter of an ellipse)
A) 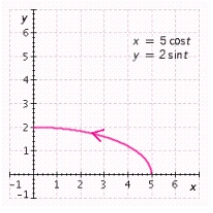
B) 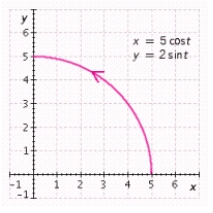
C) 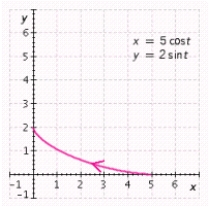
D) 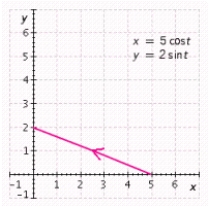
E) 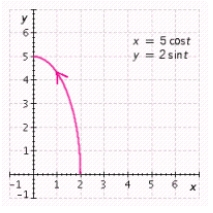
Correct Answer:
Verified
Q8: Assume that the vectors
Q9: Convert to rectangular form.
Q10: Determine the graph that reflects the
Q11: Assume that the vectors
Q12: Refer to the figure. If
Q14: Assume that the coordinates of the
Q15: Use the given information to find
Q16: Use the equation to determine polar
Q17: On a sheet of paper, graph
Q18: Determine the graph that reflects the
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents