A researcher wishes to determine if students are able to complete a certain puzzle more quickly while exposed to a pleasant floral scent. Suppose the time (in seconds) needed for high school students to complete the puzzle while exposed to the scent follows a normal distribution with mean and standard deviation = 4. Suppose also, that in the general population of all high school students, the time needed to complete the puzzle follows a normal distribution with mean 80 and standard deviation = 4. The researcher, therefore, decides to test the hypotheses:
H0: = 80 Ha: < 80
To do so, the researcher has 10,000 high school students complete the puzzle in the presence of the floral scent. The mean time for these students is 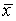
= 79.8 seconds and the P-value is less than 0.0001.
Suppose that two high school students decide to see if they get the same results as the researcher. They both do the puzzle while in the presence of the pleasant floral scent. The mean of their times is 80.2 seconds, the same as that of the researcher. It is appropriate to conclude which of the following:
A) they have reproduced the results of the researcher and their P-value will be the same as that of the researcher.
B) they have reproduced the results of the researcher, but their P-value will be slightly smaller than that of the researcher.
C) they will reach the same statistical conclusion as the researcher, but their P-value will be slightly different than that of the researcher.
D) none of the answer choices are correct.
Correct Answer:
Verified
Q1: An anthropologist wants to compare the average
Q2: Which of the following would be
Q3: How small must a P-value be in
Q4: A researcher wishes to determine if
Q5: Coleman surveys a random sample of city
Q7: A medical researcher is working on a
Q8: A television show runs a call-in survey
Q9: A government agency funds research on cancer.
Q10: An engineer designs an improved light bulb.
Q11: A popular brand of AAA batteries has
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents