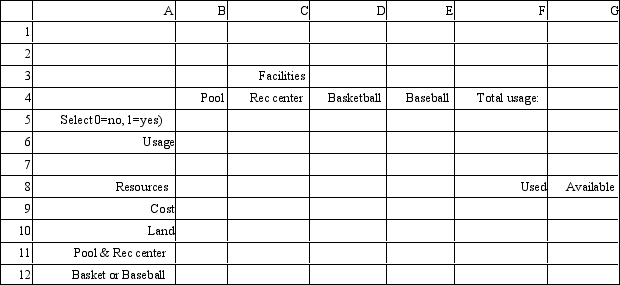
A small town wants to build some new recreational facilities.The proposed facilities include a swimming pool,recreation center,basketball court and baseball field.The town council wants to provide the facilities which will be used by the most people,but faces budget and land limitations.The town has $400,000 and 14 acres of land.The pool requires locker facilities which would be in the recreation center,so if the swimming pool is built the recreation center must also be built.Also the council has only enough flat land to build the basketball court or the baseball field.The daily usage and cost of the facilities in $1,000)are shown below.
Based on this ILP formulation of the problem and the indicated optimal solution what values should go in cells
B5:G12 of the following Excel spreadsheet?
Solution: )
Correct Answer:
Verified
Q52: A company wants to build a new
Q53: A company wants to build a new
Q54: An investor has $500,000 to invest
Q55: A company needs to hire workers
Q57: A cellular phone company wants to
Q58: An investor has $500,000 to invest
Q59: A cellular phone company wants to
Q60: A cellular phone company wants to
Q61: A certain military deployment requires supplies
Q84: A company has four projects, numbered 1
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents