Construct a 95% Z-interval or a 95% t-interval about the population mean. Assume the data come from a population that is approximately normal with no outliers.
-The heights of 20- to 29-year-old females are known to have a population standard deviation 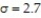 inches. A simple random sample of n = 15 females 20 to 29 years old results in the following data:
inches. A simple random sample of n = 15 females 20 to 29 years old results in the following data: 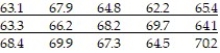
A) (65.20, 67.50) ; we are 95% confident that the mean height of 20- to 29-year-old females is between 65.20 and 67.50 inches.
B) (64.98, 67.72) ; we are 95% confident that the mean height of 20- to 29-year-old females is between 64.98 and 67.72 inches.
C) (65.12, 67.58) ; we are 95% confident that the mean height of 20- to 29-year-old females is between 65.12 and 67.58 inches.
D) (64.85, 67.85) ; we are 95% confident that the mean height of 20- to 29-year-old females is between 64.85 and 67.85 inches.
Correct Answer:
Verified
Q14: Find the t-value. Q15: Find the t-value. Q16: Find the t-value. Q17: In a random sample of 26 laptop Q18: In order to set rates, an insurance Q19: In a random sample of 60 dog Q20: A survey of 1010 college seniors working Q22: Construct a 95% Z-interval or a 95% Q23: A random sample of 20 electricians is Q24: A college nurse obtained a random sample
-Let ![]()
-Find the t-value such that
-Find the t-value such that
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents