True/False
If the columns of a matrix
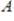 with
with
 rows and
rows and
 columns do not span Rn, then there exists a vector
columns do not span Rn, then there exists a vector
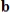 in Rn such that
in Rn such that
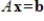 does not have a solution.
does not have a solution.
Correct Answer:
Verified
Related Questions
Q27: Determine if the system

Q28: Find all values of

Q29: For what value(s) of h do the
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents