Solve the problem.
-A satellite is to be put into an elliptical orbit around a moon. The moon is a sphere with radius of . Determine an equation for the ellipse if the distance of the satellite from the surface of the moon varies from 910 to .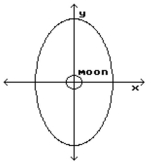
A)
B)
C)
D)
Correct Answer:
Verified
Q58: Graph the ellipse. Q59: Match the given graph with its Q60: Graph the ellipse. Q61: Match the given graph with its Q62: Find an equation in standard form Q64: Solve the problem. Q65: Find the vertices and foci of Q66: Find the vertices and foci of Q67: Find the eccentricity of the ellipse. Q68: Find an equation in standard form
-
-
-A railroad tunnel is
-
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents