Of the 23 First Year Male Students at State U $\quad$

Of the 23 first year male students at State U. admitted from Jim Thorpe High School, 8 were offered baseball scholarships and 7 were offered football scholarships. The University admissions committee looked at the students' composite ACT scores (shown in table), wondering if the University was lowering their standards for athletes. Assuming that this group of students is representative of all admitted students, what do you think?

$\quad$$\quad$$\text {Composite ACT Score }$
$\begin{array}{|c|c|c|}\hline\text { Baseball }&\text { Non-athletes }& \text {Football }\\\hline 25 & 21 & 22 \\22 & 27 & 21 \\19 & 29 & 24 \\25 & 26 & 27 \\24 & 30 & 19 \\25 & 27 & 23 \\24 & 26 & 17 \\23 & 23 & \\\hline\end{array}$


Boxplots: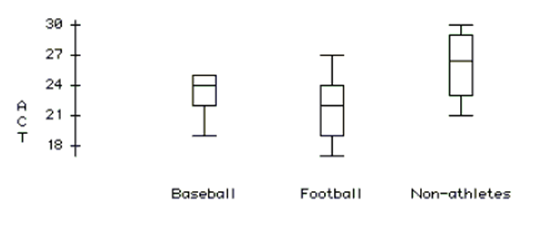




$\text {Analysis of Variance Table}$
$\begin{array}{|c|c|r|c|c|c|}\hline &\text { Sums of }&&\text { Mean }&&\text { P-} \\ \text { Source}&\text { Squares } &d f &\text { Squares } &\text { F-ratio }&\text { value }\\\hline Team & 71.00 & 2 & 35.50 & 4.56 & 0.023 \\\hline Error & 155.61 & 20 & 7.78 & & \\\hline Total & 226.61 & 22 & & & \\\hline\end{array}$

$\text {Means and Std Deviations}$
$\begin{array}{|l|r|r|r|}\hline \text {Level }& \text {Number} & \text {Mean }& \text {Std Dev} \\\hline \text {Baseball} & 8 & 23.3750 & 2.06588 \\\hline \text {Football }& 7 & 21.8571 & 3.28778 \\\hline \text {Non Athlete} & 8 & 26.1250 & 2.94897 \\\hline\end{array}$



Normal Probability Plot: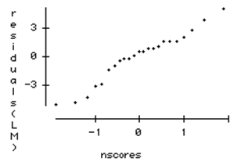


-Test an appropriate hypothesis and state your conclusion