Five-Digit Random Numbers Are Used to Simulate Scores on Each

Five-digit random numbers are used to simulate scores on each hole in an 18-hole round c The correspondence between the random numbers and scores on each hole is chosen to bc follows.
$\begin{array}{l|c}\text { Numbers } & \text { Score (Probability) } \\\hline 00000 & \text { Hole-in-one }(0.001 \%) \\00001-05000 & \text { Eagle }(5 \%) \\05001-20000 & \text { Birdie }(15 \%) \\20001-60000 & \text { Par }(40 \%) \\60001-90000 & \text { Bogey }(30 \%) \\90001-99999 & \text { Double-Bogey }(10 \%) \end{array}$

Use the following portion of a random numbers table starting with the highlighted numbe and determine how many holes the simulated golfer made par or better (i.e., how many holes-in-one, eagles, birdies, and pars were obtained in the 18 holes) . Use the highlightec as the score on the first hole; work down a column moving from left to right, column to $\mathrm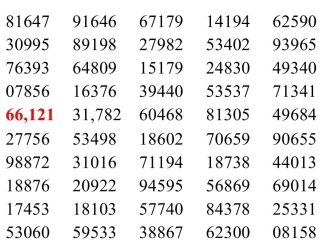 { c }$ : needed.

olf. number
Lumn as

A) 13
B) 10
C) 12
D) 11