Determine if the graph can represent a polynomial function. If so, assume the end behavior and all turning points are represented on the graph.
-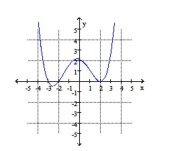
a. Determine the minimum degree of the polynomial based on the number of turning points.
b. Determine whether the leading coefficient is positive or negative based on the end behavior and Whether the degree of the polynomial is odd or even.
c. Approximate the real zeros of the function, and determine if their multiplicity is odd or even.
A) a. Minimum degree 4
b. Leading coefficient positive degree even
c. (odd multiplicity) , (odd multiplicity) , 2 (even multiplicity)
B) a. Minimum degree 3
b. Leading coefficient positive degree odd
c. , and 2 (each with odd multiplicity)
C) a. Minimum degree 3
b. Leading coefficient negative degree odd
c. (even multiplicity) , (even multiplicity) , 2 (odd multiplicity)
D) Not a polynomial function.
Correct Answer:
Verified
Q92: Determine whether the intermediate value theorem
Q93: Solve the problem. Q94: Find the zeros of the function Q95: Sketch the function. Q96: Sketch the function. Q98: Find the zeros of the function Q99: Solve the problem. Q100: Sketch the function. Q101: Write the word or phrase that Q102: Choose the one alternative that best Unlock this Answer For Free Now! View this answer and more for free by performing one of the following actions Scan the QR code to install the App and get 2 free unlocks Unlock quizzes for free by uploading documents
-
-
-
-
-

