A local business A has two competitors, B and C. No customer patronizes more than one of these businesses at the same time. Initially, the probabilities that a customer patronizes A, B, or C are 0.2, 0.6, and 0.2, respectively. Suppose A initiates an advertising campaign to improve its business and finds the following transition matrix to describe the effect. 
Find the steady-state vector for this market-that is, the long-range share of the market that each business can expect if the transition matrix holds.
A) 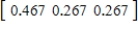
B) 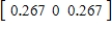
C) 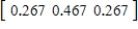
D) 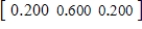
E) 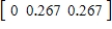
Correct Answer:
Verified
Q168: Find the steady-state vector associated with the
Q169: The local community-service funding organization in a
Q170: A Markov chain has transition matrix
Q171: Suppose a government study estimated that the
Q172: A man owns an Audi, a Ford,
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents