Perth Mining Company operates two mines for the purpose of extracting gold and silver. The Saddle Mine costs $16,000/day to operate, and it yields 50 oz of gold and 3,000 oz of silver per day. The Horseshoe Mine costs $17,000/day to operate, and it yields 75 oz of gold and 1,000 ounces of silver each day. Company management has set a target of at least 825 oz of gold and 18,000 oz of silver. How many days should each mine be operated so that the target can be met at a minimum cost? Find the range of values that the cost of operating the Saddle Mine per day can assume without changing the optimal solution.
Find the range of values that the requirement for gold can assume.
Find the shadow price for the requirement for gold.
A) Saddle Mine = 9 days, Horseshoe Mine = 3 days, Minimum cost = $201,000 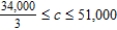
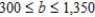 $201,200.00
$201,200.00
B) Saddle Mine = 3 days, Horseshoe Mine = 9 days, Minimum cost = $200,997 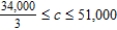
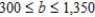 $200.00
$200.00
C) Saddle Mine = 3 days, Horseshoe Mine = 9 days, Minimum cost = $201,007 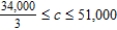
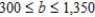
$200.00
D) Saddle Mine = 3 days, Horseshoe Mine = 9 days, Minimum cost = $201,000 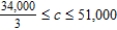
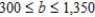 $200.00
$200.00
Correct Answer:
Verified
Q185: Kane Manufacturing has a division that produces
Q186: Solve the following linear programming problem by
Q187: Use the method of corners to solve
Q188: Use the method of corners to solve
Q189: Soundex produces two models of clock radios.
Q191: You are given a linear programming problem.
Q192: Consider the production problem: One company wishes
Q193: Use the method of corners to solve
Q194: Kane Manufacturing has a division that produces
Q195: Soundex produces two models of clock radios.
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents