Consider the production problem: One company wishes to produce two types of souvenirs: type A and type B. Each type-A souvenir will result in a profit of $1, and each type-B souvenir will result in a profit of $1.2. To manufacture a type-A souvenir requires 2 minutes on machine I and 1 minute on machine II. A type-B souvenir requires 1 minute on machine I and 3 minutes on machine II.There are 180 minutes available on machine I and 300 minutes available on machine II for processing the order.
Maximaze 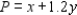 Objective function
Objective function
Subject to 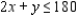 Constrained 1
Constrained 1 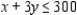 Constrained 2
Constrained 2 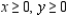 Now suppose the time available on machine I is changed from 180 minutes to (180 + h) minutes, where h is a real number. Then the constraint on machine I is changed to
Now suppose the time available on machine I is changed from 180 minutes to (180 + h) minutes, where h is a real number. Then the constraint on machine I is changed to 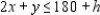 But the line with equation
But the line with equation 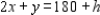 is parallel to the line
is parallel to the line 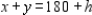 associated with the original constraint 1.
associated with the original constraint 1.
As you can see from the figure , the result of adding the constant h to the right-hand side of constraint 1 is to shift the current optimal solution from the point C to the new optimal solution occurring at the point C. To find the coordinates of C, we observe that C is the point of intersection of the lines with equations  Thus, the coordinates of the point are found by solving the system of linear equations
Thus, the coordinates of the point are found by solving the system of linear equations 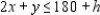
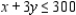
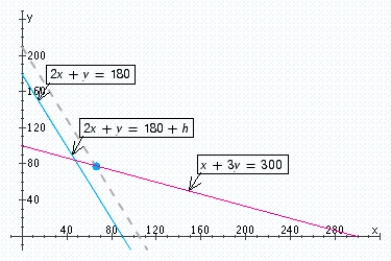 The solutions are
The solutions are 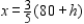 and
and 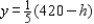 The nonnegativity of x implies that
The nonnegativity of x implies that 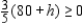
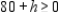
 Next, the nonnegativity of y implies that
Next, the nonnegativity of y implies that 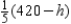

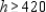 Thus, h must satisfy the inequalities
Thus, h must satisfy the inequalities 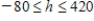 . Computations reveal that for a meaningful solution the time available for machine I must range between (180 - 80) and (180 + 420) minutes-that is, between 100 and 600 minutes.
. Computations reveal that for a meaningful solution the time available for machine I must range between (180 - 80) and (180 + 420) minutes-that is, between 100 and 600 minutes.
According to the problem, optimal solution would be 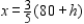 and
and 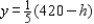 The resulting profit is calculated as follows:
The resulting profit is calculated as follows: 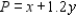
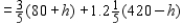
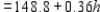 Upon setting h = 1, we find
Upon setting h = 1, we find 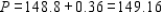 Since the optimal profit for the original problem is $159.6, we see that the shadow price for the first resource is 149.16 - 148.80, or $.36.
Since the optimal profit for the original problem is $159.6, we see that the shadow price for the first resource is 149.16 - 148.80, or $.36.
Show that if the time available on machine II is changed from 300 min to (300 + k) min, with no change in the maximum capacity for machine I, then company profit is maximized by producing 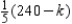 type-A souvenirs and
type-A souvenirs and 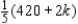 type-B souvenirs, where
type-B souvenirs, where 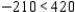 .
.
A) If the time available on machine II is changed from 300 min to (300 + k) min, with no change in the maximum capacity for machine I, the constraint on machine II is changed to 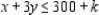 Optimal quantum of output is found by solving the system of linear equations
Optimal quantum of output is found by solving the system of linear equations 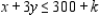 and
and 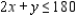 The solutions are
The solutions are  and
and 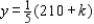 .
.
B) If the time available on machine I is changed from 180 min to (180 + h) min, with no change in the maximum capacity for machine I, the constraint on machine I is changed to 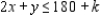 . Correspondingly constraint II is changed to
. Correspondingly constraint II is changed to 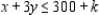 . In accordance with nonnegativity x and y (
. In accordance with nonnegativity x and y ( 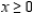 and
and 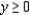 ) we could take x = 0 and y = 0 and substitute them to constraints:
) we could take x = 0 and y = 0 and substitute them to constraints: 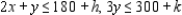 . Finally optimal value of type-A is
. Finally optimal value of type-A is 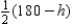 and optimal value of type-B is
and optimal value of type-B is 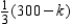
C) Suppose the time available on machine II is changed from 180 minutes to (180 + h) minutes, where h is a real number. Then the constraint on machine II is changed to 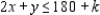 . New optimal solutions are found by solving the system of linear equations
. New optimal solutions are found by solving the system of linear equations 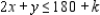 and
and  . Finally,
. Finally, 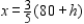 and
and 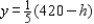 .
.
Correct Answer:
Verified
Q187: Use the method of corners to solve
Q188: Use the method of corners to solve
Q189: Soundex produces two models of clock radios.
Q190: Perth Mining Company operates two mines for
Q191: You are given a linear programming problem.
Q193: Use the method of corners to solve
Q194: Kane Manufacturing has a division that produces
Q195: Soundex produces two models of clock radios.
Q196: A farmer plans to plant two crops,
Q197: A company manufactures two products, A and
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents