Chat with AI
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060XCollege Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X Exercise 39
Step-by-step solution
Verified
Verified
Step 1 of 2
Consider the new scale score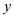 
is defined as a function of the old scale score 
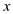by the equation
.
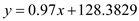(a)
Write the inequality to represent the new scale score greater than or equal to 1000 to admit a student.
It is needed to write that new scale score is greater than or equal to 1000.
That is,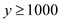 
.
But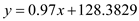 
.
Thus, the inequality becomes,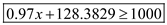 
.
Now solve for
.
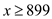
Therefore, the solution is,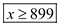 
.
Step 2 of 2
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255
