Deck 10: Nonlinear Programming
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/72
Play
Full screen (f)
Deck 10: Nonlinear Programming
1
Maximum profit is achieved everywhere the first derivative of the profit function equals zero.
False
2
If a nonlinear programming model consists of a single nonlinear objective function and a single linear constraint, it is called a(n) ________ optimization problem.
constrained or nonlinear
3
The slope of a curve at its highest point equals 1.
False
4
The first derivative of a cost function equals zero at the point V = 100. This is definitely the worst output volume for the producer to choose.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
5
Both linear and nonlinear programming models have the general form of an objective function subject to more than 1 constraint.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
6
Decision variables cannot be multiplied by each other in the objective function of a nonlinear program.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
7
Both linear and nonlinear programming models are examples of constrained optimization models.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
8
If a nonlinear program has been correctly formulated, procedures guarantee a solution.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
9
Constraints for nonlinear programs are usually nonlinear.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
10
In solving the facility location problem, the objective is to locate a centralized facility that serves customers or other facilities such that the distance traveled between the facility and customers or other facilities is minimized.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
11
Classical optimization is the use of calculus to determine the optimal value of a variable.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
12
A firm has a cost function of 3x2- 25x + 374. Without having two examples of their output volume and total cost, we cannot determine their fixed cost.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
13
Classical optimization uses calculus to determine the optimal values of a variable.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
14
The Lagrange multiplier is analogous to the dual variables in a linear programming problem.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
15
The slope of a curve at any point is equal to the derivative of the curves function.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
16
A profit function of Z = 3x2 - 12x + 5 reaches maximum profit at x = 2 units of output.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
17
In portfolio selection problems, risk is measured by the variance of the return on the portfolio.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
18
The Lagrange multiplier at the optimum gives only the instantaneous rate of change in the objective value.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
19
An optimal solution to a nonlinear programming problem will always occur at the boundary of the feasible solution space formed by the constraint.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
20
In an unconstrained nonlinear programming problem, we have a single nonlinear objective function and no constraints..

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
21
The objective of a facility location problem is to minimize ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
22
If a nonlinear programming model consists of a single nonlinear objective function and no constraints, it is called a(n) ________ optimization problem.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
23
The first derivative of the fixed cost line is ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
24
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation: 1000 - 8p.
What is the derivative of the profit function for the XYZ company? Simplify the terms as much as possible.
What is the derivative of the profit function for the XYZ company? Simplify the terms as much as possible.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
25
Assume price and demand are related by the following function: v = 100 - 2.5p. If fixed cost = $5000 and variable cost = $10, then the expression for profit is ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
26
________, a measure of correlation between returns on investment i and returns on investment j is used to reflect risk.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
27
Assume price and demand are related by the following function: v = 200 - p. If fixed cost = $10,000 and variable cost = $8, then the expression for profit is ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
28
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation: 1000 - 8p.
-What is the nonlinear profit function for the XYZ company? Simplify the terms as much as possible.
-What is the nonlinear profit function for the XYZ company? Simplify the terms as much as possible.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
29
Assume a nonlinear programming problem with a single constraint has been solved. The value of the Lagrange multiplier is $0.75 and the value of the optimal profit (Z) is $25. If the right-hand side of the constraint is increased from 38 to 42, the new value of Z will be ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
30
The distance formula of d = 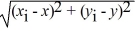
will find the ________ distance between two locations.
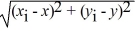
will find the ________ distance between two locations.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
31
The ________ measure of distance between two points on a set of X and Y coordinates is the hypotenuse of a right triangle.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
32
If a firm's profit is Z = 20p -2p2 + 40, then the optimal value of I yields a maximum profit of ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
33
The ________ the variability in an investment portfolio, the ________ the risk of the investment portfolio.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
34
If price and demand are related by the function v = 15 + 15p and the fixed cost is $150 while the variable cost is $5, then the expression for profit is ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
35
The ________ reflects the approximate change in the objective function resulting from a unit change in the quantity (right-hand-side) value of the constraint.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
36
If price and demand are related by the function v = 15 + 15p and the fixed cost is $150 while the variable cost is $5, then the profit at a price of 20 Rupees is ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
37
If a nonlinear programming problem results in profit (Z) of $50, and the Lagrange multiplier for a constraint is -2, the new profit will be ________ if the right-hand side of the constraint is increased by 1 unit.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
38
The ________ of the value of investment is a measure of risk.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
39
The dual value of a resource in a nonlinear programming model is given by the ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
40
If a firm's profit is Z = 100p -8p2 +16, then the maximum profit occurs where p = ________.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
41
A custom molder produces 6-ounce juice glasses and 10-ounce cocktail glasses. The per unit contribution for the juice glasses (x1) is equal to 60 - 5x1, and the per unit contribution for the cocktail glasses (x2) is 80 - 4x2. An expression for the total contribution is:
A) 20 - 4x2 - 5 x1
B) 60 x1 - 5x12 + 80x2 - 4x22
C) 80x1 - 5x122 - 4x22
D) 20 - (4x2)(5x1)
A) 20 - 4x2 - 5 x1
B) 60 x1 - 5x12 + 80x2 - 4x22
C) 80x1 - 5x122 - 4x22
D) 20 - (4x2)(5x1)

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
42
Lush Lawns, Inc. provides a lawn fertilizer and weed control service. They are adding a special aeration treatment as a low-cost extra service option, which it hopes will help attract new customers. Management is planning to promote this new service in two media: radio and direct-mail advertising. A budget of $2000 is to be used on this promotional campaign over the next quarter. Based on past experience in promoting its other services, Lush Lawns has been able to obtain an estimate of the relationship between sales and the amount spent on promotion in these two media:
s = 2x12 - 10x22 - 2x1x2 + 18x1 + 34x2
s.t. x1 + x2 = 2
Solve.
s = 2x12 - 10x22 - 2x1x2 + 18x1 + 34x2
s.t. x1 + x2 = 2
Solve.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
43
Sara's Sensible Critters makes two kinds of catnip toys: balls (x1) and mice (x2). The relationship between demand and price for balls and mice is:
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Sara has found an unlimited source of catnip so that is no longer a constraint. However, customer demand dictates that she produce 2.5 times more catnip balls than mice. Write the new constraint.
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Sara has found an unlimited source of catnip so that is no longer a constraint. However, customer demand dictates that she produce 2.5 times more catnip balls than mice. Write the new constraint.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
44
Zoey's Catnip Toys faces the following relationship between price and demand: v = 2000 - 200p. The fixed cost is $500 and variable cost is $1. What price should Zoey charge to maximize profit?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
45
Sara's Sensible Critters makes two kinds of catnip toys: balls (x1) and mice (x2). The relationship between demand and price for balls and mice is:
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Sara has found an unlimited source of catnip so that is no longer a constraint. However, customer demand dictates that she produce 2.5 times more catnip balls than mice. How will this impact the prices that she should charge to maximize profit?
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Sara has found an unlimited source of catnip so that is no longer a constraint. However, customer demand dictates that she produce 2.5 times more catnip balls than mice. How will this impact the prices that she should charge to maximize profit?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
46
The Salt Creek Soap Company has determined the following nonlinear model to determine the optimal pounds of industrial soap (X1) and shampoo (X2) it should produce each day.
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-Determine the quantity of soap and shampoo that should be produced to maximize profit.
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-Determine the quantity of soap and shampoo that should be produced to maximize profit.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
47
If a firm's profit is Z = 12x - 6x2 + 30, and their minimum production level of x is equal to 0.5, then the level of x that maximizes profit is:
A) .5
B) 1
C) 1.5
D) 2
A) .5
B) 1
C) 1.5
D) 2

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
48
Sara's Sensible Critters makes two kinds of catnip toys: balls (x1) and mice (x2). The relationship between demand and price for balls and mice is:
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Write the formulation for this problem
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Write the formulation for this problem

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
49
The slope of a curve at its highest point equals:
A) 0
B) 1
C) 2
D) 3
A) 0
B) 1
C) 2
D) 3

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
50
A store has determined that the weekly sales of a product is related to the number of customers who visit the store and the square feet of shelf space, x, according to the following equation: -20x2 - 10C2 + 40Cx + 120x - 200. C represents the hundreds of customers who visit their store. If a store averages 200 customers per week, how many square feet of shelf space is required to maximize sales?
A) 3
B) 4
C) 5
D) 1 or 9
A) 3
B) 4
C) 5
D) 1 or 9

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
51
Zoey's Catnip Toys faces the following relationship between price and demand: v = 2000 - 200p. The fixed cost is $500 and variable cost is $1. Write an expression for the total profit.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
52
Consider the curve 7x2 - 14x + 28. What is the highest point on this curve?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
53
Sara's Sensible Critters makes two kinds of catnip toys: balls (x1) and mice (x2). The relationship between demand and price for balls and mice is:
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Determine the prices that Sara should charge to maximize profit.
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Determine the prices that Sara should charge to maximize profit.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
54
A store has determined that the weekly sales of a product is related to the number of customers who visit the store and the square feet of shelf space, x, according to the following equation: -20x2 - 10C2 + 40Cx + 120x - 200C + 600. C represents the hundreds of customers who visit their store. If a store averages 200 customers per week, how many square feet of shelf space is required to maximize sales?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
55
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation: 1000 - 8p.
What price for the ball bearings will maximize the profit?
What price for the ball bearings will maximize the profit?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
56
The Salt Creek Soap Company has determined the following nonlinear model to determine the optimal pounds of industrial soap (X1) and shampoo (X2) it should produce each day.
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-Determine the profit for the optimal production quantities of soap and shampoo.
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-Determine the profit for the optimal production quantities of soap and shampoo.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
57
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation: 1000 - 8p.
What is the optimal production quantity?
What is the optimal production quantity?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
58
Consider the curve 7x2 - 14x + 28. What is the slope at x = 5?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
59
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation: 1000 - 8p.
What is the optimal profit?
What is the optimal profit?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
60
Consider the curve 7x2 - 14x + 28. What is the second derivative at x = 10?

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
61
Sara's Sensible Critters makes two kinds of catnip toys: balls (x1) and mice (x2). The relationship between demand and price for balls and mice is:
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Write the appropriate expression for the demand constraint.
A) 2.5x1 = x2
B) x1 - 2.5x2 ? 0
C) x1 + 2.5x2 ? 0
D) x1 = 2.5x2
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Write the appropriate expression for the demand constraint.
A) 2.5x1 = x2
B) x1 - 2.5x2 ? 0
C) x1 + 2.5x2 ? 0
D) x1 = 2.5x2

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
62
The Salt Creek Soap Company has determined the following nonlinear model to determine the optimal pounds of industrial soap (X1) and shampoo (X2) it should produce each day.
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-What is profit when the optimal values of soap and shampoo are produced?
A) Z = 12
B) Z = 14
C) Z = 16
D) Z = 18
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-What is profit when the optimal values of soap and shampoo are produced?
A) Z = 12
B) Z = 14
C) Z = 16
D) Z = 18

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
63
The Lagrange multiplier is
A) the shadow price for the constraint coefficients.
B) valid over a range of changes in the RHS.
C) the rate of change in the objective value as the RHS of the constraint increases.
D) the minimum threshold for decision variables to enter the solution.
A) the shadow price for the constraint coefficients.
B) valid over a range of changes in the RHS.
C) the rate of change in the objective value as the RHS of the constraint increases.
D) the minimum threshold for decision variables to enter the solution.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
64
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation:
V = 1000 - 8p. What is the optimal price of the ball bearings that will maximize the profit?
A) $47.99
B) $53.99
C) $58.99
D) $63.99
V = 1000 - 8p. What is the optimal price of the ball bearings that will maximize the profit?
A) $47.99
B) $53.99
C) $58.99
D) $63.99

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
65
Both linear and nonlinear programming models are examples of
A) goal programming models.
B) simplex tableaus.
C) constrained likelihood models.
D) constrained optimization models.
A) goal programming models.
B) simplex tableaus.
C) constrained likelihood models.
D) constrained optimization models.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
66
Sara's Sensible Critters makes two kinds of catnip toys: balls (x1) and mice (x2). The relationship between demand and price for balls and mice is:
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Write the appropriate expression for profit.
A) Max Z = (p1 - 3)x1 + (p1 - 2)x2
B) Max Z = (p1 + 1)x1 + (p1 + 3)x2
C) Max Z = (p1 - 1)x1 + (p1 - 3)x2
D) Min Z = (p1 -1)x1 + (p1 - 3)x2
x1 = 1800 - 150p1
x2 = 1500 - 300p2
The cost for a catnip ball is $2 and for the mouse, $3.
Sara has only 200 ounces of catnip on hand. A ball uses a tenth of an ounce and a toy mouse uses one-quarter of an ounce.
-Write the appropriate expression for profit.
A) Max Z = (p1 - 3)x1 + (p1 - 2)x2
B) Max Z = (p1 + 1)x1 + (p1 + 3)x2
C) Max Z = (p1 - 1)x1 + (p1 - 3)x2
D) Min Z = (p1 -1)x1 + (p1 - 3)x2

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
67
The Salt Creek Soap Company has determined the following nonlinear model to determine the optimal pounds of industrial soap (X1) and shampoo (X2) it should produce each day.
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-What quantities of soap and shampoo maximize profit?
A) X1 = 0, X2 = 2
B) X1 = 1, X2 = 2
C) X1 = 2, X2 = 2
D) X1 = 2, X2 = 1
Maximize Z = X12 + 2X22 - 8X1 - 12X2 + 34
Subject to: X1 + 2X2 = 4 lbs
-What quantities of soap and shampoo maximize profit?
A) X1 = 0, X2 = 2
B) X1 = 1, X2 = 2
C) X1 = 2, X2 = 2
D) X1 = 2, X2 = 1

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
68
The Lagrange multiplier reflects the appropriate change in the objective function resulting from a unit change in the ________ of the constraint equation.
A) coefficient
B) objective function
C) right-hand side
D) shadow price
A) coefficient
B) objective function
C) right-hand side
D) shadow price

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
69
The Lagrange multiplier is ________ to the dual variables in a linear programming problem.
A) analogous
B) contradictory
C) inversely related
D) opposite
A) analogous
B) contradictory
C) inversely related
D) opposite

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
70
The derivative of a function ________ the slope of the curve defined by that function.
A) is larger than
B) equals
C) is smaller than
D) is similar to
A) is larger than
B) equals
C) is smaller than
D) is similar to

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
71
The XYZ manufacturing company produces ball bearings. The annual fixed cost is $20,000 and the variable cost per ball bearing is $3. The price is related to demand according to the following equation:
V = 1000 - 8p. What is optimal profit?
A) $6668
B) $7668
C) $8668
D) $9768
V = 1000 - 8p. What is optimal profit?
A) $6668
B) $7668
C) $8668
D) $9768

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
72
Classical optimization is the use of ________ to determine the optimal value of a variable.
A) calculus
B) linear programming
C) nonlinear programming
D) goal programming
A) calculus
B) linear programming
C) nonlinear programming
D) goal programming

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck



