Deck 11: Analysis of Variance

Question 1

Hartley's test measures the equality of the means for several groups.

Answer 1

False

Question 2

Tukey's test is not needed if we have the overall F statistic for the ANOVA.

Answer 2

False

Question 3

Analysis of variance is a procedure intended to compare the means of c samples.

Answer 3

True

Question 4

In a two-factor ANOVA with three columns and four rows,there can be more than two interaction effects.

Question 5

One-factor ANOVA with two groups is equivalent to a two-tailed t-test.

Question 6

Hartley's test is the largest sample mean divided by the smallest sample mean.

Question 7

Three-factor ANOVA is required if we have three treatment groups (i.e. ,three data columns).

Question 8

One factor ANOVA stacked data for five groups will be arranged in five separate columns.

Question 9

Sample sizes must be equal in one-factor ANOVA.

Question 10

ANOVA assumes equal variances within each treatment group.

Question 11

Tukey's test compares pairs of treatment means in an ANOVA.

Question 12

Hartley's test is to check for unequal variances for c groups.

Question 13

ANOVA assumes normal populations.

Question 14

Interaction plots that show crossing lines indicate likely interactions.

Question 15

If you have four factors (call them A,B,C,and D)in an ANOVA experiment with replication,you could have a maximum of four different two-factor interactions.

Question 16

Interaction plots that show parallel lines would suggest interaction effects.

Question 17

One-factor ANOVA is a procedure intended to compare the variances of c samples.

Question 18

Comparison of c means in one-factor ANOVA can equivalently be done by using c individual t-tests on c pairs of means at the same α.

Question 19

In a 3×4 randomized block (two-factor unreplicated)ANOVA,we have 12 treatment groups.

Question 20

Tukey's test is similar to a two-sample t-test except that it pools the variances for all c samples.

Question 21

Which of the following is not a characteristic of the F distribution?

A) It is always right-skewed.
B) It describes the ratio of two variances.
C) It is a family based on two sets of degrees of freedom.
D) It is negative when s₁² is smaller than s₂².

Question 22

Analysis of variance is a technique used to test for:

A) equality of two or more variances.
B) equality of two or more means.
C) equality of a population mean and a given value.
D) equality of more than two variances.

Question 23

ANOVA is robust to violations of the equal-variance assumption as long as group sizes are equal.

Question 24

Tukey's test for five groups would require 10 comparisons of means.

Question 25

Tukey's test pools all the sample variances.

Question 26

It is desirable,but not necessary,that sample sizes be equal in a one-factor ANOVA.

Question 27

In an ANOVA,when would the F-test statistic be zero?

A) When there is no difference in the variances.
B) When the treatment means are the same.
C) When the observations are normally distributed.
D) The F-test statistic cannot ever be zero.

Question 28

Degrees of freedom for the between-group variation in a one-factor ANOVA with n₁ = 8,n₂ = 5,n₃ = 7,n₄ = 9 would be:

A) 28.
B) 3.
C) 29.

Question 29

Levene's test for homogeneity of variance is attractive because it does not depend on the assumption of normality.

Question 30

ANOVA is used to compare:

A) proportions of several groups.
B) variances of several groups.
C) means of several groups.
D) both means and variances.

Question 31

Which is not assumed in ANOVA?

A) Observations are independent.
B) Populations are normally distributed.
C) Variances of all treatment groups are the same.
D) Population variances are known.

Question 32

To test the null hypothesis H₀: μ₁ = μ₂ = μ₃ using samples from normal populations with unknown but equal variances,we:

A) cannot safely use ANOVA.
B) can safely employ ANOVA.
C) would prefer three separate t-tests.
D) would need three-factor ANOVA.

Question 33

In a one-factor ANOVA,the computed value of F will be negative:

A) when there is no difference in the treatment means.
B) when there is no difference within the treatments.
C) when the SST (total)is larger than SSE (error).
D) under no circumstances.

Question 34

Using one-factor ANOVA with 30 observations we find at α = .05 that we cannot reject the null hypothesis of equal means.We increase the sample size from 30 observations to 60 observations and obtain the same value for the sample F-test statistic.Which is correct?

A) We might now be able to reject the null hypothesis.
B) We surely must reject H₀ for 60 observations.
C) We cannot reject H₀ since we obtained the same F-value.
D) It is impossible to get the same F-value for n = 60 as for n = 30.

Question 35

Which is the Excel function to find the critical value of F for α = .05,df₁ = 3,df₂ = 25?

A) =F.DIST(.05,2,24)
B) =F.INV.RT(.05,3,25)
C) =F.DIST(.05,3,25)
D) =F.INV(.05,2,24)

Question 36

Tukey's test with seven groups would entail 21 comparisons of means.

Question 37

In an ANOVA,the SSE (error)sum of squares reflects:

A) the effect of the combined factor(s).
B) the overall variation in Y that is to be explained.
C) the variation that is not explained by the factors.
D) the combined effect of treatments and sample size.

Question 38

Which is not an assumption of ANOVA?

A) Normality of the treatment populations.
B) Homogeneous treatment variances.
C) Independent sample observations.
D) Equal population sizes for groups.

Question 39

Which Excel function gives the right-tail p-value for an ANOVA test with a test statistic F_calc = 4.52,n = 29 observations,and c = 4 groups?

A) =F.DIST.RT(4.52,3,25)
B) =F.INV(4.52,4,28)
C) =F.DIST(4.52,4,28)
D) =F.INV(4.52,3,25)

Question 40

Variation "within" the ANOVA treatments represents:

A) random variation.
B) differences between group means.
C) differences between group variances.
D) the effect of sample size.

Question 41

Refer to the following partial ANOVA results from Excel (some information is missing). 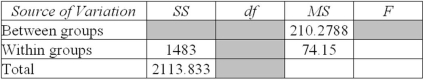The F-test statistic is:

A) 2.84.
B) 3.56.
C) 2.80.
D) 2.79.

Question 42

One-factor analysis of variance:

A) requires that the number of observations in each group be identical.
B) has less power when the number of observations per group is not identical.
C) is extremely sensitive to slight departures from normality.
D) is a generalization of the t-test for paired observations.

Question 43

Refer to the following partial ANOVA results from Excel (some information is missing). 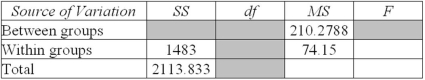The sample size is:

A) 20.
B) 23.
C) 24.

Question 44

In a one-factor ANOVA,the total sum of squares is equal to:

A) the sum of squares within groups plus the sum of squares between groups.
B) the sum of squares within groups times the sum of squares between groups.
C) the sum of squares within groups divided by the sum of squares between groups.
D) the means of all the groups squared.

Question 45

The within-treatment variation reflects:

A) variation among individuals of the same group.
B) variation between individuals in different groups.
C) variation explained by factors included in the ANOVA model.
D) variation that is not part of the ANOVA model.

Question 46

The Internal Revenue Service wishes to study the time required to process tax returns in three regional centers.A random sample of three tax returns is chosen from each of three centers.The time (in days)required to process each return is recorded as shown below. 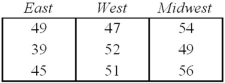The test to use to compare the means for all three groups would require:

A) three-factor ANOVA.
B) one-factor ANOVA.
C) repeated two-sample test of means.
D) two-factor ANOVA with replication.

Question 47

The Internal Revenue Service wishes to study the time required to process tax returns in three regional centers.A random sample of three tax returns is chosen from each of three centers.The time (in days)required to process each return is recorded as shown below. 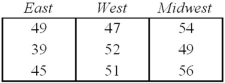Degrees of freedom for the between-groups sum of squares in the ANOVA would be:

A) 11.
B) 2.
C) 4.

Question 48

Refer to the following partial ANOVA results from Excel (some information is missing). 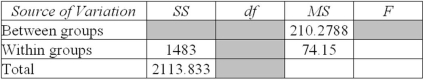Degrees of freedom for the F-test are:

A) 5,22.
B) 4,21.
C) 3,20.
D) impossible to determine.

Question 49

Systolic blood pressure of randomly selected HMO patients was recorded on a particular Wednesday,with the results shown here: 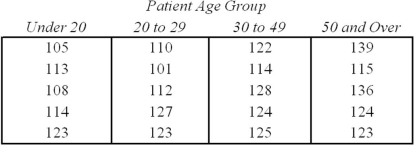The appropriate hypothesis test is:

A) one-factor ANOVA.
B) two-factor ANOVA.
C) three-factor ANOVA.
D) four-factor ANOVA.

Question 50

Prof.Gristmill sampled exam scores for five randomly chosen students from each of his two sections of ACC 200.His sample results are shown. He could test the population means for equality using:

A) a t-test for two means from independent samples.
B) a t-test for two means from paired (related)samples.
C) a one-factor ANOVA.
D) either a one-factor ANOVA or a two-tailed t-test.

Question 51

For this one-factor ANOVA (some information is missing),what is the F-test statistic?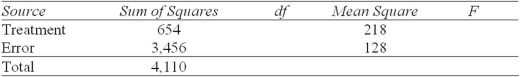

A) 0.159
B) 2.833
C) 1.703
D) Cannot be determined

Question 52

Identify the degrees of freedom for the treatment and error in this one-factor ANOVA (blanks indicate missing information).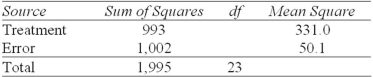

A) 4,24
B) 3,20
C) 5,23

Question 53

For this one-factor ANOVA (some information is missing),how many treatment groups were there?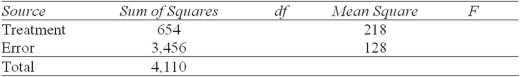

A) Cannot be determined
B) 3
C) 4
D) 2

Question 54

Refer to the following partial ANOVA results from Excel (some information is missing). 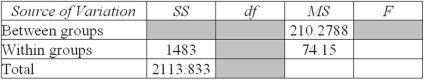Degrees of freedom for between groups variation are:

A) 3.
B) 4.
C) 5.
D) Can't tell from given information.

Question 55

Refer to the following partial ANOVA results from Excel (some information is missing). At α = 0.05,the difference between group means is:

A) highly significant.
B) barely significant.
C) not quite significant.
D) clearly insignificant.

Question 56

Refer to the following partial ANOVA results from Excel (some information is missing). 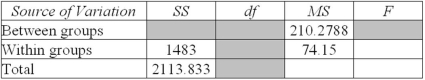SS for between groups variation will be:

A) 129.99.
B) 630.83.
C) 1233.4.
D) Can't tell.

Question 57

Refer to the following partial ANOVA results from Excel (some information is missing). 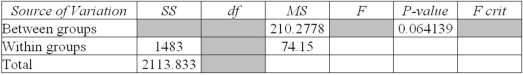The critical value of F at α = 0.05 is:

A) 1.645.
B) 2.84.
C) 3.10.
D) 4.28.

Question 58

Refer to the following partial ANOVA results from Excel (some information is missing). 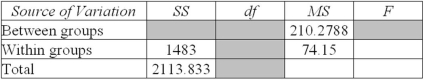The number of treatment groups is:

A) 4.
B) 3.
C) 2.
D)

Question 59

Given the following ANOVA table (some information is missing),find the critical value of F_.05.

A) 3.06
B) 2.90
C) 2.36
D) 3.41

Question 60

Given the following ANOVA table (some information is missing),find the F statistic.

A) 3.71
B) 0.99
C) 0.497
D) 4.02

Question 61

Refer to the following partial ANOVA results from Excel (some information is missing). ANOVA Table 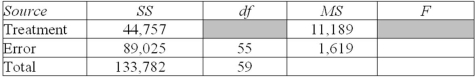The number of treatment groups is:

A) 5.
B) 4.
C) 3.
D) impossible to ascertain from given.

Question 62

Refer to the following partial ANOVA results from Excel (some information is missing). 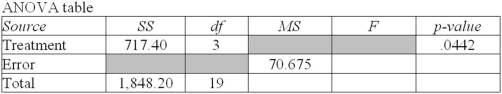The number of observations in the entire sample is:

A) 20.
B) 19.
C)

Question 63

Systolic blood pressure of randomly selected HMO patients was recorded on a particular Wednesday,with the results shown here.An ANOVA test was performed using these data. 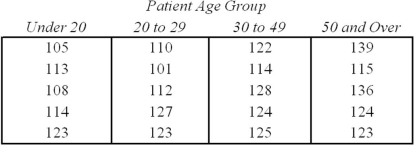What are the degrees of freedom for the error sum of squares?

A) 3
B) 19
C) 16
D) It depends on α.

Question 64

Refer to the following partial ANOVA results from Excel (some information is missing). ANOVA Table 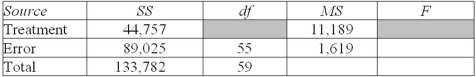The number of observations in the original sample was:

A) 59.
B) 60.
C) 58.

Question 65

Refer to the following partial ANOVA results from Excel (some information is missing). 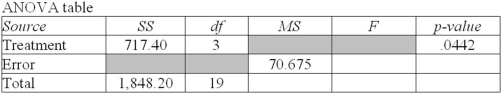The MS (mean square)for the treatments is:

A) 239.13.
B) 106.88.
C) 1,130.8.
D) impossible to ascertain from the information given.

Question 66

To compare the cost of three shipping methods,a random sample of four shipments is taken for each of three firms.The cost per shipment is shown below. 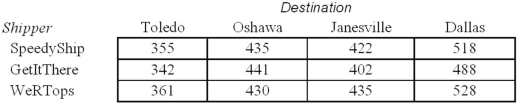In a one-factor ANOVA,degrees of freedom for the between-groups sum of squares will be:

A) 11.
B) 3.
C) 2.

Question 67

Refer to the following partial ANOVA results from Excel (some information is missing). 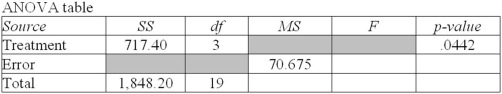Our decision about the hypothesis of equal treatment means is that the null hypothesis:

A) cannot be rejected at α = .05.
B) can be rejected at α = .05.
C) can be rejected for any typical value of α.
D) cannot be assessed from the given information.

Question 68

Refer to the following partial ANOVA results from Excel (some information is missing). 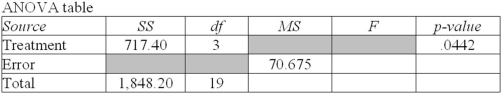The F statistic is:

A) 4.87.
B) 3.38.
C) 5.93.
D) 6.91.

Question 69

Systolic blood pressure of randomly selected HMO patients was recorded on a particular Wednesday,with the results shown here.An ANOVA test was performed using these data. 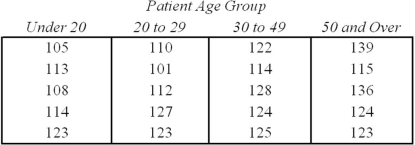Degrees of freedom for the between-treatments sum of squares would be:

A) 3.
B) 19.
C) 17.
D) depends on α.

Question 70

To compare the cost of three shipping methods,a random sample of four shipments is taken for each of three firms.The cost per shipment is shown below. 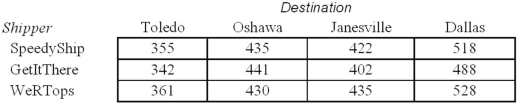In a one-factor ANOVA,degrees of freedom for the within-groups sum of squares will be:

A) 11.
B) 3.
C) 9.

Question 71

Refer to the following partial ANOVA results from Excel (some information is missing). ANOVA Table 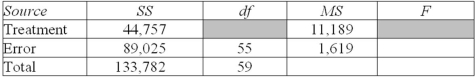The F statistic is:

A) 2.88.
B) 4.87.
C) 5.93.
D) 6.91.

Question 72

What is the .05 critical value of Tukey's test statistic for a one-factor ANOVA with n₁ = 6,n₂ = 6,n₃ = 6? Note: This question requires access to a Tukey table.

A) 3.67
B) 2.60
C) 3.58
D) 2.75

Question 73

Refer to the following MegaStat output (some information is missing).The sample size was n = 65 in a one-factor ANOVA. 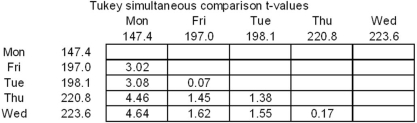At α = .05,which is the critical value of the test statistic for a two-tailed test for a significant difference in means that are to be compared simultaneously? Note: This question requires a Tukey table.

A) 2.81
B) 2.54
C) 2.33
D) 1.96

Question 74

Refer to the following MegaStat output (some information is missing).The sample size was n = 24 in a one-factor ANOVA. 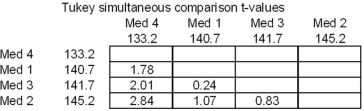At α = .05,what is the critical value of the Tukey test statistic for a two-tailed test for a significant difference in means that are to be compared simultaneously? Note: This question requires access to a Tukey table.

A) 2.07
B) 2.80
C) 2.76
D) 1.96

Question 75

Refer to the following MegaStat output (some information is missing).The sample size was n = 65 in a one-factor ANOVA. 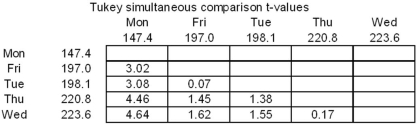Which pairs of days differ significantly? Note: This question requires access to a Tukey table.

A) (Mon,Thu)and (Mon,Wed)only.
B) (Mon,Wed)only.
C) (Mon,Thu)only.
D) (Mon,Thu)and (Mon,Wed)and (Mon,Fri)and (Mon,Tue).

Question 76

What is the .05 critical value of Hartley's test statistic for a one-factor ANOVA with n₁ = 5,n₂ = 8,n₃ = 7,n₄ = 8,n₅ = 6,n₆ = 8? Note: This question requires access to a Hartley table.

A) 10.8
B) 11.8
C) 13.7
D) 15.0

Question 77

Sound levels are measured at random moments under typical driving conditions for various full-size truck models.The ANOVA results are shown below. 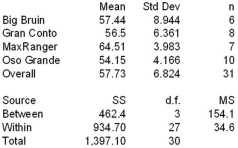The test statistic for Hartley's test for homogeneity of variance is:

A) 2.25.
B) 5.04.
C) 4.61.
D) 4.45.

Question 78

Refer to the following MegaStat output (some information is missing).The sample size was n = 24 in a one-factor ANOVA. 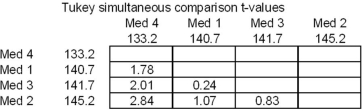Which pairs of meds differ at α = .05? Note: This question requires access to a Tukey table.

A) Med 1,Med 2
B) Med 2,Med 4
C) Med 3,Med 4
D) None of them.

Question 79

Sound levels are measured at random moments under typical driving conditions for various full-size truck models.The Excel ANOVA results are shown below. 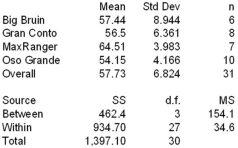The test statistic to compare the five means simultaneously is:

A) 2.96.
B) 15.8.
C) 5.56.
D) 4.45.

Question 80

To compare the cost of three shipping methods,a random sample of four shipments is taken for each of three firms.The cost per shipment is shown below. 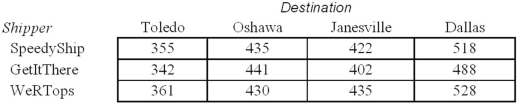Degrees of freedom for the total sum of squares in a one-factor ANOVA would be:

A) 11.
B) 8.
C) 2.