Deck 14: Section 3: Multiple Integration
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/20
Play
Full screen (f)
Deck 14: Section 3: Multiple Integration
1
Evaluate the double integral below. 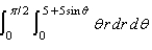
A)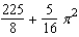
B)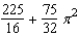
C)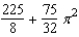
D)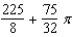
E)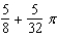
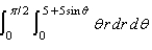
A)
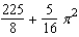
B)
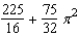
C)
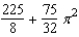
D)
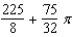
E)
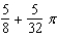
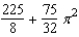
2
Use a double integral in polar coordinates to find the volume of the solid inside the hemisphere 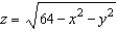 but outside the cylinder
but outside the cylinder 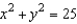 .
.
A)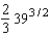
B)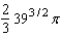
C)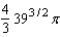
D)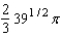
E)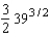
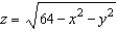 but outside the cylinder
but outside the cylinder 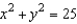 .
.A)
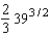
B)
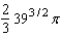
C)
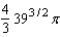
D)
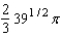
E)
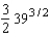
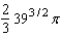
3
Evaluate the following iterated integral by converting to polar coordinates. 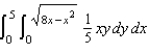
A)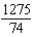
B)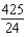
C)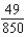
D)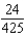
E)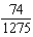
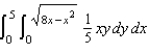
A)
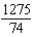
B)
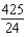
C)
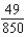
D)
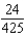
E)
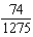
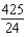
4
Use polar coordinates to describe the region as shown in the figure below: 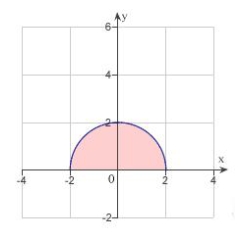
A)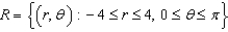
B)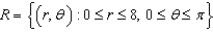
C)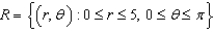
D)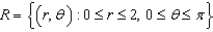
E)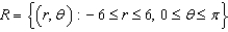

A)
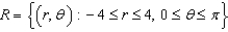
B)
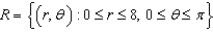
C)
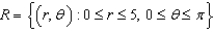
D)
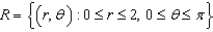
E)
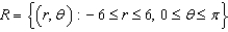

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
5
Determine the diameter of a hole that is drilled vertically through the center of the solid bounded by the graphs of the equations 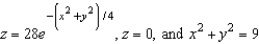 if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
A) 1.2245
B) 31.4490
C) 7.2245
D) 5.4490
E) 15.2245
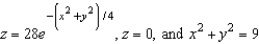 if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.A) 1.2245
B) 31.4490
C) 7.2245
D) 5.4490
E) 15.2245

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
6
Evaluate the double integral below. 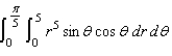
A)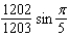
B)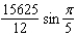
C)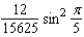
D)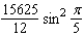
E)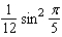
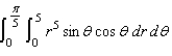
A)
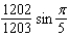
B)
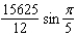
C)
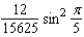
D)
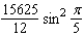
E)
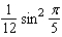

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
7
Given 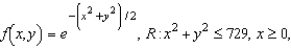 use polar coordinates to set up and evaluate the double integral
use polar coordinates to set up and evaluate the double integral 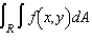 .
.
A)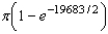
B)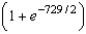
C)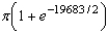
D)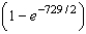
E)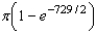
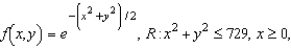 use polar coordinates to set up and evaluate the double integral
use polar coordinates to set up and evaluate the double integral 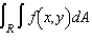 .
.A)
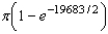
B)
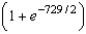
C)
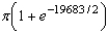
D)
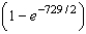
E)
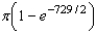

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose the population density of a city is approximated by the model 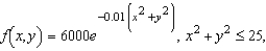 where x and y are measured in miles. Integrate the density function over the indicated circular region to approximate the population of the city. Round your answer to the nearest integer.
where x and y are measured in miles. Integrate the density function over the indicated circular region to approximate the population of the city. Round your answer to the nearest integer.
A) 417,127
B) 417,029
C) 833,901
D) 833,903
E) 416,951
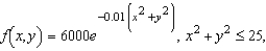 where x and y are measured in miles. Integrate the density function over the indicated circular region to approximate the population of the city. Round your answer to the nearest integer.
where x and y are measured in miles. Integrate the density function over the indicated circular region to approximate the population of the city. Round your answer to the nearest integer.A) 417,127
B) 417,029
C) 833,901
D) 833,903
E) 416,951

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
9
Combine the sum of the two iterated integrals into a single integral by converting to polar coordinates. Evaluate the resulting iterated integral. 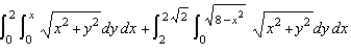
A)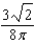
B)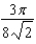
C)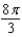
D)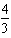
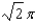
E)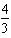
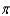
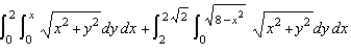
A)
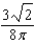
B)
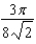
C)
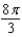
D)
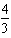
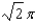
E)
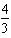
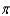

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
10
Use a double integral to find the area of the region inside the circle 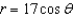 and outside the cardioid
and outside the cardioid 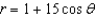 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A) 46.68
B) 58.34
C) 20.34
D) 55.34
E) 22.34
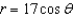 and outside the cardioid
and outside the cardioid 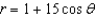 . Round your answer to two decimal places.
. Round your answer to two decimal places.A) 46.68
B) 58.34
C) 20.34
D) 55.34
E) 22.34

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
11
Use a double integral in polar coordinates to find the volume of the solid in the first octant bounded by the graphs of the equations given below. 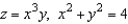
A)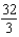
B)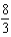
C)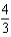
D)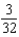
E)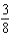
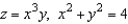
A)
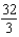
B)
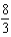
C)
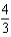
D)
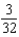
E)
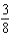

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
12
Find a such that the volume inside the hemisphere 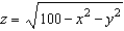 and outside the cylinder
and outside the cylinder 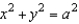 is one-half the volume of the hemisphere. Round your answer to four decimal places.
is one-half the volume of the hemisphere. Round your answer to four decimal places.
A)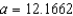
B)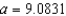
C)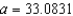
D)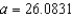
E)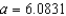
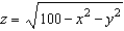 and outside the cylinder
and outside the cylinder 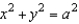 is one-half the volume of the hemisphere. Round your answer to four decimal places.
is one-half the volume of the hemisphere. Round your answer to four decimal places.A)
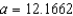
B)
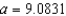
C)
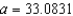
D)
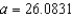
E)
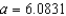

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
13
Identify the region of integration for the following integral. 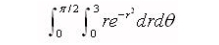
A)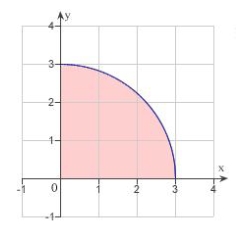
B)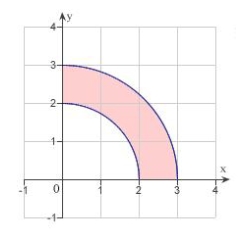
C)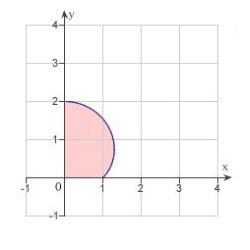
D)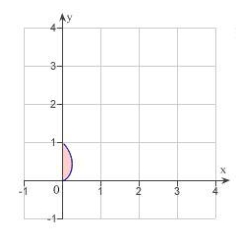
E)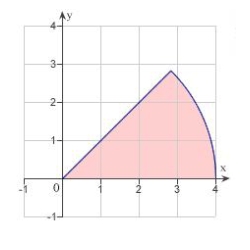
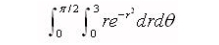
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
14
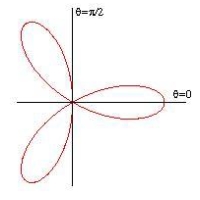
Use a double integral to find the area enclosed by the graph of 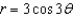 .
. 
A)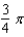
B)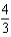
C)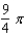
D)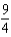
E)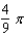
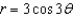 .
. 
A)
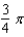
B)
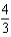
C)
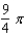
D)
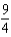
E)
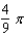

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
15
Evaluate the iterated integral 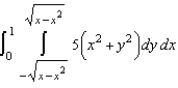 by converting to polar coordinates.
by converting to polar coordinates.
A)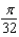
B)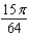
C)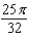
D)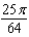
E)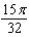
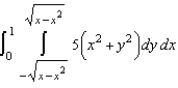 by converting to polar coordinates.
by converting to polar coordinates.A)
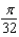
B)
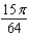
C)
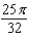
D)
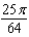
E)
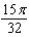

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
16
Evaluate the following iterated integral by converting to polar coordinates. 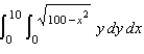
A)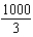
B)
C)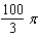
D)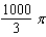
E)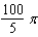
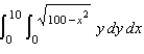
A)
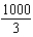
B)

C)
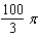
D)
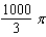
E)
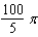

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
17
Evaluate the iterated integral 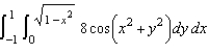 by converting to polar coordinates. Round your answer to four decimal places.
by converting to polar coordinates. Round your answer to four decimal places.
A) 10.5742
B) 13.5742
C) 17.5742
D) 28.5742
E) 14.5742
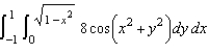 by converting to polar coordinates. Round your answer to four decimal places.
by converting to polar coordinates. Round your answer to four decimal places.A) 10.5742
B) 13.5742
C) 17.5742
D) 28.5742
E) 14.5742

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
18
Evaluate the double integral below. 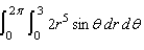
A)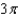
B)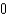
C)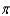
D)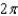
E)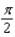
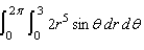
A)
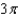
B)
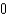
C)
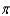
D)
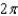
E)
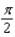

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
19
Use a double integral to find the area of the shaded region as shown in the figure below. 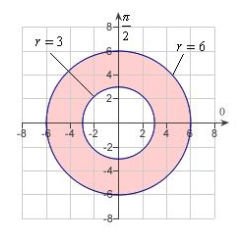
A)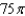
B)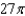
C)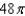
D)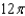
E)

A)
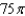
B)
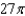
C)
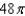
D)
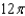
E)


Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
20
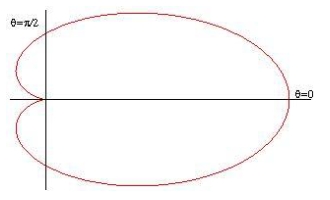
Use a double integral to find the area enclosed by the graph of 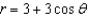 .
. 
A)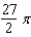
B)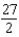
C)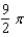
D)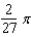
E)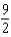
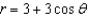 .
. 
A)
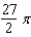
B)
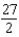
C)
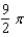
D)
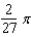
E)
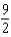

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck



