Deck 15: Section 4: Vector Analysis
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/18
Play
Full screen (f)
Deck 15: Section 4: Vector Analysis
1
Find the maximum value of 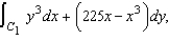 where C is any closed curve in the xy-plane, oriented counterclockwise.
where C is any closed curve in the xy-plane, oriented counterclockwise.
A)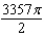
B)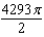
C)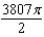
D)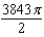
E)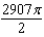
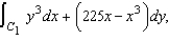 where C is any closed curve in the xy-plane, oriented counterclockwise.
where C is any closed curve in the xy-plane, oriented counterclockwise.A)
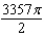
B)
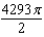
C)
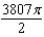
D)
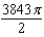
E)
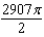
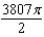
2
Use Green's Theorem to calculate the work done by the force 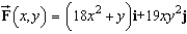 on a particle that is moving counterclockwise around the closed path C where C is the boundary of the region lying between the graphs of
on a particle that is moving counterclockwise around the closed path C where C is the boundary of the region lying between the graphs of 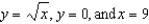 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A)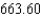
B)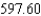
C)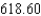
D)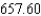
E)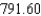
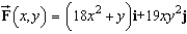 on a particle that is moving counterclockwise around the closed path C where C is the boundary of the region lying between the graphs of
on a particle that is moving counterclockwise around the closed path C where C is the boundary of the region lying between the graphs of 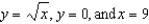 . Round your answer to two decimal places.
. Round your answer to two decimal places.A)
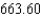
B)
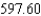
C)
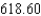
D)
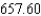
E)
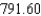
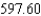
3
Set up and evaluate a line integral to find the area of the region R bounded by the graph of 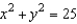 .
.
A)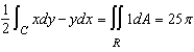 where
where 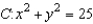
B)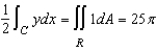 where
where 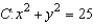
C)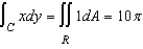 where
where 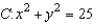
D)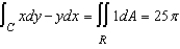 where
where 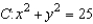
E)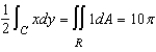 where
where 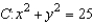
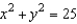 .
.A)
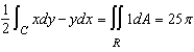 where
where 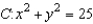
B)
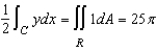 where
where 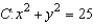
C)
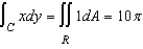 where
where 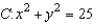
D)
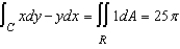 where
where 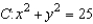
E)
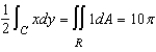 where
where 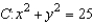
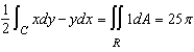 where
where 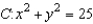
4
Use a computer algebra system and the result "The centroid of the region having area A bounded by the simple closed path C is 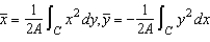 " to find the centroid of the region bounded by the graphs of
" to find the centroid of the region bounded by the graphs of 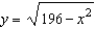 and
and 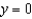 .
.
A)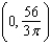
B)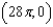
C)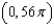
D)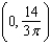
E)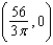
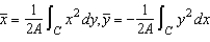 " to find the centroid of the region bounded by the graphs of
" to find the centroid of the region bounded by the graphs of 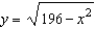 and
and 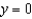 .
.A)
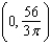
B)
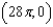
C)
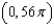
D)
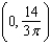
E)
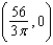

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
5
Use Green's Theorem to calculate the work done by the force 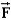 on a particle that is moving counterclockwise around the closed path C.
on a particle that is moving counterclockwise around the closed path C. 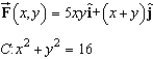
A)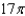
B)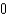
C)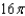
D)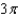
E)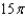
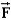 on a particle that is moving counterclockwise around the closed path C.
on a particle that is moving counterclockwise around the closed path C. 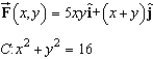
A)
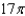
B)
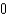
C)
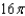
D)
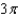
E)
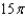

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
6
Use Green's Theorem to evaluate the line integral 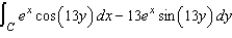 where C is
where C is 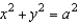 .
.
A)
B)
C)
D)
E)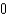
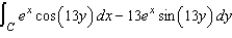 where C is
where C is 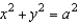 .
.A)
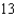
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
7
Use Green's Theorem to evaluate the integral 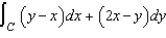 where C is the boundary of the region lying inside the rectangle bounded by
where C is the boundary of the region lying inside the rectangle bounded by 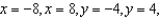 and outside the square bounded by
and outside the square bounded by 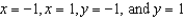 .
.
A)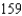
B)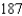
C)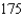
D)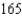
E)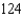
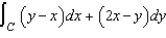 where C is the boundary of the region lying inside the rectangle bounded by
where C is the boundary of the region lying inside the rectangle bounded by 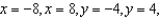 and outside the square bounded by
and outside the square bounded by 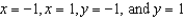 .
.A)
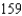
B)
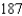
C)
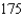
D)
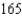
E)
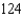

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
8
Use Green's Theorem to evaluate the line integral 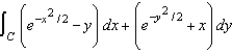 where C is the boundary of the region lying between the graphs of the circle
where C is the boundary of the region lying between the graphs of the circle 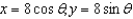 and the ellipse
and the ellipse 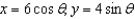 .
.
A)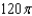
B)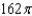
C)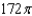
D)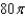
E)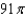
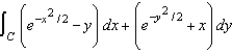 where C is the boundary of the region lying between the graphs of the circle
where C is the boundary of the region lying between the graphs of the circle 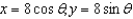 and the ellipse
and the ellipse 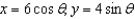 .
.A)
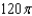
B)
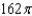
C)
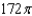
D)
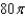
E)
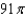

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
9
Use Green's Theorem to evaluate the integral 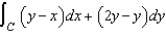 for the path C defined as
for the path C defined as 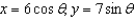 .
.
A)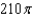
B)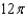
C)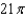
D)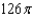
E)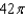
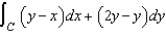 for the path C defined as
for the path C defined as 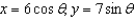 .
.A)
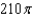
B)
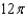
C)
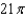
D)
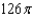
E)
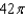

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
10
Use Green's Theorem to evaluate the integral 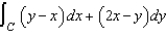 for the path C: boundary of the region lying between the graphs of y = x and y =
for the path C: boundary of the region lying between the graphs of y = x and y = 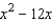 .
.
A)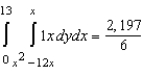
B)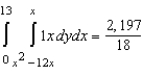
C)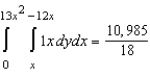
D)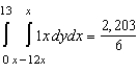
E)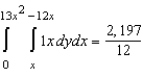
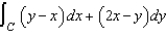 for the path C: boundary of the region lying between the graphs of y = x and y =
for the path C: boundary of the region lying between the graphs of y = x and y = 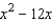 .
.A)
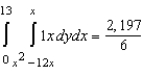
B)
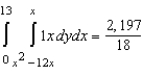
C)
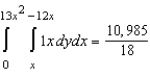
D)
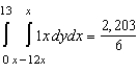
E)
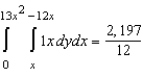

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
11
Use Green's Theorem to evaluate the integral 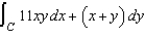 for the path C: boundary of the region lying between the graphs of
for the path C: boundary of the region lying between the graphs of 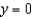 and
and 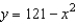 .
.
A)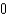
B)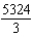
C)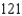
D)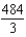
E)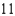
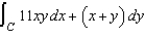 for the path C: boundary of the region lying between the graphs of
for the path C: boundary of the region lying between the graphs of 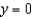 and
and 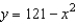 .
.A)
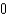
B)
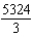
C)
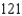
D)
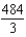
E)
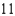

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
12
Use a computer algebra system and the result "The area of a plane region bounded by the simple closed path C given in polar coordinates is 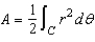 " to find the area of the region bounded by the graphs of the polar equation
" to find the area of the region bounded by the graphs of the polar equation 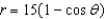 .
.
A)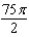
B)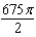
C)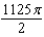
D)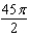
E)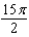
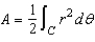 " to find the area of the region bounded by the graphs of the polar equation
" to find the area of the region bounded by the graphs of the polar equation 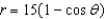 .
.A)
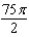
B)
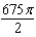
C)
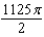
D)
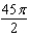
E)
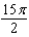

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
13
Evaluate 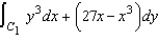 , where
, where 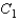 is the unit circle given by
is the unit circle given by 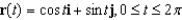 .
.
A)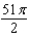
B)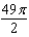
C)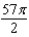
D)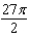
E)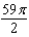
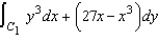 , where
, where  is the unit circle given by
is the unit circle given by 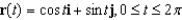 .
.A)
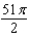
B)
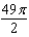
C)
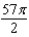
D)
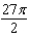
E)
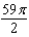

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
14
Use a computer algebra system and the result "The area of a plane region bounded by the simple closed path C given in polar coordinates is 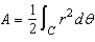 " to find the area of the region bounded by the graphs of the polar equation
" to find the area of the region bounded by the graphs of the polar equation 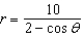 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A)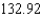
B)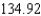
C)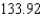
D)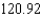
E)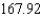
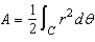 " to find the area of the region bounded by the graphs of the polar equation
" to find the area of the region bounded by the graphs of the polar equation 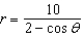 . Round your answer to two decimal places.
. Round your answer to two decimal places.A)
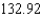
B)
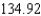
C)
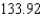
D)
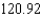
E)
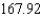

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
15
Verify Green's Theorem by evaluating both integrals 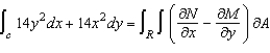 for the path C defined as the boundary of the region lying between the graphs of
for the path C defined as the boundary of the region lying between the graphs of 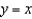 and
and 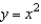 .
.
A)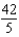
B)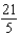
C)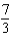
D)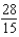
E)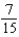
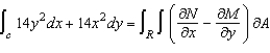 for the path C defined as the boundary of the region lying between the graphs of
for the path C defined as the boundary of the region lying between the graphs of 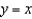 and
and 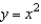 .
.A)
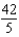
B)
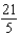
C)
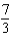
D)
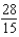
E)
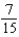

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
16
Verify Green's Theorem by setting up and evaluating both integrals 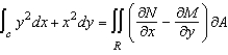 for the path C: square with vertices (0,0), (10,0), (10,10), (0,10).
for the path C: square with vertices (0,0), (10,0), (10,10), (0,10).
A)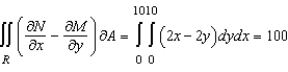
B)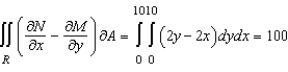
C)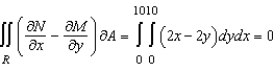
D)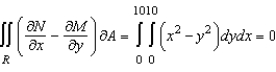
E)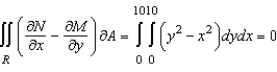
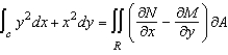 for the path C: square with vertices (0,0), (10,0), (10,10), (0,10).
for the path C: square with vertices (0,0), (10,0), (10,10), (0,10).A)
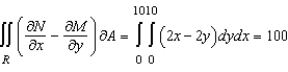
B)
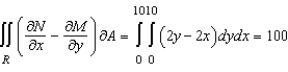
C)
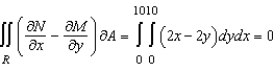
D)
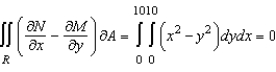
E)
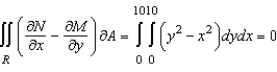

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
17
Use Green's Theorem to evaluate the integral 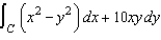 for the path C:
for the path C: 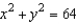 .
.
A)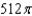
B)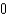
C)
D)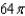
E)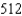
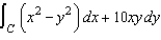 for the path C:
for the path C: 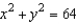 .
.A)
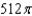
B)
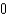
C)

D)
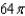
E)
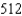

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck
18
Use Green's Theorem to evaluate the integral 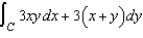 for C: boundary of the region lying between the graphs of
for C: boundary of the region lying between the graphs of 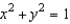 and
and 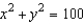 .
.
A)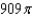
B)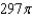
C)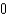
D)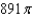
E)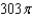
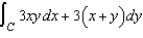 for C: boundary of the region lying between the graphs of
for C: boundary of the region lying between the graphs of 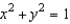 and
and 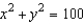 .
.A)
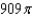
B)
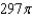
C)
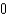
D)
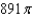
E)
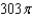

Unlock Deck
Unlock for access to all 18 flashcards in this deck.
Unlock Deck
k this deck



