Deck 13: Section 3: Functions of Several Variables
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/23
Play
Full screen (f)
Deck 13: Section 3: Functions of Several Variables
1
Find the first partial derivative for the function 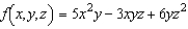 with respect to z.
with respect to z.
A)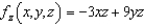
B)
C)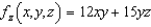
D)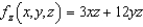
E)
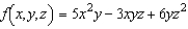 with respect to z.
with respect to z.A)
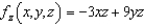
B)

C)
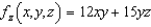
D)
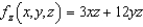
E)


2
For 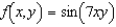 , evaluate
, evaluate 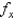 at the point
at the point 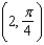 .
.
A)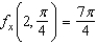
B)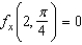
C)
D)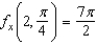
E)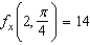
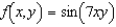 , evaluate
, evaluate 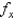 at the point
at the point 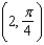 .
.A)
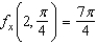
B)
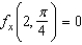
C)

D)
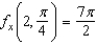
E)
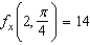
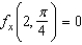
3
Suppose a pharmaceutical corporation has two plants that produce the same over-the-counter medicine. If 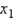 and
and 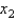 are the numbers of units produced at plant 1 and plant 2, respectively, then the total revenue for the product is given by
are the numbers of units produced at plant 1 and plant 2, respectively, then the total revenue for the product is given by 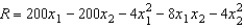 . When
. When 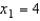 and
and 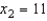 find the marginal revenue
find the marginal revenue 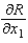 for plant 1.
for plant 1.
A) 174
B) 172
C) 80
D) 124
E) 93
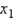 and
and 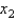 are the numbers of units produced at plant 1 and plant 2, respectively, then the total revenue for the product is given by
are the numbers of units produced at plant 1 and plant 2, respectively, then the total revenue for the product is given by 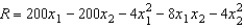 . When
. When 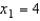 and
and 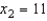 find the marginal revenue
find the marginal revenue 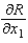 for plant 1.
for plant 1.A) 174
B) 172
C) 80
D) 124
E) 93
80
4
For 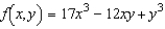 find all values of x and y such that
find all values of x and y such that 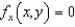 and
and 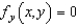 simultaneously.
simultaneously.
A)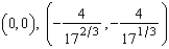
B)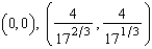
C)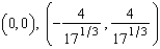
D)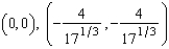
E)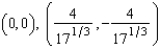
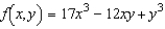 find all values of x and y such that
find all values of x and y such that 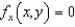 and
and 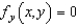 simultaneously.
simultaneously.A)
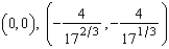
B)
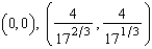
C)
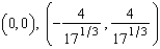
D)
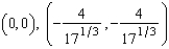
E)
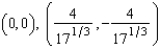

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
5
Find the partial derivative 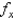 for the function
for the function 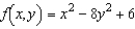 .
.
A)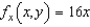
B)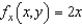
C)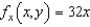
D)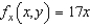
E)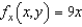
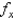 for the function
for the function 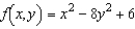 .
.A)
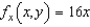
B)
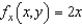
C)
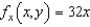
D)
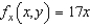
E)
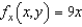

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
6
Suppose a company manufactures two types of wood-burning stoves: a freestanding model and a fireplace-insert model. The cost function for producing x freestanding and y fireplace-insert stoves is 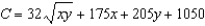 . Find the marginal costs
. Find the marginal costs 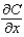 when
when 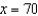 and
and 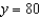 . Round your answer to the nearest integer.
. Round your answer to the nearest integer.
A) 192
B) 234
C) 210
D) 402
E) 403
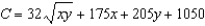 . Find the marginal costs
. Find the marginal costs 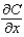 when
when 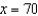 and
and 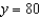 . Round your answer to the nearest integer.
. Round your answer to the nearest integer.A) 192
B) 234
C) 210
D) 402
E) 403

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
7
Find the partial derivative 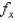 for the function
for the function 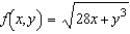 .
.
A)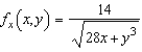
B)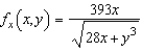
C)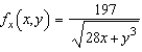
D)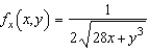
E)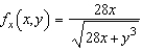
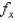 for the function
for the function 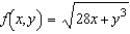 .
.A)
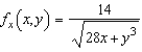
B)
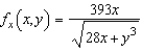
C)
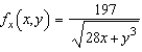
D)
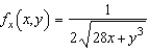
E)
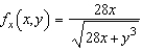

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
8
For 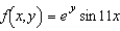 , evaluate
, evaluate 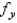 at the point
at the point 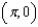 .
.
A)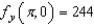
B)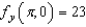
C)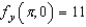
D)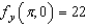
E)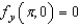
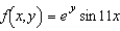 , evaluate
, evaluate 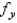 at the point
at the point 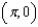 .
.A)
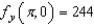
B)
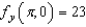
C)
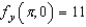
D)
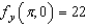
E)
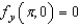

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
9
For 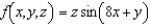 , evaluate
, evaluate 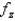 at the point
at the point 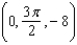 .
.
A)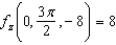
B)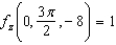
C)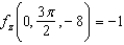
D)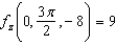
E)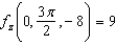
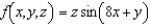 , evaluate
, evaluate 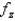 at the point
at the point 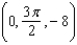 .
.A)
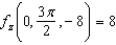
B)
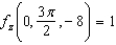
C)
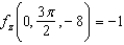
D)
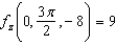
E)
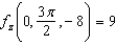

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
10
Find the partial derivative 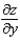 for the function
for the function 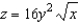 .
.
A)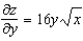
B)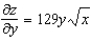
C)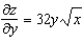
D)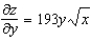
E)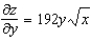
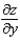 for the function
for the function 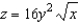 .
.A)
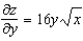
B)
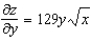
C)
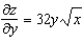
D)
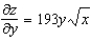
E)
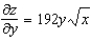

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
11
Find the partial derivative 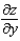 for the function
for the function 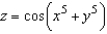 .
.
A)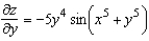
B)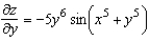
C)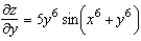
D)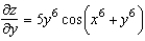
E)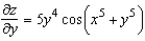
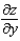 for the function
for the function 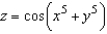 .
.A)
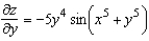
B)
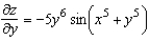
C)
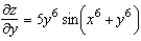
D)
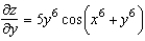
E)
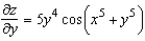

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
12
Find the partial derivative 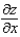 for the function
for the function 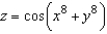 .
.
A)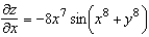
B)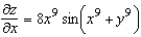
C)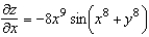
D)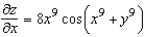
E)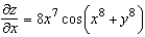
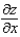 for the function
for the function 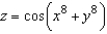 .
.A)
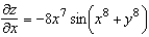
B)
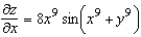
C)
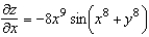
D)
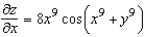
E)
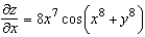

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
13
Find the partial derivative 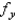 for the function
for the function 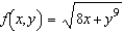 .
.
A)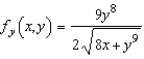
B)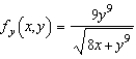
C)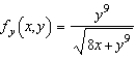
D)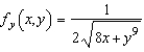
E)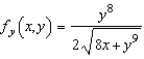
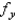 for the function
for the function 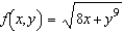 .
.A)
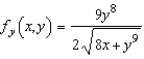
B)
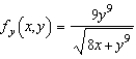
C)
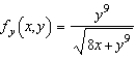
D)
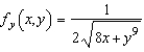
E)
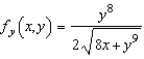

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
14
For 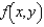 , find all values of x and y such that
, find all values of x and y such that 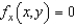 and
and 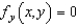 simultaneously.
simultaneously. 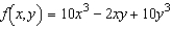
A)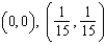
B)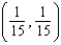
C)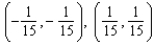
D)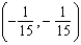
E)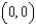
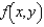 , find all values of x and y such that
, find all values of x and y such that 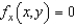 and
and 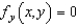 simultaneously.
simultaneously. 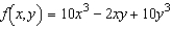
A)
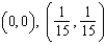
B)
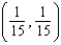
C)
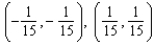
D)
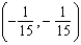
E)
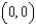

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
15
Find the partial derivative 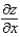 for the function
for the function 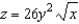 .
.
A)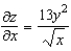
B)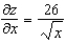
C)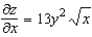
D)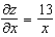
E)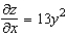
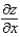 for the function
for the function 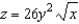 .
.A)
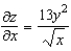
B)
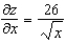
C)
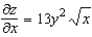
D)
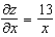
E)
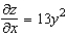

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
16
For 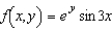 , evaluate
, evaluate 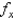 at the point
at the point 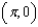 .
.
A)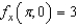
B)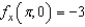
C)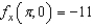
D)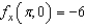
E)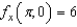
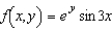 , evaluate
, evaluate 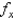 at the point
at the point 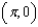 .
.A)
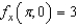
B)
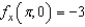
C)
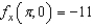
D)
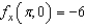
E)
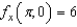

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
17
For 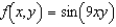 , evaluate
, evaluate 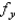 at the point
at the point 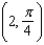 .
.
A)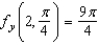
B)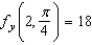
C)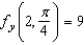
D)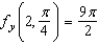
E)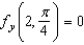
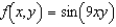 , evaluate
, evaluate 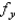 at the point
at the point 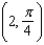 .
.A)
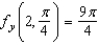
B)
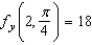
C)
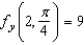
D)
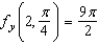
E)
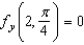

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
18
Find the partial derivative 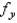 for the function
for the function 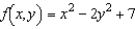 .
.
A)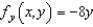
B)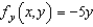
C)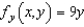
D)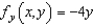
E)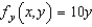
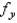 for the function
for the function 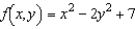 .
.A)
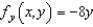
B)
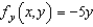
C)
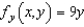
D)
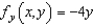
E)
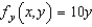

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
19
Find the second partial derivative for the function 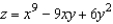 with respect to x.
with respect to x.
A)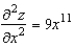
B)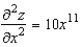
C)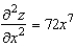
D)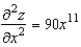
E)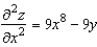
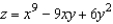 with respect to x.
with respect to x.A)
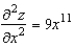
B)
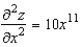
C)
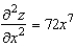
D)
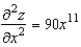
E)
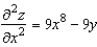

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
20
For 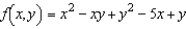 find all values of x and y such that
find all values of x and y such that 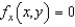 and
and 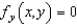 simultaneously.
simultaneously.
A)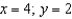
B)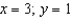
C)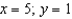
D)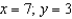
E)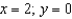
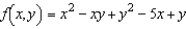 find all values of x and y such that
find all values of x and y such that 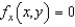 and
and 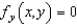 simultaneously.
simultaneously.A)
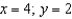
B)
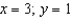
C)
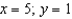
D)
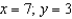
E)
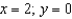

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
21
Suppose the utility function 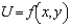 is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product
is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product 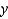 if the utility function is
if the utility function is 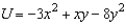 .
.
A)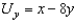
B)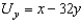
C)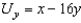
D)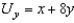
E)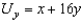
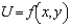 is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product
is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product 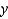 if the utility function is
if the utility function is 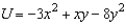 .
.A)
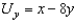
B)
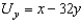
C)
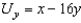
D)
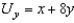
E)
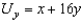

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose the utility function 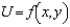 is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product x if the utility function is
is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product x if the utility function is 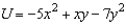 .
.
A)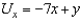
B)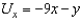
C)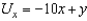
D)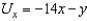
E)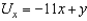
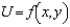 is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product x if the utility function is
is a measure of the utility (or satisfaction) derived by a person from the consumption of two products x and y. Determine the marginal utility of product x if the utility function is 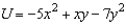 .
.A)
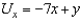
B)
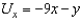
C)
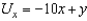
D)
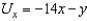
E)
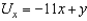

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck
23
Suppose the temperature at any point  in a steel plate is
in a steel plate is 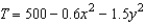 where x and y are measured in meters. At the point
where x and y are measured in meters. At the point 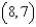 find the rate of change of the temperature with respect to the distance moved along the plate in the direction of the x-axis. Round your answer to one decimal place.
find the rate of change of the temperature with respect to the distance moved along the plate in the direction of the x-axis. Round your answer to one decimal place.
A)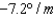
B)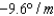
C)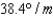
D)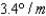
E)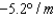
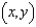 in a steel plate is
in a steel plate is 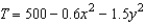 where x and y are measured in meters. At the point
where x and y are measured in meters. At the point 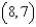 find the rate of change of the temperature with respect to the distance moved along the plate in the direction of the x-axis. Round your answer to one decimal place.
find the rate of change of the temperature with respect to the distance moved along the plate in the direction of the x-axis. Round your answer to one decimal place.A)
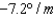
B)
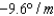
C)
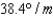
D)
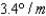
E)
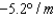

Unlock Deck
Unlock for access to all 23 flashcards in this deck.
Unlock Deck
k this deck



