Deck 56: The Binomial Theorem
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/67
Play
Full screen (f)
Deck 56: The Binomial Theorem
1
Evaluate using Pascal's Triangle.
A)8
B)6
C)10
D)9
E)7
A)8
B)6
C)10
D)9
E)7
8
2
Evaluate using Pascal's Triangle.
A)496
B)495
C)497
D)494
E)493
A)496
B)495
C)497
D)494
E)493
495
3
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Calculate the binomial coefficient.
A)16
B)15
C)17
D)14
E)13
A)16
B)15
C)17
D)14
E)13

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
5
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
6
Calculate the binomial coefficient.
A)0
B)4
C)1
D)3
E)2
A)0
B)4
C)1
D)3
E)2

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
7
Calculate the binomial coefficient.
A)2
B)3
C)1
D)4
E)0
A)2
B)3
C)1
D)4
E)0

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
8
Calculate the binomial coefficient.
A)496
B)493
C)498
D)497
E)495
A)496
B)493
C)498
D)497
E)495

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
9
Calculate the binomial coefficient.
A)33
B)37
C)34
D)36
E)35
A)33
B)37
C)34
D)36
E)35

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
10
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
11
Expand the binomial by using Pascal's Triangle to determine the coefficients.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
12
Evaluate using Pascal's Triangle.
A)54
B)58
C)56
D)55
E)57
A)54
B)58
C)56
D)55
E)57

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
13
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
14
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
15
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
16
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
17
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
18
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
19
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
20
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
21
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
22
Calculate the binomial coefficient:
A)12
B)24
C)0
D)4
E)1
A)12
B)24
C)0
D)4
E)1

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
23
Find the coefficient a of the term in the expansion of the binomial. Binomial Terms
A)2,208
B)2,204
C)2,206
D)2,205
E)2,203
A)2,208
B)2,204
C)2,206
D)2,205
E)2,203

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
24
Find the specified nth term in the expansion of the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
25
Use the Binomial Theorem to expand the complex number.Simplify your result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
26
Expand the expression in the difference quotient and simplify. Difference quotient
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
27
Find the specified nth term in the expansion of the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
28
Find the specified nth term in the expansion of the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
29
Find the specified nth term in the expansion of the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
30
Find the coefficient a of the term in the expansion of the binomial. Binomial Terms
A)8,304,721,920
B)8,304,721,921
C)8,304,721,923
D)8,304,721,918
E)8,304,721,919
A)8,304,721,920
B)8,304,721,921
C)8,304,721,923
D)8,304,721,918
E)8,304,721,919

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
31
Find the specified nth term in the expansion of the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
32
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
33
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
34
Find the specified nth term in the expansion of the binomial.
A)251
B)254
C)253
D)252
E)250
A)251
B)254
C)253
D)252
E)250

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
35
Find the coefficient a of the term in the expansion of the binomial. Binomial Terms
A)-6,300,001
B)-6,300,002
C)-6,299,999
D)-6,300,000
E)-6,299,997
A)-6,300,001
B)-6,300,002
C)-6,299,999
D)-6,300,000
E)-6,299,997

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
36
Use the Binomial Theorem to expand the complex number.Simplify your result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
37
Find the coefficient a of the term in the expansion of the binomial. Binominal Terms
A)-407,653,785
B)-652,246,056
C)760,953,732
D)181,179,460
E)652,246,056
A)-407,653,785
B)-652,246,056
C)760,953,732
D)181,179,460
E)652,246,056

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
38
Find the coefficient a of the term in the expansion of the binomial. Binomial Terms
A)1,621
B)1,620
C)1,619
D)1,623
E)1,618
A)1,621
B)1,620
C)1,619
D)1,623
E)1,618

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
39
Find the specified nth term in the expansion of the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
40
Expand the binomial by using Pascal's Triangle to determine the coefficients.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
41
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
42
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
43
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
44
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
45
Use the binomial theorem to expand the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
46
Use the binomial theorem to expand the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
47
Calculate the binomial coefficient:
A)30
B)1
C)12
D)0
E)15
A)30
B)1
C)12
D)0
E)15

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
48
Find the specified nth term in the expansion of the binomial.(Write the expansion in descending powers of x. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
49
Use the Binominal Theorem to expand the complex number.Simplify your result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
50
Find the coefficient a of the term in the expansion of the binomial. Binomial Term
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
51
Find the specified nth term in the expansion of the binomial.(Write the expansion in descending powers of x. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
52
Find the coefficient a of the term in the expansion of the binomial. Binomial Term
A)a = 13
B)a = 10206
C)a = 45
D)a = -19683
E)a = 3024
A)a = 13
B)a = 10206
C)a = 45
D)a = -19683
E)a = 3024

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
53
Use the Binomial Theorem to expand and simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
54
The probability that a basketball player will make a given free throw is .To find the probability that the player makes exactly 8 out of her next 10 free throws,evaluate the term in the expansion of .Round to four decimal places.
A)0.2721
B)0.0106
C)0.0047
D)0.7558
E)0.1209
A)0.2721
B)0.0106
C)0.0047
D)0.7558
E)0.1209

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
55
Evaluate using Pascal's triangle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
56
Evaluate using Pascal's triangle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
57
Use the Binominal Theorem to expand the complex number.Simplify your result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
58
Calculate the binomial coefficient:
A)6652800
B)88
C)165
D)1
E)0
A)6652800
B)88
C)165
D)1
E)0

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
59
Use the binomial theorem to expand the binomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
60
Calculate the binomial coefficient:
A)332640
B)66
C)462
D)1
E)0
A)332640
B)66
C)462
D)1
E)0

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
61
Evaluate using Pascal's triangle.Show your work. 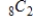
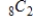

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
62
Use the binomial theorem to expand the binomial. 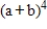
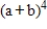

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
63
Expand the binomial by using Pascal's triangle to determine the coefficients.Show your work. 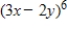
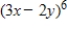

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
64
Find the 3rd term in the binomial expansion. 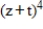
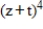

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
65
Evaluate using Pascal's triangle.Show your work. 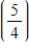
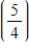

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
66
The probability that a basketball player will make a given free throw is .To find the probability that the player makes exactly 7 out of her next 10 free throws,evaluate the term in the expansion of .Round to four decimal places.
A)0.0090
B)3.3897
C)0.0007
D)0.2668
E)9.8825
A)0.0090
B)3.3897
C)0.0007
D)0.2668
E)9.8825

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck
67
Use the binomial theorem to expand the binomial. 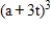
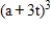

Unlock Deck
Unlock for access to all 67 flashcards in this deck.
Unlock Deck
k this deck



