Deck 63: Rotation of Conics
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/52
Play
Full screen (f)
Deck 63: Rotation of Conics
1
Select the graph of the following equation.
A)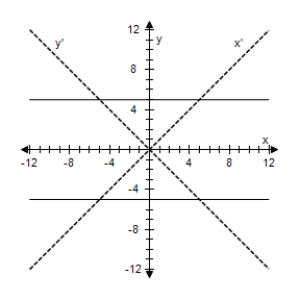
B)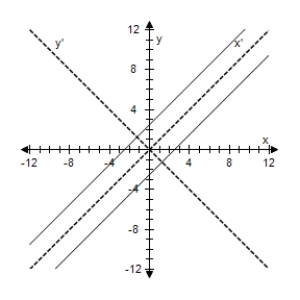
C) 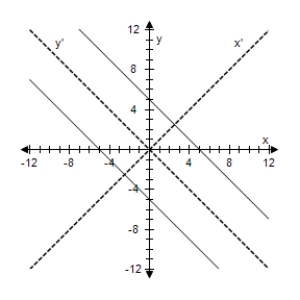
D)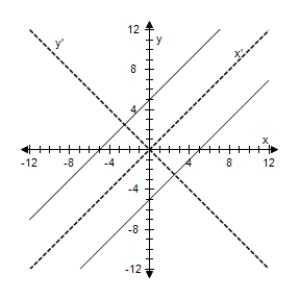
E)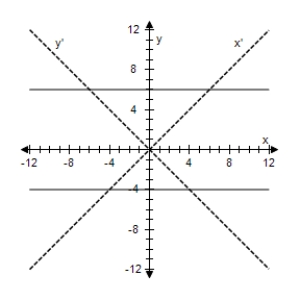
A)

B)

C)

D)

E)



2
Select the graph of the following equation,showing both sets of axes.
A)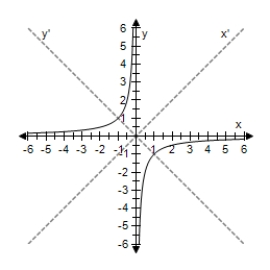
B)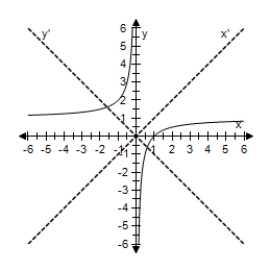
C)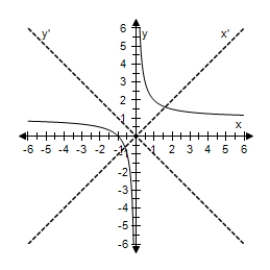
D)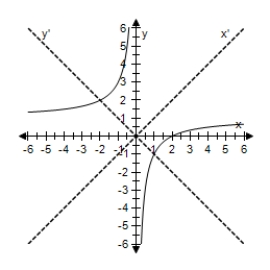
E)
A)

B)

C)

D)

E)



3
The -coordinate system has been rotated degrees from the -coordinate system.The coordinates of a point in the -coordinate system are given.Find the coordinates of the point in the rotated coordinate system. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Select the graph of the following equation,showing both sets of axes.
A)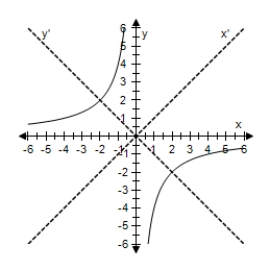
B)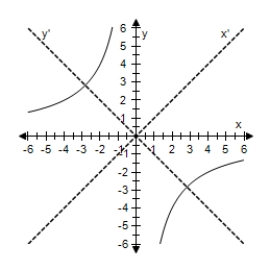
C)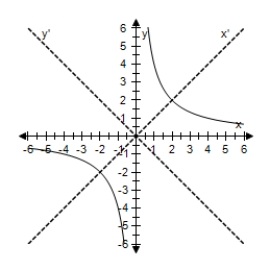
D)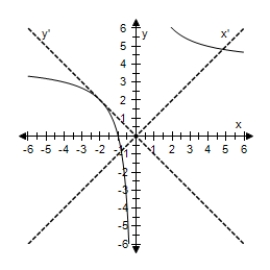
E)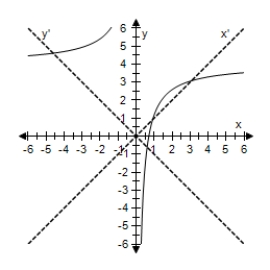
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
5
The -coordinate system has been rotated degrees from the -coordinate system.The coordinates of a point in the -coordinate system are given.Find the coordinates of the point in the rotated coordinate system. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
6
The -coordinate system has been rotated degrees from the -coordinate system.The coordinates of a point in the -coordinate system are given.Find the coordinates of the point in the rotated coordinate system. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
7
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
8
Use a graphing utility to graph the conic.Determine the angle through which the axes are rotated.
A) ,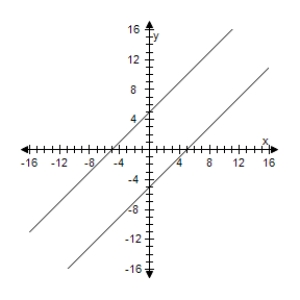
B) ,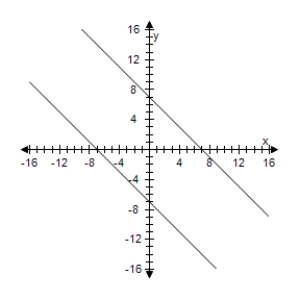
C) ,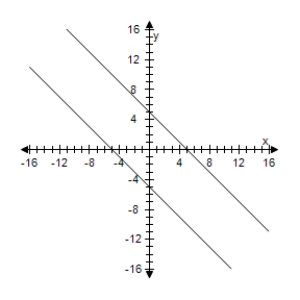
D) ,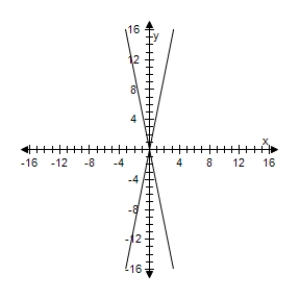
E) ,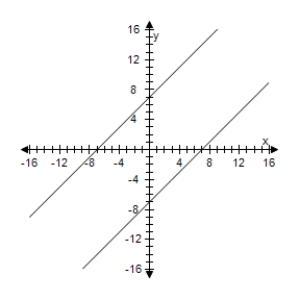
A) ,

B) ,

C) ,

D) ,

E) ,


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
9
The -coordinate system has been rotated degrees from the -coordinate system.The coordinates of a point in the -coordinate system are given.Find the coordinates of the point in the rotated coordinate system. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
10
Use a graphing utility to graph the conic.
A)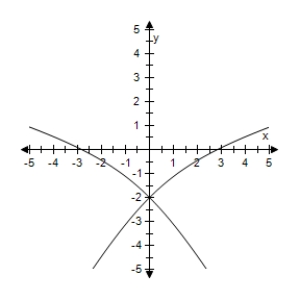
B)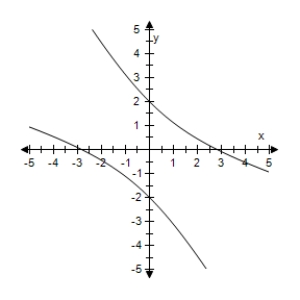
C)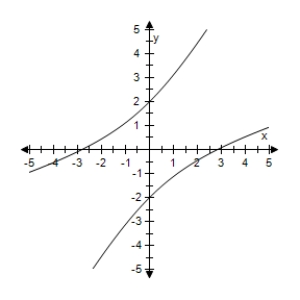
D)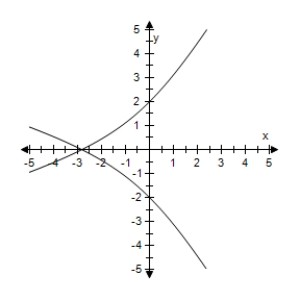
E)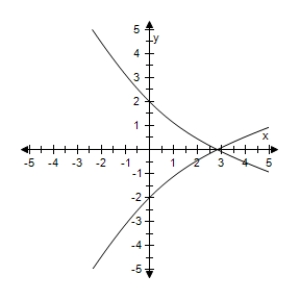
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
11
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
12
The -coordinate system has been rotated degrees from the -coordinate system.The coordinates of a point in the -coordinate system are given.Find the coordinates of the point in the rotated coordinate system. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
13
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
14
Rotate the axes to eliminate the xy-term in the equation.Then write the equation in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
15
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
16
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
17
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
18
The -coordinate system has been rotated degrees from the -coordinate system.The coordinates of a point in the -coordinate system are given.Find the coordinates of the point in the rotated coordinate system. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
19
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
20
Rotate the axes to eliminate the xy-term in the equation.Then write the equation in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
21
Select the graph of degenerate conic.
A)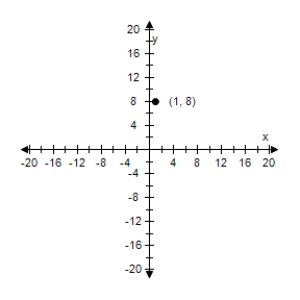
B)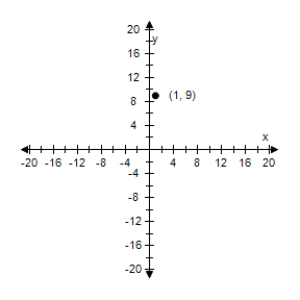
C)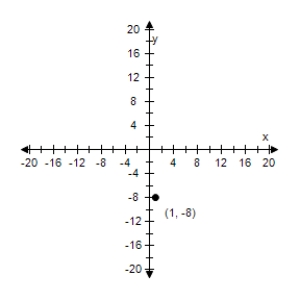
D)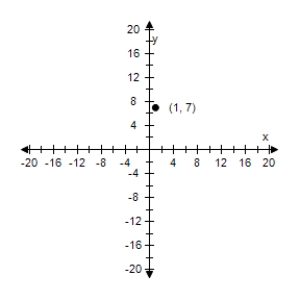
E)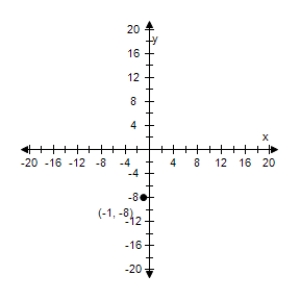
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
22
Select the graph of the following equation.
A)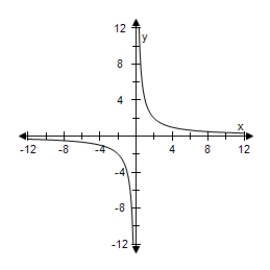
B)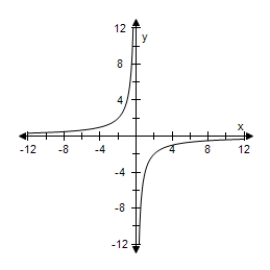
C)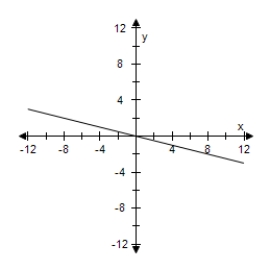
D) 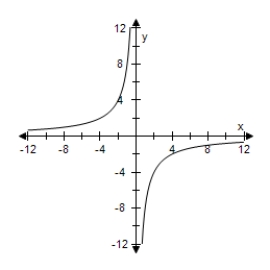
E)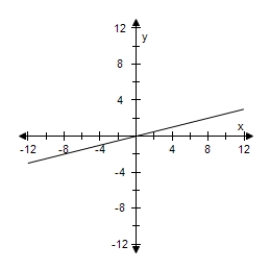
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
23
Use the discriminant to classify the graph.
A)The graph is a cone.
B)The graph is a parabola.
C)The graph is a ellipse or circle.
D)The graph is a hyperbola.
E)The graph is a line.
A)The graph is a cone.
B)The graph is a parabola.
C)The graph is a ellipse or circle.
D)The graph is a hyperbola.
E)The graph is a line.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
24
Use the discriminant to classify the graph.
A)The graph is a parabola.
B)The graph is a hyperbola.
C)The graph is a ellipse.
D)The graph is a cone.
E)The graph is a circle.
A)The graph is a parabola.
B)The graph is a hyperbola.
C)The graph is a ellipse.
D)The graph is a cone.
E)The graph is a circle.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
25
Use the discriminant to classify the graph.
A)The graph is a hyperbola.
B)The graph is a ellipse.
C)The graph is a circle.
D)The graph is a line.
E)The graph is a parabola.
A)The graph is a hyperbola.
B)The graph is a ellipse.
C)The graph is a circle.
D)The graph is a line.
E)The graph is a parabola.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
26
Use the discriminant to classify the graph.
A)The graph is a hyperbola.
B)The graph is a line.
C)The graph is a ellipse or Circle.
D)The graph is a parabola.
E)The graph is a cone.
A)The graph is a hyperbola.
B)The graph is a line.
C)The graph is a ellipse or Circle.
D)The graph is a parabola.
E)The graph is a cone.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
27
Select the graph of degenerate conic.
A)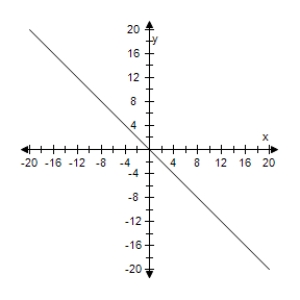
B)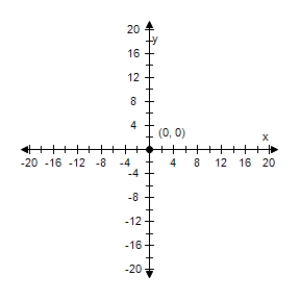
C)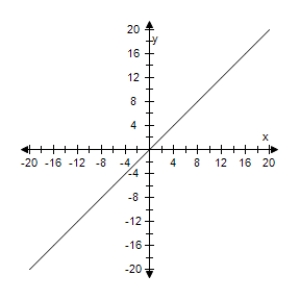
D)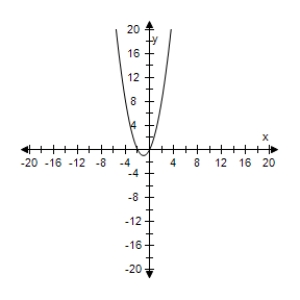
E)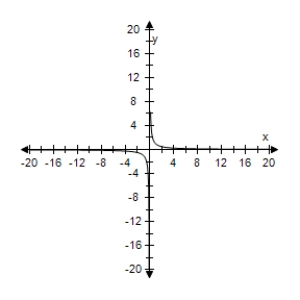
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
28
Use the Quadratic Formula to solve for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
29
Use the Quadratic Formula to solve for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
30
Use the Quadratic Formula to solve for .
A)
B)
C) .
D)
E)
A)
B)
C) .
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
31
Select the graph of degenerate conic.
A)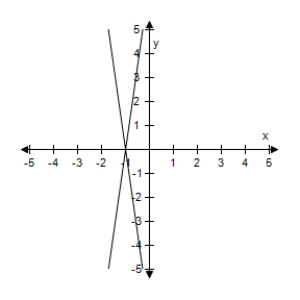
B)
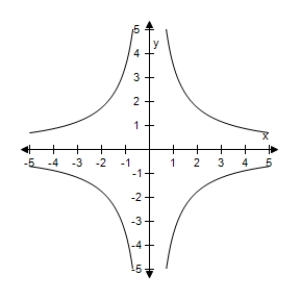
C)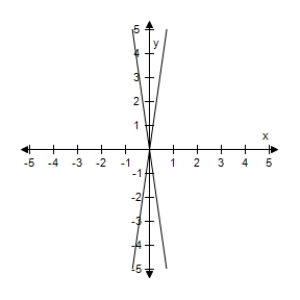
D)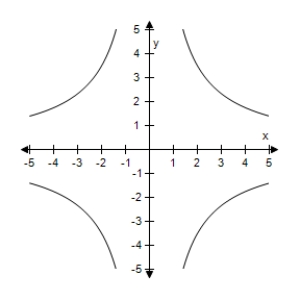
E)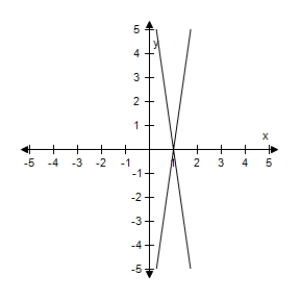
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
32
Select the graph of the following equation
A)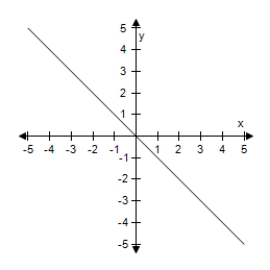
B)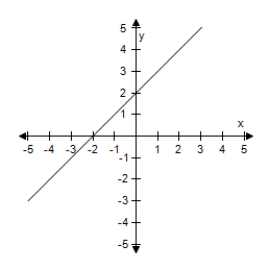
C)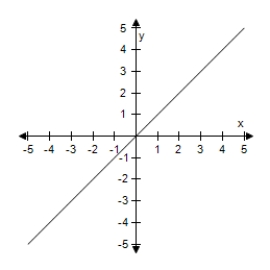
D)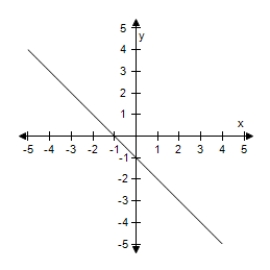
E)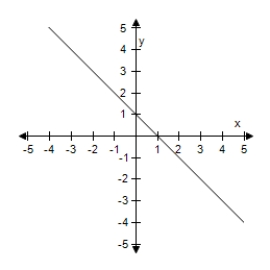
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
33
Use the Quadratic Formula to solve for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
34
Use the Quadratic Formula to solve for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
35
Use the Quadratic Formula to solve for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
36
Use the discriminant to classify the graph.
A)The graph is a ellipse.
B)The graph is a circle.
C)The graph is a hyperbola.
D)The graph is a line.
E)The graph is a parabola.
A)The graph is a ellipse.
B)The graph is a circle.
C)The graph is a hyperbola.
D)The graph is a line.
E)The graph is a parabola.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
37
Use the Quadratic Formula to solve for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
38
Use the discriminant to classify the graph.
A)The graph is a cone.
B)The graph is a circle.
C)The graph is a parabola.
D)The graph is a ellipse.
E)The graph is a hyperbola.
A)The graph is a cone.
B)The graph is a circle.
C)The graph is a parabola.
D)The graph is a ellipse.
E)The graph is a hyperbola.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
39
Use the discriminant to classify the graph.
A)The graph is a hyperbola.
B)The graph is a ellipse or circle.
C)The graph is a parabola.
D)The graph is a line.
E)The graph is a cone.
A)The graph is a hyperbola.
B)The graph is a ellipse or circle.
C)The graph is a parabola.
D)The graph is a line.
E)The graph is a cone.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
40
Use the discriminant to classify the graph.
A)The graph is a parabola.
B)The graph is a hyperbola.
C)The graph is a line.
D)The graph is a circle.
E)The graph is a ellipse.
A)The graph is a parabola.
B)The graph is a hyperbola.
C)The graph is a line.
D)The graph is a circle.
E)The graph is a ellipse.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
41
Identify the conic by writing the equation in standard form.
A) ;ellipse
B) ;ellipse
C) ;circle
D) ;circle
E) ;circle
A) ;ellipse
B) ;ellipse
C) ;circle
D) ;circle
E) ;circle

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
42
Find the lengths of the major and minor axes of the ellipse graphed by following equation.
A)Length of major axis is Length of minor axis is
B)Length of major axis is Length of minor axis is
C)Length of major axis is Length of minor axis is
D)Length of major axis is Length of minor axis is
E)None of the above
A)Length of major axis is Length of minor axis is
B)Length of major axis is Length of minor axis is
C)Length of major axis is Length of minor axis is
D)Length of major axis is Length of minor axis is
E)None of the above

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
43
Consider the equation. Without calculating,explain how to rewrite the equation so that it does not have an -term.
A)To rewrite the equation so that it does not have an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
B)To rewrite the equation so that it does not have an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
C)To rewrite the equation so that it has an -term,you can solve for in terms of by taking square root or using the Quadratic Formula.
D)To rewrite the equation so that it has an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
E)To rewrite the equation so that it has an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
A)To rewrite the equation so that it does not have an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
B)To rewrite the equation so that it does not have an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
C)To rewrite the equation so that it has an -term,you can solve for in terms of by taking square root or using the Quadratic Formula.
D)To rewrite the equation so that it has an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.
E)To rewrite the equation so that it has an -term,you can solve for in terms of by completing the square or using the Quadratic Formula.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
44
Find any points of intersection of the graphs algebraically.
A)The points of intersection are and .
B)The points of intersection are and .
C)The points of intersection are and .
D)The points of intersection are and .
E)The points of intersection are and .
A)The points of intersection are and .
B)The points of intersection are and .
C)The points of intersection are and .
D)The points of intersection are and .
E)The points of intersection are and .

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
45
Select the graph of degenerate conic.
A)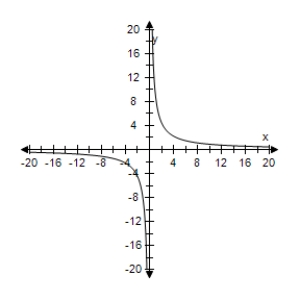
B)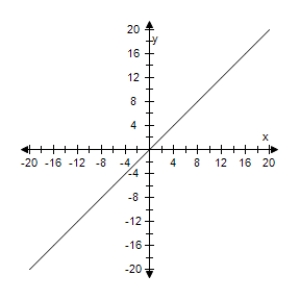
C)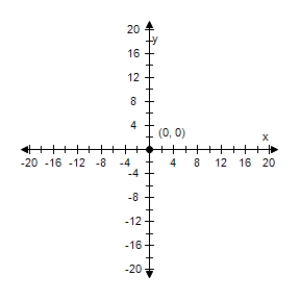
D)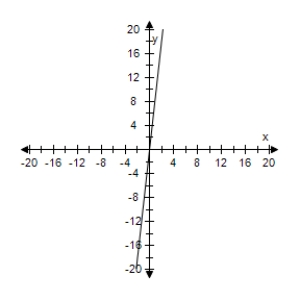
E)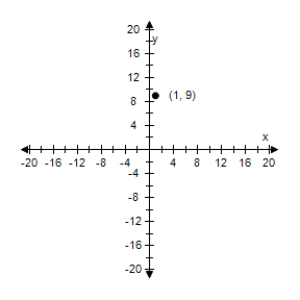
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
46
Identify the conic by writing the equation in standard form.
A) ;hyperbola
B) ;hyperbola
C) ;hyperbola
D) ;ellipse
E) ;ellipse
A) ;hyperbola
B) ;hyperbola
C) ;hyperbola
D) ;ellipse
E) ;ellipse

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
47
Consider the equation. Explain how to identify the graph of the equation.
A)Find the discriminant of the equation to identify the graph of the equation.
B)Find the foci of the equation to identify the graph of the equation.
C)Find the major axis of the equation to identify the graph of the equation.
D)Find the minor axis of the equation to identify the graph of the equation.
E)Find the vertices of the equation to identify the graph of the equation.
A)Find the discriminant of the equation to identify the graph of the equation.
B)Find the foci of the equation to identify the graph of the equation.
C)Find the major axis of the equation to identify the graph of the equation.
D)Find the minor axis of the equation to identify the graph of the equation.
E)Find the vertices of the equation to identify the graph of the equation.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
48
Select the graph of degenerate conic.
A)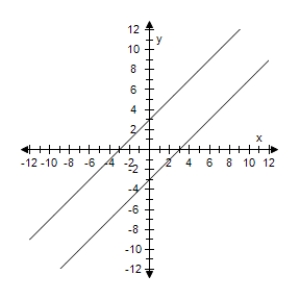
B)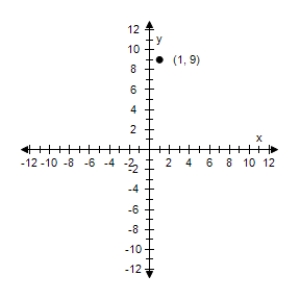
C)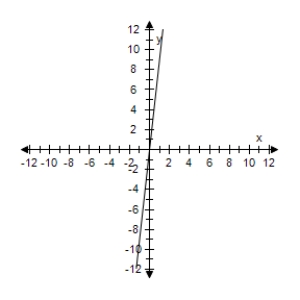
D)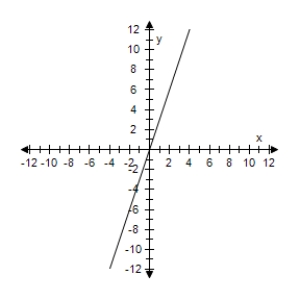
E)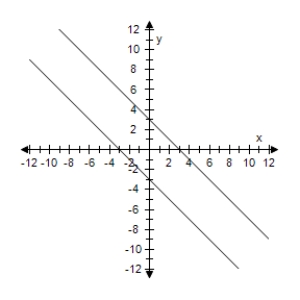
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
49
Identify the conic by writing the equation in standard form.
A) ;ellipse
B) ;ellipse
C) ;ellipse
D) ;ellipse
E) ;circle
A) ;ellipse
B) ;ellipse
C) ;ellipse
D) ;ellipse
E) ;circle

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
50
Select the graph of degenerate conic.
A)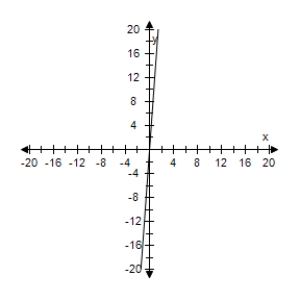
B)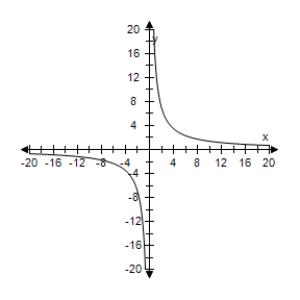
C)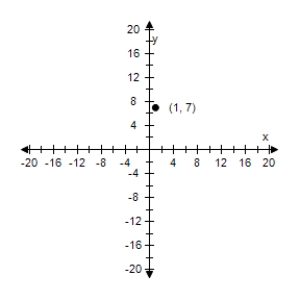
D)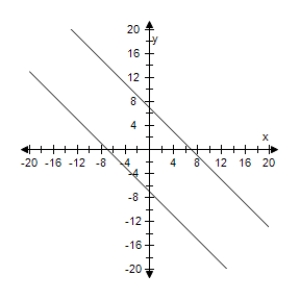
E)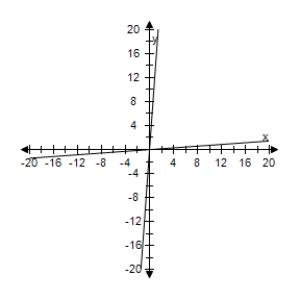
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
51
Determine whether the statement is true or false? Justify your answer.
The graph of the equation where, is any constant less than ,is a hyperbola.
A)False.For the graph to be a hyperbola,the discriminant must be less than zero.
B)True.For the graph to be a hyperbola,the discriminant must be greater than zero.
The graph of the equation where, is any constant less than ,is a hyperbola.
A)False.For the graph to be a hyperbola,the discriminant must be less than zero.
B)True.For the graph to be a hyperbola,the discriminant must be greater than zero.

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck
52
Determine the angle through which the axes are rotated.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 52 flashcards in this deck.
Unlock Deck
k this deck



