Deck 64: Parametric Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/50
Play
Full screen (f)
Deck 64: Parametric Equations
1
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Select the curve represented by the parametric equations.
A)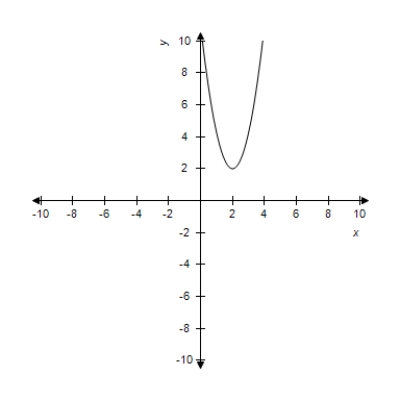
B)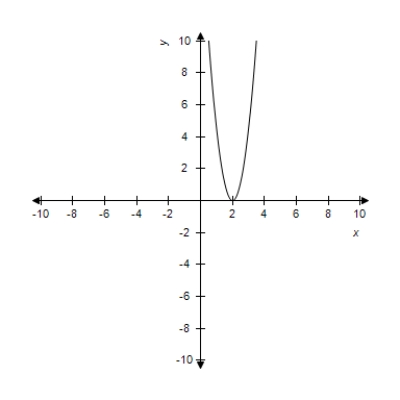
C)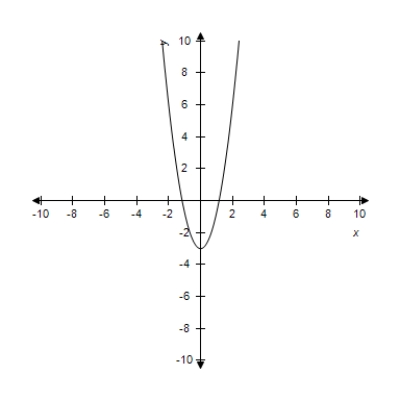
D)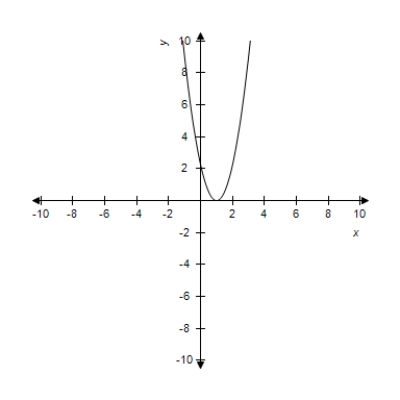
E)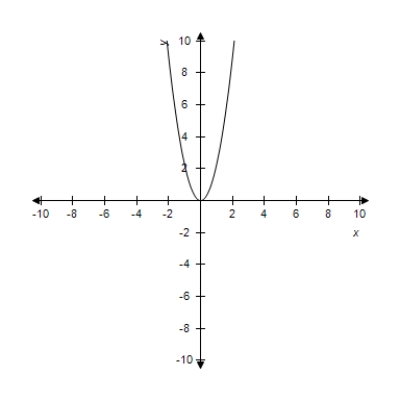
A)

B)

C)

D)

E)



3
Select the curve represented by the parametric equations.
A)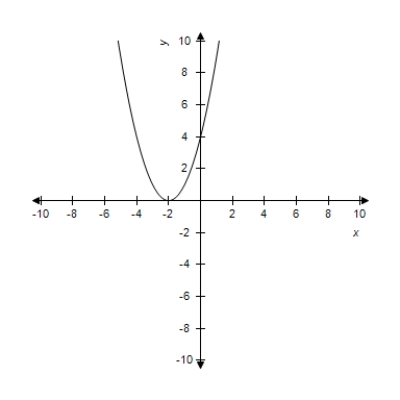
B)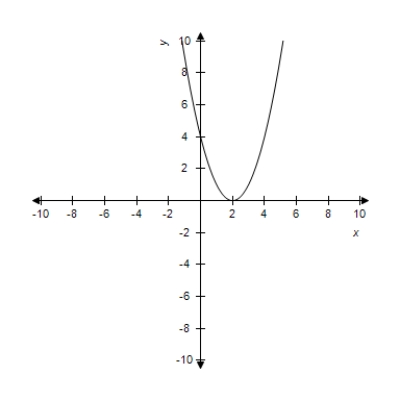
C)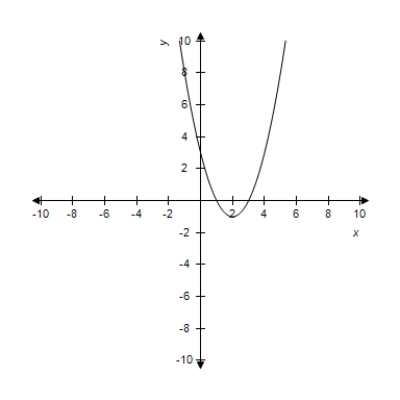
D)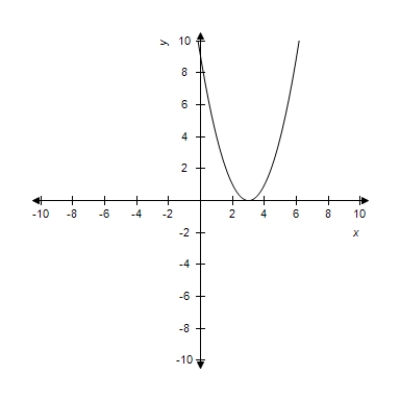
E)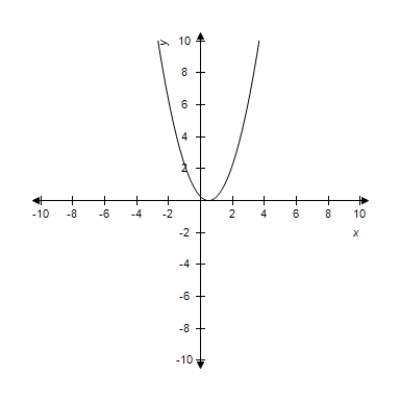
A)

B)

C)

D)

E)



4
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
5
Select the curve represented by the parametric equations.
A)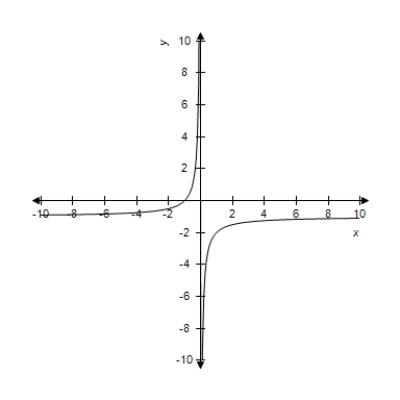
B)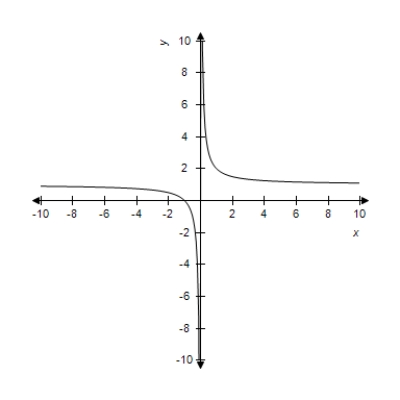
C)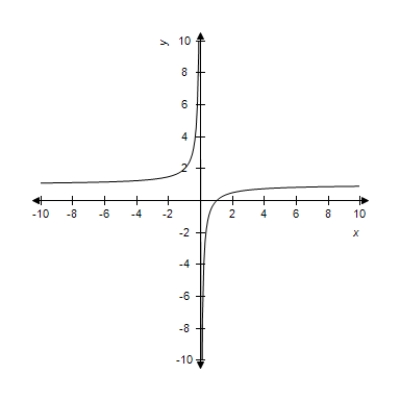
D) 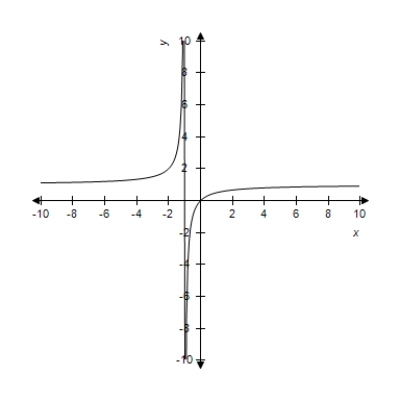
E)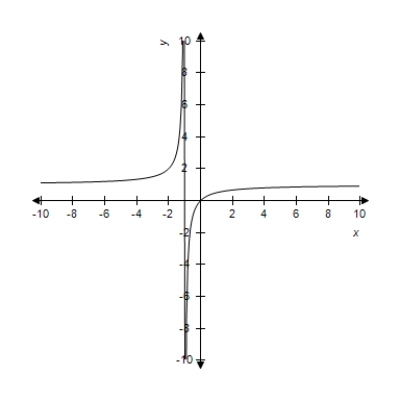
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
6
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
7
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C) .
D)
A)
B)
C) .
D)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
8
Select the curve represented by the parametric equations.
A)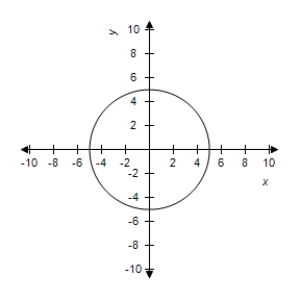
B)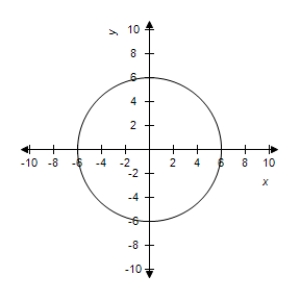
C)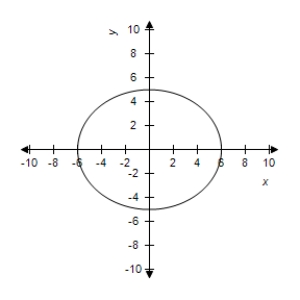
D)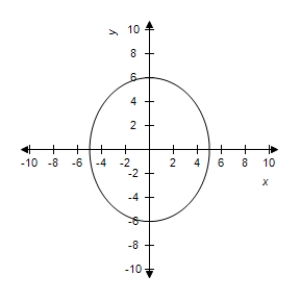
E)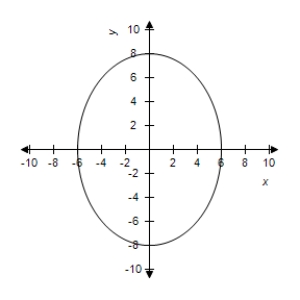
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
9
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
10
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
11
Select the curve represented by the parametric equations.
A)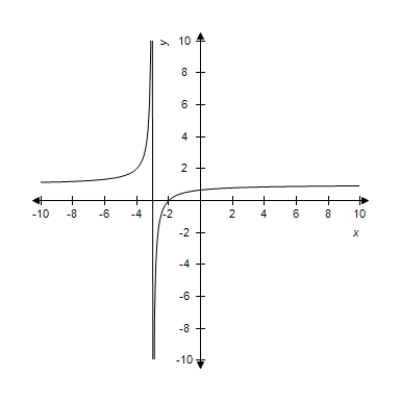
B)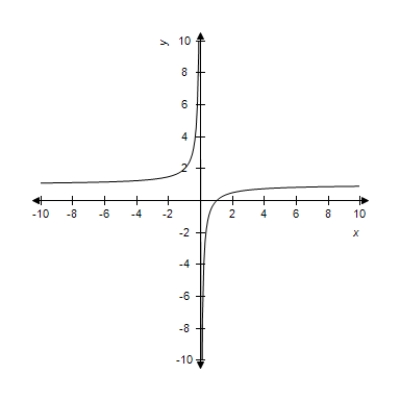
C)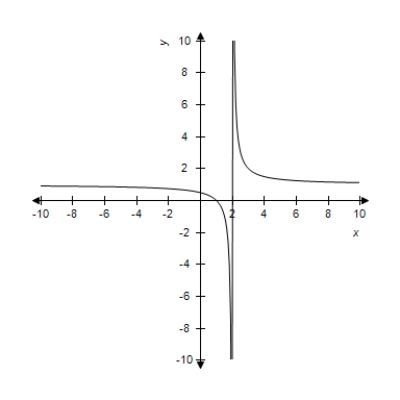
D)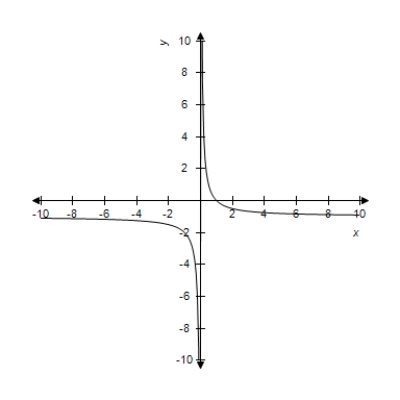
E)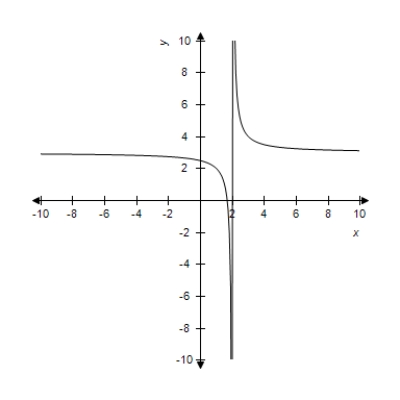
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
12
Select the curve represented by the parametric equations.
A)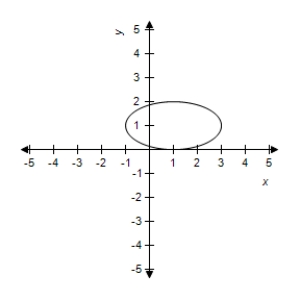
B)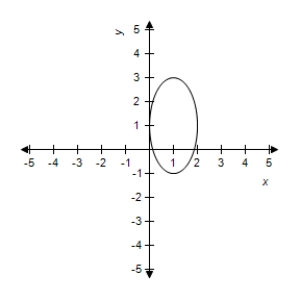
C)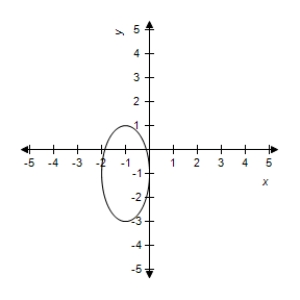
D)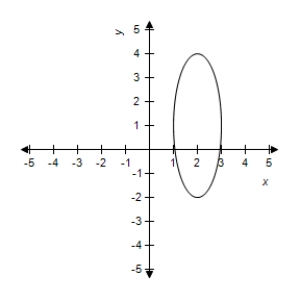
E)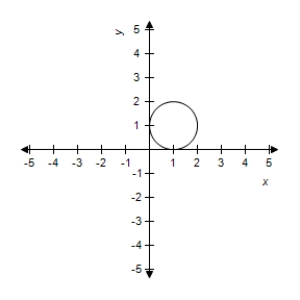
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
13
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
14
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
15
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
16
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
17
Select the curve represented by the parametric equations.
A)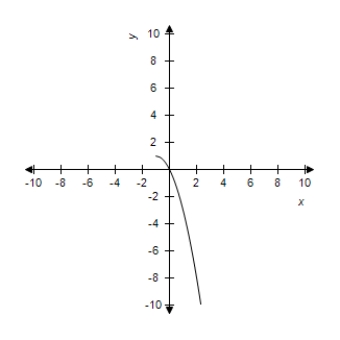
B)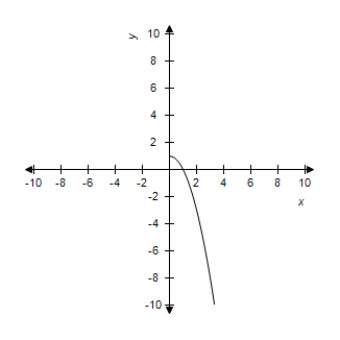
C)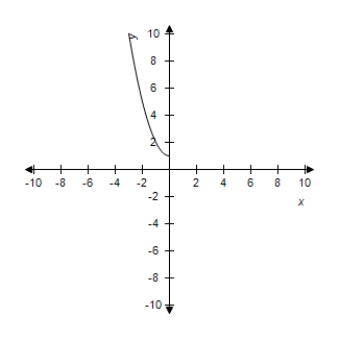
D)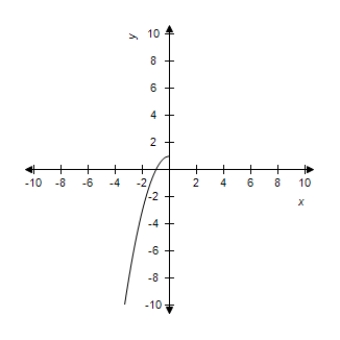
E)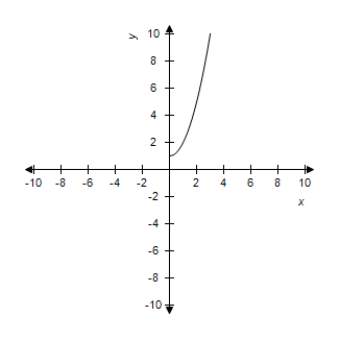
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
18
Select the curve represented by the parametric equations.(indicate the orientation of the curve)
A)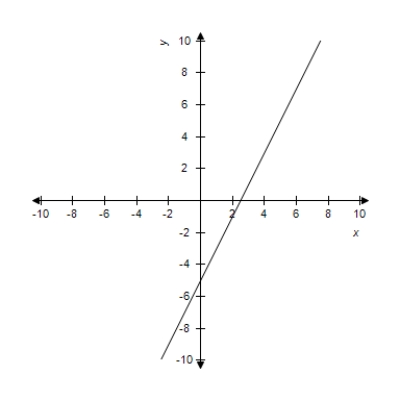
B)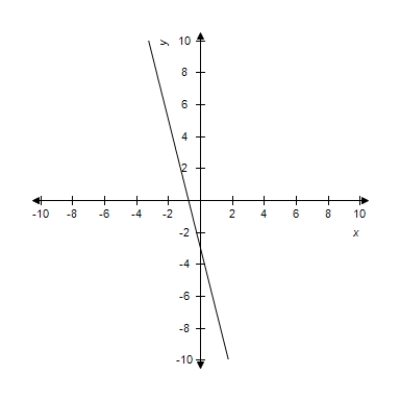
C)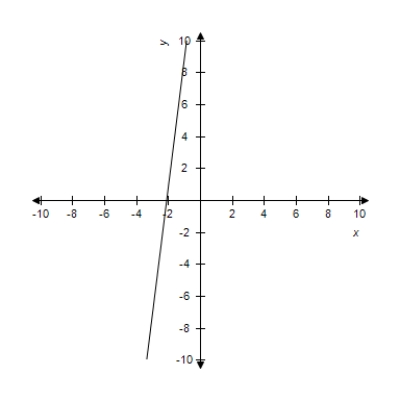
D)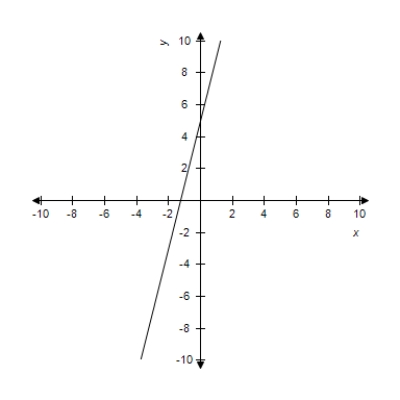
E)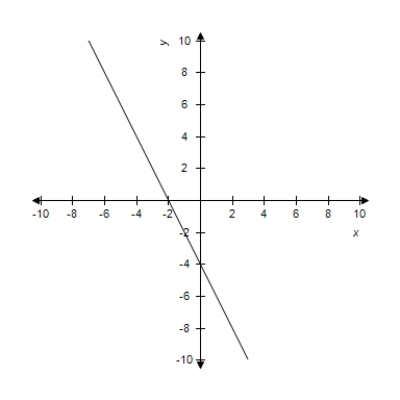
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
19
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
20
Select the curve represented by the parametric equations.(indicate the orientation of the curve)
A)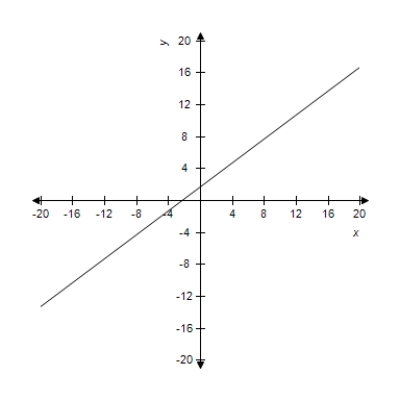
B)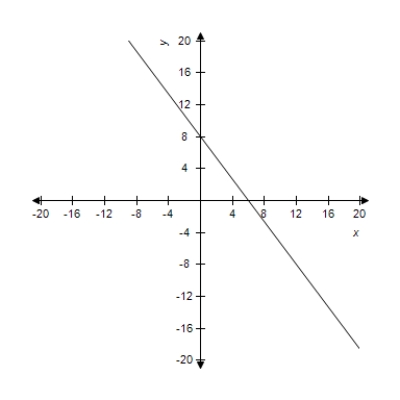
C)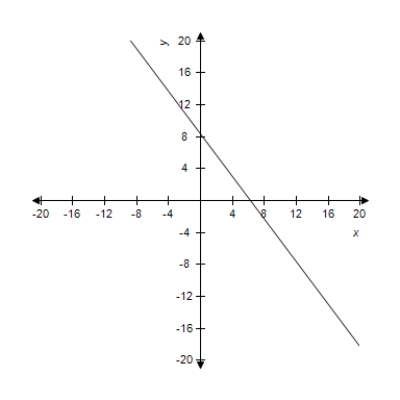
D)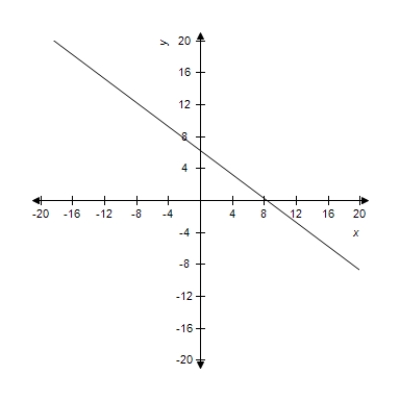
E)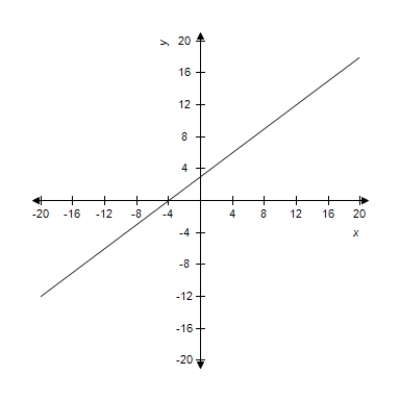
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
21
Using following result find a set of parametric equation of conic.
Circle:
Circle: center: ;radius: 8
A)
B)
C)
D)
E)
Circle:
Circle: center: ;radius: 8
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
22
Using following result find a set of parametric equation of conic. vertices: ;foci:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
23
Select the curve represented by the parametric equations.
Curtate cycloid:
A)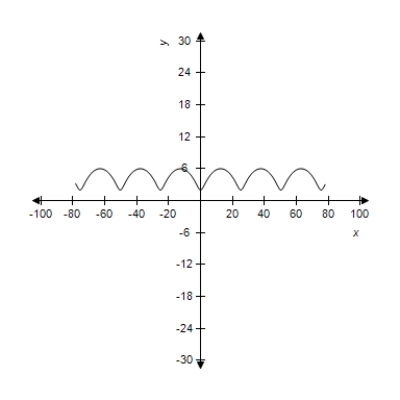
B)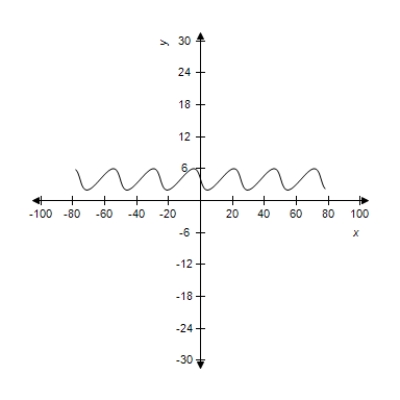
C)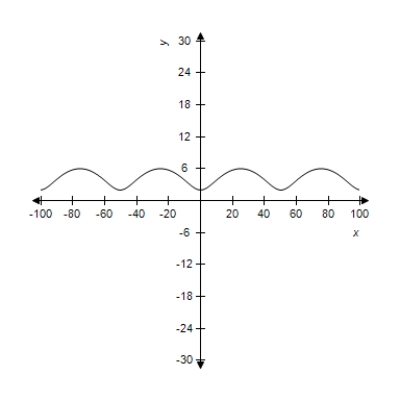
D)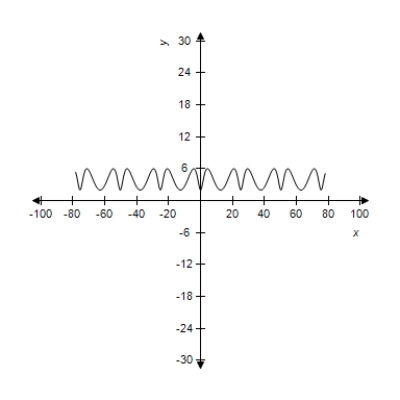
E)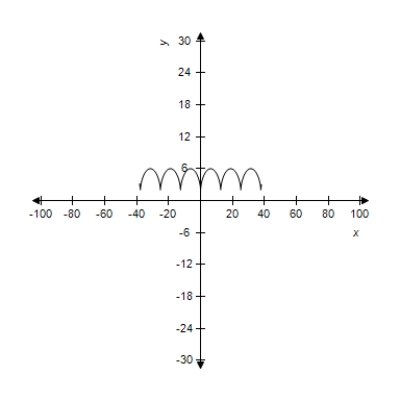
Curtate cycloid:
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
24
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
25
Select the curve represented by the parametric equations.
Prolate cycloid:
A)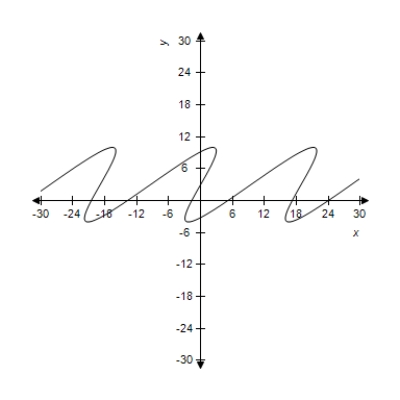
B)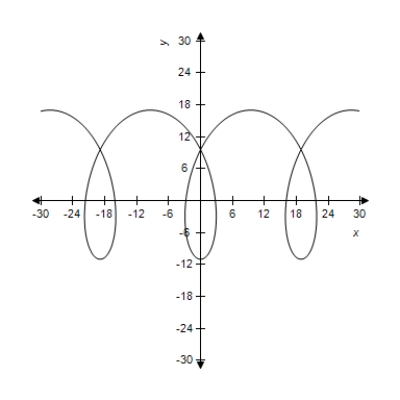
C)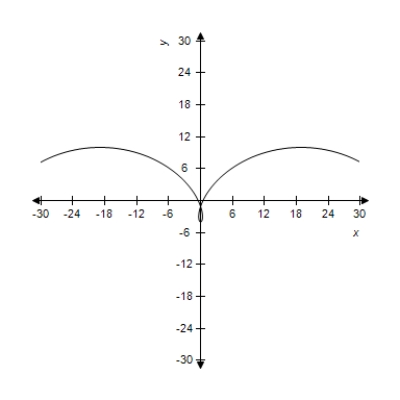
D)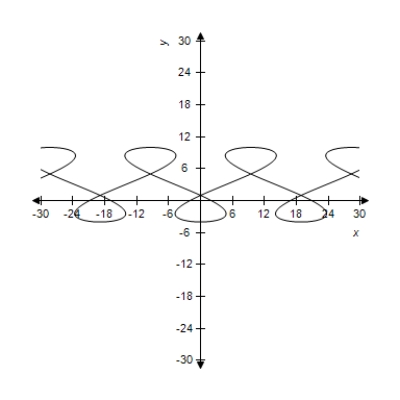
E)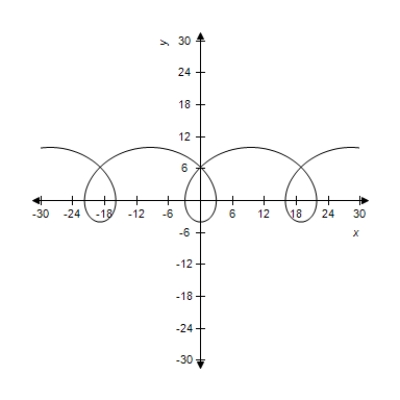
Prolate cycloid:
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
26
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
27
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
28
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
29
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
30
Using following result find a set of parametric equation of the line. Line: passes through (0,0)and
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
31
Using following result find a set of parametric equation of conic.
Hyperbola:
Hyperbola: vertices: ;foci:
A)
B)
C)
D)
E)
Hyperbola:
Hyperbola: vertices: ;foci:
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
32
Select the curve represented by the parametric equations.
Cycloid:
A)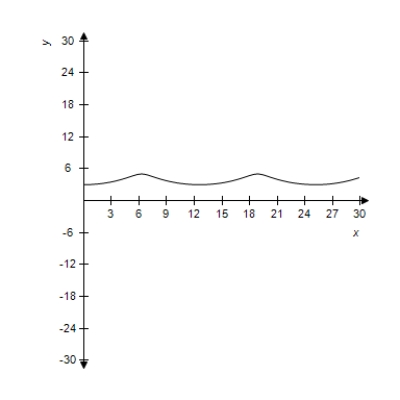
B)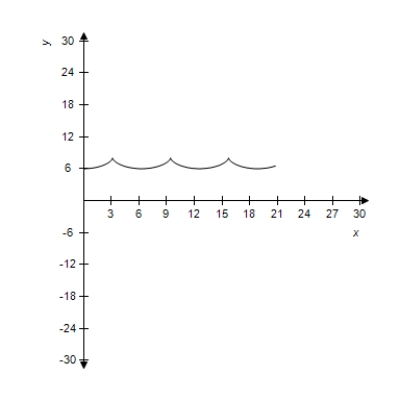
C)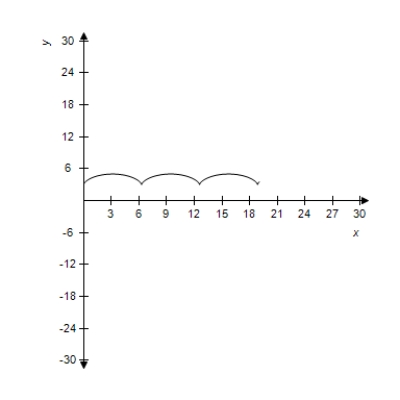
D)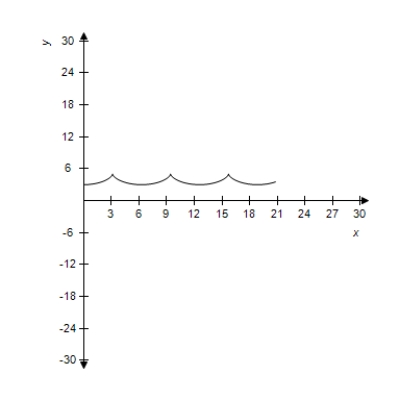
E)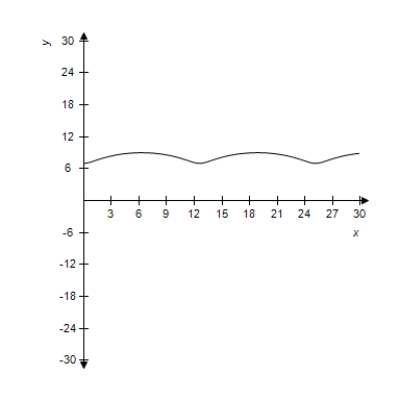
Cycloid:
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
33
Select the parametric equations matching with the following graph. 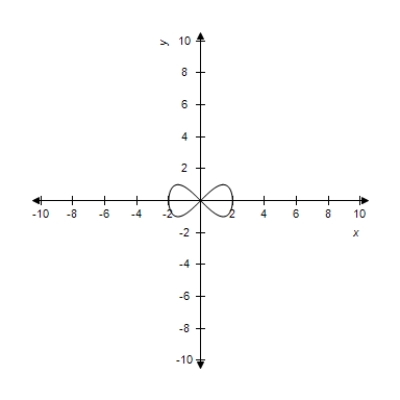
A)Lissajous curve:
B)Lissajous curve:
C)Lissajous curve:
D)Lissajous curve: ,
E)Lissajous curve:

A)Lissajous curve:
B)Lissajous curve:
C)Lissajous curve:
D)Lissajous curve: ,
E)Lissajous curve:

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
34
Using following result find a set of parametric equation of the line. Line: passes through and
A)
B) ,
C) ,
D)
E)
A)
B) ,
C) ,
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
35
Select the curve represented by the parametric equations.
Prolate cycloid:
A)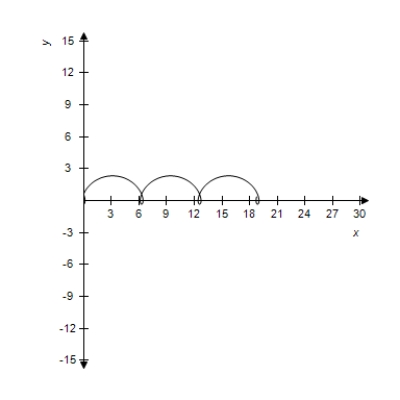
B)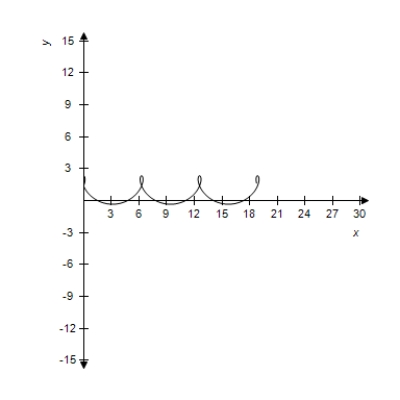
C)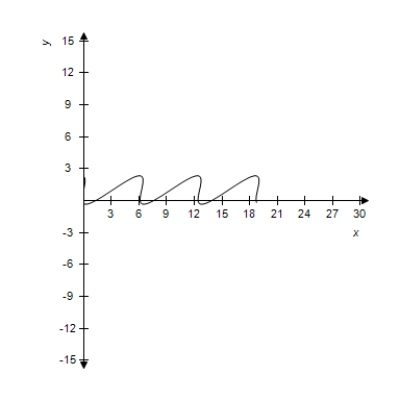
D)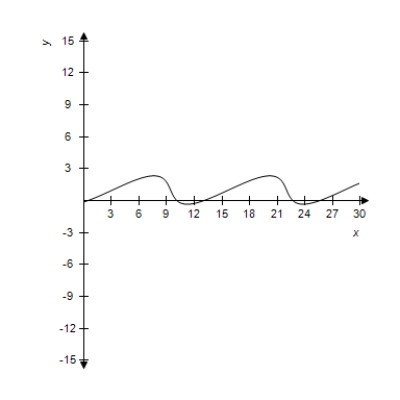
E)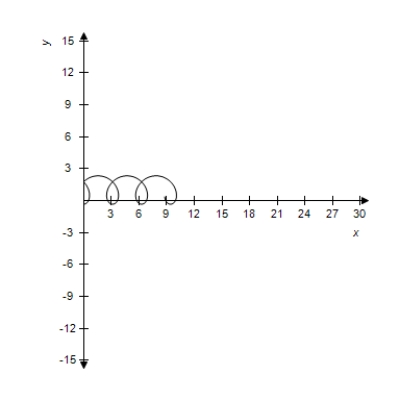
Prolate cycloid:
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
36
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
37
Find a set of parametric equations for the rectangular equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
38
Using following result find a set of parametric equation of conic.
Circle:
Circle: center: ;radius: 5
A)
B)
C)
D)
E)
Circle:
Circle: center: ;radius: 5
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
39
Select the curve represented by the parametric equations.
Cycloid:
A)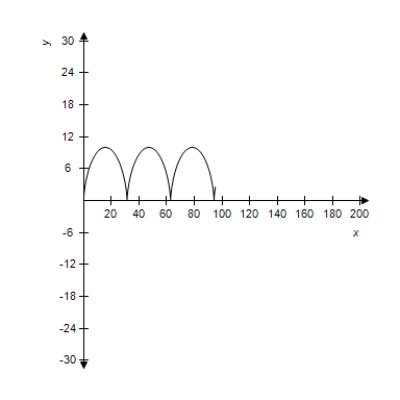
B)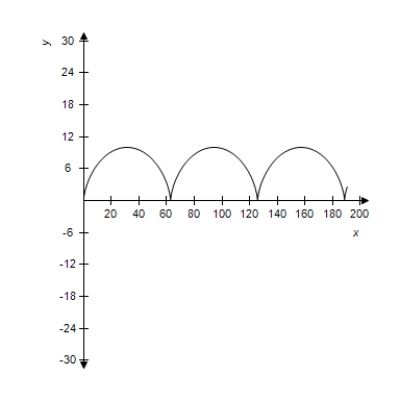
C)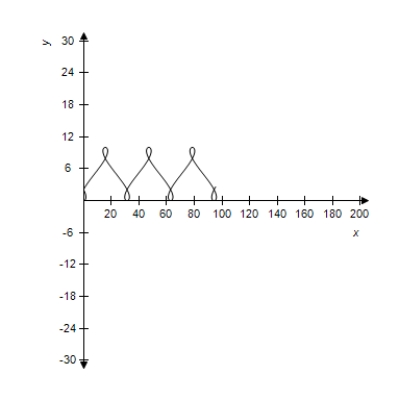
D)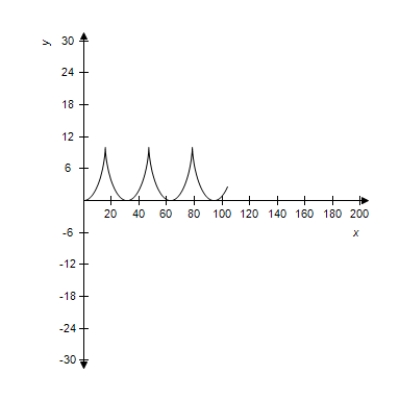
E)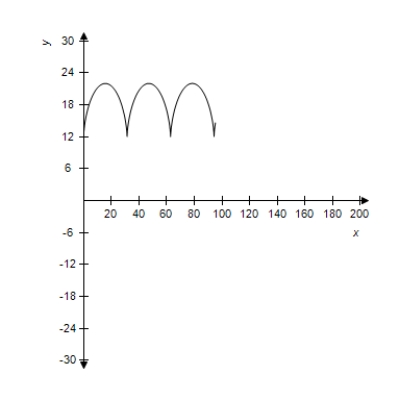
Cycloid:
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
40
Using following result find a set of parametric equation of conic.
Hyperbola:
Hyperbola: vertices: ;foci:
A)
B)
C)
D)
E)
Hyperbola:
Hyperbola: vertices: ;foci:
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
41
A projectile is launched at a height of h feet above the ground at an angle of with the horizontal.The initial velocity is feet per second,and the path of the projectile is modeled by the parametric equations . Select the correct graph of the path of a projectile launched from ground level at the value of and . feet per second
A)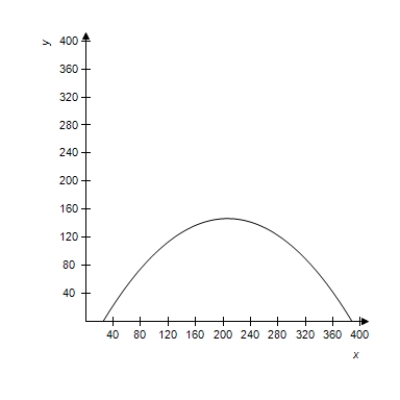
B)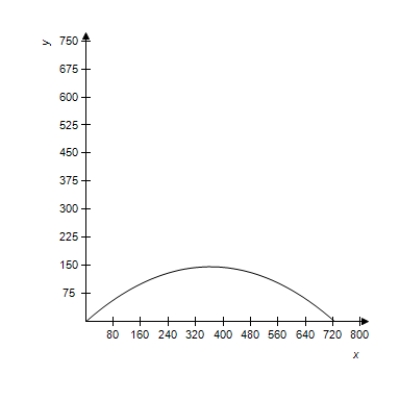
C)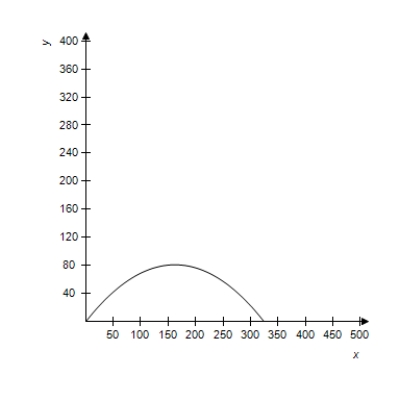
D)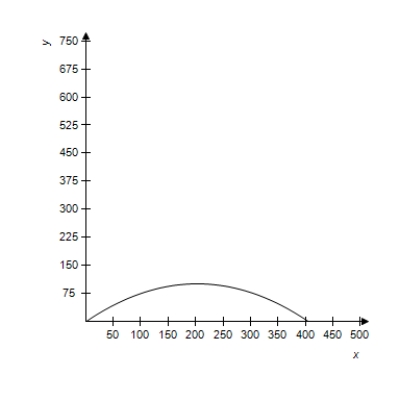
E)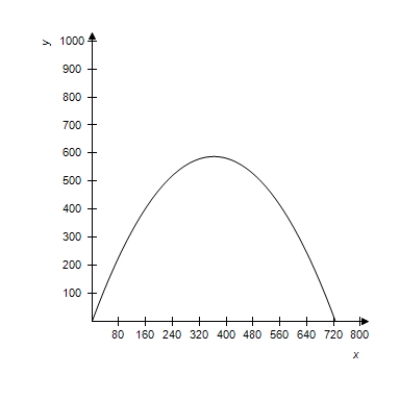
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
42
Select the parametric equations matching with the following graph. 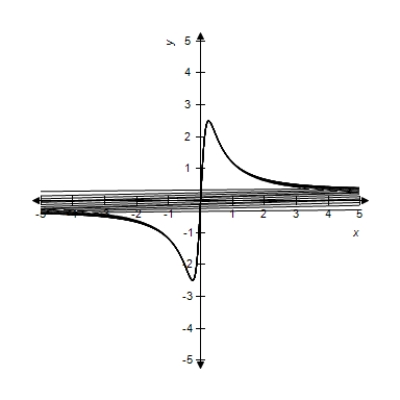
A)Serpentine curve:
B)Serpentine curve:
C)Serpentine curve:
D)Serpentine curve:
E)Serpentine curve:

A)Serpentine curve:
B)Serpentine curve:
C)Serpentine curve:
D)Serpentine curve:
E)Serpentine curve:

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
43
A projectile is launched at a height of h feet above the ground at an angle of with the horizontal.The initial velocity is feet per second,and the path of the projectile is modeled by the parametric equations . Select the correct graph of the path of a projectile launched from ground level at the value of and . , feet per second
A)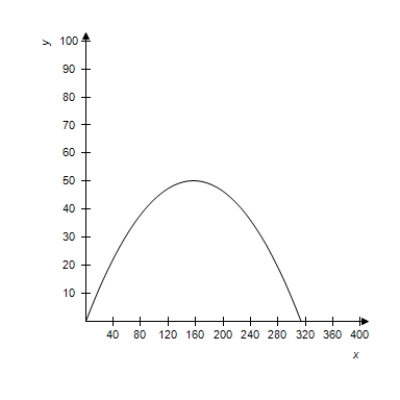
B)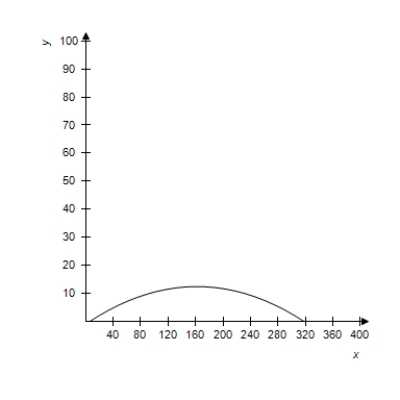
C)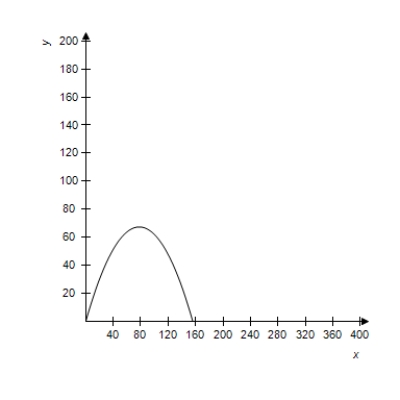
D)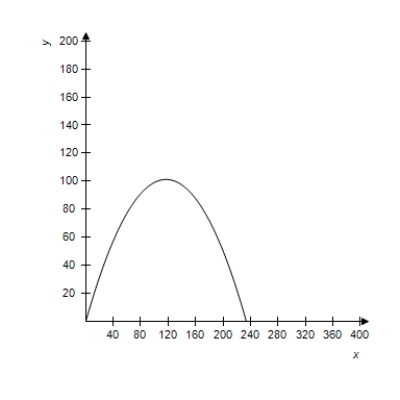
E)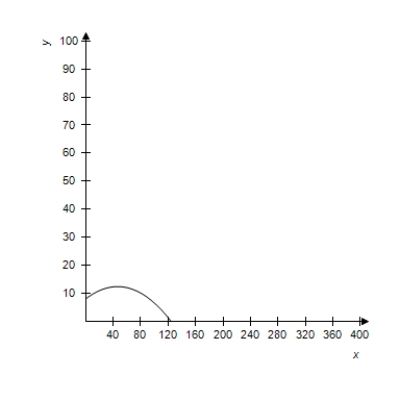
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
44
A projectile is launched at a height of h feet above the ground at an angle of with the horizontal.The initial velocity is feet per second,and the path of the projectile is modeled by the parametric equations . Select the correct graph of the path of a projectile launched from ground level at the value of and . feet per second
A)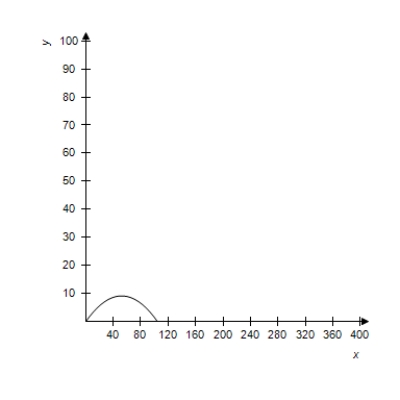
B)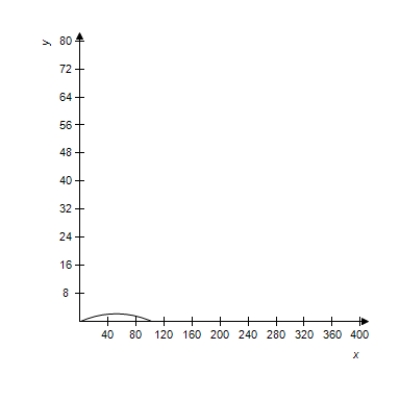
C)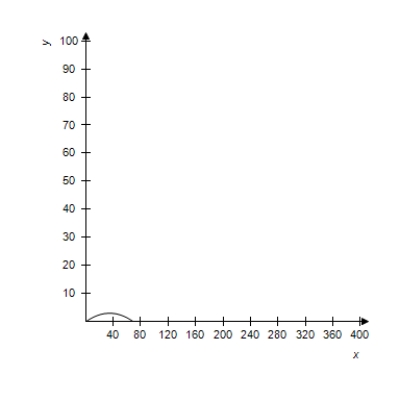
D)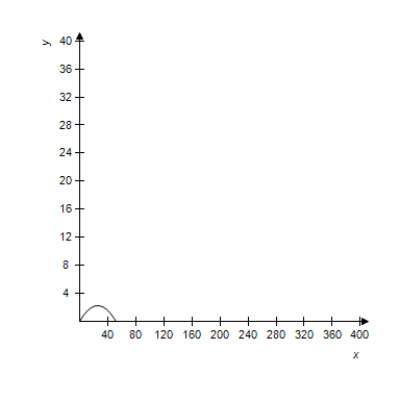
E)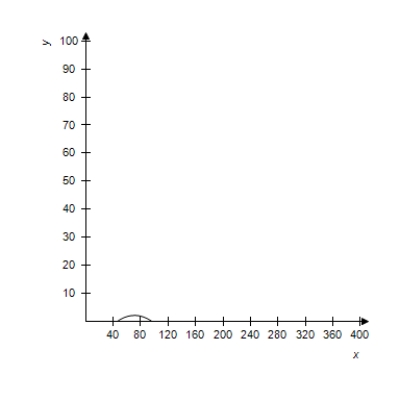
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
45
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
46
A projectile is launched at a height of h feet above the ground at an angle of with the horizontal.The initial velocity is feet per second,and the path of the projectile is modeled by the parametric equations . Select the correct graph of the path of a projectile launched from ground level at the value of and . feet per second
A)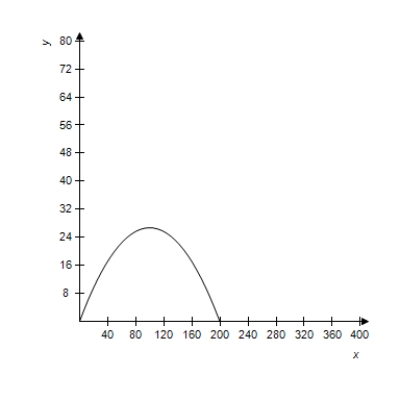
B)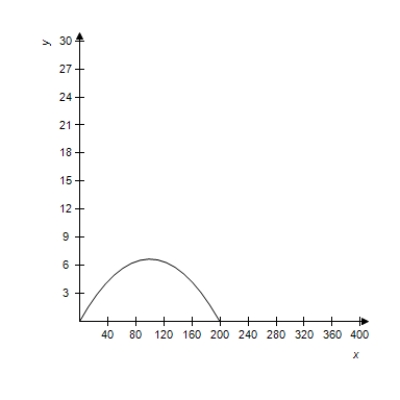
C)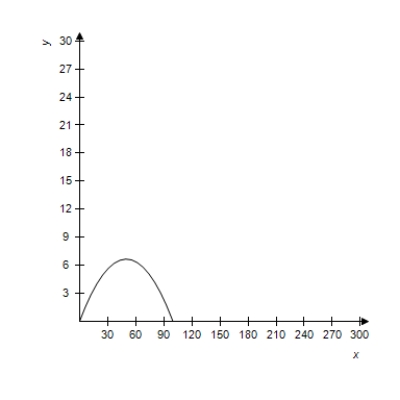
D)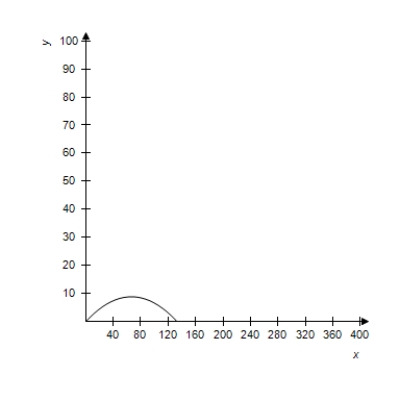
E)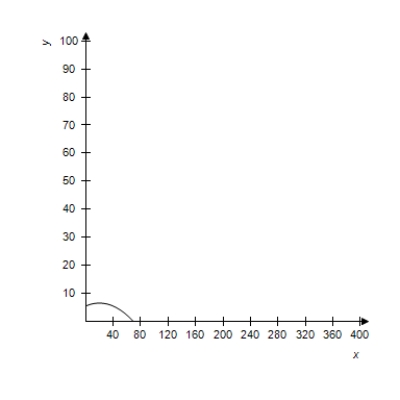
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
47
Select the parametric equations matching with the following graph. 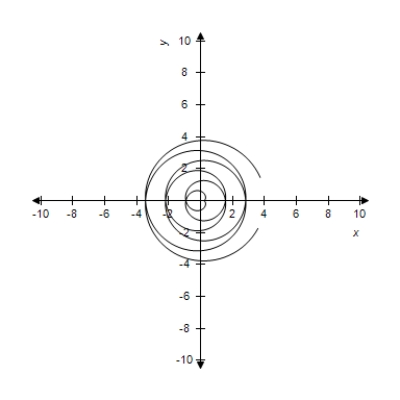
A)Involute of circle:
B)Involute of circle:
C)Involute of circle:
D)Involute of circle:
E)Involute of circle:

A)Involute of circle:
B)Involute of circle:
C)Involute of circle:
D)Involute of circle:
E)Involute of circle:

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
48
Select the curve represented by the parametric equations.
A)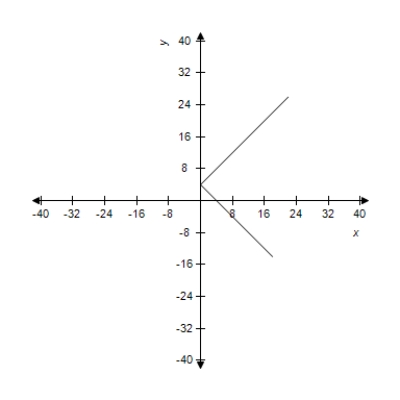
B)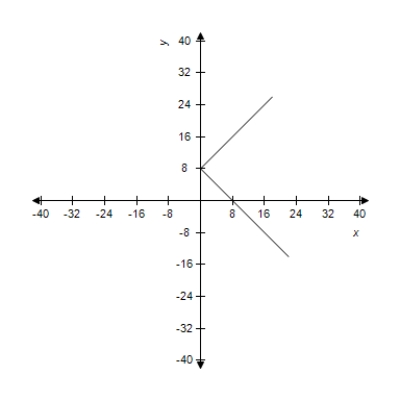
C)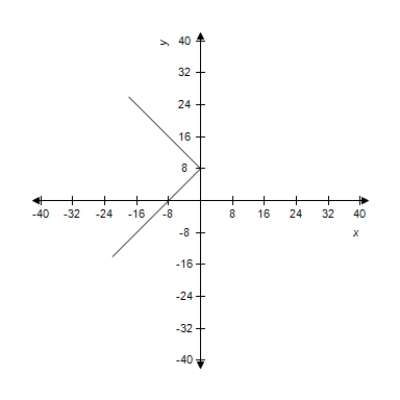
D)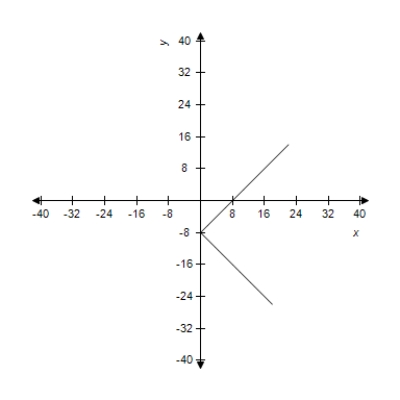
E)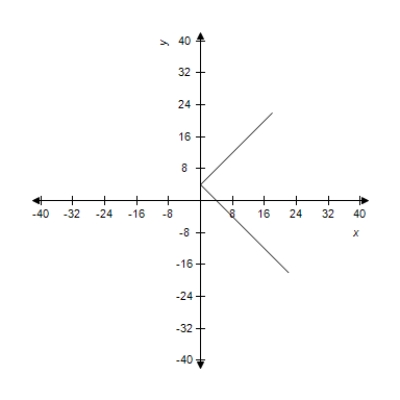
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
49
Eliminate the parameter and write the corresponding rectangular equation whose graph represents the curve.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
50
Select the curve represented by the parametric equations.
A)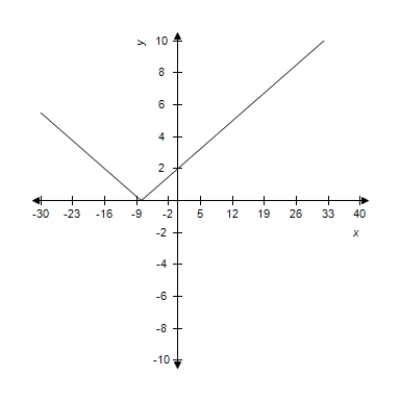
B)
C)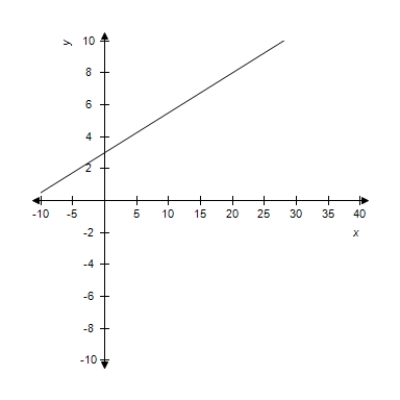
D)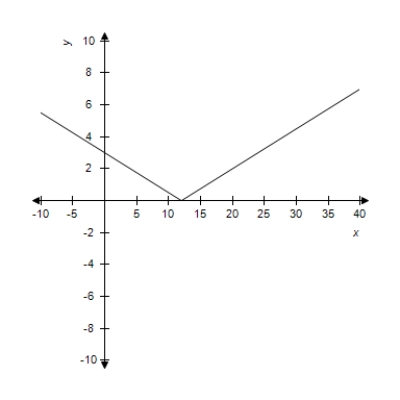
E)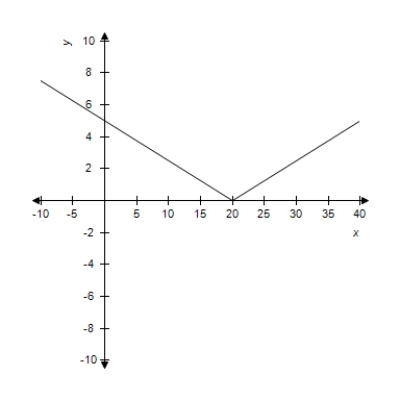
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck



