Deck 67: Graphs of Polar Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/49
Play
Full screen (f)
Deck 67: Graphs of Polar Equations
1
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to the polar axis 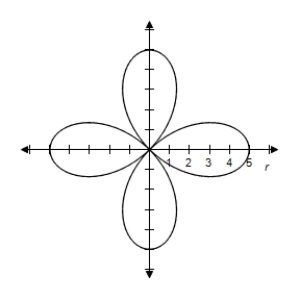
B)Symmetric with respect to the polar axis 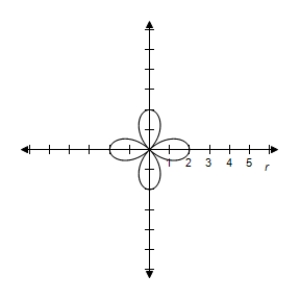
C)Symmetric with respect to the polar axis 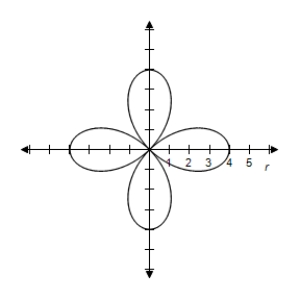
D)Symmetric with respect to the polar axis 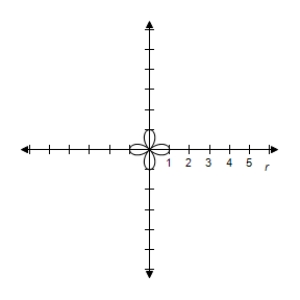
E)Symmetric with respect to the polar axis 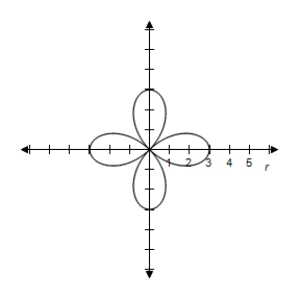
A)Symmetric with respect to the polar axis

B)Symmetric with respect to the polar axis

C)Symmetric with respect to the polar axis

D)Symmetric with respect to the polar axis

E)Symmetric with respect to the polar axis

Symmetric with respect to the polar axis 

2
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to polar axis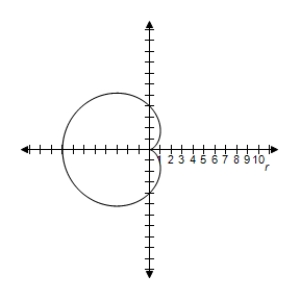
B)Symmetric with respect to polar axis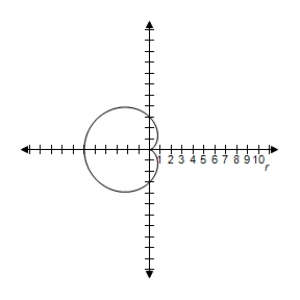
C)Symmetric with respect to polar axis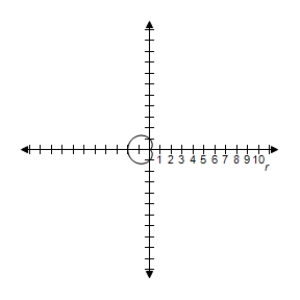
D)Symmetric with respect to polar axis 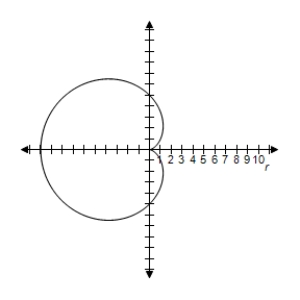
E)Symmetric with respect to polar axis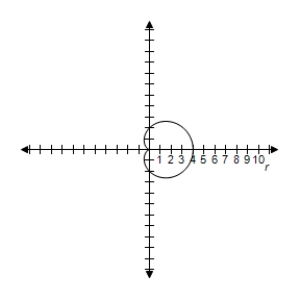
A)Symmetric with respect to polar axis

B)Symmetric with respect to polar axis

C)Symmetric with respect to polar axis

D)Symmetric with respect to polar axis

E)Symmetric with respect to polar axis

Symmetric with respect to polar axis 

3
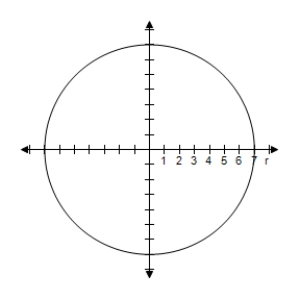
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to ,polar axis,pole Circle with radius
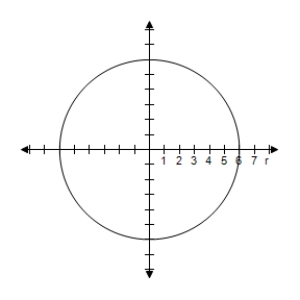
B)Symmetric with respect to ,polar axis,pole Circle with radius
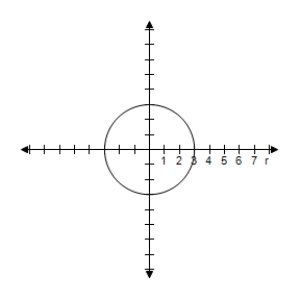
C)Symmetric with respect to ,polar axis,pole Circle with radius
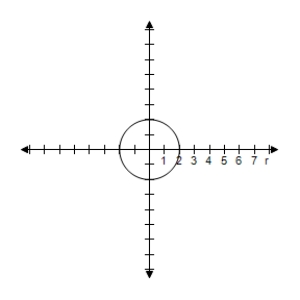
D)Symmetric with respect to ,polar axis,pole Circle with radius
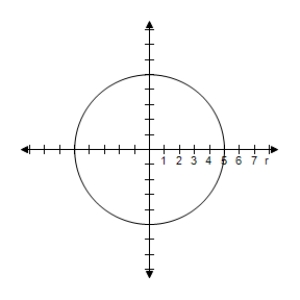
E)Symmetric with respect to ,polar axis,pole Circle with radius
A)Symmetric with respect to ,polar axis,pole Circle with radius

B)Symmetric with respect to ,polar axis,pole Circle with radius

C)Symmetric with respect to ,polar axis,pole Circle with radius

D)Symmetric with respect to ,polar axis,pole Circle with radius

E)Symmetric with respect to ,polar axis,pole Circle with radius

Symmetric with respect to ,polar axis,pole Circle with radius


4
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to the polar axis when
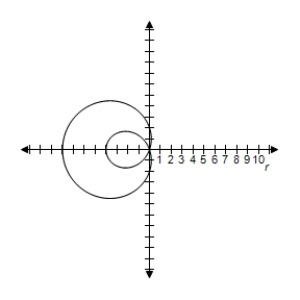
B)Symmetric with respect to the polar axis when
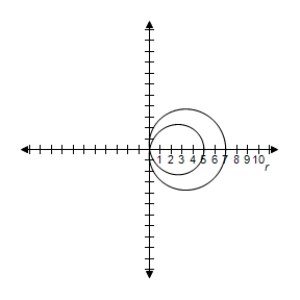
C)Symmetric with respect to the polar axis
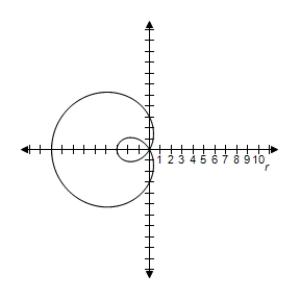
D)Symmetric with respect to the polar axis when
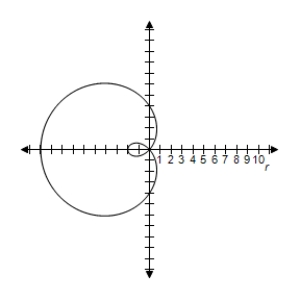
E)Symmetric with respect to the polar axis when
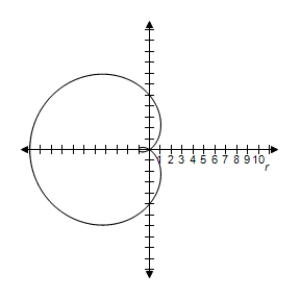
A)Symmetric with respect to the polar axis when

B)Symmetric with respect to the polar axis when

C)Symmetric with respect to the polar axis

D)Symmetric with respect to the polar axis when

E)Symmetric with respect to the polar axis when


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
5
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to when
when 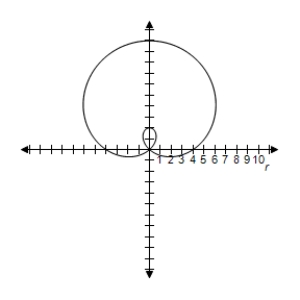
B)Symmetric with respect to when
when 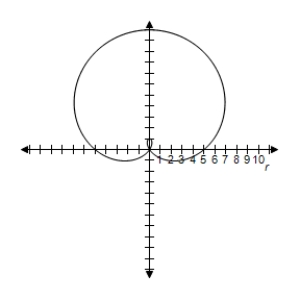
C)Symmetric with respect to when
when 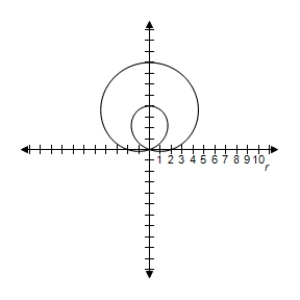
D)Symmetric with respect to when
when 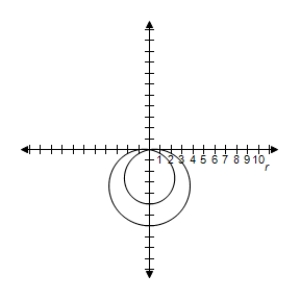
E)Symmetric with respect to when
when 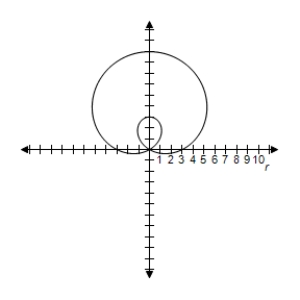
A)Symmetric with respect to when
when

B)Symmetric with respect to when
when

C)Symmetric with respect to when
when

D)Symmetric with respect to when
when

E)Symmetric with respect to when
when


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
6
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to 
B)Symmetric with respect to 
C)Symmetric with respect to 
D)Symmetric with respect to 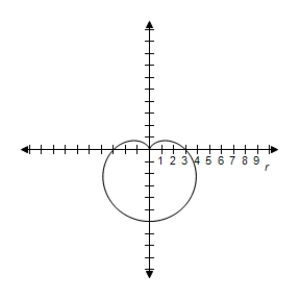
E)Symmetric with respect to 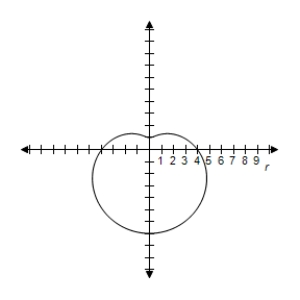
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
7
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to
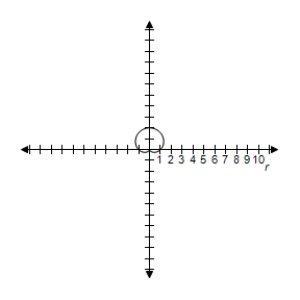
B)Symmetric with respect to 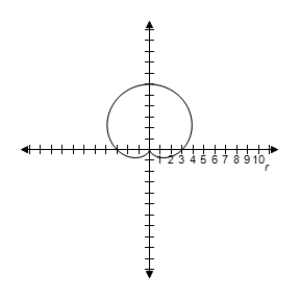
C)Symmetric with respect to 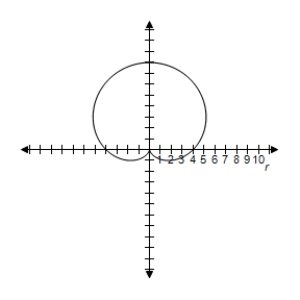
D)Symmetric with respect to 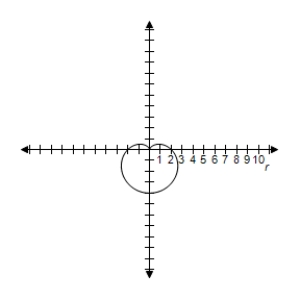
E)Symmetric with respect to 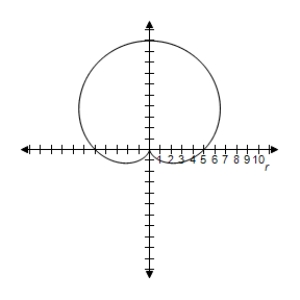
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
8
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to ,polar axis,pole Circle with radius
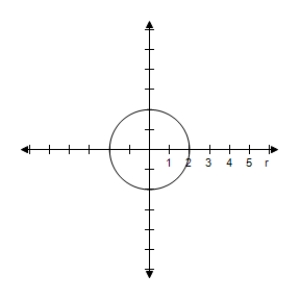
B)Symmetric with respect to ,polar axis,pole Circle with radius
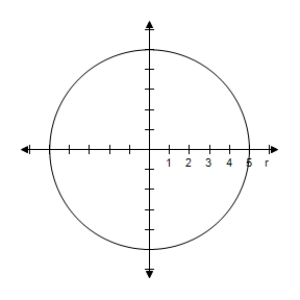
C)Symmetric with respect to ,polar axis,pole Circle with radius
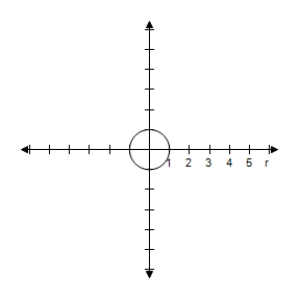
D)Symmetric with respect to ,polar axis,pole Circle with radius
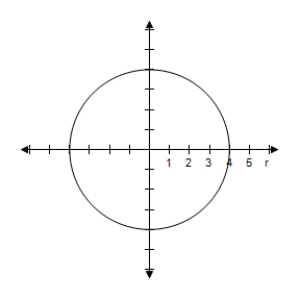
E)Symmetric with respect to ,polar axis,pole Circle with radius
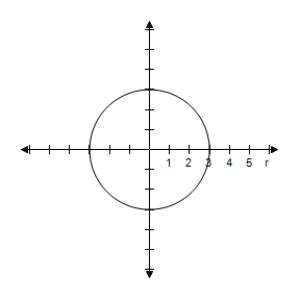
A)Symmetric with respect to ,polar axis,pole Circle with radius

B)Symmetric with respect to ,polar axis,pole Circle with radius

C)Symmetric with respect to ,polar axis,pole Circle with radius

D)Symmetric with respect to ,polar axis,pole Circle with radius

E)Symmetric with respect to ,polar axis,pole Circle with radius


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
9
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to the polar axis when
when 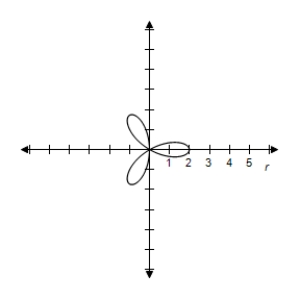
B)Symmetric with respect to the polar axis when
when 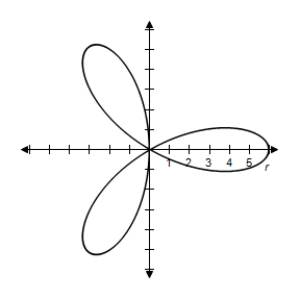
C)Symmetric with respect to the polar axis when
when 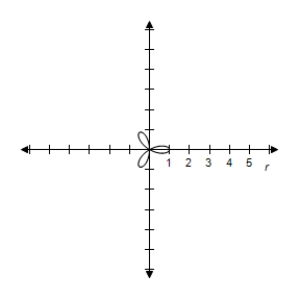
D)Symmetric with respect to the polar axis when
when 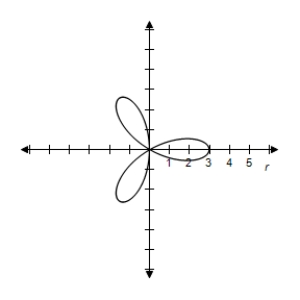
E)Symmetric with respect to the polar axis when
when 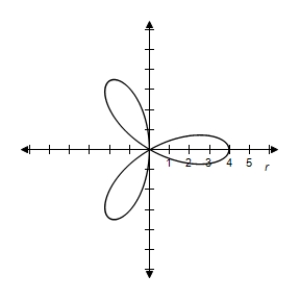
A)Symmetric with respect to the polar axis when
when

B)Symmetric with respect to the polar axis when
when

C)Symmetric with respect to the polar axis when
when

D)Symmetric with respect to the polar axis when
when

E)Symmetric with respect to the polar axis when
when


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
10
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to ,the polar axis,and the pole 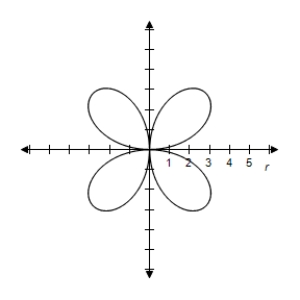
B)Symmetric with respect to ,the polar axis,and the pole 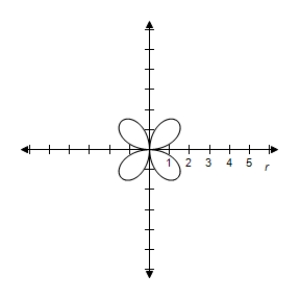
C)Symmetric with respect to ,the polar axis,and the pole 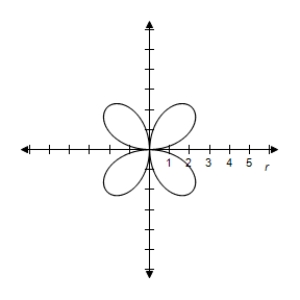
D)Symmetric with respect to ,the polar axis,and the pole 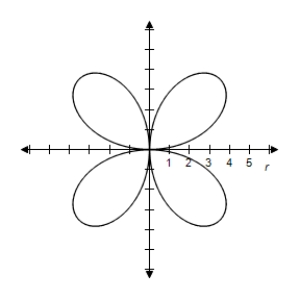
E)Symmetric with respect to ,the polar axis,and the pole 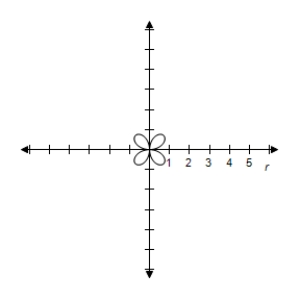
A)Symmetric with respect to ,the polar axis,and the pole

B)Symmetric with respect to ,the polar axis,and the pole

C)Symmetric with respect to ,the polar axis,and the pole

D)Symmetric with respect to ,the polar axis,and the pole

E)Symmetric with respect to ,the polar axis,and the pole


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
11
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to ,polar axis,pole Circle with radius
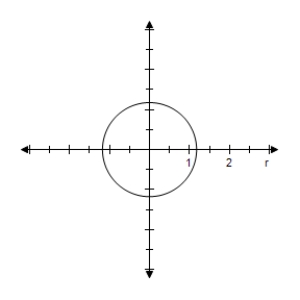
B)Symmetric with respect to ,polar axis,pole Circle with radius
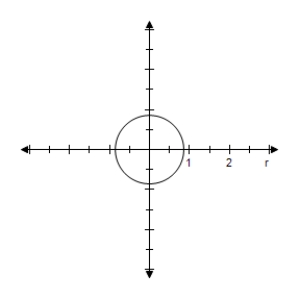
C)Symmetric with respect to ,polar axis,pole Circle with radius
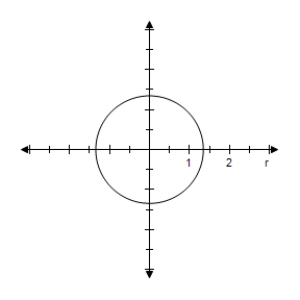
D)Symmetric with respect to ,polar axis,pole Circle with radius
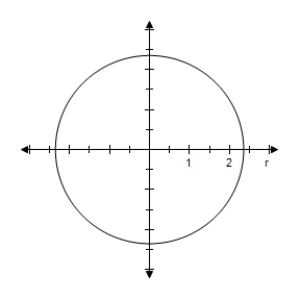
E)Symmetric with respect to ,polar axis,pole Circle with radius
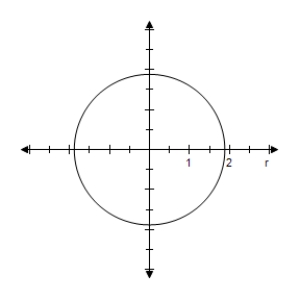
A)Symmetric with respect to ,polar axis,pole Circle with radius

B)Symmetric with respect to ,polar axis,pole Circle with radius

C)Symmetric with respect to ,polar axis,pole Circle with radius

D)Symmetric with respect to ,polar axis,pole Circle with radius

E)Symmetric with respect to ,polar axis,pole Circle with radius


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
12
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to ,polar axis,pole Circle with radius
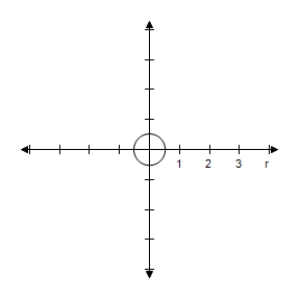
B)Symmetric with respect to ,polar axis,pole Circle with radius
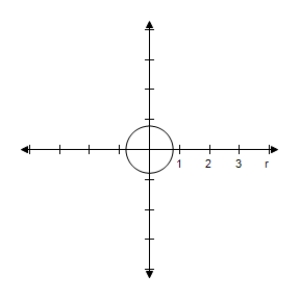
C)Symmetric with respect to ,polar axis,pole Circle with radius
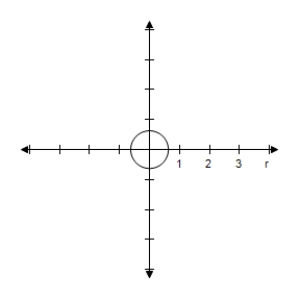
D)Symmetric with respect to ,polar axis,pole Circle with radius
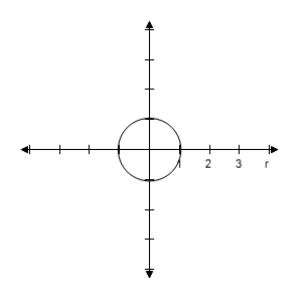
E)Symmetric with respect to ,polar axis,pole Circle with radius
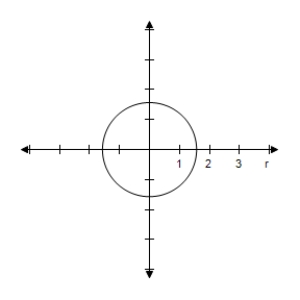
A)Symmetric with respect to ,polar axis,pole Circle with radius

B)Symmetric with respect to ,polar axis,pole Circle with radius

C)Symmetric with respect to ,polar axis,pole Circle with radius

D)Symmetric with respect to ,polar axis,pole Circle with radius

E)Symmetric with respect to ,polar axis,pole Circle with radius


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
13
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to polar axis when
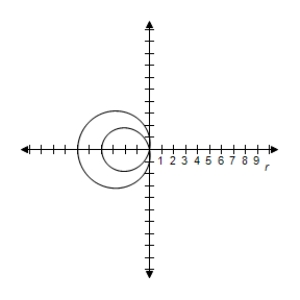
B)Symmetric with respect to polar axis when
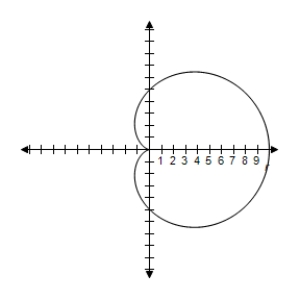
C)Symmetric with respect to polar axis when
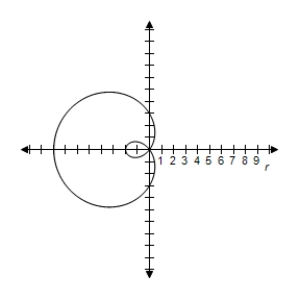
D)Symmetric with respect to polar axis when
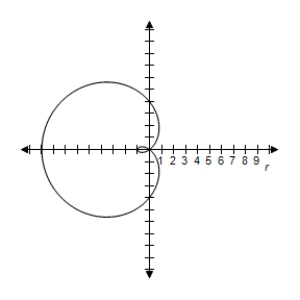
E)Symmetric with respect to polar axis when
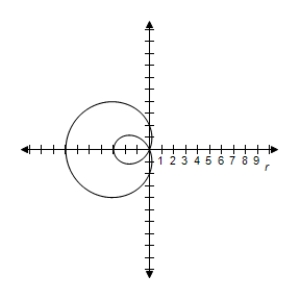
A)Symmetric with respect to polar axis when

B)Symmetric with respect to polar axis when

C)Symmetric with respect to polar axis when

D)Symmetric with respect to polar axis when

E)Symmetric with respect to polar axis when


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
14
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to 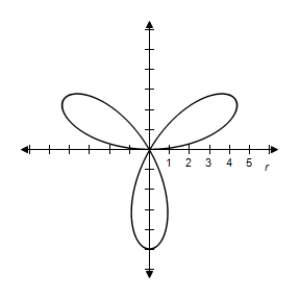
B)Symmetric with respect to 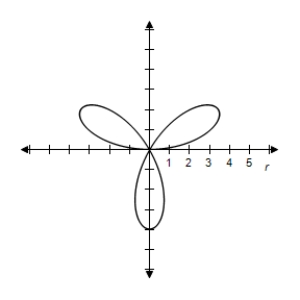
C)Symmetric with respect to 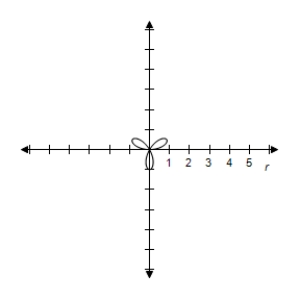
D)Symmetric with respect to 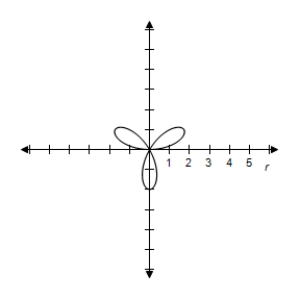
E)Symmetric with respect to 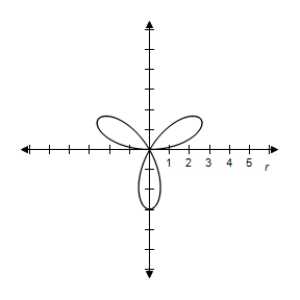
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
15
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to the polar axis 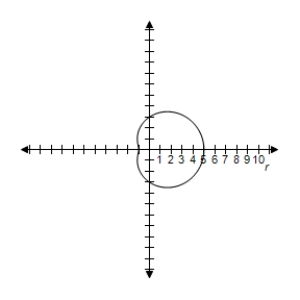
B)Symmetric with respect to the polar axis 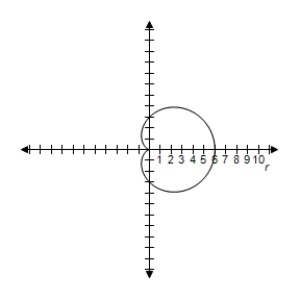
C)Symmetric with respect to the polar axis 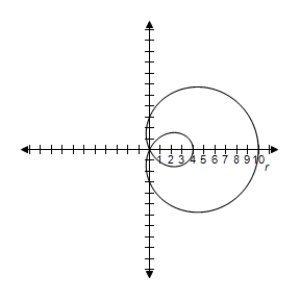
D)Symmetric with respect to the polar axis 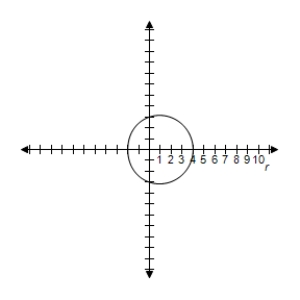
E)Symmetric with respect to the polar axis 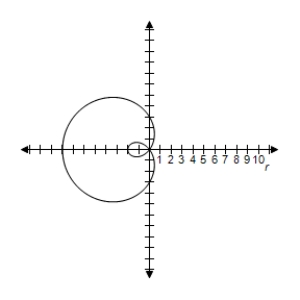
A)Symmetric with respect to the polar axis

B)Symmetric with respect to the polar axis

C)Symmetric with respect to the polar axis

D)Symmetric with respect to the polar axis

E)Symmetric with respect to the polar axis


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
16
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to polar axis Circle with radius 2.5
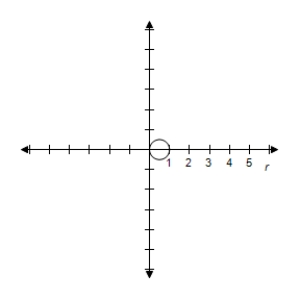
B)Symmetric with respect to polar axis Circle with radius 2.5
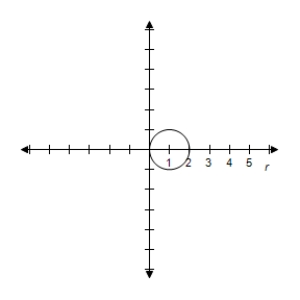
C)Symmetric with respect to polar axis Circle with radius 2.5
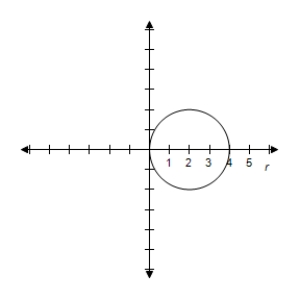
D)Symmetric with respect to polar axis Circle with radius 2.5
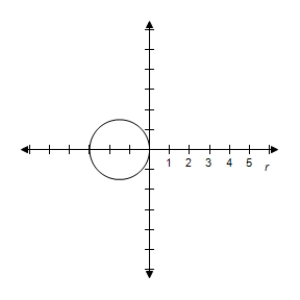
E)Symmetric with respect to polar axis Circle with radius 2.5
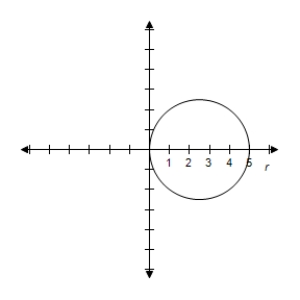
A)Symmetric with respect to polar axis Circle with radius 2.5

B)Symmetric with respect to polar axis Circle with radius 2.5

C)Symmetric with respect to polar axis Circle with radius 2.5

D)Symmetric with respect to polar axis Circle with radius 2.5

E)Symmetric with respect to polar axis Circle with radius 2.5


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
17
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to polar axis
when 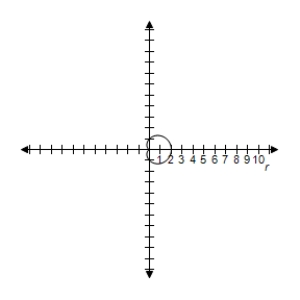
B)Symmetric with respect to polar axis
when
C)Symmetric with respect to polar axis
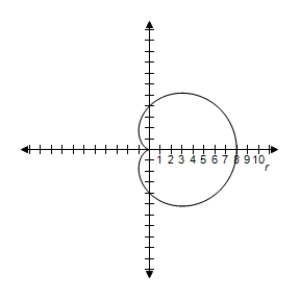
when 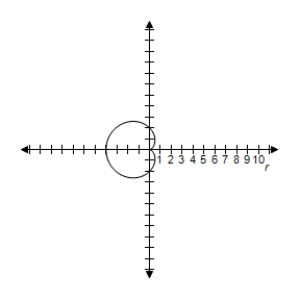
D)Symmetric with respect to polar axis
when 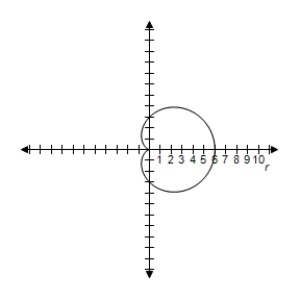
E)Symmetric with respect to polar axis
when 
A)Symmetric with respect to polar axis
when

B)Symmetric with respect to polar axis
when

C)Symmetric with respect to polar axis
when

D)Symmetric with respect to polar axis
when

E)Symmetric with respect to polar axis
when


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
18
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to 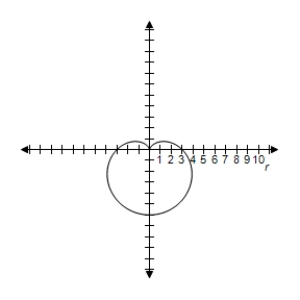
B)Symmetric with respect to 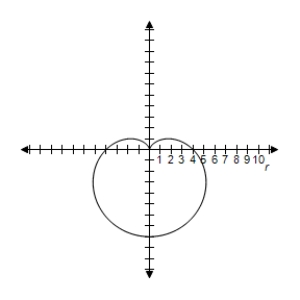
C)Symmetric with respect to 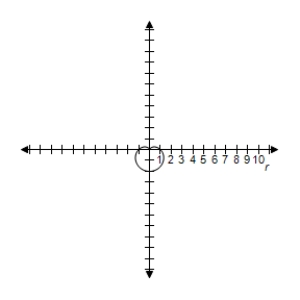
D)Symmetric with respect to 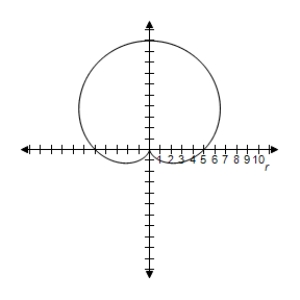
E)Symmetric with respect to 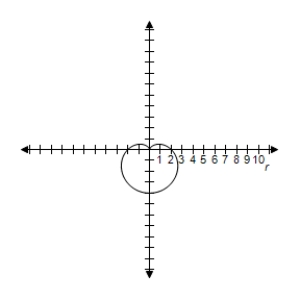
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
19
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to 
B)Symmetric with respect to 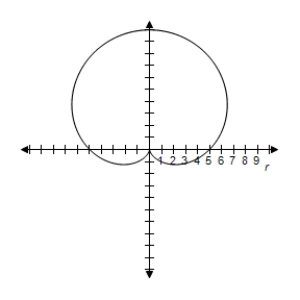
C)Symmetric with respect to 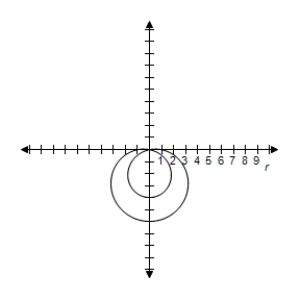
D)Symmetric with respect to 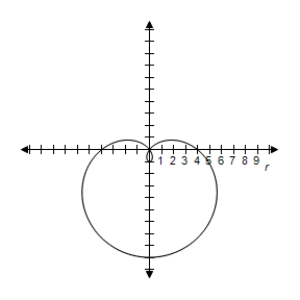
E)Symmetric with respect to 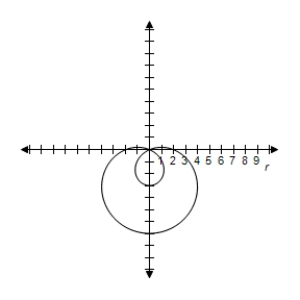
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
20
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)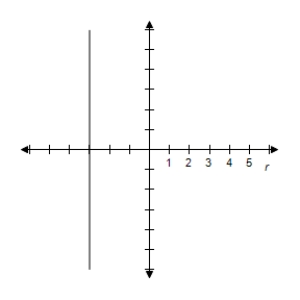
B)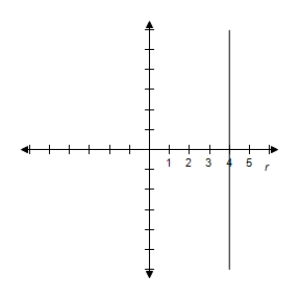
C)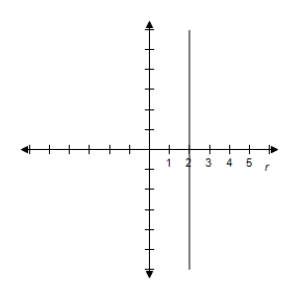
D)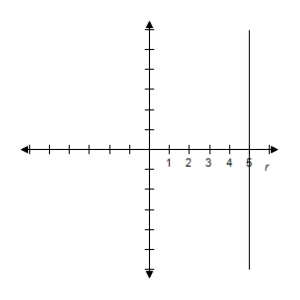
E)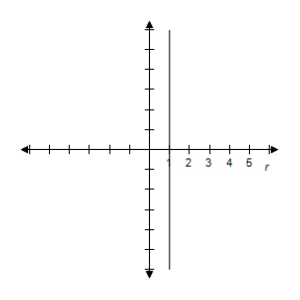
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
21
Select the graph of the equation.
A)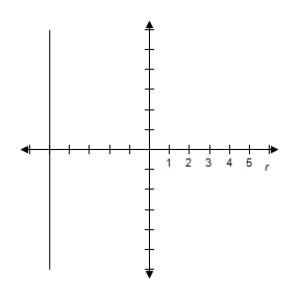
B)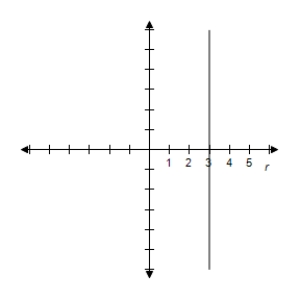
C)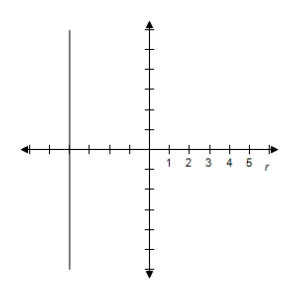
D)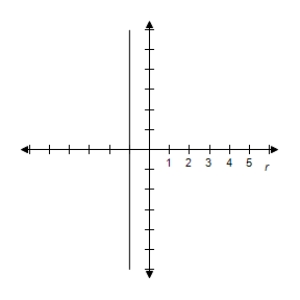
E)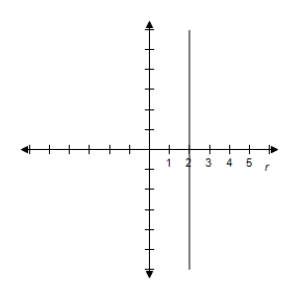
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
22
Select the graph of the equation.
A)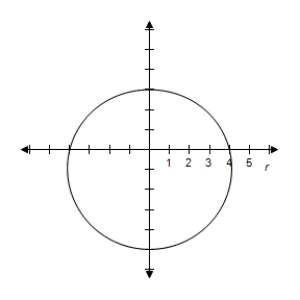
B)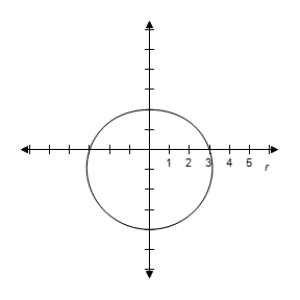
C)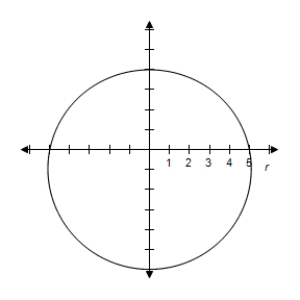
D)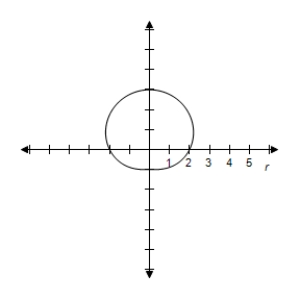
E)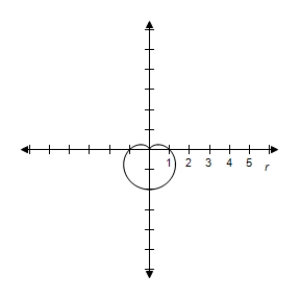
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
23
Select the graph of the equation.
A)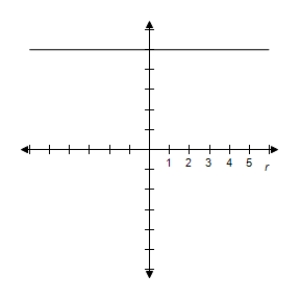
B)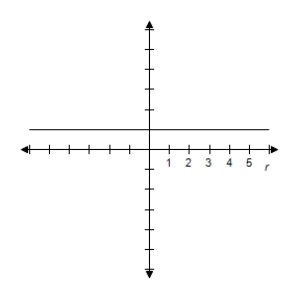
C)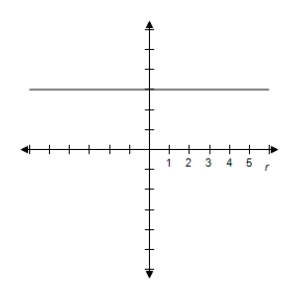
D)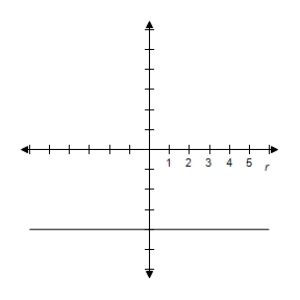
E)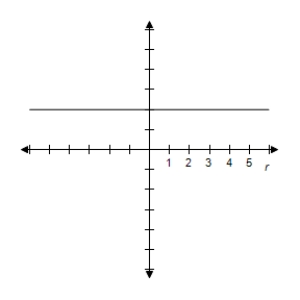
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
24
Select the correct graph of the polar equation.Find an interval for for which the graph is traced only once.
A)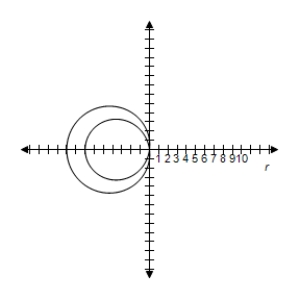
B)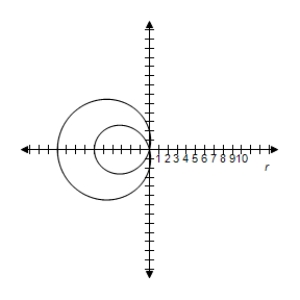
C)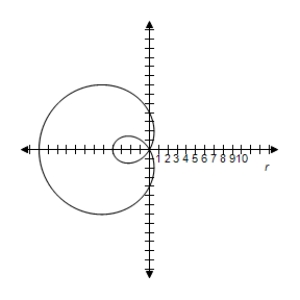
D)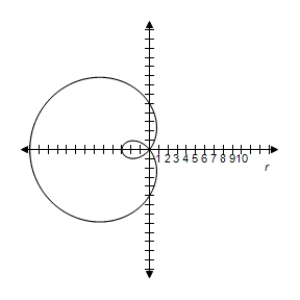
E)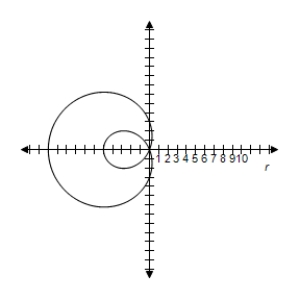
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
25
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)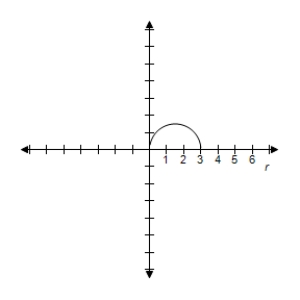 Upper half of circle
Upper half of circle
B)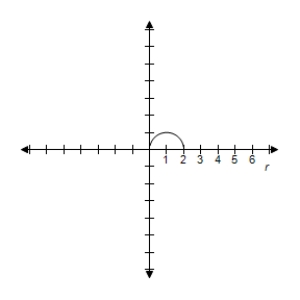 Upper half of circle
Upper half of circle
C)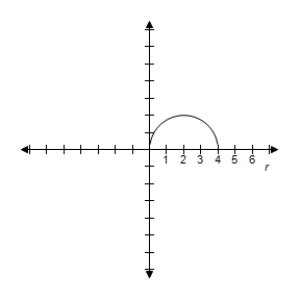 Upper half of circle
Upper half of circle
D)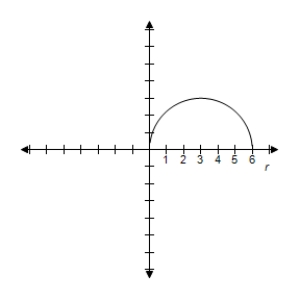 Upper half of circle
Upper half of circle
E)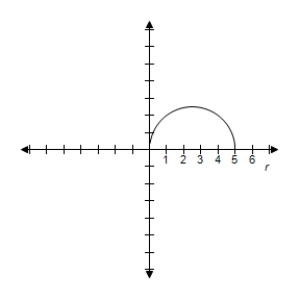 Upper half of circle
Upper half of circle
A)
 Upper half of circle
Upper half of circleB)
 Upper half of circle
Upper half of circleC)
 Upper half of circle
Upper half of circleD)
 Upper half of circle
Upper half of circleE)
 Upper half of circle
Upper half of circle
Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
26
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)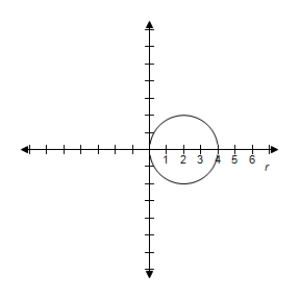 Entire circle
Entire circle
B)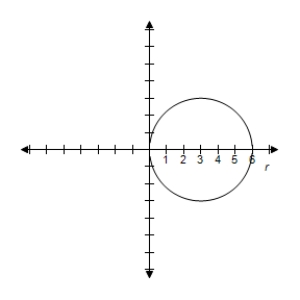 Entire circle
Entire circle
C)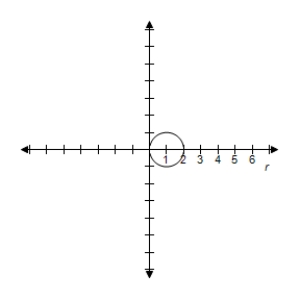 Entire circle
Entire circle
D)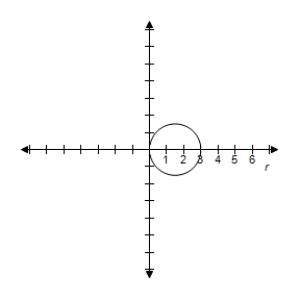 Entire circle
Entire circle
E)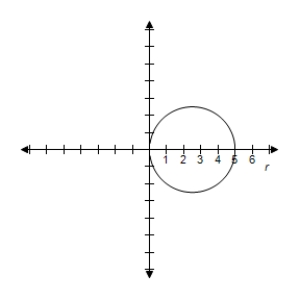 Entire circle
Entire circle
A)
 Entire circle
Entire circleB)
 Entire circle
Entire circleC)
 Entire circle
Entire circleD)
 Entire circle
Entire circleE)
 Entire circle
Entire circle
Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
27
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)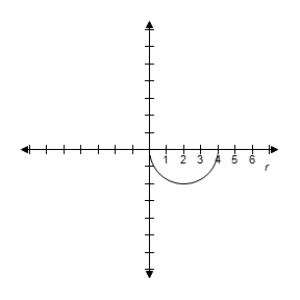 Lower half of circle
Lower half of circle
B)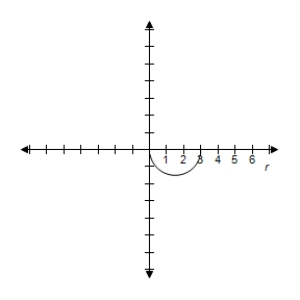 Lower half of circle
Lower half of circle
C)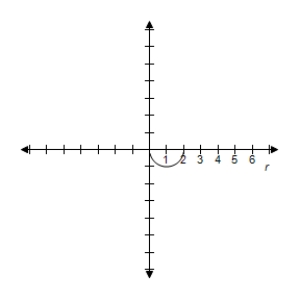 Lower half of circle
Lower half of circle
D)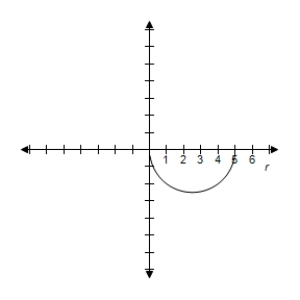 Lower half of circle
Lower half of circle
E)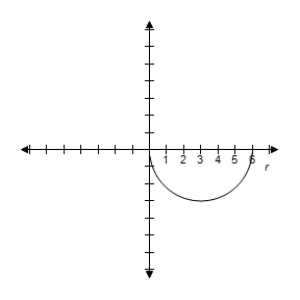 Lower half of circle
Lower half of circle
A)
 Lower half of circle
Lower half of circleB)
 Lower half of circle
Lower half of circleC)
 Lower half of circle
Lower half of circleD)
 Lower half of circle
Lower half of circleE)
 Lower half of circle
Lower half of circle
Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
28
Select the correct graph of the polar equation.Find an interval for for which the graph is traced only once.
A)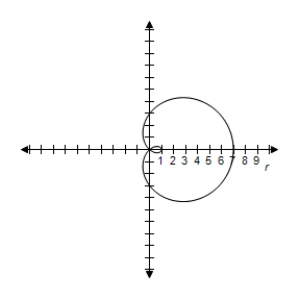
B)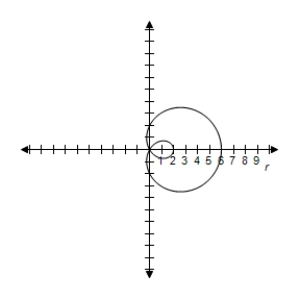
C)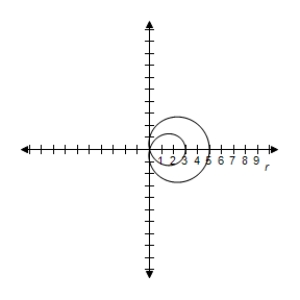
D)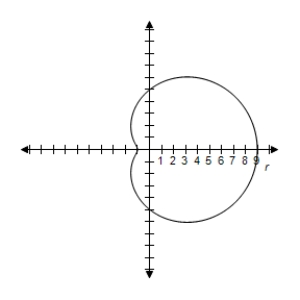
E)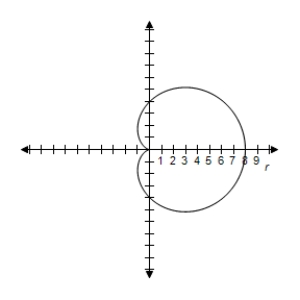
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
29
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A) 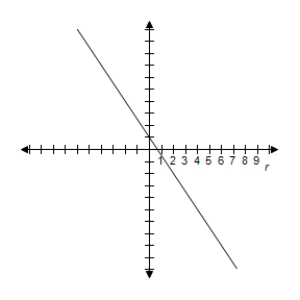
B) 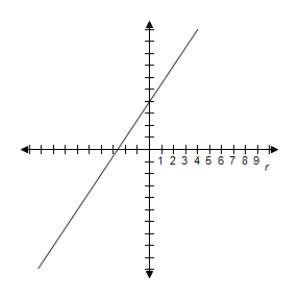
C) 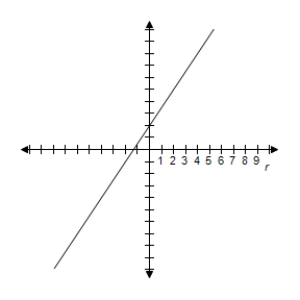
D) 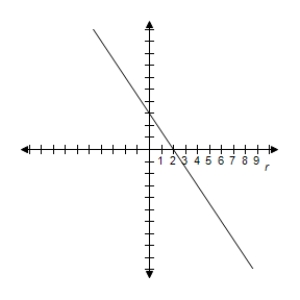
E) 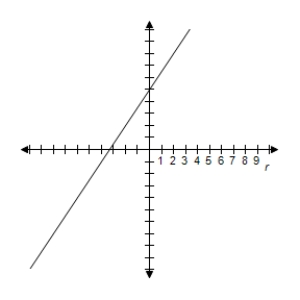
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
30
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)Symmetric with respect to the polar axis, ,and the pole
Lemniscate
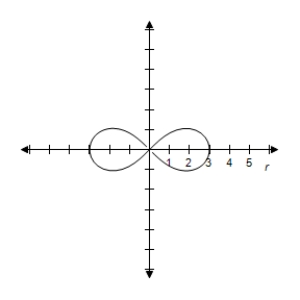
B)Symmetric with respect to the polar axis, ,and the pole
Lemniscate
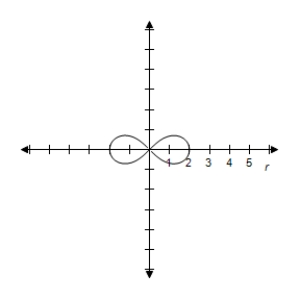
C)Symmetric with respect to the polar axis, ,and the pole
Lemniscate
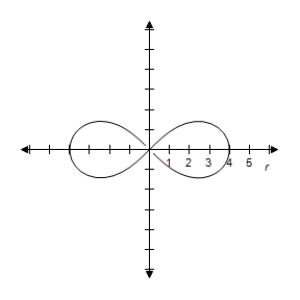
D)Symmetric with respect to the polar axis, ,and the pole
Lemniscate
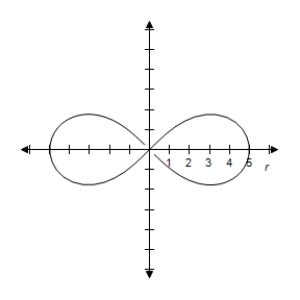
E)Symmetric with respect to the polar axis, ,and the pole
Lemniscate
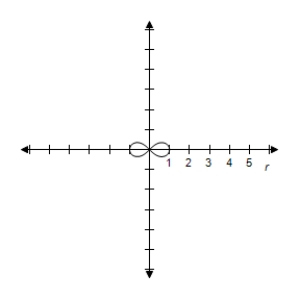
A)Symmetric with respect to the polar axis, ,and the pole
Lemniscate

B)Symmetric with respect to the polar axis, ,and the pole
Lemniscate

C)Symmetric with respect to the polar axis, ,and the pole
Lemniscate

D)Symmetric with respect to the polar axis, ,and the pole
Lemniscate

E)Symmetric with respect to the polar axis, ,and the pole
Lemniscate


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
31
Consider the equation .Select the correct graph of the equation for .
A)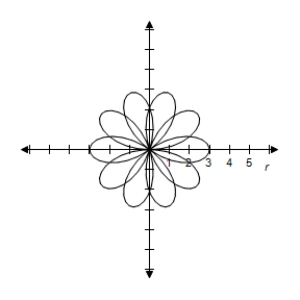
B)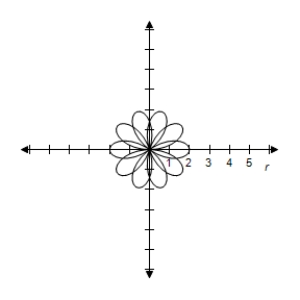
C)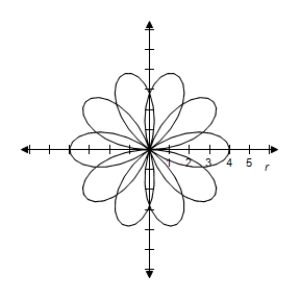
D)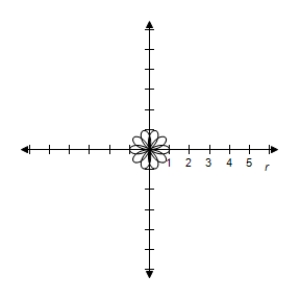
E)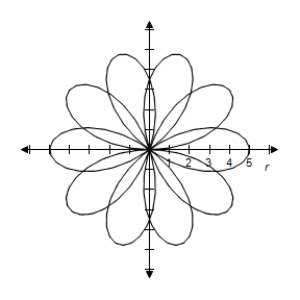
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
32
Select the graph of the equation.
A)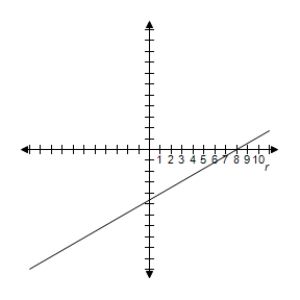
B)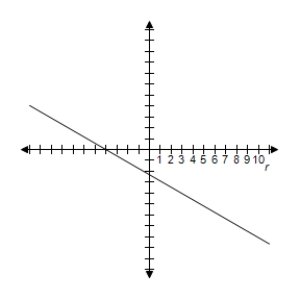
C)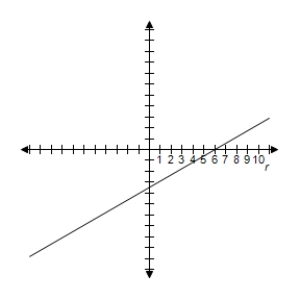
D)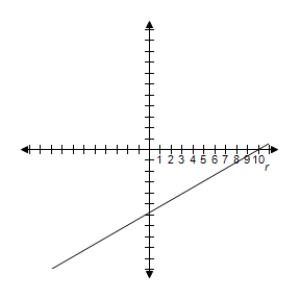
E)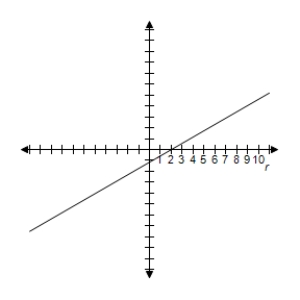
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
33
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A) 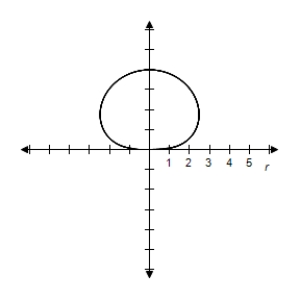
B) 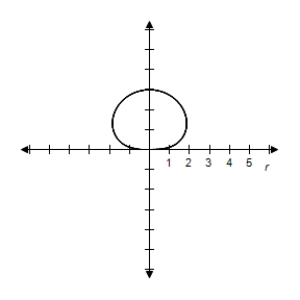
C) 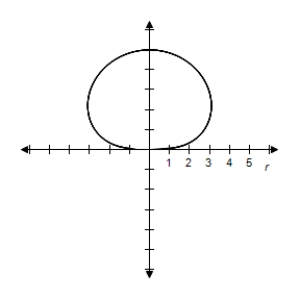
D) 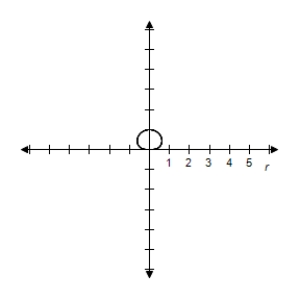
E) 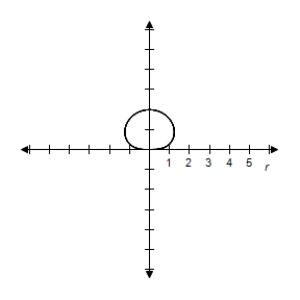
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
34
Consider the equation .Select the correct graph of the equation for .
A)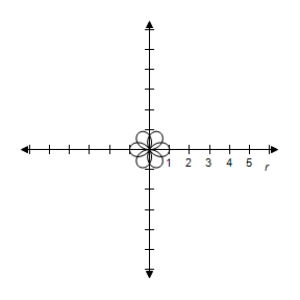
B)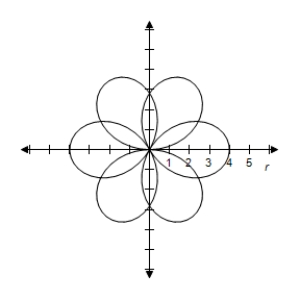
C)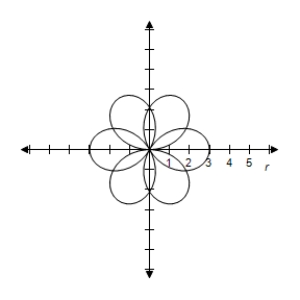
D)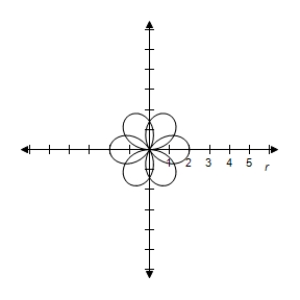
E)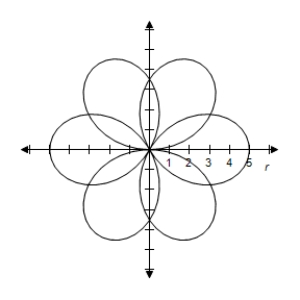
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
35
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A) 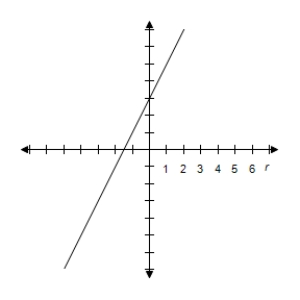
B) 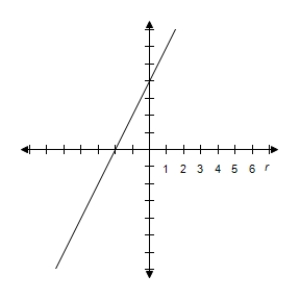
C) 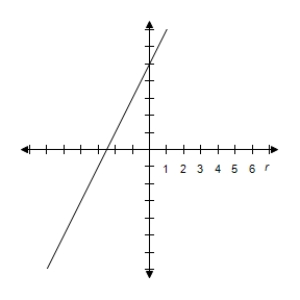
D) 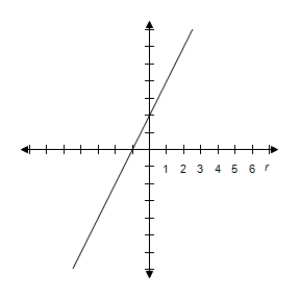
E) 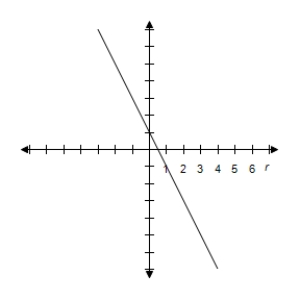
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
36
Select the correct graph of the polar equation.Find an interval for for which the graph is traced only once.
A)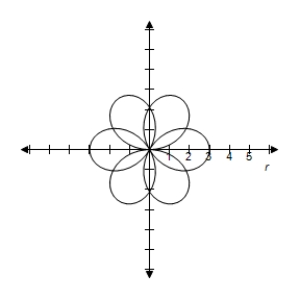
B)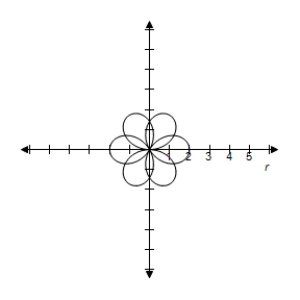
C)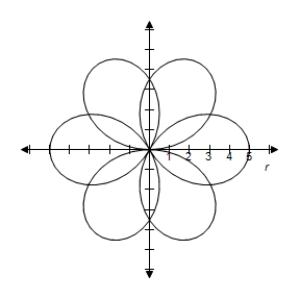
D)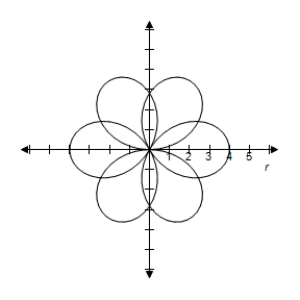
E)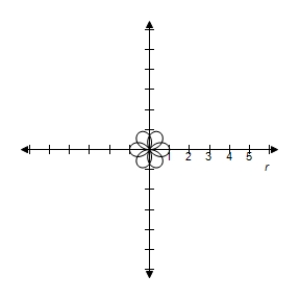
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
37
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)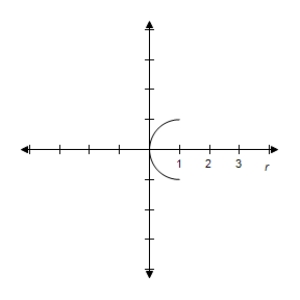 Left half of circle
Left half of circle
B)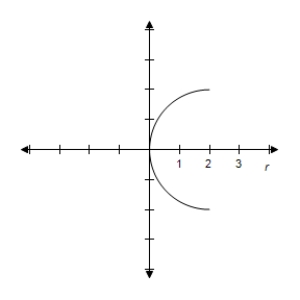 Left half of circle
Left half of circle
C)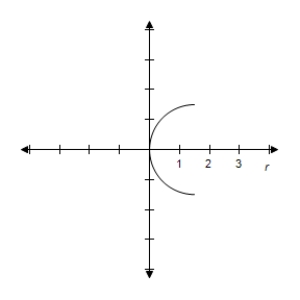 Left half of circle
Left half of circle
D)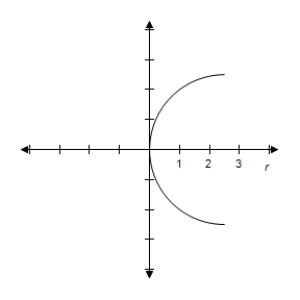 Left half of circle
Left half of circle
E)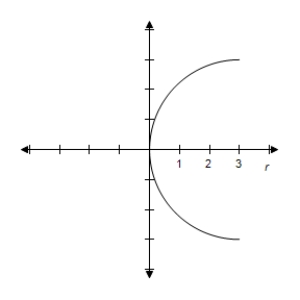 Left half of circle
Left half of circle
A)
 Left half of circle
Left half of circleB)
 Left half of circle
Left half of circleC)
 Left half of circle
Left half of circleD)
 Left half of circle
Left half of circleE)
 Left half of circle
Left half of circle
Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
38
Select the graph of the polar equation using symmetry,zeros,maximum r-values,and any other additional points.
A)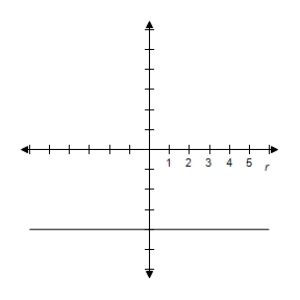
B)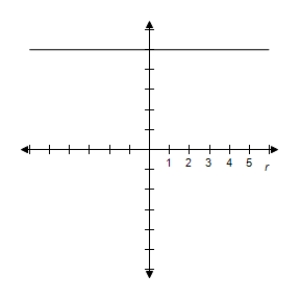
C)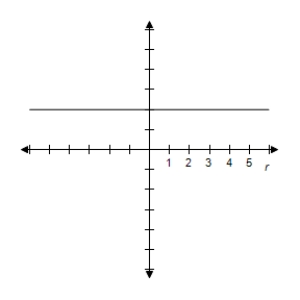
D)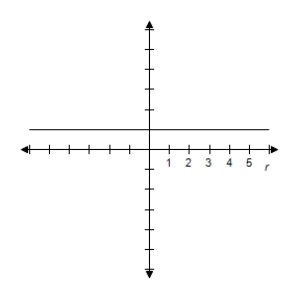
E)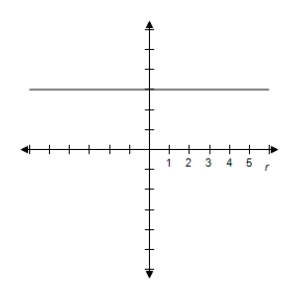
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
39
Select the graph of the equation.
A)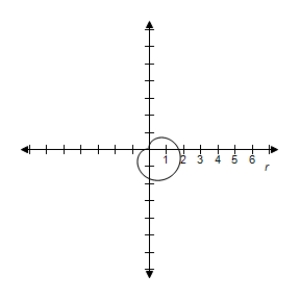
B)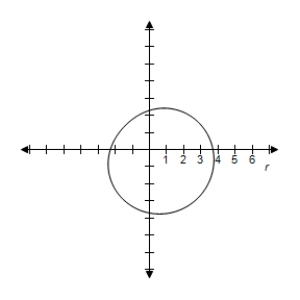
C)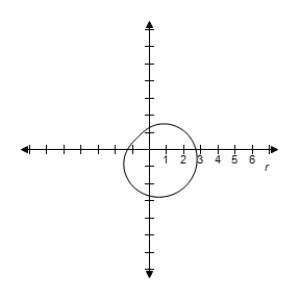
D)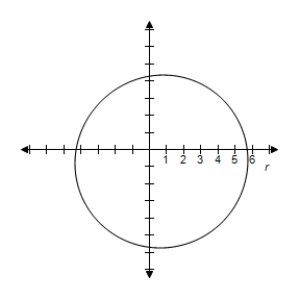
E)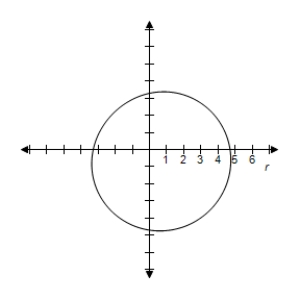
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
40
Select the graph of the equation.
A)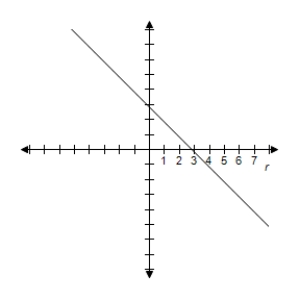
B)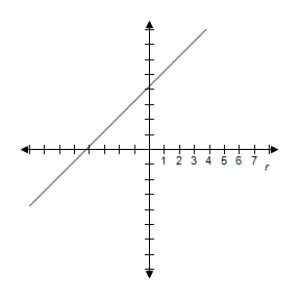
C)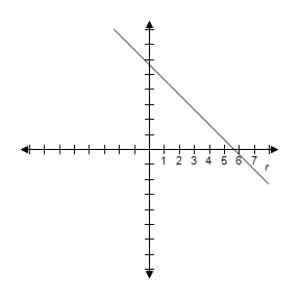
D)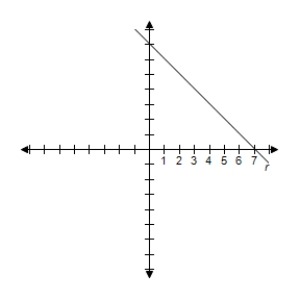
E)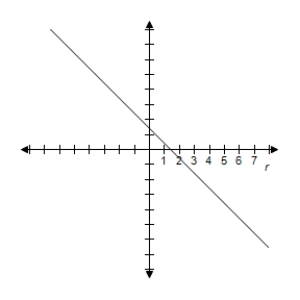
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
41
Select the correct graph of the polar equation.Find an interval for for which the graph is traced only once.
A)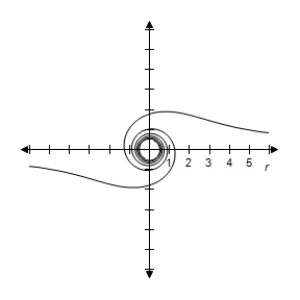
B)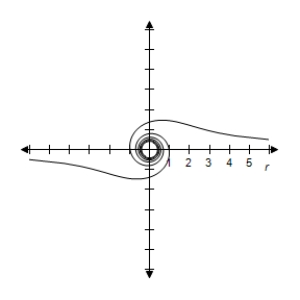
C)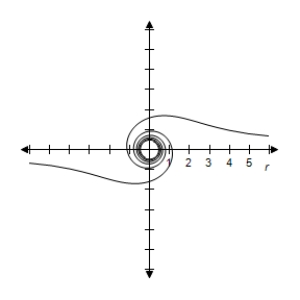
D)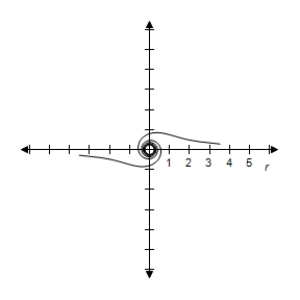
E)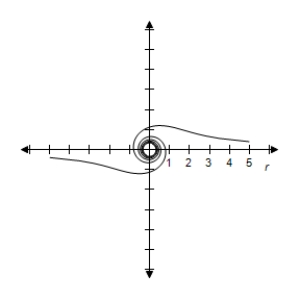
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
42
Select the correct graph of the polar equation.Describe your viewing window.
A)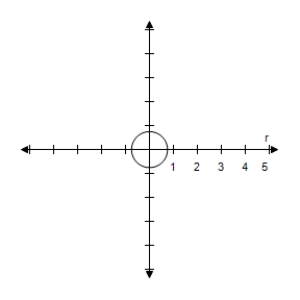
B)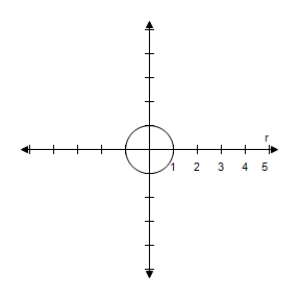
C)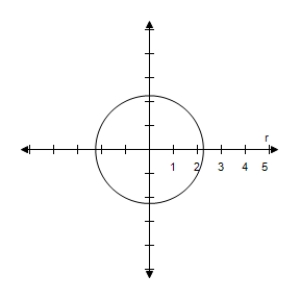
D)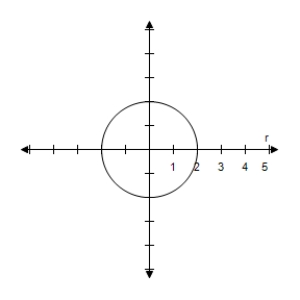
E)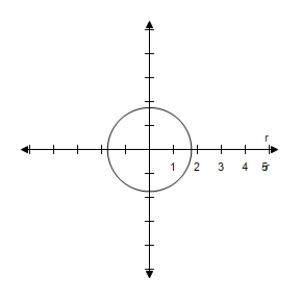
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
43
Select the correct graph of the polar equation.Describe your viewing window.
A)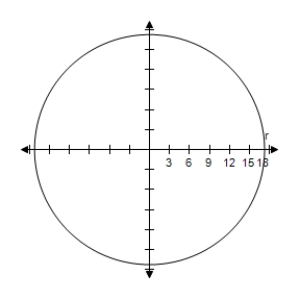
B)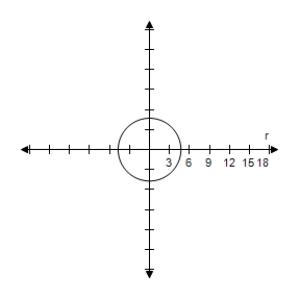
C)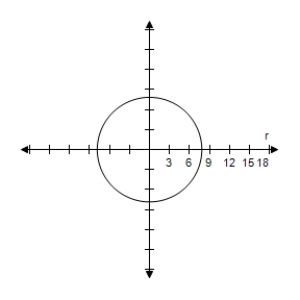
D)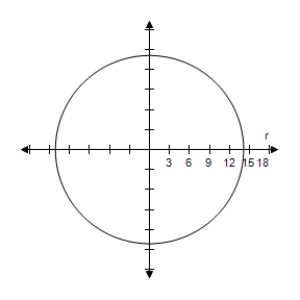
E)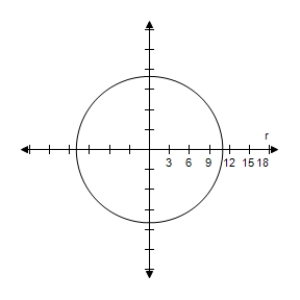
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
44
Select the correct graph of the polar equation.Describe your viewing window.
A)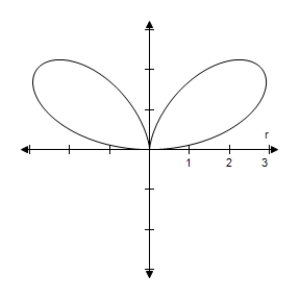
B)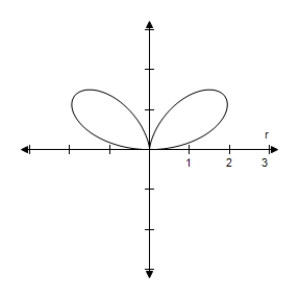
C)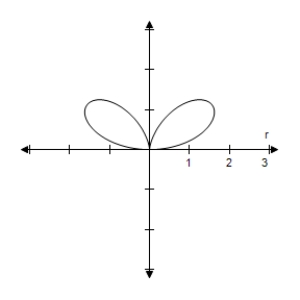
D)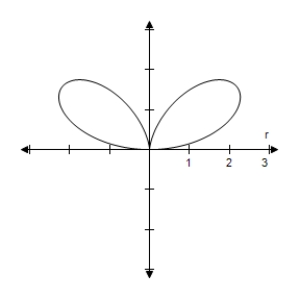
E)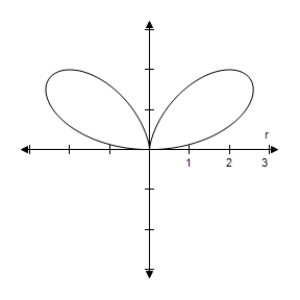
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
45
Select the correct graph of the polar equation.Describe your viewing window.
A)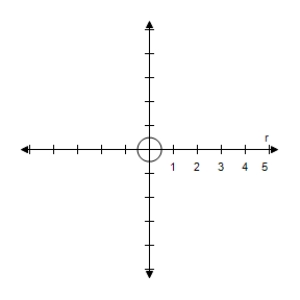
B)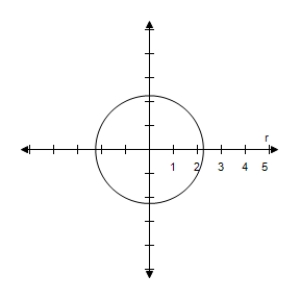
C)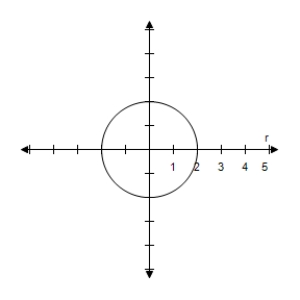
D)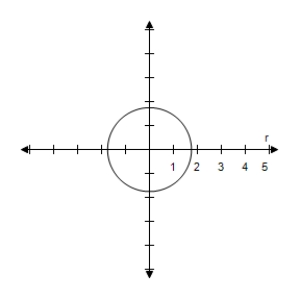
E)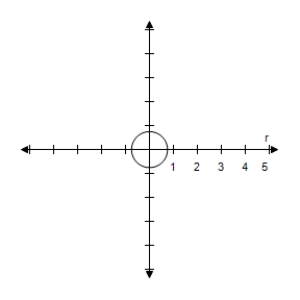
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
46
Select the correct graph of the polar equation.Find an interval for for which the graph is traced only once.
A)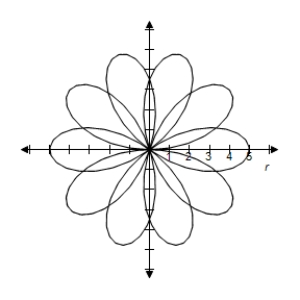
B)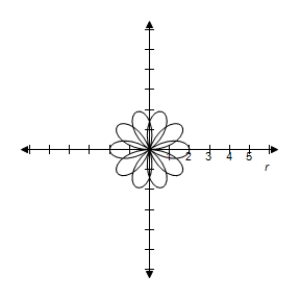
C)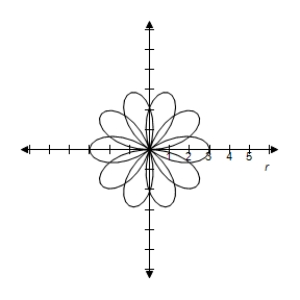
D)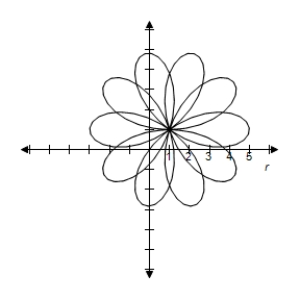
E)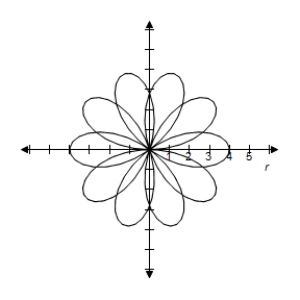
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
47
Select the correct graph of the polar equation.Find an interval for for which the graph is traced only once.
A)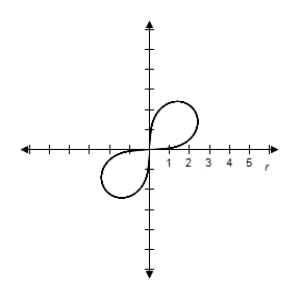
B)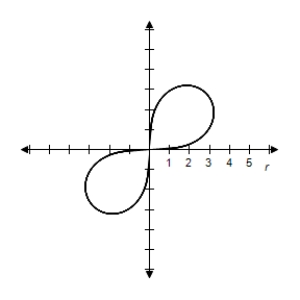
C)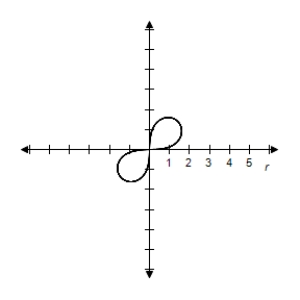
D)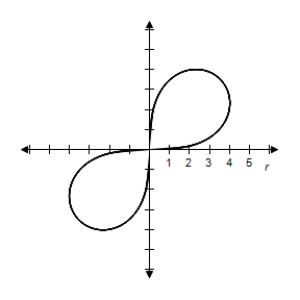
E)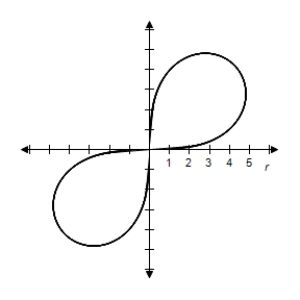
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
48
Select the correct graph of the polar equation.Describe your viewing window.
A)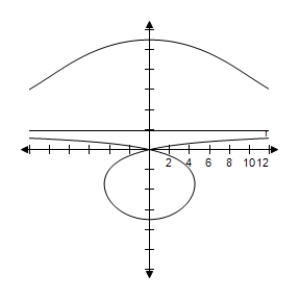
B)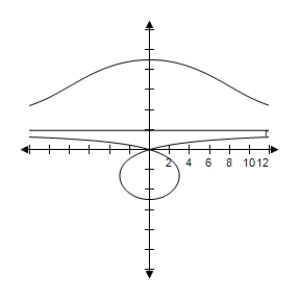
C)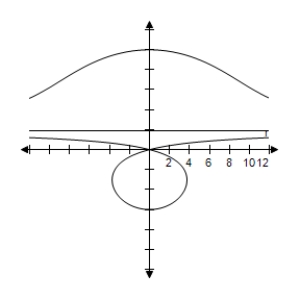
D)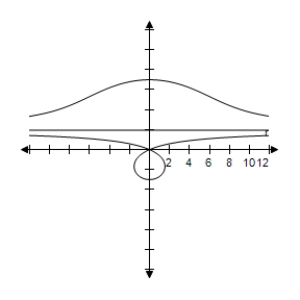
E)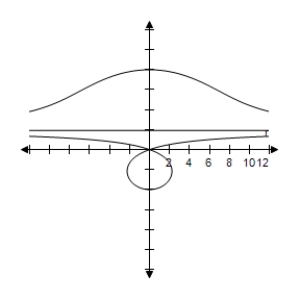
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
49
Select the correct graph of the polar equation.Describe your viewing window.
A)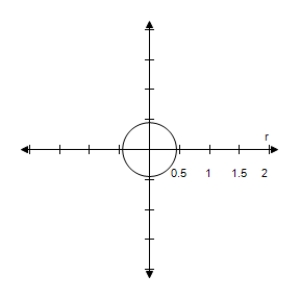
B)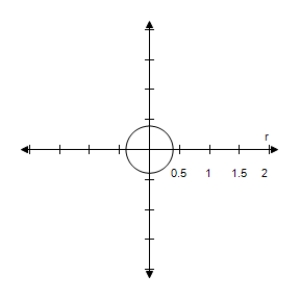
C)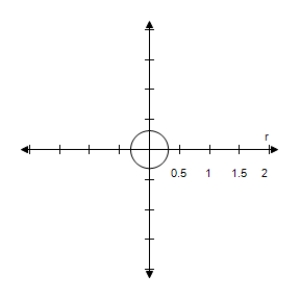
D)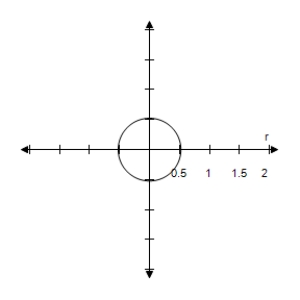
E)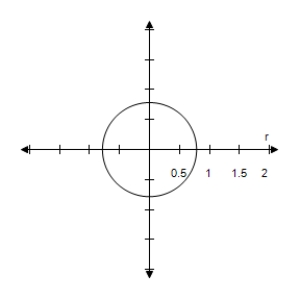
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck



