Deck 15: Zeros of Polynomial Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/49
Play
Full screen (f)
Deck 15: Zeros of Polynomial Functions
1
Find all real solutions of the polynomial equation x4 - 7x3 + 42x - 36 = 0. ?
?
A)x = 1,6,
B)x = 1,36
C)x = 1,
D)x = 1,-7,-6
E)x = 1,-36,12
?
A)x = 1,6,
B)x = 1,36
C)x = 1,
D)x = 1,-7,-6
E)x = 1,-36,12
x = 1,6,
2
A bulk food storage bin with dimensions 3 feet by 4 feet by 5 feet needs to be increased in size to hold five times as much food as the current bin.(Assume each dimension is increased by the same amount. ).Write a function that represents the volume of the new bin.
A)V(x)= x3 + 12x2 + 47x + 60 = -300
B)V(x)= x3 + 12x2 + 47x + 60 = 300
C)V(x)= x3 + 12x2 + 47x = 300
D)V(x)= x3 + 12x2 - 47x - 60 = 300
E)V(x)= x3 - 12x2 + 47x - 60 = 300
A)V(x)= x3 + 12x2 + 47x + 60 = -300
B)V(x)= x3 + 12x2 + 47x + 60 = 300
C)V(x)= x3 + 12x2 + 47x = 300
D)V(x)= x3 + 12x2 - 47x - 60 = 300
E)V(x)= x3 - 12x2 + 47x - 60 = 300
V(x)= x3 + 12x2 + 47x + 60 = 300
3
Find a polynomial function with real coefficients that has the given zeros.
1,3i
A)x3 - x2 - 9x - 9
B)x3 + x2 + 9x - 9
C)x3 - x2 + 9x - 9
D)x3 + x2 + 9x + 9
E)x3 - x2 + 9x + 9
1,3i
A)x3 - x2 - 9x - 9
B)x3 + x2 + 9x - 9
C)x3 - x2 + 9x - 9
D)x3 + x2 + 9x + 9
E)x3 - x2 + 9x + 9
x3 - x2 + 9x - 9
4
Find all the rational zeros of the function.
X3 - 18x2 + 108x - 216
A)-6
B)0,-1
C)0,6
D)6
E)±6
X3 - 18x2 + 108x - 216
A)-6
B)0,-1
C)0,6
D)6
E)±6

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
5
Find all real solutions of the polynomial equation 6x6 + x5 + 29x4 + 5x3 + 19x2 + 4x - 4 = 0.
A)x = , ,±1
B)x = , ,±2
C)x = ,
D)x = ± ,± ,±1
E)x = ± ,± ,±2
A)x = , ,±1
B)x = , ,±2
C)x = ,
D)x = ± ,± ,±1
E)x = ± ,± ,±2

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
6
Use Descartes' Rule of Signs to determine the possible number of positive and negative zeros of f(x)= 6x3 + 3x2 + 3x + 4.
A)no positive reals;no negative reals
B)1 positive real;3 negative reals or 1 negative real
C)0 positive real;3 negative reals or 1 negative real
D)3 positive reals or 1 positive real;no negative reals
E)3 positive reals or 1 positive real;3 negative reals or 1 negative real
A)no positive reals;no negative reals
B)1 positive real;3 negative reals or 1 negative real
C)0 positive real;3 negative reals or 1 negative real
D)3 positive reals or 1 positive real;no negative reals
E)3 positive reals or 1 positive real;3 negative reals or 1 negative real

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
7
Find all the real zeros of f(x)= 4x3 - 12x2 - 15x - 4.
A)x = ,2
B)x = ,
C)x = ,
D)x = ,4
E)x = ,-4
A)x = ,2
B)x = ,
C)x = ,
D)x = ,4
E)x = ,-4

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
8
Find all the rational zeros of the function f(x)= 2x4 - 12x3 - 34x2 + 300x - 400.
A)x = -4,-5,5,
B)x = 4,-5,5,2
C)x = 2,20, ,2
D)x = 2,20,-5
E)x = , ,2,5
A)x = -4,-5,5,
B)x = 4,-5,5,2
C)x = 2,20, ,2
D)x = 2,20,-5
E)x = , ,2,5

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
9
A rectangular package to be sent by a delivery service can have a maximum combined length and girth (perimeter of a cross section)of 168 inches. 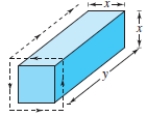 Write a function that represents the volume of the package.
Write a function that represents the volume of the package.
A)V(x)= - 4x2(42 + x)
B)V(x)= 4x2(42 + x)
C)V(x)= 4x2(42 - x)
D)V(x)= 4x2(-42 - x)
E)V(x)= - 4x2(42 - x)
 Write a function that represents the volume of the package.
Write a function that represents the volume of the package.
A)V(x)= - 4x2(42 + x)
B)V(x)= 4x2(42 + x)
C)V(x)= 4x2(42 - x)
D)V(x)= 4x2(-42 - x)
E)V(x)= - 4x2(42 - x)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
10
Find all the zeros of the function.
(t - 4)(t - 3)(t - 6i)(t + 6i)
A)-4,-3,±6i
B)4,3,6i
C)4,3,±6i
D)-4,3,±6i
E)4,-3,±6i
(t - 4)(t - 3)(t - 6i)(t + 6i)
A)-4,-3,±6i
B)4,3,6i
C)4,3,±6i
D)-4,3,±6i
E)4,-3,±6i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
11
Use Descartes' Rule of Signs to determine the possible number of positive and negative zeros of f(x)= x5 + 2x.
A)1 positive real;3 negative reals or 1 negative real
B)no positive reals;no negative reals
C)1 positive real;5 negative reals or 3 negative reals or 1 negative real
D)5 positive reals or 3 positive reals or 1 positive real;no negative reals
E)3 positive reals or 1 positive real;3 negative reals or 1 negative real
A)1 positive real;3 negative reals or 1 negative real
B)no positive reals;no negative reals
C)1 positive real;5 negative reals or 3 negative reals or 1 negative real
D)5 positive reals or 3 positive reals or 1 positive real;no negative reals
E)3 positive reals or 1 positive real;3 negative reals or 1 negative real

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
12
Use Descartes' Rule of Signs to determine the possible number of positive and negative zeros of f(x)= 6x3 - 5x2 + 6x - 6.
A)3 positive reals or 1 positive real;3 negative reals or 1 negative real
B)no positive reals;no negative reals
C)3 positive reals or 1 positive real;1 negative real
D)1 positive real;3 negative reals or 1 negative real
E)3 positive reals or 1 positive real;no negative reals
A)3 positive reals or 1 positive real;3 negative reals or 1 negative real
B)no positive reals;no negative reals
C)3 positive reals or 1 positive real;1 negative real
D)1 positive real;3 negative reals or 1 negative real
E)3 positive reals or 1 positive real;no negative reals

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
13
Find all the zeros of the function.
X(x - 5)2
A)0,5
B)1,5
C)±5
D)1,-5
E)0,-5
X(x - 5)2
A)0,5
B)1,5
C)±5
D)1,-5
E)0,-5

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
14
Find all the rational zeros of the function.
X3 + 18x2 + 105x + 200
A)-8,5
B)5,8
C)-8,-5
D)-8,-5,5
E)-5,8
X3 + 18x2 + 105x + 200
A)-8,5
B)5,8
C)-8,-5
D)-8,-5,5
E)-5,8

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
15
Use Descartes's Rule of Signs to determine the possible numbers of positive and negative zeros of the function.
4x5 - 8x
A)One negative zero
B)One negative zero or three positive zeros
C)Two negative zeros or no positive zero
D)One positive zero,one negative zero
E)One negative zero,four negative zeros
4x5 - 8x
A)One negative zero
B)One negative zero or three positive zeros
C)Two negative zeros or no positive zero
D)One positive zero,one negative zero
E)One negative zero,four negative zeros

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
16
Find all the rational zeros of the function f(x)= 2x5 - 9x4 + 9x3 - 3x2 + 7x + 6.
A)x = ,3,2
B)x = ,-1,2
C)x = ,3,1
D)x = ,
E)x = , ,2
A)x = ,3,2
B)x = ,-1,2
C)x = ,3,1
D)x = ,
E)x = , ,2

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
17
Find all the zeros of the function.
(x - 2)(x + 6)3
a.-2,6
b.
(x - 2)(x + 6)3
a.-2,6
b.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
18
Find all the real zeros of f(x)= 3x3 - 9x2 + 3x - 9.
A)x = 3
B)x = 3,
C)x = ,
D)x = ±1,3
E)x = 3,1,-9
A)x = 3
B)x = 3,
C)x = ,
D)x = ±1,3
E)x = 3,1,-9

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
19
Find all the rational zeros of the function.
X3 - 7x2 + 14x - 8
A)-1,-2,-4
B)1,2,4
C)1,-2,4
D)-1,2,4
E)-1,2,-4
X3 - 7x2 + 14x - 8
A)-1,-2,-4
B)1,2,4
C)1,-2,4
D)-1,2,4
E)-1,2,-4

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
20
A manufacturer wants to enlarge an existing manufacturing facility such that the total floor area is 1.5 times that of the current facility.The floor area of the current facility is rectangular and measures 290 feet by 80 feet.The manufacturer wants to increase each dimension by the same amount.
A)A(x)= (290 - x)(80 - x)= 34,800 ft2
B)A(x)= (290 - x)(80 + x)= 34,800 ft2
C)A(x)= (290 + x)(80 - x)= 34,800 ft2
D)A(x)= (290 + x)(80 + x)= 34,800 ft2
E)A(x)= (290 - x)(80 - x)= -34,800 ft2
A)A(x)= (290 - x)(80 - x)= 34,800 ft2
B)A(x)= (290 - x)(80 + x)= 34,800 ft2
C)A(x)= (290 + x)(80 - x)= 34,800 ft2
D)A(x)= (290 + x)(80 + x)= 34,800 ft2
E)A(x)= (290 - x)(80 - x)= -34,800 ft2

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
21
Find a polynomial function with real coefficients that has the given zeros.
6,2 + i
A)x3 - 10x2 - 29x - 30
B)x3 + 10x2 + 29x - 30
C)x3 - 10x2 + 29x - 30
D)x3 - 10x2 + 29x + 30
E)x3 + 10x2 + 29x + 30
6,2 + i
A)x3 - 10x2 - 29x - 30
B)x3 + 10x2 + 29x - 30
C)x3 - 10x2 + 29x - 30
D)x3 - 10x2 + 29x + 30
E)x3 + 10x2 + 29x + 30

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
22
Find a polynomial function with real coefficients that has the given zeros.
2,4 + 3i
A)x3 - 10x2 + 41x + 50
B)x3 + 10x2 + 41x + 50
C)x3 - 10x2 + 41x - 50
D)x3 - 10x2 - 41x - 50
E)x3 + 10x2 + 41x - 50
2,4 + 3i
A)x3 - 10x2 + 41x + 50
B)x3 + 10x2 + 41x + 50
C)x3 - 10x2 + 41x - 50
D)x3 - 10x2 - 41x - 50
E)x3 + 10x2 + 41x - 50

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
23
Find all the zeros of the function and write the polynomial as a product of linear factors.
X3 - 6x2 + x + 164
A)-4,5 ± 4i; (x + 4)(x - 5 + 4i)(x - 5 - 4i)
B)-4,5 ± 4i; (x + 4)(x - 5 + 4i)(x + 5 - 4i)
C)4,5 ± 4i; (x - 4)(x - 5 + 4i)(x - 5 - 4i)
D)-4,5 ± 4i; (x + 4)(x + 5 + 4i)(x + 5 - 4i)
E)-4,5 ± 4i; (x + 4)(x - 5 - 4i)(x - 5 - 4i)
X3 - 6x2 + x + 164
A)-4,5 ± 4i; (x + 4)(x - 5 + 4i)(x - 5 - 4i)
B)-4,5 ± 4i; (x + 4)(x - 5 + 4i)(x + 5 - 4i)
C)4,5 ± 4i; (x - 4)(x - 5 + 4i)(x - 5 - 4i)
D)-4,5 ± 4i; (x + 4)(x + 5 + 4i)(x + 5 - 4i)
E)-4,5 ± 4i; (x + 4)(x - 5 - 4i)(x - 5 - 4i)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
24
Use the given zero to find all the zeros of the function.
Function Zero
X3 - 3x2 + 16x - 48 4i
A)±4i,±3
B)±4i,-3
C)±4i,3
D)4,±3
E)±4i,±4
Function Zero
X3 - 3x2 + 16x - 48 4i
A)±4i,±3
B)±4i,-3
C)±4i,3
D)4,±3
E)±4i,±4

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
25
Write the polynomial as the product of linear and quadratic factors that are irreducible over the reals. ?
F(x)= x4 + 42x2 - 343
?
A)
B)
C)
D)
E)
F(x)= x4 + 42x2 - 343
?
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
26
Write the polynomial in completely factored form. ?
F(x)= x4 + 20x2 - 125
?
A)
B)
C)
D)
E)
F(x)= x4 + 20x2 - 125
?
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
27
Find all the zeros of the function and write the polynomial as a product of linear factors.
Y4 - 2401
A)±7; (y - 7)(y + 7)
B)±7,±7i; (y - 7)(y + 7)(y - 7i)(y + 7i)
C)7,7i; (y + 7)(y + 7)(y - 7i)(y - 7i)
D)±7i; (y - 7i)(y + 7i)
E)±7,±7i; (y - 7)(y - 7)(y + 7i)(y + 7i)
Y4 - 2401
A)±7; (y - 7)(y + 7)
B)±7,±7i; (y - 7)(y + 7)(y - 7i)(y + 7i)
C)7,7i; (y + 7)(y + 7)(y - 7i)(y - 7i)
D)±7i; (y - 7i)(y + 7i)
E)±7,±7i; (y - 7)(y - 7)(y + 7i)(y + 7i)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
28
Find a polynomial function with real coefficients that has the given zeros.
6,-6i
A)x3 - 6x2 - 36x - 216
B)x3 - 6x2 + 36x + 216
C)x3 + 6x2 + 36x + 216
D)x3 - 6x2 + 36x - 216
E)x3 + 6x2 + 36x - 216
6,-6i
A)x3 - 6x2 - 36x - 216
B)x3 - 6x2 + 36x + 216
C)x3 + 6x2 + 36x + 216
D)x3 - 6x2 + 36x - 216
E)x3 + 6x2 + 36x - 216

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
29
Use the given zero to find all the zeros of the function. ?
Function Zero
7x3 + 8x2 + 175x + 200 5i
?
?
A)±5i,
B)±5i,
C)±5i,-8
D)±5i,
E)±5i,
Function Zero
7x3 + 8x2 + 175x + 200 5i
?
?
A)±5i,
B)±5i,
C)±5i,-8
D)±5i,
E)±5i,

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
30
Find all zeros of the function f(x)= (x - 4)(x + 3)[x + (4 + 3i)][x - (4 - 3i)].
A)x = 4,-3,-4 - 3i,4 - 3i
B)x = -4,3,4 - 3i,4 + 3i
C)x = -4,3,4 + 3i,-4 - 3i
D)x = 4,-3,4 + 3i,-4 + 3i
E)x = 4,-3,-4 - 3i,4 + 3i
A)x = 4,-3,-4 - 3i,4 - 3i
B)x = -4,3,4 - 3i,4 + 3i
C)x = -4,3,4 + 3i,-4 - 3i
D)x = 4,-3,4 + 3i,-4 + 3i
E)x = 4,-3,-4 - 3i,4 + 3i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
31
Find all the zeros of the function and write the polynomial as a product of linear factors.
X4 - 81
A)3,3i; (x + 3)(x + 3)(x - 3i)(x - 3i)
B)±3i; (x - 3i)(x + 3i)
C)±3,±3i; (x - 3)(x - 3)(x + 3i)(x + 3i)
D)±3; (x - 3)(x + 3)
E)±3,±3i; (x - 3)(x + 3)(x - 3i)(x + 3i)
X4 - 81
A)3,3i; (x + 3)(x + 3)(x - 3i)(x - 3i)
B)±3i; (x - 3i)(x + 3i)
C)±3,±3i; (x - 3)(x - 3)(x + 3i)(x + 3i)
D)±3; (x - 3)(x + 3)
E)±3,±3i; (x - 3)(x + 3)(x - 3i)(x + 3i)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
32
Write the number of rational and irrational zeros of the given cubic function.
X3 - 2
A)Rational zeros: 1;irrational zeros: 0
B)Rational zeros: 1;irrational zeros: 2
C)Rational zeros: 1;irrational zeros: 1
D)Rational zeros: 0;irrational zeros: 1
E)Rational zeros: 0;irrational zeros: 0
X3 - 2
A)Rational zeros: 1;irrational zeros: 0
B)Rational zeros: 1;irrational zeros: 2
C)Rational zeros: 1;irrational zeros: 1
D)Rational zeros: 0;irrational zeros: 1
E)Rational zeros: 0;irrational zeros: 0

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
33
Write the polynomial as the product of factors that are irreducible over the rationals. ?
F(x)= x4 + 6x2 - 27
?
A)
B)
C)
D)
E)
F(x)= x4 + 6x2 - 27
?
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
34
Find all zeros of the function f(x)= x2(x + 1)(x3 - 64).
A)x = 0,1,-4
B)x = 0,-1,4
C)x = -1,64
D)x = 1,-64
E)x = 0,-1,4, ,
A)x = 0,1,-4
B)x = 0,-1,4
C)x = -1,64
D)x = 1,-64
E)x = 0,-1,4, ,

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
35
Find all the zeros of the function and write the polynomial as a product of linear factors.
X2 + 49
A)7i; (x + 7i)(x - 7i)
B)-7i; (x + 7i)(x - 7i)
C)±7i; (x + 7)(x - 7)
D)-7i; (x + 7)(x - 7)
E)±7i; (x + 7i)(x - 7i)
X2 + 49
A)7i; (x + 7i)(x - 7i)
B)-7i; (x + 7i)(x - 7i)
C)±7i; (x + 7)(x - 7)
D)-7i; (x + 7)(x - 7)
E)±7i; (x + 7i)(x - 7i)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
36
Find all the zeros of the function.
X3 + 7x2 + 32x + 60
A)-4,-3 ± 2i
B)-3,-2 ± 4i
C)-3,2 ± 4i
D)-3,2 ± i
E)-3,-4 ± 2i
X3 + 7x2 + 32x + 60
A)-4,-3 ± 2i
B)-3,-2 ± 4i
C)-3,2 ± 4i
D)-3,2 ± i
E)-3,-4 ± 2i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
37
Find all zeros of the function f(x)= (x - 4)(x + 4i)(x - 4i).
A)x = 4,-4i,4i
B)x = 4
C)x = 4,4i
D)x = 4,-4,4
E)x = -4,-4i,4i
A)x = 4,-4i,4i
B)x = 4
C)x = 4,4i
D)x = 4,-4,4
E)x = -4,-4i,4i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
38
Use the given zero to find all the zeros of the function.
Function Zero
X3 + 13x2 + 59x + 87 -5 - 2i
A)3,5 ± 2i
B)±3i,-5 ± 2i
C)-3,5 ± 2i
D)-3,-5 ± 2i
E)3,-5 ± 2i
Function Zero
X3 + 13x2 + 59x + 87 -5 - 2i
A)3,5 ± 2i
B)±3i,-5 ± 2i
C)-3,5 ± 2i
D)-3,-5 ± 2i
E)3,-5 ± 2i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
39
Find all the zeros of the function and write the polynomial as a product of linear factors.
X3 - 10x2 + 29x - 30
A)2 ± i;6; (x - 2 + i)(x + 2 - i)(x - 6)
B)2 ± i;6; (x - 2 + i)(x - 2 + i)(x - 6)
C)-2 ± i;6; (x + 2 + i)(x + 2 - i)(x - 6)
D)2 ± i;-6; (x - 2 + i)(x - 2 - i)(x + 6)
E)2 ± i;6; (x - 2 + i)(x - 2 - i)(x - 6)
X3 - 10x2 + 29x - 30
A)2 ± i;6; (x - 2 + i)(x + 2 - i)(x - 6)
B)2 ± i;6; (x - 2 + i)(x - 2 + i)(x - 6)
C)-2 ± i;6; (x + 2 + i)(x + 2 - i)(x - 6)
D)2 ± i;-6; (x - 2 + i)(x - 2 - i)(x + 6)
E)2 ± i;6; (x - 2 + i)(x - 2 - i)(x - 6)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
40
Find all the zeros of the function and write the polynomial as a product of linear factors.
X4 + 13x2 + 36
A)±3i,±2i; (x + 3i)(x - 3i)(x + 2)(x - 2)
B)±3i,±2i; (x + 3i)(x - 3i)(x + 2i)(x - 2i)
C)-3i,-2i; (x - 3i)(x - 3i)(x - 2i)(x - 2i)
D)3i,2i; (x + 3i)(x + 3i)(x + 2i)(x + 2i)
E)±3i,±2i; (x + 3)(x - 3)(x + 2i)(x - 2i)
X4 + 13x2 + 36
A)±3i,±2i; (x + 3i)(x - 3i)(x + 2)(x - 2)
B)±3i,±2i; (x + 3i)(x - 3i)(x + 2i)(x - 2i)
C)-3i,-2i; (x - 3i)(x - 3i)(x - 2i)(x - 2i)
D)3i,2i; (x + 3i)(x + 3i)(x + 2i)(x + 2i)
E)±3i,±2i; (x + 3)(x - 3)(x + 2i)(x - 2i)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
41
Use the zero or root feature of a graphing utility to approximate the zeros of the function f(x)= x6 - 9x4 + 11x2 + 21 accurate to the nearest thousandth.
A)2.646,1.732
B)±1,-2.646,1.732
C)±i,-2.646,-1.732
D)±1,2.646,1.732
E)±i,±2.646,±1.732
A)2.646,1.732
B)±1,-2.646,1.732
C)±i,-2.646,-1.732
D)±1,2.646,1.732
E)±i,±2.646,±1.732

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
42
Given 3 + i is a root,determine all other roots of f(x)= x4 - 12x3 + 59 x2 - 138x + 130.
A)x = 3 - i,3 ± 2i
B)x = 3 - i,2 ± 3i
C)x = 3 + i,3 ± 2i,2 - i
D)x = 3 - i,2 ± i
E)x = 3 - i,3 - 2i,2 - i
A)x = 3 - i,3 ± 2i
B)x = 3 - i,2 ± 3i
C)x = 3 + i,3 ± 2i,2 - i
D)x = 3 - i,2 ± i
E)x = 3 - i,3 - 2i,2 - i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
43
Given -6i is a root,determine all other roots of f(x)= x3 + 2x2 + 36x + 72.
A)x = -2,±6
B)x = -2,6i
C)x = -6,±2i
D)x = ±2,6i
E)x = ±2,6
A)x = -2,±6
B)x = -2,6i
C)x = -6,±2i
D)x = ±2,6i
E)x = ±2,6

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
44
Write f(x)= x3 - 5x2 + 16x - 80 as a product of linear factors.
A)f(x)= (x - 5)(x - 4)2
B)f(x)= (x - 5)(x + 4i)(x - 4i)
C)f(x)= (x - 5)2(x - 4i)
D)f(x)= (x - 5)(x + 4)2
E)f(x)= (x + 5)(x - 5)(x + 4)
A)f(x)= (x - 5)(x - 4)2
B)f(x)= (x - 5)(x + 4i)(x - 4i)
C)f(x)= (x - 5)2(x - 4i)
D)f(x)= (x - 5)(x + 4)2
E)f(x)= (x + 5)(x - 5)(x + 4)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
45
Find a polynomial with real coefficients that has zeros -1,3i,and -3i.
A)x3 + 9x2 + x + 9
B)x3 + x2 - 9x - 9
C)x3 + x2 + 3x + 3
D)x3 + x2 + 9x + 9
E)x3 - x2 + 9x - 9
A)x3 + 9x2 + x + 9
B)x3 + x2 - 9x - 9
C)x3 + x2 + 3x + 3
D)x3 + x2 + 9x + 9
E)x3 - x2 + 9x - 9

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
46
Write f(x)= x4 - 2x3 - x2 - 38 x + 130 as a product of linear factors.
A)(x - 3 - i)(x - 3 + i)(x - 2 - 3i)(x - 2 + i)
B)(x - 3 + i)(x - 3 - i)(x - 3 + 2i)(x - 3 - 2i)
C)(x - 3 - i)(x - 2 + 3i)(x - 2 - 3i)(x - 2 + i)
D)(x - 3 - i)(x - 3 + i)(x - 2 - i)(x - 2 + i)
E)(x - 3 + i)(x - 3 - i)(x + 2 + 3i)(x + 2 - 3i)
A)(x - 3 - i)(x - 3 + i)(x - 2 - 3i)(x - 2 + i)
B)(x - 3 + i)(x - 3 - i)(x - 3 + 2i)(x - 3 - 2i)
C)(x - 3 - i)(x - 2 + 3i)(x - 2 - 3i)(x - 2 + i)
D)(x - 3 - i)(x - 3 + i)(x - 2 - i)(x - 2 + i)
E)(x - 3 + i)(x - 3 - i)(x + 2 + 3i)(x + 2 - 3i)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
47
Find a polynomial with the given zeros.? ?
A)?
B)
C)
D)
E)none of these
A)?
B)
C)
D)
E)none of these

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
48
Given -3i is a root,determine all other roots of f(x)= x3 + 2x2 + 9x + 18.
A)x = ±2,3i
B)x = -3,±2i
C)x = -2,±3
D)x = -2,3i
E)x = ±2,3
A)x = ±2,3i
B)x = -3,±2i
C)x = -2,±3
D)x = -2,3i
E)x = ±2,3

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
49
Given 3 + i is a root,determine all other roots of f(x)= x5 - 8x4 + 24x3 - 32x2 + 20x.
A)x = 1 - i,3 - i
B)x = 1 - i,3 - i,0
C)x = 1 + i,3 - i
D)x = 1 ± i,3 - i,0
E)x = 1 + i,3 - i,0
A)x = 1 - i,3 - i
B)x = 1 - i,3 - i,0
C)x = 1 + i,3 - i
D)x = 1 ± i,3 - i,0
E)x = 1 + i,3 - i,0

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck



