Deck 25: Right Triangle Trigonometry
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/56
Play
Full screen (f)
Deck 25: Right Triangle Trigonometry
1
Find the exact values of the six trigonometric functions of the angle ? shown in the figure. (Use the Pythagorean Theorem to find the third side of the triangle. ) 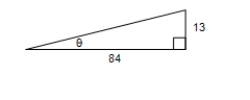
A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?

A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
2
Evaluate each function.Round your answers to four decimal places.? and ?
A)1.1998 and 0.4463
B)1.2998 and 0.5463
C)1.1498 and 0.3963
D)1.0998 and 0.3463
E)1.2498 and 0.4963
A)1.1998 and 0.4463
B)1.2998 and 0.5463
C)1.1498 and 0.3963
D)1.0998 and 0.3463
E)1.2498 and 0.4963
1.0998 and 0.3463
3
Find the exact values of the six trigonometric functions of the angle ? for each of the two triangles. 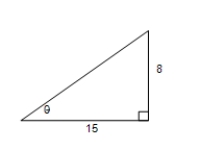
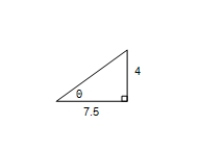 ?
?
A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?

 ?
?A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
4
Select a right triangle corresponding to the trigonometric function of the acute angle ?.Use the Pythagorean Theorem to determine the third side and then find the other five trigonometric functions of ?. ?
Tan ? = ?
?
Sin ? = csc ? = cos ? = sec ? = tan ? = cot ? = ?
A)?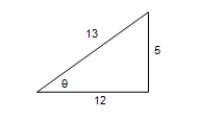 sin ? =
sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)?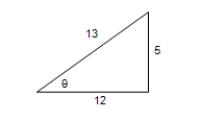 sin ? =
sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)?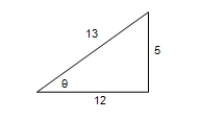 sin ? =
sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)? sin ? =
sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)?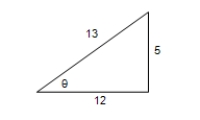 sin ? =
sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
Tan ? = ?
?
Sin ? = csc ? = cos ? = sec ? = tan ? = cot ? = ?
A)?
 sin ? =
sin ? = Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)?
 sin ? =
sin ? = Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)?
 sin ? =
sin ? = Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)?
 sin ? =
sin ? = Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)?
 sin ? =
sin ? = Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
5
Evaluate each function.Round your answers to four decimal places. ?
Csc3 and ?
A)7.0862 and 0.2027
B)7.1862 and 0.3027
C)7.2362 and 0.3527
D)7.1362 and 0.2527
E)7.2862 and 0.4027
Csc3 and ?
A)7.0862 and 0.2027
B)7.1862 and 0.3027
C)7.2362 and 0.3527
D)7.1362 and 0.2527
E)7.2862 and 0.4027

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
6
Construct an appropriate triangle to complete the table. Function
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
7
Evaluate each function.Round your answers to four decimal places.? and ?
A)4.7046 and 0.2126
B)4.7546 and 0.2626
C)4.8546 and 0.3626
D)4.8046 and 0.3126
E)4.9046 and 0.4126
A)4.7046 and 0.2126
B)4.7546 and 0.2626
C)4.8546 and 0.3626
D)4.8046 and 0.3126
E)4.9046 and 0.4126

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
8
Evaluate each function.Round your answers to four decimal places.
Cos17°36' and sin67°23'
A)1.0032 and 0.9731
B)1.0532 and 1.0231
C)1.1532 and 1.1231
D)1.1032 and 1.0731
E)0.9532 and 0.9231
Cos17°36' and sin67°23'
A)1.0032 and 0.9731
B)1.0532 and 1.0231
C)1.1532 and 1.1231
D)1.1032 and 1.0731
E)0.9532 and 0.9231

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
9
Construct an appropriate triangle to complete the table.
A)?
B)?
C)? ?
D)?
E)?
A)?
B)?
C)? ?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
10
Evaluate each function.Round your answers to four decimal places.
Sec0.74 and cos0.74
A)1.4042 and 0.7885
B)1.5542 and 0.9385
C)1.3542 and 0.7385
D)1.4542 and 0.8385
E)1.5042 and 0.8885
Sec0.74 and cos0.74
A)1.4042 and 0.7885
B)1.5542 and 0.9385
C)1.3542 and 0.7385
D)1.4542 and 0.8385
E)1.5042 and 0.8885

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
11
Find the exact values of the six trigonometric functions of the angle ? for the triangle. 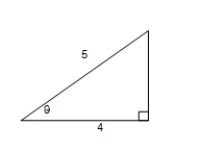 ?
?
A)? sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
 ?
?A)? sin ? =
Csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
12
Construct an appropriate triangle to complete the table.
?
A)?
B)?
C)?
D)?
E)?
?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
13
Evaluate each function.Round your answers to four decimal places.
Sec48°38' and csc31°57'
A)1.7131 and 2.0897
B)1.6131 and 1.9897
C)1.5631 and 1.9397
D)1.5131 and 1.8897
E)1.6631 and 2.0397
Sec48°38' and csc31°57'
A)1.7131 and 2.0897
B)1.6131 and 1.9897
C)1.5631 and 1.9397
D)1.5131 and 1.8897
E)1.6631 and 2.0397

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
14
Construct an appropriate triangle to complete the table.
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
15
Find the exact values of the six trigonometric functions of the angle ? shown in the figure. (Use the Pythagorean Theorem to find the third side of the triangle. ) 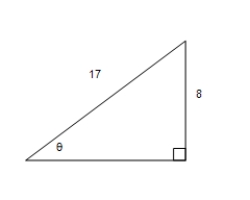 ?
?
A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
 ?
?A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
C)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
16
Construct an appropriate triangle to complete the table.
A)?
B)?
C)?
D)?
?E)?
A)?
B)?
C)?
D)?
?E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
17
Construct an appropriate triangle to complete the table.
?
A)?
B)?
C)?
D)?
E)?
?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
18
Find the exact values of the six trigonometric functions of the angle ? shown in the figure. (Use the Pythagorean Theorem to find the third side of the triangle. ) 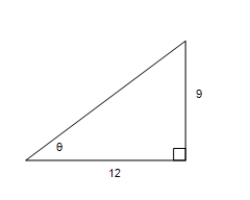 ?
?
A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = ? csc ? = ?
Cos ? = ?
Sec ? = ?
Tan ? = ?
Cot ? = ?
?
C)sin ? = ? csc ? = ?
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
 ?
?A)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
B)sin ? = ? csc ? = ?
Cos ? = ?
Sec ? = ?
Tan ? = ?
Cot ? = ?
?
C)sin ? = ? csc ? = ?
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
D)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?
E)sin ? = csc ? =
Cos ? =
Sec ? =
Tan ? =
Cot ? =
?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
19
Evaluate each function.Round your answers to four decimal places.
Sin22.3° and csc22.3°
A)0.5795 and 2.8354
B)0.3795 and 2.6354
C)0.4795 and 2.7354
D)0.4295 and 2.6854
E)0.5295 and 2.7854
Sin22.3° and csc22.3°
A)0.5795 and 2.8354
B)0.3795 and 2.6354
C)0.4795 and 2.7354
D)0.4295 and 2.6854
E)0.5295 and 2.7854

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
20
Evaluate each function.Round your answers to four decimal places.
Tan15.7° and cot74.3°
A)0.4811 and 0.4811
B)0.3311 and 0.3311
C)0.2811 and 0.2811
D)0.3811 and 0.3811
E)0.4311 and 0.4311
Tan15.7° and cot74.3°
A)0.4811 and 0.4811
B)0.3311 and 0.3311
C)0.2811 and 0.2811
D)0.3811 and 0.3811
E)0.4311 and 0.4311

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
21
Use the given function value(s),and trigonometric identities (including the cofunction identities),to find the indicated trigonometric functions. ?sin 30° = ,cos 30° = ?
?sin 60° and cos 60°
A)?sin 60° = 2 and cos 60° =
B)?sin 60° = and cos 60° =
C)?sin 60° = and cos 60° =
D)?sin 60° = and cos 60° =
E)?sin 60° = and cos 60° = 2
?sin 60° and cos 60°
A)?sin 60° = 2 and cos 60° =
B)?sin 60° = and cos 60° =
C)?sin 60° = and cos 60° =
D)?sin 60° = and cos 60° =
E)?sin 60° = and cos 60° = 2

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
22
Use a graphing utility to complete the table.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
23
Use a compass to sketch a quarter of a circle of radius 10 centimeters.Using a protractor, construct an angle of in standard position (see figure).Drop a perpendicular line from the point of intersection of the terminal side of the angle and the arc of the circle.By actual measurement,calculate the coordinates of the point of intersection and use these measurements to approximate the six trigonometric functions of a angle.(Round your answer to two decimal places. )? 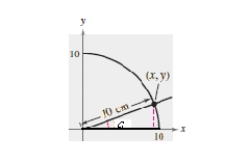 ?
?
A)sin10° ? 0.98,cos10° ? 0.17,tan10° ? 5.76, csc10° ? 0.18,sec10° ? 1.02,cot10° ? 5.67
B)sin10° ? 5.67,cos10° ? 1.02,tan10° ? 5.76, csc10° ? 0.18,sec10° ? 0.98,cot10° ? 5.67
?
C)sin10° ? 0.17,cos10° ? 0.98,tan10° ? 0.18, csc10° ? 5.76,sec10° ? 1.02,cot10° ? 5.67
?
D)sin10° ? 0.18,cos10° ? 0.98,tan10° ? 0.17, csc10° ? 10,sec10° ? 0.98,cot10° ? 5.76
?
E)sin10° ? 0.17,cos10° ? 0.98,tan10° ? 0.18, csc10° ? 5.76,sec10° ? 5.67,cot10° ? 1.02
 ?
?A)sin10° ? 0.98,cos10° ? 0.17,tan10° ? 5.76, csc10° ? 0.18,sec10° ? 1.02,cot10° ? 5.67
B)sin10° ? 5.67,cos10° ? 1.02,tan10° ? 5.76, csc10° ? 0.18,sec10° ? 0.98,cot10° ? 5.67
?
C)sin10° ? 0.17,cos10° ? 0.98,tan10° ? 0.18, csc10° ? 5.76,sec10° ? 1.02,cot10° ? 5.67
?
D)sin10° ? 0.18,cos10° ? 0.98,tan10° ? 0.17, csc10° ? 10,sec10° ? 0.98,cot10° ? 5.76
?
E)sin10° ? 0.17,cos10° ? 0.98,tan10° ? 0.18, csc10° ? 5.76,sec10° ? 5.67,cot10° ? 1.02

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
24
Use the given function value(s),and trigonometric identities (including the cofunction identities),to find the indicated trigonometric functions.? ? ?
A) and
B)? and
C)? and
D)? and
E)? and
A) and
B)? and
C)? and
D)? and
E)? and

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
25
Find the values of ? in degrees and radians without the aid of a calculator. ?sin ? = 1 and csc ? = 1
?
?
A)90° =
B)?30° =
C)?0° = 0
D)?60° =
E)?45° =
?
?
A)90° =
B)?30° =
C)?0° = 0
D)?60° =
E)?45° =

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
26
A biologist wants to know the width w of a river so that instruments for studying the pollutants in the water can be set properly.From point A the biologist walks downstream feet and sights to point C (see figure).From this sighting,it is determined that ? = 54°.How wide is the river?? 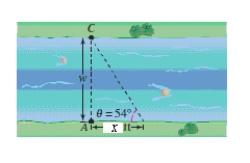 (Round your answer to three decimal places. ) ?
(Round your answer to three decimal places. ) ?
A)212.693
B)197.693
C)207.693
D)202.693
E)192.693
 (Round your answer to three decimal places. ) ?
(Round your answer to three decimal places. ) ?A)212.693
B)197.693
C)207.693
D)202.693
E)192.693

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
27
You are skiing down a mountain with a vertical height of 1250 feet.The distance from the top of the mountain to the base is 2500 feet.What is the angle of elevation from the base to the top of the mountain? ?
A)60° =
B)30° =
C)0° = 0
D)90° =
E)45° =
A)60° =
B)30° =
C)0° = 0
D)90° =
E)45° =

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
28
In traveling across flat land,you notice a mountain directly in front of you.Its angle of elevation (to the peak)is 3.5°.After you drive miles closer to the mountain,the angle of elevation is 9°.Approximate the height of the mountain.(Round your answer upto one decimal place. )? 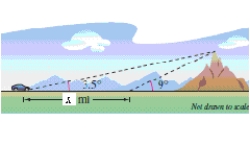 ?
?
A)10.3 mi
B)2.3 mi
C)4.3 mi
D)8.3 mi
E)6.3 mi
 ?
?A)10.3 mi
B)2.3 mi
C)4.3 mi
D)8.3 mi
E)6.3 mi

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
29
Find the values of ? in degrees and radians without the aid of a calculator. cos ? = 0 and tan ? = Not defined
?
?
A)90° =
B)?60° =
C)?0° = 0
D)?30° =
E)?45° =
?
?
A)90° =
B)?60° =
C)?0° = 0
D)?30° =
E)?45° =

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
30
Use the given function value(s),and trigonometric identities (including the cofunction identities),to find the indicated trigonometric functions.
sin 90° = 1,tan 90° = Not defined
Sin 0° and tan 0°
A)sin 0° = 0 and tan 0° = 0
B)sin 0° = 1 and tan 0° = 1
C)sin 0° = Not defined and tan 0° = Not defined
D)sin 0° = Not defined and tan 0° = 1
E)sin 0° = 1 and tan 0° = Not defined
sin 90° = 1,tan 90° = Not defined
Sin 0° and tan 0°
A)sin 0° = 0 and tan 0° = 0
B)sin 0° = 1 and tan 0° = 1
C)sin 0° = Not defined and tan 0° = Not defined
D)sin 0° = Not defined and tan 0° = 1
E)sin 0° = 1 and tan 0° = Not defined

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
31
A 20-meter line is used to tether a helium-filled balloon.Because of a breeze,the line makes an angle of approximately 85° with the ground.Use a trigonometric function to write an equation involving the unknown quantity. ?
A) (where h is height. )
B) (where h is height. )
C) (where h is height. )
D) (where h is height. )
E) (where h is height. )
A) (where h is height. )
B) (where h is height. )
C) (where h is height. )
D) (where h is height. )
E) (where h is height. )

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
32
Find the values of ? in degrees and radians without the aid of a calculator. sec ? = and cot ? = 1
?
?
A)45° =
B)?90° =
C)?0° = 0
D)?60° =
E)?30° =
?
?
A)45° =
B)?90° =
C)?0° = 0
D)?60° =
E)?30° =

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
33
Complete the table.
(Round your answer to four decimal places. )
A)
B)
C)
D)
E)
(Round your answer to four decimal places. )
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
34
A 10-meter line is used to tether a helium-filled balloon.Complete the table,which shows the heights (in meters)of the balloon for decreasing angle measures .(Round your answers to one decimal places. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
35
A tapered shaft has a diameter of centimeters at the small end and is 15 centimeters long (see figure).The taper is 3°.Find the diameter d of the large end of the shaft.(Round your answer to two decimal places. )? 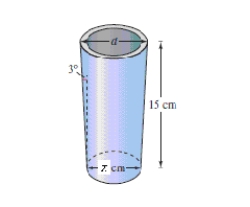 ? ?
? ?
A)10.57 cm
B)14.57 cm
C)12.57 cm
D)18.57 cm
E)16.57 cm
 ? ?
? ?A)10.57 cm
B)14.57 cm
C)12.57 cm
D)18.57 cm
E)16.57 cm

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
36
Use the given function value(s),and trigonometric identities (including the cofunction identities),to find the indicated trigonometric functions.? ? sec ? and csc(90° - ?)
?
A)? and
B) and
C)? and
D)? and
E)? and
?
A)? and
B) and
C)? and
D)? and
E)? and

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
37
A guy wire runs from the ground to a cell tower.The wire is attached to the cell tower feet above the ground.The angle formed between the wire and the ground is 43° (see figure).How long is the guy wire. ?? 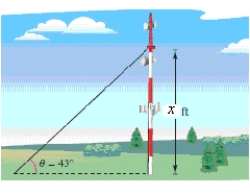 ?
?
A)166.6 ft
B)?146.6 ft
C)?156.6 ft
D)?161.6 ft
E)?151.6 ft
 ?
?A)166.6 ft
B)?146.6 ft
C)?156.6 ft
D)?161.6 ft
E)?151.6 ft

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
38
Use the given function value(s),and trigonometric identities (including the cofunction identities),to find the indicated trigonometric functions.? ? cos ?
?
A)
B)?
C)?
D)?
E)?
?
A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
39
A 50-meter line is used to tether a helium-filled balloon.Because of a breeze,the line makes an angle of approximately 55° with the ground.What is the height of the balloon? (Round the answer to one decimal place. )
A)45 m
B)41 m
C)43 m
D)49 m
E)47 m
A)45 m
B)41 m
C)43 m
D)49 m
E)47 m

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
40
Use the given function value(s),and trigonometric identities (including the cofunction identities),to find the indicated trigonometric functions. ?? ? ?
A)? and
B)? and
C) and
D)? and
E)? and
A)? and
B)? and
C) and
D)? and
E)? and

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
41
If ,find the value of ? in degrees without the aid of a calculator.
A)? = 30°
B)?? = 45°
C)??? = 90°
D)??? = 75°
E)??? = 15°
A)? = 30°
B)?? = 45°
C)??? = 90°
D)??? = 75°
E)??? = 15°

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
42
Use a calculator to evaluate tan 49°34´.Round your answer to four decimal places.
A)-1.3283
B)0.0423
C)-0.8403
D)1.1643
E)1.1736
A)-1.3283
B)0.0423
C)-0.8403
D)1.1643
E)1.1736

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
43
Use a calculator to evaluate tan 68°23'.Round your answer to four decimal places.
A)2.5236
B)2.5040
C)-1.2222
D)-0.8980
E)0.7269
A)2.5236
B)2.5040
C)-1.2222
D)-0.8980
E)0.7269

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
44
Find the exact value of csc ?,using the triangle shown in the figure below,if a = 3 and b = 4. 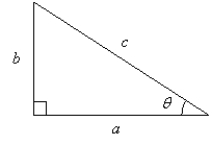
A)
B)?
C)?
D)?
E)?

A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
45
Use a calculator to evaluate .Round your answer to four decimal places.
A)-1.8382
B)-24.0997
C)0.3090
D)3.2361
E)-0.0415
A)-1.8382
B)-24.0997
C)0.3090
D)3.2361
E)-0.0415

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
46
If ,find the value of ? in degrees without the aid of a calculator.
A)?? = 45°
B)?? = 30°
C)?? = 15°
D)?? = 90°
E)?? = 75°
A)?? = 45°
B)?? = 30°
C)?? = 15°
D)?? = 90°
E)?? = 75°

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
47
Will Barrow wanted to know how tall the flagpole was in front of his school.To find its height,he drove a stake into the ground at the tip of the flagpole's shadow and recorded the angle of elevation at two different times during the day.He then measured the distance between the stakes.Will's data is below: Stake
Time
Angle of Elevation
A
2:00 PM
82°
B
3:00 PM
61°
Distance between stakes A & B
10 feet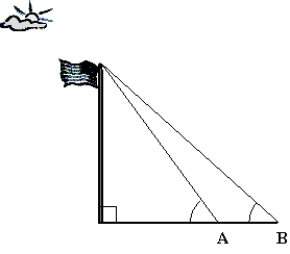 Determine the height of the flagpole.Round your answer to nearest foot.
Determine the height of the flagpole.Round your answer to nearest foot.
A)22 feet
B)24 feet
C)20 feet
D)26 feet
E)18 feet
Time
Angle of Elevation
A
2:00 PM
82°
B
3:00 PM
61°
Distance between stakes A & B
10 feet
 Determine the height of the flagpole.Round your answer to nearest foot.
Determine the height of the flagpole.Round your answer to nearest foot.A)22 feet
B)24 feet
C)20 feet
D)26 feet
E)18 feet

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
48
Given and tan ? = 3,determine the following. ?
Cos(90° - ?)
?
A)?cos(90° - ?)=
B)?cos(90° - ?)=
C)?cos(90° - ?)=
D)?cos(90° - ?)= 3
E)undefined
Cos(90° - ?)
?
A)?cos(90° - ?)=
B)?cos(90° - ?)=
C)?cos(90° - ?)=
D)?cos(90° - ?)= 3
E)undefined

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
49
Find the exact value of csc ?,using the triangle shown in the figure below,if and . 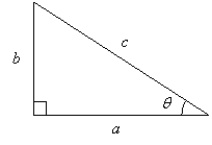
A)?
B)?
C)?
D)?
E)?

A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
50
If ? is an acute angle and ,determine sin ?.
A)
B)?
C)?
D)?
E)?
A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
51
Given and ,determine the following: ?sec 30°
?
A)?sec 30° = 1
B)?sec 30° =
C)?sec 30° =
D)?sec 30° =
E)undefined
?
A)?sec 30° = 1
B)?sec 30° =
C)?sec 30° =
D)?sec 30° =
E)undefined

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
52
Using the figure below,if ? = 26° and y = 6,determine the exact value of x. 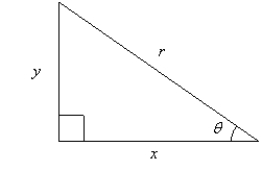
A)?
B)?
C)
D)?
E)?

A)?
B)?
C)
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
53
Given sin 30° = and cos 30° = ,determine the following: ?cot 30°
A)?cot 30° =
B)undefined
C)?cot 30° =
D)?cot 30° =
E)?cot 30° = 2
A)?cot 30° =
B)undefined
C)?cot 30° =
D)?cot 30° =
E)?cot 30° = 2

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
54
Using the figure below,if ? = 33° and y = 9,determine the exact value of x. 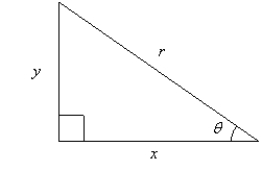
A)
B)?
C)?
D)?
E)?

A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
55
Using the figure below,if ? = 20° and y = 16,determine the exact value of r. 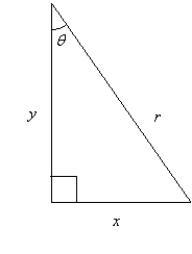
A)
B)?
C)?
D)?
E)?

A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
56
Using trigonometric identities,determine which of the following is equivalent to the following expression. ?
Tan ? + cot ? ?
?
A)?1
B)?csc ? sec ?
C) ? ? + ?
D)?cos ? + sec ?
E)?sec ? + csc ?
Tan ? + cot ?
 ?
?A)?1
B)?csc ? sec ?
C) ? ? + ?
D)?cos ? + sec ?
E)?sec ? + csc ?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck



