Deck 29: Inverse Trigonometric Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/50
Play
Full screen (f)
Deck 29: Inverse Trigonometric Functions
1
Use an inverse trigonometric function to write θ as a function of x. 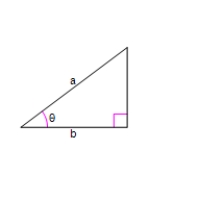 a = x
a = x
B = 10
A)
B)
C)
D)
E)
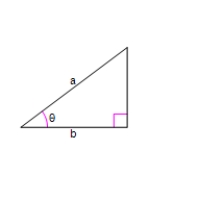 a = x
a = xB = 10
A)
B)
C)
D)
E)
2
Use an inverse trigonometric function to write θ as a function of x. 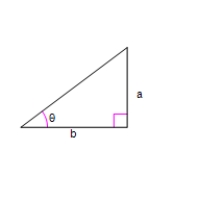 a = x
a = x
B = 6
A)
B)
C)
D)
E)
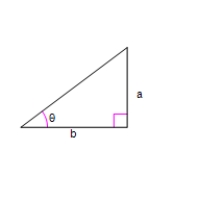 a = x
a = xB = 6
A)
B)
C)
D)
E)
3
Evaluate the expression.Round your result to two decimal places.
A)2.12
B)0.12
C)1.12
D)-0.88
E)3.12
A)2.12
B)0.12
C)1.12
D)-0.88
E)3.12
1.12
4
Use an inverse trigonometric function to write θ as a function of x. 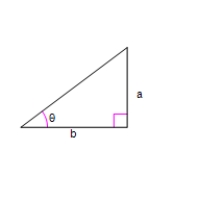 a = x + 5
a = x + 5
B = 10
A)
B)
C)
D)
E)
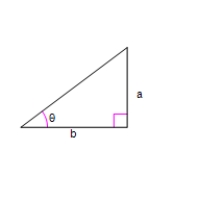 a = x + 5
a = x + 5B = 10
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
5
Use an inverse trigonometric function to write θ as a function of x. 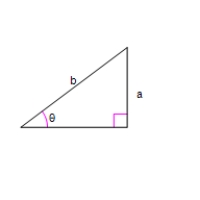 a = x + 6
a = x + 6
B = 2
A)
B)
C)
D)
E)
 a = x + 6
a = x + 6B = 2
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
6
Evaluate the expression.Round your result to two decimal places.
A)1.87
B)-2.13
C)-1.13
D)0.87
E)-0.13
A)1.87
B)-2.13
C)-1.13
D)0.87
E)-0.13

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
7
Evaluate the expression.Round your result to two decimal places.
A)3.54
B)0.54
C)1.54
D)2.54
E)-0.46
A)3.54
B)0.54
C)1.54
D)2.54
E)-0.46

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
8
Evaluate the expression.Round your result to two decimal places.
A)-0.66
B)2.34
C)1.34
D)3.34
E)0.34
A)-0.66
B)2.34
C)1.34
D)3.34
E)0.34

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
9
Use the properties of inverse trigonometric functions to evaluate the expression.
A)
B)
C)
D)
E)40
A)
B)
C)
D)
E)40

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
10
Evaluate the expression.Round your result to two decimal places.
A)0.30
B)1.30
C)4.30
D)3.30
E)2.30
A)0.30
B)1.30
C)4.30
D)3.30
E)2.30

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
11
Evaluate the expression.Round your result to two decimal places.
A)-1.53
B)-0.53
C)2.47
D)0.47
E)1.47
A)-1.53
B)-0.53
C)2.47
D)0.47
E)1.47

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
12
Evaluate the expression.Round your result to two decimal places.
A)-3.53
B)0.47
C)-0.53
D)-1.53
E)-2.53
A)-3.53
B)0.47
C)-0.53
D)-1.53
E)-2.53

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
13
Evaluate the expression.Round your result to two decimal places.
A)4.21
B)2.21
C)3.21
D)0.21
E)1.21
A)4.21
B)2.21
C)3.21
D)0.21
E)1.21

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
14
Evaluate the expression.Round your result to two decimal places.
A)2.71
B)-0.29
C)1.71
D)0.71
E)-1.29
A)2.71
B)-0.29
C)1.71
D)0.71
E)-1.29

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
15
Evaluate the expression.Round your result to two decimal places.
A)-0.53
B)-1.53
C)0.47
D)-3.53
E)-2.53
A)-0.53
B)-1.53
C)0.47
D)-3.53
E)-2.53

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
16
Use the properties of inverse trigonometric functions to evaluate the expression.
A)
B)
C)0.4
D)
E)
A)
B)
C)0.4
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
17
Use an inverse trigonometric function to write θ as a function of x. 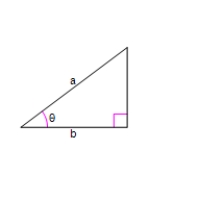 a = 5x
a = 5x
B = x + 9
A)
B)
C)
D)
E)
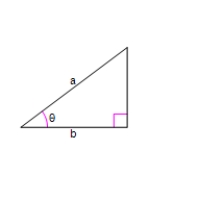 a = 5x
a = 5xB = x + 9
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
18
Use an inverse trigonometric function to write θ as a function of x. 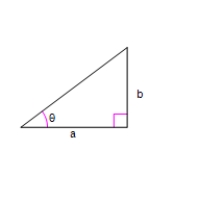
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
19
Evaluate the expression.Round your result to two decimal places.
A)1.73
B)-0.27
C)-1.27
D)2.73
E)0.73
A)1.73
B)-0.27
C)-1.27
D)2.73
E)0.73

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
20
Evaluate the expression.Round your result to two decimal places.
A)2.20
B)0.20
C)3.20
D)-0.80
E)1.20
A)2.20
B)0.20
C)3.20
D)-0.80
E)1.20

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
21
An airplane flies at an altitude of miles toward a point directly over an observer.Consider θ and x as shown in the figure.Write θ as a function of x. 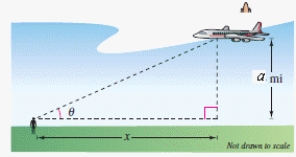
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
22
Evaluate without using a calculator.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
23
Find an algebraic expression that is equivalent to the expression..
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
24
Find an algebraic expression that is equivalent to the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
25
Find the value of the expression.Round your result to two decimal places.
A)0.28
B)1.28
C)1.29
D)-1.29
E)-0.28
A)0.28
B)1.28
C)1.29
D)-1.29
E)-0.28

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
26
A television camera at ground level is filming the lift-off of a space shuttle at a point meters from the launch pad (see figure).Let θ be the angle of elevation to the shuttle and let s be the height of the shuttle.Write θ as a function of s. 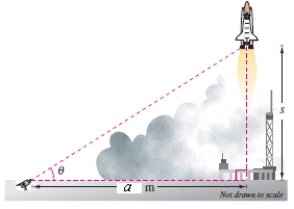
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
27
A boat is pulled in by means of a winch located on a dock feet above the deck of the boat (see figure).Let θ be the angle of elevation from the boat to the winch and let s be the length of the rope from the winch to the boat.Write θ as a function of s. 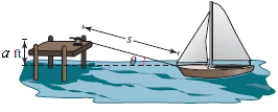
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
28
Find the value of the expression.Round your result to two decimal places.
A)1.61
B)1.53
C)0.04
D)0.96
E)-0.04
A)1.61
B)1.53
C)0.04
D)0.96
E)-0.04

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
29
Use the properties of inverse trigonometric functions to evaluate .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
30
A security car with its spotlight on is parked meters from a warehouse.Consider θ and x as shown in the figure.Write θ as a function of x. 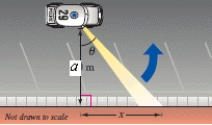
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
31
Use a calculator to evaluate .Round your answer to two decimal places.
A)0.68
B)
C)0.72
D)
E)2.51
A)0.68
B)
C)0.72
D)
E)2.51

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
32
Use the properties of inverse trigonometric functions to evaluate .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
33
Use an inverse function to write θ as a function of x. 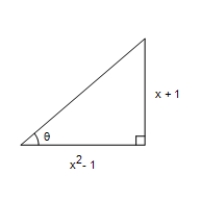
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
34
Find the value of the expression.Round your result to two decimal places.
A)-1.32
B)1.83
C)-0.26
D)-1.26
E)0.74
A)-1.32
B)1.83
C)-0.26
D)-1.26
E)0.74

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
35
Use the properties of inverse trigonometric functions to evaluate the expression.
A)1
B)-1
C)
D)0
E)
A)1
B)-1
C)
D)0
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
36
Use a calculator to evaluate .Round your answer to two decimal places.
A)1.25
B)0.58
C)0.32
D)1.40
E)0.76
A)1.25
B)0.58
C)0.32
D)1.40
E)0.76

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
37
Find an algebraic expression that is equivalent to the expression..
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
38
Evaluate without using a calculator.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
39
Find the value of the expression.Round your result to two decimal places.
A)-1.25
B)1.25
C)1.89
D)3.14
E)-3.14
A)-1.25
B)1.25
C)1.89
D)3.14
E)-3.14

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
40
Use an inverse function to write θ as a function of x. 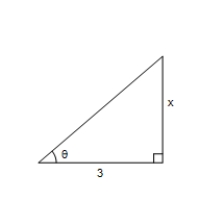
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
41
Use a graphing utility to graph the function below. π -π
Π -π
A)π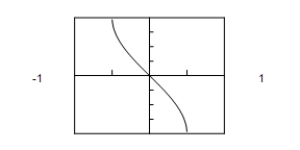 -π
-π
B)π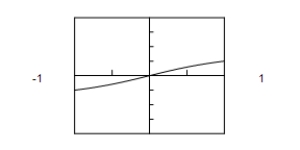 -π
-π
C) π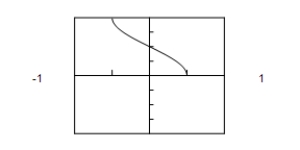 -π
-π
D)π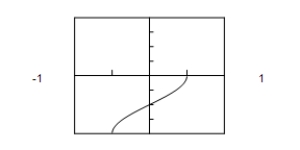 -π
-π
E)π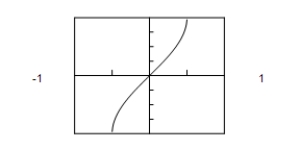 -π
-π
Π -π
A)π
 -π
-πB)π
 -π
-πC) π
 -π
-πD)π
 -π
-πE)π
 -π
-π
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
42
Find the exact value of .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
43
Use a graphing utility to graph the function below.
A)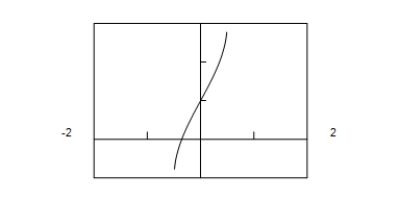
B)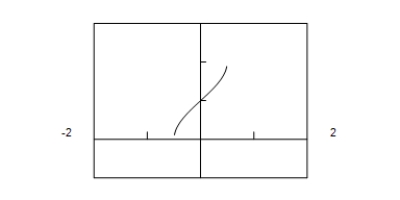
C)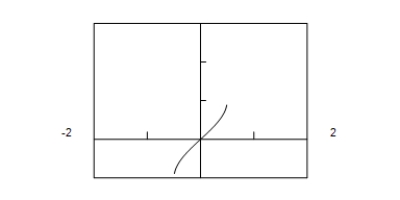
D)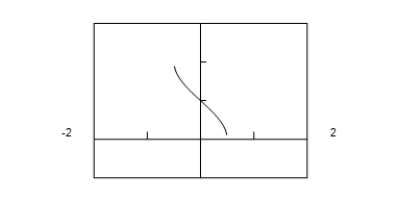
E)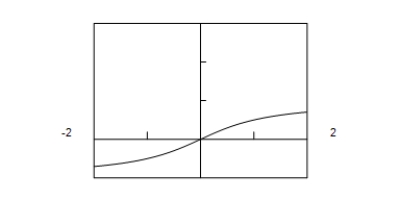
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
44
Write an algebraic expression that is equivalent to .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
45
Write an algebraic expression that is equivalent to .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
46
A granular substance such as sand naturally settles into a cone-shaped pile when poured from a small aperture.Its height depends on the humidity and adhesion between granules.The angle of elevation of a pile,θ,is called the angle of repose.If the height of a pile of sand is 14 feet and its diameter is approximately 47 feet,determine the angle of repose.Round your answer to the nearest degree. 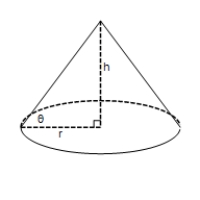
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
47
Find the exact value of .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
48
Which of the following functions is represented by the graph below? 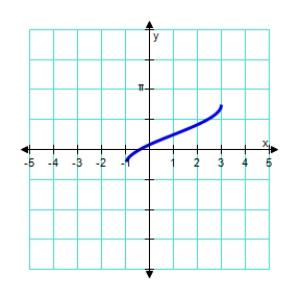
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
49
Which of the following functions is represented by the graph below? 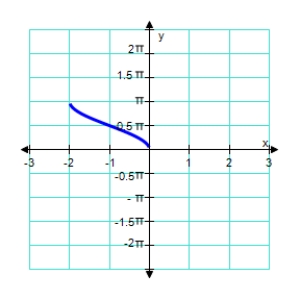
A)2arccos x
B)arcsin 2x
C)arccos (x + 1)
D)2arcsin
E)arccos

A)2arccos x
B)arcsin 2x
C)arccos (x + 1)
D)2arcsin
E)arccos

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
50
Which of the following can be inserted to make the statement true?
A)
B)
C)
D)
E)x
A)
B)
C)
D)
E)x

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck



