Deck 31: Using Fundamental Identities
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/60
Play
Full screen (f)
Deck 31: Using Fundamental Identities
1
Use the given values to evaluate (if possible)three trigonometric functions cos x,sin x,tan x.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)16 cos θ
B)16 sin θ
C)4 sin θ
D)- 4 sin θ
E)4 cos θ
A)16 cos θ
B)16 sin θ
C)4 sin θ
D)- 4 sin θ
E)4 cos θ
4 sin θ
3
Perform the multiplication and use the fundamental identities to simplify.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Use the given values to evaluate (if possible)three trigonometric functions cos x,csc x,tan x. ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
5
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)2 cos θ
B)36 sin θ
C)- 2 sin θ
D)36 cos θ
E)6 sin θ
A)2 cos θ
B)36 sin θ
C)- 2 sin θ
D)36 cos θ
E)6 sin θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
6
Use the given values to evaluate (if possible)three trigonometric functions csc x,tan x,cot x.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
7
Use the given values to evaluate (if possible)three trigonometric functions .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
8
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)4 sin θ
B)- 4 cos θ
C)4 cos θ
D)16 sin θ
E)16 cos θ
A)4 sin θ
B)- 4 cos θ
C)4 cos θ
D)16 sin θ
E)16 cos θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
9
Use the given values to evaluate (if possible)three trigonometric functions cos x,csc x,tan x.
A) tan x = -
B) tan x = -
C) tan x =
D) tan x =
E) tan x = -
A) tan x = -
B) tan x = -
C) tan x =
D) tan x =
E) tan x = -

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
10
Use the given values to evaluate (if possible)three trigonometric functions .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
11
By using a graphing utility to complete the following table.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
12
Use the given values to evaluate (if possible)three trigonometric functions sin x,cos x,cot x.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
13
By using a graphing utility to complete the following table.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
14
Use the given values to evaluate (if possible)three trigonometric functions .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
15
Use the given values to evaluate (if possible)two trigonometric functions and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
16
By using a graphing utility to complete the following table.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
17
Use the fundamental identities to simplify the expression.
A)cot θ
B)cot θ
C)sec θ
D)cos θ
E)csc θ
A)cot θ
B)cot θ
C)sec θ
D)cos θ
E)csc θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
18
By using a graphing utility to complete the following table.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
19
Use the fundamental identities to simplify the expression.
Cot θ sec θ
A)tan θ
B)sec θ cot θ
C)csc θ
D)cot θ
E)cos θ
Cot θ sec θ
A)tan θ
B)sec θ cot θ
C)csc θ
D)cot θ
E)cos θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
20
Use the given values to evaluate (if possible)three trigonometric functions cos x,sin x,cot x.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
21
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .Then find sin θ and cos θ.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
22
Use fundamental identities to simplify the expression below and then determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
23
Find the rate of change of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
24
Find the rate of change of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
25
Use the trigonometric substitution u = a csc θ,where 0 < θ < π/2 and a > 0 to simplify the expression .
A)a tan θ
B)- a cot θ
C)a csc θ
D)a cos θ
E)a cot θ
A)a tan θ
B)- a cot θ
C)a csc θ
D)a cos θ
E)a cot θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
26
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)25 tan θ
B)5 tan θ
C)25 sec θ
D)6 sec θ
E)- 5 sec θ
A)25 tan θ
B)5 tan θ
C)25 sec θ
D)6 sec θ
E)- 5 sec θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
27
Rewrite the expression as a single logarithm and simplify the result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
28
Use a calculator to demonstrate the identity for the value of θ.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
29
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .Then find sin θ and cos θ.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
30
Find the rate of change of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
31
Use the trigonometric substitution u = a sin θ,where - π/2 < θ < π/2 and a > 0 to simplify the expression .
A)a csc θ
B)a sin θ
C)a tan θ
D)a cos θ
E)- a cos θ
A)a csc θ
B)a sin θ
C)a tan θ
D)a cos θ
E)- a cos θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
32
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
33
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)- 5 sec θ
B)5 tan θ
C)25 sec θ
D)25 tan θ
E)5 sec θ
A)- 5 sec θ
B)5 tan θ
C)25 sec θ
D)25 tan θ
E)5 sec θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
34
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)6 sin θ
B)
C)
D)
E)6 cos θ
A)6 sin θ
B)
C)
D)
E)6 cos θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
35
Rewrite the expression as a single logarithm and simplify the result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
36
Use the trigonometric substitution u = a tan θ,where 0 < θ < π/2 and to simplify the expression .
A)- a sec θ
B)a sec θ
C)a tan θ
D)a sin θ
E)a csc θ
A)- a sec θ
B)a sec θ
C)a tan θ
D)a sin θ
E)a csc θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
37
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .Then find sin θ and cos θ.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
38
If ,evaluate the following function. cot x
A)cot x =
B)cot x =
C)cot x = 2
D)cot x =
E)cot x =
A)cot x =
B)cot x =
C)cot x = 2
D)cot x =
E)cot x =

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
39
Use the trigonometric substitution to rewrite the algebraic expression as a trigonometric function of θ,where .
A)4 tan θ
B)16 sec θ
C)- 4 tan θ
D)4 sec θ
E)16 tan θ
A)4 tan θ
B)16 sec θ
C)- 4 tan θ
D)4 sec θ
E)16 tan θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
40
The forces acting on an object weighing W units on an inclined plane positioned at an angle of θ with the horizontal (see figure)are modeled by where μ is the coefficient of friction.Solve the equation for μ and simplify the result. 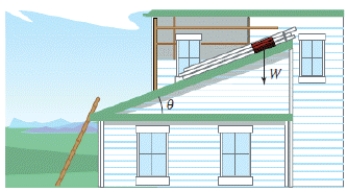
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
41
Factor;then use fundamental identities to simplify the expression below and determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
42
If x = 6 sin θ,use trigonometric substitution to write as a trigonometric function of θ,where .
A)6 csc θ
B)6 sec θ
C)6 sin θ
D)6 cos θ
E)6 tan θ
A)6 csc θ
B)6 sec θ
C)6 sin θ
D)6 cos θ
E)6 tan θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
43
Factor;then use fundamental identities to simplify the expression below and determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
44
Rewrite as a single logarithm and then simplify the result.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following is equivalent to the given expression?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
46
Use fundamental identities to simplify the expression below and then determine which of the following is not equivalent.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
47
Multiply;then use fundamental identities to simplify the expression below and determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
48
If x = 2 tan θ,use trigonometric substitution to write as a trigonometric function of θ,where .
A)2 csc θ
B)2 sec θ
C)2 cos θ
D)2 tan θ
E)2 sin θ
A)2 csc θ
B)2 sec θ
C)2 cos θ
D)2 tan θ
E)2 sin θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
49
If x = 4 cot θ,use trigonometric substitution to write as a trigonometric function of θ,where 0 < θ < π.
A)4 cos θ
B)4 csc θ
C)4 cot θ
D)4 sec θ
E)4 sin θ
A)4 cos θ
B)4 csc θ
C)4 cot θ
D)4 sec θ
E)4 sin θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
50
Use fundamental identities to simplify the expression below and then determine which of the following is not equivalent.
A)-1
B)
C)cot x
D)1/ tan x
E)cos x csc x
A)-1
B)
C)cot x
D)1/ tan x
E)cos x csc x

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
51
Use fundamental identities to simplify the expression below and then determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
52
Multiply;then use fundamental identities to simplify the expression below and determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
53
Factor;then use fundamental identities to simplify the expression below and determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
54
Factor;then use fundamental identities to simplify the expression below and determine which of the following is not equivalent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
55
If x = 5 sin θ,use trigonometric substitution to write as a trigonometric function of θ,where .
A)5 sin θ
B)5 cos θ
C)5 tan θ
D)5 csc θ
E)5 sec θ
A)5 sin θ
B)5 cos θ
C)5 tan θ
D)5 csc θ
E)5 sec θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
56
Which of the following is equivalent to the given expression?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
57
Use a graphing utility to determine which of the trigonometric functions is equal to the following expression.
A) y = cos x
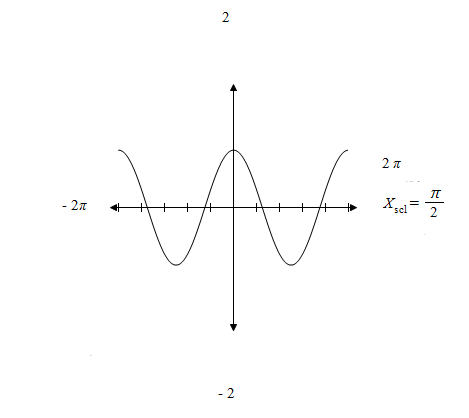
B) y = sin x
2
- 2π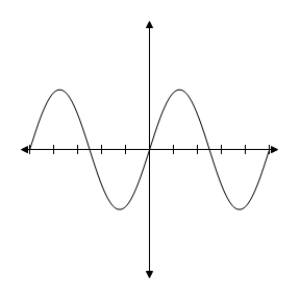
2π
- 2
C) y = csc x
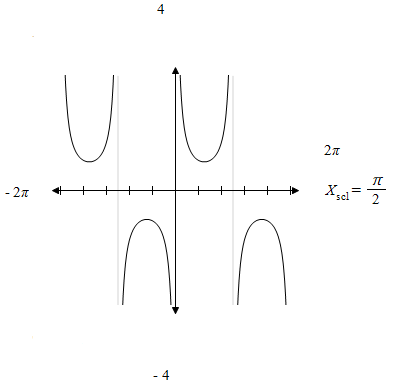
D) y = cot x
4
- 2π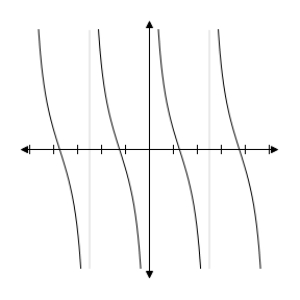
2π
- 4
E) y = tan x
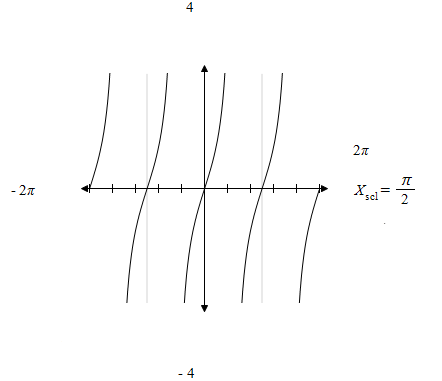
A) y = cos x

B) y = sin x
2
- 2π

2π
- 2
C) y = csc x

D) y = cot x
4
- 2π

2π
- 4
E) y = tan x


Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
58
If x = 3 cot θ,use trigonometric substitution to write as a trigonometric function of θ,where .
A)3 sec θ
B)3 csc θ
C)3 cos θ
D)3 sin θ
E)3 cot θ
A)3 sec θ
B)3 csc θ
C)3 cos θ
D)3 sin θ
E)3 cot θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
59
Rewrite as a single logarithm and then simplify the result.
A)ln |sin θ|
B)ln |tan θ|
C)ln |cos θ|
D)ln |sec θ|
E)ln |csc θ|
A)ln |sin θ|
B)ln |tan θ|
C)ln |cos θ|
D)ln |sec θ|
E)ln |csc θ|

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck
60
If x = 2 tan θ,use trigonometric substitution to write as a trigonometric function of θ,where .
A)2 cos θ
B)2 tan θ
C)2 sin θ
D)2 sec θ
E)2 csc θ
A)2 cos θ
B)2 tan θ
C)2 sin θ
D)2 sec θ
E)2 csc θ

Unlock Deck
Unlock for access to all 60 flashcards in this deck.
Unlock Deck
k this deck



