Deck 33: Solving Trigonometric Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/54
Play
Full screen (f)
Deck 33: Solving Trigonometric Equations
1
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Find all solutions of the following equation in the interval [0,2π).
A)
B)No solution
C)
D)
E)
A)
B)No solution
C)
D)
E)
No solution
3
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
5
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
6
Find all solutions of the following equation in the interval [0,2π).
A)
B)0
C)
D)
E)
A)
B)0
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
7
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
8
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
9
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
10
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
11
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
12
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
13
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
14
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
15
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
16
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
17
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
18
Solve the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
19
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
20
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
21
Solve the following equation.
Secx - 2 = 0
A) and ,where n is a integer
B) and ,where n is a integer
C) and ,where n is a integer
D) and ,where n is a integer
E) and ,where n is a integer
Secx - 2 = 0
A) and ,where n is a integer
B) and ,where n is a integer
C) and ,where n is a integer
D) and ,where n is a integer
E) and ,where n is a integer

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
22
Use a graphing utility to graph the function.
A)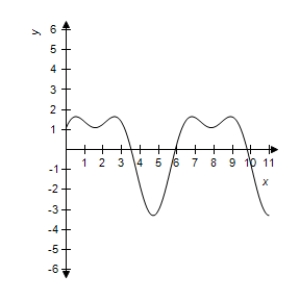
B)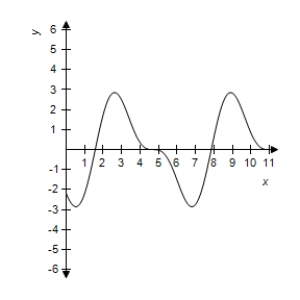
C)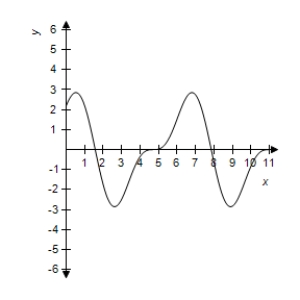
D)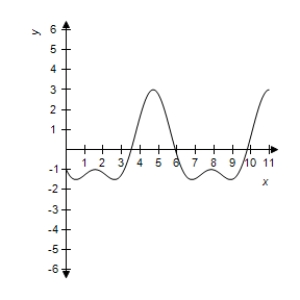
E)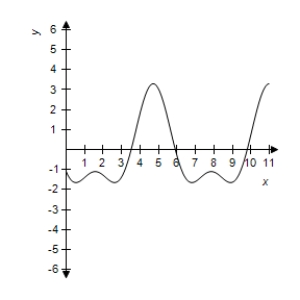
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
23
Solve the multiple-angle equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
24
Use inverse functions where needed to find all solutions of the equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the multiple-angle equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
26
Use a graphing utility to graph the function.
A)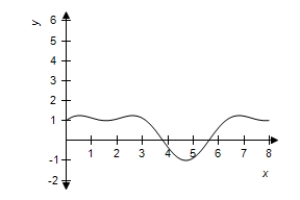
B)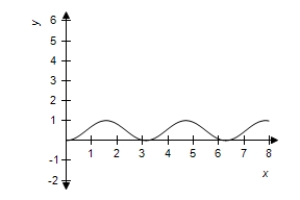
C)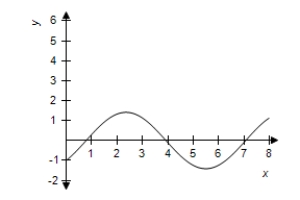
D)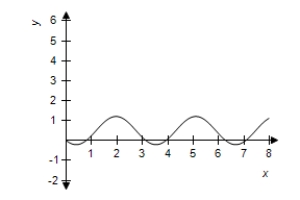
E)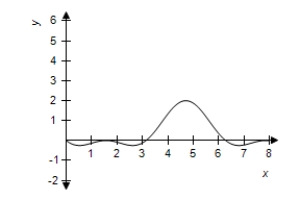
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
27
Solve the multiple-angle equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
28
Use inverse functions where needed to find all solutions of the equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
29
A weight is oscillating on the end of a spring (see figure).The position of the weight relative to the point of equilibrium is given by ,where y is the displacement (in meters)and t is the time (in seconds).Find the times when the weight is at the point of equilibrium (y = 0)for . 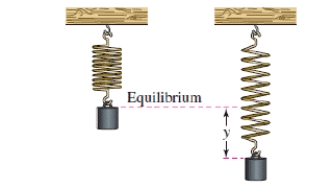
A)0.05sec,0.49sec,0.94sec
B)0.05sec,0.05sec,0.94sec
C)-0.40sec,0.49sec,0.94sec
D)-0.40sec,0.49sec,-0.40sec
E)0.05sec,-0.40sec,0.94sec

A)0.05sec,0.49sec,0.94sec
B)0.05sec,0.05sec,0.94sec
C)-0.40sec,0.49sec,0.94sec
D)-0.40sec,0.49sec,-0.40sec
E)0.05sec,-0.40sec,0.94sec

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
30
The table shows the average daily high temperatures in Houston H (in degrees Fahrenheit)for month t,with t = 1 corresponding to January.
Select the correct scatter plot from the above data.
A)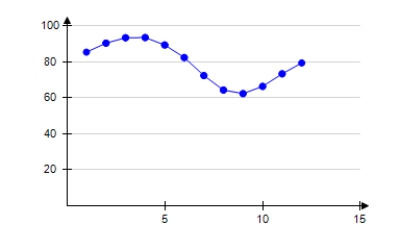
B)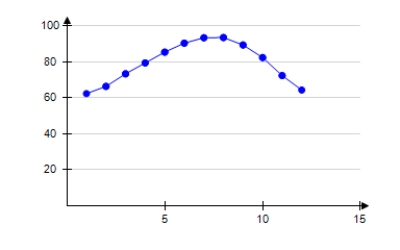
C)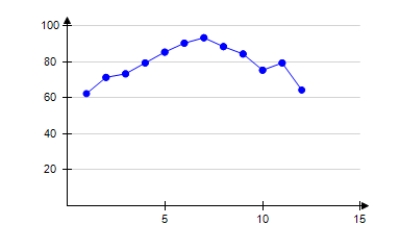
D)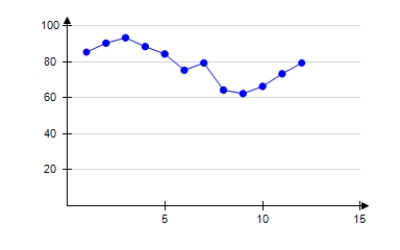
E)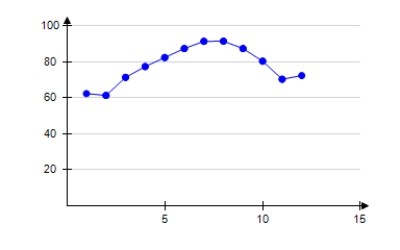
Select the correct scatter plot from the above data.
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
31
Solve the following equation.
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
E) ,where n is an integer
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
E) ,where n is an integer

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
32
Solve the following equation.
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
E) ,where n is an integer
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
E) ,where n is an integer

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
33
Use a graphing utility to graph the function.
A)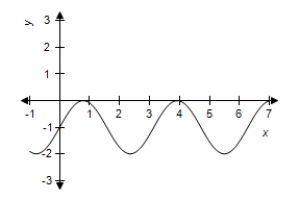
B)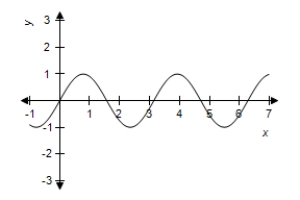
C)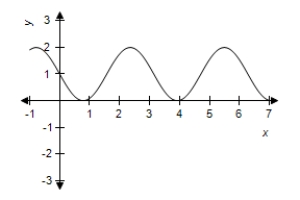
D)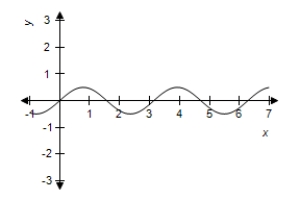
E)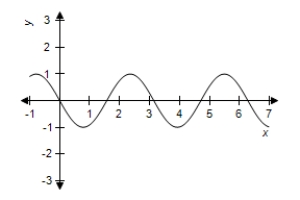
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
34
Use a graphing utility to graph the function.
A)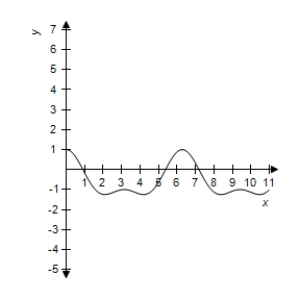
B)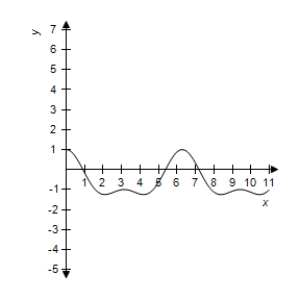
C)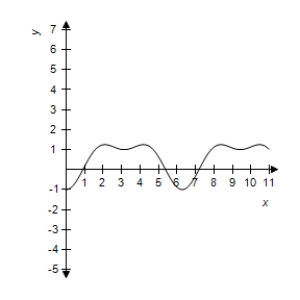
D)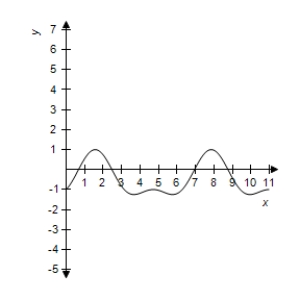
E)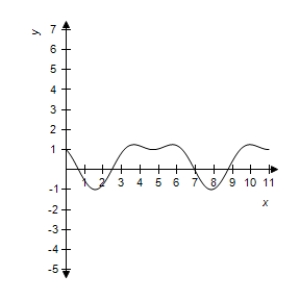
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
35
Solve the multiple-angle equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
36
Use inverse functions where needed to find all solutions of the equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
37
Which of the following is a solution to the given equation?
Cscx + 2 = 0
A)
B)
C)
D)
E)
Cscx + 2 = 0
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
38
Which of the following is a solution to the given equation?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
39
Use a graphing utility to graph the function.
A)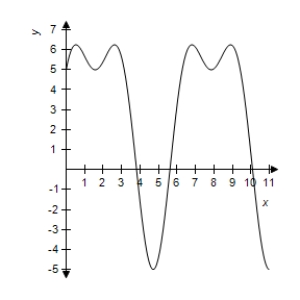
B)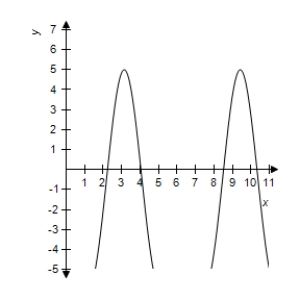
C)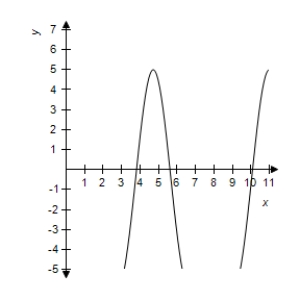
D)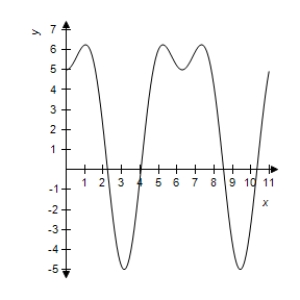
E)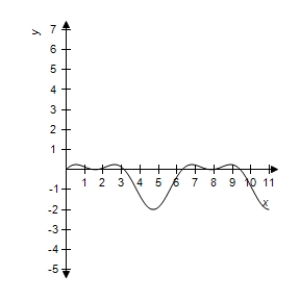
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
40
Determine whether the statement is true or false.Justify your answer.
The equation has four times the number of solutions in the interval [0,2π)as the equation .
A)False
B)True
The equation has four times the number of solutions in the interval [0,2π)as the equation .
A)False
B)True

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
41
Solve the following equation.
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
42
Using the grid provided,sketch the graph of the given function in the interval (-5,5)and then determine the x-intercepts,if any. 

A)(-3,0)and (1,0)
B)(-2,0)
C)(-2,0)and (2,0)
D)no x-intercepts
E)(-4,0), (0,0),and (4,0)


A)(-3,0)and (1,0)
B)(-2,0)
C)(-2,0)and (2,0)
D)no x-intercepts
E)(-4,0), (0,0),and (4,0)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
43
Which of the following is a solution to the given equation?
Cscx - 2 = 0
A)
B)
C) .
D)
E)
Cscx - 2 = 0
A)
B)
C) .
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
44
A Ferris wheel is built such that the height h (in feet)above the ground of a seat on the wheel at time t (in seconds)can be modeled by .The wheel makes one revolution every 36 seconds and the ride begins when t = 0.During the first 36 seconds of the ride,when will a person,who starts at the bottom of the Ferris wheel,be 67 feet above the ground?
A)9 seconds and 22 seconds
B)9 seconds and 27 seconds
C)10 seconds and 22 seconds
D)9 seconds and 17 seconds
E)10 seconds and 17 seconds
A)9 seconds and 22 seconds
B)9 seconds and 27 seconds
C)10 seconds and 22 seconds
D)9 seconds and 17 seconds
E)10 seconds and 17 seconds

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
45
Use the Quadratic Formula to solve the given equation on the interval [ );then use a graphing utility to approximate the angle x.Round answers to three decimal places.
A)x = 1.229,1.433
B)x = 1.227,1.422
C)x = 1.233,1.469
D)x = 1.231,1.451
E)x = 1.235,1.480
A)x = 1.229,1.433
B)x = 1.227,1.422
C)x = 1.233,1.469
D)x = 1.231,1.451
E)x = 1.235,1.480

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
46
Solve the multiple-angle equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
47
Use a graphing utility to approximate the solutions (to three decimal places)of the given equation in the interval [0,2π).
A)x = 1.624,1.932,5.776,5.997
B)x = 1.101,2.118,3.982,5.104
C)x = 1.571,3.990,4.712,5.435
D)x = 1.055,3.785,4.652,5.721
E)x = 1.484,3.799,4.626,5.490
A)x = 1.624,1.932,5.776,5.997
B)x = 1.101,2.118,3.982,5.104
C)x = 1.571,3.990,4.712,5.435
D)x = 1.055,3.785,4.652,5.721
E)x = 1.484,3.799,4.626,5.490

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
48
The monthly sales S (in hundreds of units)of baseball equipment for an Internet sporting goods site are approximated by where t is the time (in months),with t = 1 corresponding to January.Determine the months when sales exceed 7700 units at any time during the month.
A)April through August
B)July through May
C)June through August
D)April through July
E)May through July
A)April through August
B)July through May
C)June through August
D)April through July
E)May through July

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
49
Use a graphing utility to approximate the solutions (to three decimal places)of the given equation in the interval .
A)x = 0.398
B)x = 1.336
C)x = 0.094
D)x = 0.73
E)x = 0.139
A)x = 0.398
B)x = 1.336
C)x = 0.094
D)x = 0.73
E)x = 0.139

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
50
Use inverse functions where needed to find all solutions (if they exist)of the given equation on the interval [0,2π).
A)
B)
C)
D)
E)solution does not exist
A)
B)
C)
D)
E)solution does not exist

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
51
Find all solutions of the following equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the multiple-angle equation.
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
E) ,where n is an integer
A) ,where n is an integer
B) ,where n is an integer
C) ,where n is an integer
D) ,where n is an integer
E) ,where n is an integer

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
53
The horizontal distance d (in feet)traveled by a projectile with an initial speed of v feet per second is modeled by , where θ is the angle at which the projectile is launched.
Find the horizontal distance traveled by a golf ball that is hit with an initial speed of 80 feet per second when the ball is hit at an angle of .Round to the nearest foot.
A)260
B)346
C)173
D)303
E)87
Find the horizontal distance traveled by a golf ball that is hit with an initial speed of 80 feet per second when the ball is hit at an angle of .Round to the nearest foot.
A)260
B)346
C)173
D)303
E)87

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck
54
Which of the following is a solution to the given equation?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 54 flashcards in this deck.
Unlock Deck
k this deck



