Deck 38:Vectors in the Plane
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/50
Play
Full screen (f)
Deck 38:Vectors in the Plane
1
Find a unit vector in the direction of the given vector.? ?
A)j
B)i
C)- j
D)- i
E)?i - j
A)j
B)i
C)- j
D)- i
E)?i - j
j
2
Find the component form of v given its magnitude and the angle it makes with the positive x-axis. ?
Magnitude
Angle 0°
?
?
A)
B)?
C)?
D)?
E)?
Magnitude
Angle 0°
?
?
A)
B)?
C)?
D)?
E)?
?
3
Find 2u - 3v.? ?
A)?
B)
C) ?
D)
E) ?
A)?
B)
C) ?
D)
E) ?
4
A gun with a muzzle velocity of 1700 feet per second is fired at an angle of 4° above the horizontal.Find the vertical and horizontal components of the velocity.(Round your answer to one decimal place. )
A)Vertical ≈ 118.6 ft/sec,Horisontal ≈ 1,695.9 ft/sec.
B)Vertical ≈ 119.6 ft/sec,Horisontal ≈ 1,696.9 ft/sec.
C)Vertical ≈ 1,695.9 ft/sec,Horisontal ≈ 1,695.9 ft/sec.
D)Vertical ≈ 1,695.9 ft/sec,Horisontal ≈ 118.6 ft/sec.
E)Vertical ≈ 118.6 ft/sec,Horisontal ≈ 118.6 ft/sec.
A)Vertical ≈ 118.6 ft/sec,Horisontal ≈ 1,695.9 ft/sec.
B)Vertical ≈ 119.6 ft/sec,Horisontal ≈ 1,696.9 ft/sec.
C)Vertical ≈ 1,695.9 ft/sec,Horisontal ≈ 1,695.9 ft/sec.
D)Vertical ≈ 1,695.9 ft/sec,Horisontal ≈ 118.6 ft/sec.
E)Vertical ≈ 118.6 ft/sec,Horisontal ≈ 118.6 ft/sec.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
5
Find u + v.? ?
A)?
B) ?
C)?
D)?
E)?
A)?
B) ?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
6
Find the component form of v given its magnitude and the angle it makes with the positive x-axis. ?
Magnitude
Angle ?
A)?
B)?
C)?
D)?
E)?
Magnitude
Angle ?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
7
Find a unit vector in the direction of the given vector. ?? ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
8
Find a unit vector in the direction of the given vector.? ?
A)?
B)?
C)?
D)
E)?
A)?
B)?
C)?
D)
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
9
Find a unit vector in the direction of the given vector.? ?
A)?
B)?
C)?
D)?
E)
A)?
B)?
C)?
D)?
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
10
Find 2u - 3v.? ?
A)
B)?
C)?
D)?
E)?
A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
11
Find u - v.? ?
A)?
B)?
C)
D)?
E)?
A)?
B)?
C)
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
12
Detroit Tigers pitcher Joel Zumaya was recorded throwing a pitch at a velocity of 107 miles per hour.If he threw the pitch at an angle of 32° below the horizontal,find the vertical and horizontal components of the velocity.(Round your answers to one decimal place. )
A)Vertical ≈ -55.7 mi/h,Horisontal ≈ 91.7 mi/h.
B)Vertical ≈ 90.7 mi/h,Horisontal ≈ -56.7 mi/h.
C)Vertical ≈ -56.7 mi/h,Horisontal ≈ -56.7 mi/h.
D)Vertical ≈ -56.7 mi/h,Horisontal ≈ 90.7 mi/h.
E)Vertical ≈ 90.7 mi/h,Horisontal ≈ 90.7 mi/h.
A)Vertical ≈ -55.7 mi/h,Horisontal ≈ 91.7 mi/h.
B)Vertical ≈ 90.7 mi/h,Horisontal ≈ -56.7 mi/h.
C)Vertical ≈ -56.7 mi/h,Horisontal ≈ -56.7 mi/h.
D)Vertical ≈ -56.7 mi/h,Horisontal ≈ 90.7 mi/h.
E)Vertical ≈ 90.7 mi/h,Horisontal ≈ 90.7 mi/h.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
13
Select a linear combination of the standard unit vectors i and j of given initial and terminal points of a vector..
Initial Point
Terminal Point
(0,-8)
(9,12)
A)9i + 20j
B)-9i - 20j
C)20i - 9j
D)20i + 9j
E)9i - 20j
Initial Point
Terminal Point
(0,-8)
(9,12)
A)9i + 20j
B)-9i - 20j
C)20i - 9j
D)20i + 9j
E)9i - 20j

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
14
Find u + v.? ?
A)?
B)?
C)?
D)?
E)
A)?
B)?
C)?
D)?
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
15
Find a unit vector in the direction of the given vector.? ?
A)
B)?
C)?
D)?
E)?
A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
16
Select a linear combination of the standard unit vectors i and j of given initial and terminal points of a vector. Initial Point
Terminal Point
(-9,9)
(10,-10)
A)19i - 19j
B)-19i + 19j
C)-19i - 19j
D)19i + 19j
E)-19i - 19j
Terminal Point
(-9,9)
(10,-10)
A)19i - 19j
B)-19i + 19j
C)-19i - 19j
D)19i + 19j
E)-19i - 19j

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
17
Find the component form and the magnitude of the vector v. Initial Point
Terminal Point
(1,3)
(-8,-9)
A)
B)
C)?
D)?
E)?
Terminal Point
(1,3)
(-8,-9)
A)
B)
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
18
Find u - v.? ?
A)?
B)? .
C)? .
D)? .
E)?
A)?
B)? .
C)? .
D)? .
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
19
Find u - v.? ?
A)?-i - 4j
B)-2i - 4j
C)-i + 4j
D)-2i + 4j
E)-i + 5j
A)?-i - 4j
B)-2i - 4j
C)-i + 4j
D)-2i + 4j
E)-i + 5j

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
20
Find u + v.? ?
A)?
B)
C)?
D)?
E)?
A)?
B)
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
21
Let w be a vector with initial point (4,-4)and terminal point (2,5).Write w as a linear combination of the standard unit vectors i and j.
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
22
?Find a unit vector in the direction of . ?
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
23
?Find the magnitude of vector v with initial point (7,5)and terminal point (-3,-5). ?
A)?
B)?14
C)?
D)?
E)?
A)?
B)?14
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
24
A force of F pounds is required to pull an object weighing W pounds up a ramp inclined at θ degrees from the horizontal.
Find F if W = 5,700 pounds and θ = 29°.Approximate the answer to one decimal place.
A)2,761.4 lb
B)2,764.4 lb
C)2,763.4 lb
D)2,765.4 lb
E)2,762.4 lb
Find F if W = 5,700 pounds and θ = 29°.Approximate the answer to one decimal place.
A)2,761.4 lb
B)2,764.4 lb
C)2,763.4 lb
D)2,765.4 lb
E)2,762.4 lb

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
25
A loaded barge is being towed by two tugboats,and the magnitude of the resultant is 6,400 pounds directed along the axis of the barge (see figure).Find the tension in the tow lines if they each make an angle 21° with the axis of the barge.Approximate the answer to one decimal place. 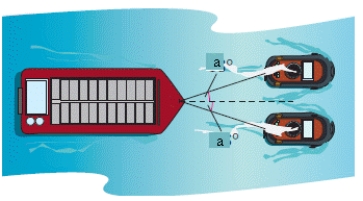 where a = 21°.
where a = 21°.
A)3,425.7 lb
B)3,429.7 lb
C)3,426.7 lb
D)3,428.7 lb
E)3,427.7 lb
 where a = 21°.
where a = 21°.A)3,425.7 lb
B)3,429.7 lb
C)3,426.7 lb
D)3,428.7 lb
E)3,427.7 lb

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
26
A force of F pounds is required to pull an object weighing W pounds up a ramp inclined at θ degrees from the horizontal.
Find F if W = 100 pounds and θ = 11°.Approximate the answer to one decimal place.
A)18.1 lb
B)21.1 lb
C)19.1 lb
D)20.1 lb
E)17.1 lb
Find F if W = 100 pounds and θ = 11°.Approximate the answer to one decimal place.
A)18.1 lb
B)21.1 lb
C)19.1 lb
D)20.1 lb
E)17.1 lb

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
27
A traffic light weighing 15 pounds is suspended by two cables (see figure).Find the tension in each cable if θ1 = 40° and θ2 = 35°.Approximate the answers to one decimal place. 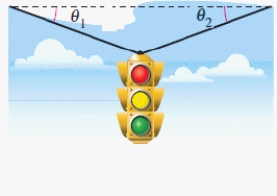
A)TL = 12.7 lb;TR = 12.7 lb
B)TL = 11.9 lb;TR = 11.9 lb
C)TL = 11.9 lb;TR = 12.7 lb
D)TL = 12.7 lb;TR = 11.9 lb
E)TL = 13.7 lb;TR = 12.9 lb

A)TL = 12.7 lb;TR = 12.7 lb
B)TL = 11.9 lb;TR = 11.9 lb
C)TL = 11.9 lb;TR = 12.7 lb
D)TL = 12.7 lb;TR = 11.9 lb
E)TL = 13.7 lb;TR = 12.9 lb

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
28
Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? ![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41d_f143_94ac_17cab916aa01_TB4590_00.jpg) ?
?
A)?![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41d_f144_94ac_51f25f06f180_TB4590_00.jpg)
B)?![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41e_1855_94ac_d91e8b9eab53_TB4590_00.jpg)
C)?![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41e_1856_94ac_5bd2c2b98d41_TB4590_00.jpg)
D)?![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41e_3f67_94ac_9b28badea5f7_TB4590_00.jpg)
E)none of these
![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41d_f143_94ac_17cab916aa01_TB4590_00.jpg) ?
?A)?
![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41d_f144_94ac_51f25f06f180_TB4590_00.jpg)
B)?
![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41e_1855_94ac_d91e8b9eab53_TB4590_00.jpg)
C)?
![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41e_1856_94ac_5bd2c2b98d41_TB4590_00.jpg)
D)?
![<strong>Using the figure below,sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]? -u ?</strong> A)? B)? C)? D)? E)none of these](https://d2lvgg3v3hfg70.cloudfront.net/TB4590/11ea99db_b41e_3f67_94ac_9b28badea5f7_TB4590_00.jpg)
E)none of these

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
29
The initial and terminal points of vector v are (3,-8)and (7,1),respectively.Select v in component form. ?
A)?
B)?
C)
D)
E)?
A)?
B)?
C)
D)
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
30
Find the magnitude of vector v.? 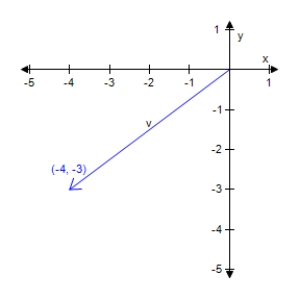
A)
B)?
C)?
D)?
E)?

A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
31
Find the component form of vector v. ?? 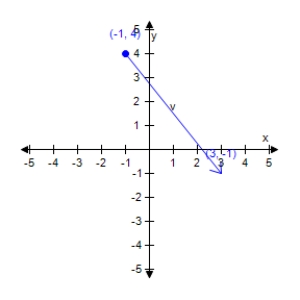 ?
?
A)
B)?
C)?
D)?
E)?
 ?
?A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
32
Given and ,determine .
A)?
B)
C)?
D)?
E)?
A)?
B)
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
33
A traffic light weighing 16 pounds is suspended by two cables (see figure).Find the tension in each cable.Approximate the answer to one decimal place. 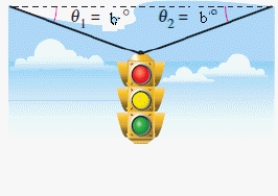
Where b = 28°
A)18.0 lb
B)17.0 lb
C)16.0 lb
D)19.0 lb
E)15.0 lb

Where b = 28°
A)18.0 lb
B)17.0 lb
C)16.0 lb
D)19.0 lb
E)15.0 lb

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
34
?Find the vector v that has a magnitude of 9 and is in the same direction as u,where . ?
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
35
A force of F pounds is required to pull an object weighing W pounds up a ramp inclined at θ degrees from the horizontal.
Find θ if F = 5,100 pounds and W = 14,000 lb.Approximate the answer to one decimal place.
A)19.4°
B)21.4°
C)23.4°
D)20.4°
E)22.4°
Find θ if F = 5,100 pounds and W = 14,000 lb.Approximate the answer to one decimal place.
A)19.4°
B)21.4°
C)23.4°
D)20.4°
E)22.4°

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
36
Find the component form of vector v with initial point (1,-7)and terminal point (-3,1).
A)?
B)
C)?
D)?
E)?
A)?
B)
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
37
?Find a unit vector in the direction of . ?
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
38
A force of F pounds is required to pull an object weighing W pounds up a ramp inclined at θ degrees from the horizontal.
Find W if F = 620 pounds and θ = 20°.Approximate the answer to one decimal place.
A)1,812.8 lb
B)1,810.8 lb
C)1,814.8 lb
D)1,811.8 lb
E)1,813.8 lb
Find W if F = 620 pounds and θ = 20°.Approximate the answer to one decimal place.
A)1,812.8 lb
B)1,810.8 lb
C)1,814.8 lb
D)1,811.8 lb
E)1,813.8 lb

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
39
Given and ,determine .
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
40
Select a linear combination of the standard unit vectors i and j of given initial and terminal points of a vector. Initial Point
Terminal Point
(-9,7)
(0,2)
A)-9i - 5j
B)-5i + 9j
C)-5i - 9j
D)9i - 5j
E)9i + 5j
Terminal Point
(-9,7)
(0,2)
A)-9i - 5j
B)-5i + 9j
C)-5i - 9j
D)9i - 5j
E)9i + 5j

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
41
Given that Force 1 = 55 pounds and Force 2 = 130 pounds,find the angle between the forces if the magnitude of the resultant force is 120 pounds.Round your answer to the nearest degree.
A)72°
B)59°
C)67°
D)79°
E)64°
A)72°
B)59°
C)67°
D)79°
E)64°

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
42
?If and ,find .
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
43
If and ,and the vectors make angles of 140° and 20° with the x-axis respectively,find the component form of the sum of u and v.Round answers to two decimal places.
A)
B)?
C)?
D)?
E)?
A)
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
44
Find the component form of v if and the angle it makes with the x-axis is 30°.
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
45
?Find the magnitude and direction angle of .Round the direction angle to the nearest degree.
A)??||v|| = ;? = 269°
B)??||v|| = ;? = 239°
C)??||v|| = ;? = 260°
D)?||v|| = ;? = 252°
E)??||v|| = ;? = 279°
A)??||v|| = ;? = 269°
B)??||v|| = ;? = 239°
C)??||v|| = ;? = 260°
D)?||v|| = ;? = 252°
E)??||v|| = ;? = 279°

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
46
Find the magnitude and direction angle of .
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
47
Three forces with magnitudes of 74 pounds,98 pounds,and 105 pounds act on an object at angles 170°,220°,and 330°,respectively,with the positive x-axis.Find the magnitude and direction of the resultant force.Round answers to two decimal places.
A)99.08 pounds;240.95°
B)99.08 pounds;60.95°
C)117.42 pounds;240.95°
D)139.13 pounds;272.47°
E)117.42 pounds;60.95°
A)99.08 pounds;240.95°
B)99.08 pounds;60.95°
C)117.42 pounds;240.95°
D)139.13 pounds;272.47°
E)117.42 pounds;60.95°

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
48
Find the component form of v if and the angle it makes with the x-axis is 60°.
A)?
B)?
C)
D)?
E)?
A)?
B)?
C)
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
49
Find the component form of v if and the angle it makes with the x-axis is 120°.
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
50
Find the component form of v if and the angle it makes with the x-axis is 150°.
A)?
B)?
C)?
D)?
E)?
A)?
B)?
C)?
D)?
E)?

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck



