Deck 4: Inequalities and Linear Programming
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/119
Play
Full screen (f)
Deck 4: Inequalities and Linear Programming
1
The Video Star Company makes two different models of DVD players, which are assembled on two different assembly lines. Line 1 can assemble 32 units of the Star model and 44 units of the Prostar model per hour, and Line 2 can assemble 150 units of the Star model and 40 units of the Prostar model per hour. The company needs to produce at least 260 units of the Star model and 225 units of the Prostar model to fill an order. Let x represent the number of assembly hours for Line 1 and y represent the number of assembly hours for Line 2. Write the inequalities that describe the production constraints on the number of each type of DVD player needed to fill the order.
A)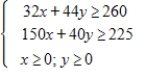
B)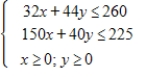
C)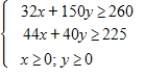
D)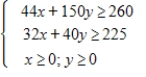
E)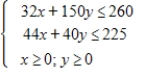
A)
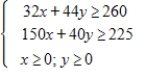
B)
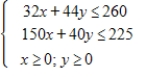
C)
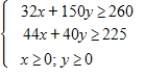
D)
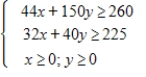
E)
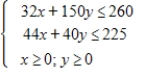
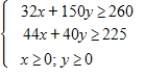
2
The graph of the boundary equations for the system of inequalities is shown with that system. Locate the solution region and find the corners. 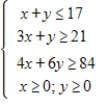
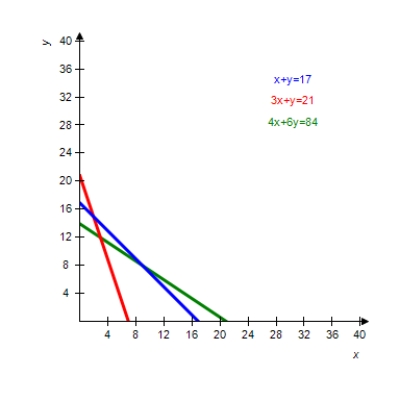
A) (0,14); (0,17); (2,15); (3,12)
B) (0,0); (0,14); (3,12); (7,0)
C) (3,12); (2,15); (9,8)
D) (7,0); (3,12); (9,8); (17,0)
E) (0,17); (9,8); (0,14)
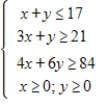

A) (0,14); (0,17); (2,15); (3,12)
B) (0,0); (0,14); (3,12); (7,0)
C) (3,12); (2,15); (9,8)
D) (7,0); (3,12); (9,8); (17,0)
E) (0,17); (9,8); (0,14)
(3,12); (2,15); (9,8)
3
The Wellbuilt Company produces two types of wood chippers, economy and deluxe. The deluxe model requires 4 hours to assemble and 0.5 hour to paint, and the economy model requires 2 hours to assemble and 1 hour to paint. The maximum number of assembly hours available is 22 per day, and the maximum number of painting hours available is 8 per day. Let x represent the number of deluxe models and y represent the number of economy models. Write the system of inequalities that describes the constraints on the number of each type of wood chipper produced.
A)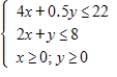
B)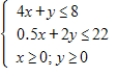
C)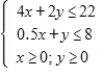
D)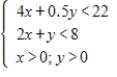
E)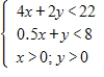
A)
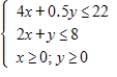
B)
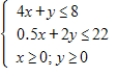
C)
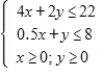
D)
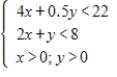
E)
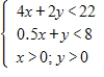
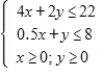
4
Graph the solution of the system of inequalities. 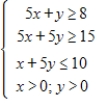
A) 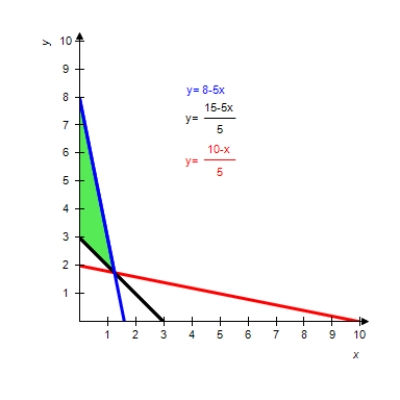
B) 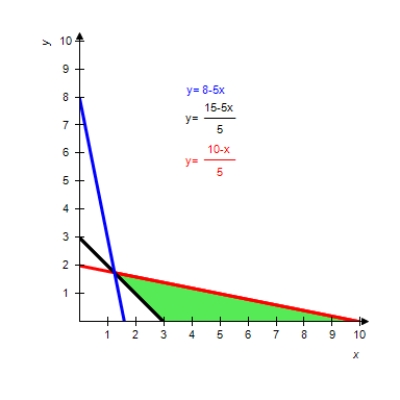
C) 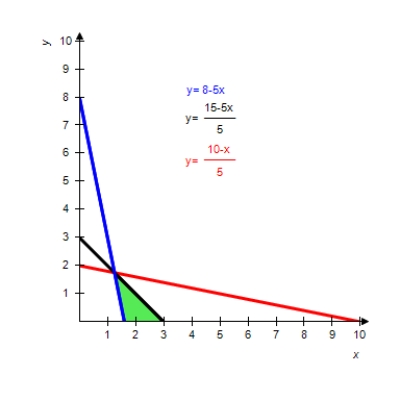
D) 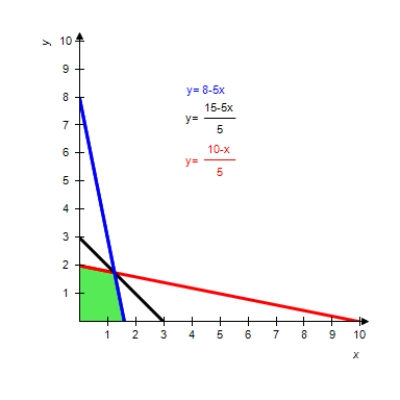
E) 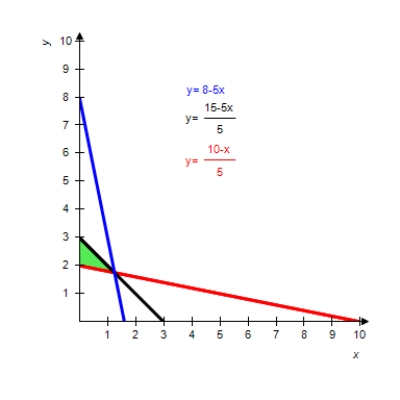
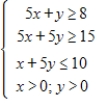
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
5
The Video Star Company makes two different models of DVD players, which are assembled on two different assembly lines. Line 1 can assemble 30 units of the Star model and 42 units of the Prostar model per hour, and Line 2 can assemble 150 units of the Star model and 40 units of the Prostar model per hour. The company needs to produce at least 250 units of the Star model and 210 units of the Prostar model to fill an order. Let x represent the number of assembly hours for Line 1 and y represent the number of assembly hours for Line 2. Graph the region determined by the constraint inequalities.
A) 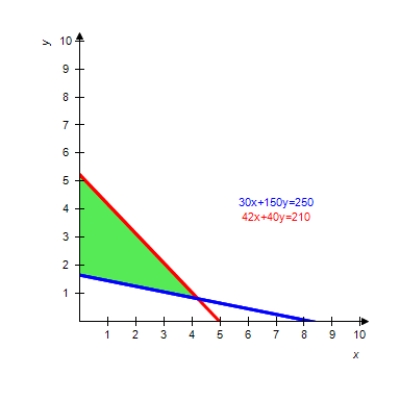
B) 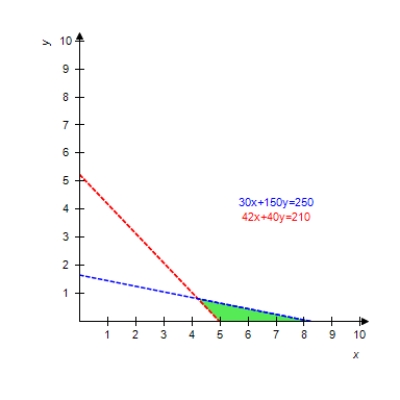
C) 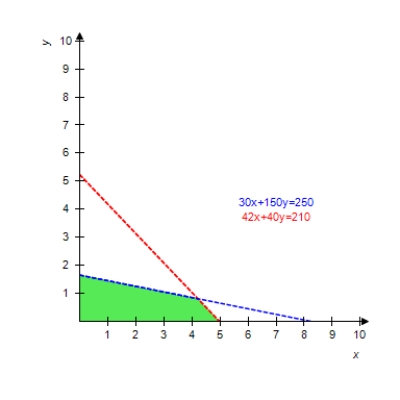
D) 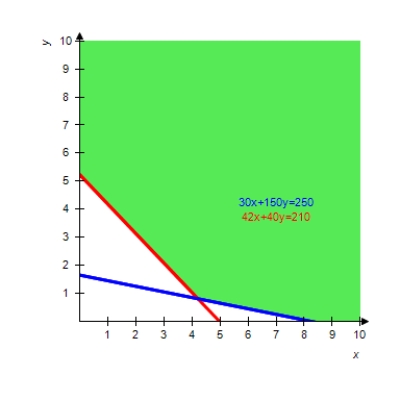
E) 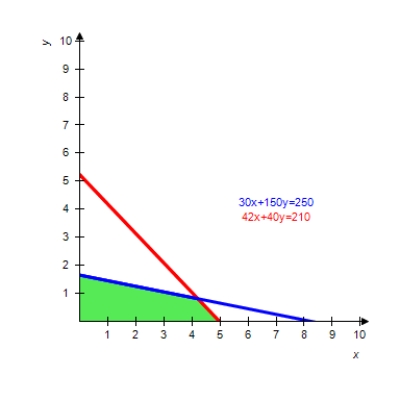
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
6
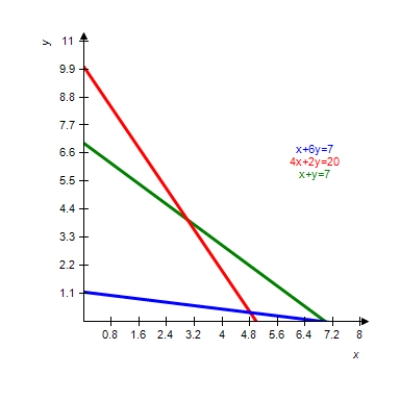
The graph of the boundary equations for the system of inequalities is shown with that system. Locate the solution region and find the corners. 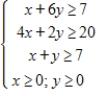
A)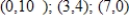
B)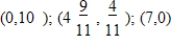
C)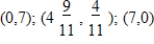
D) 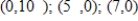
E)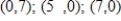
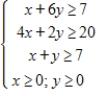

A)
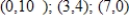
B)
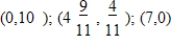
C)
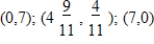
D)
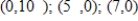
E)
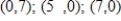

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
7
Graph the inequality. 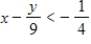
A) 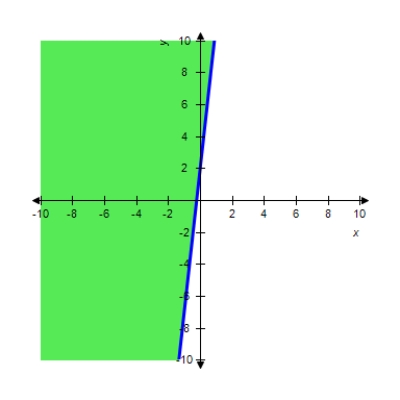
B) 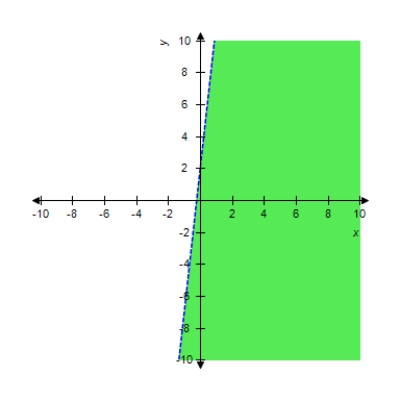
C) 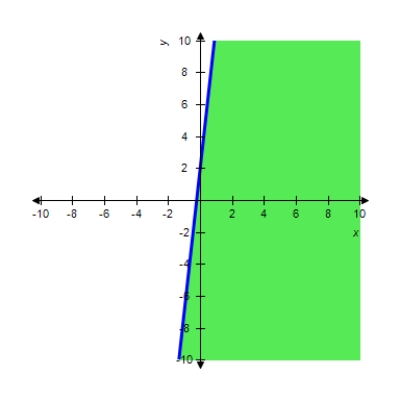
D) 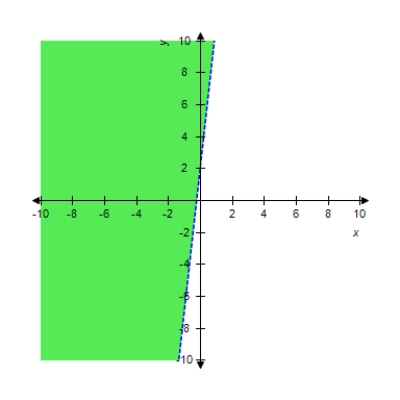
E) 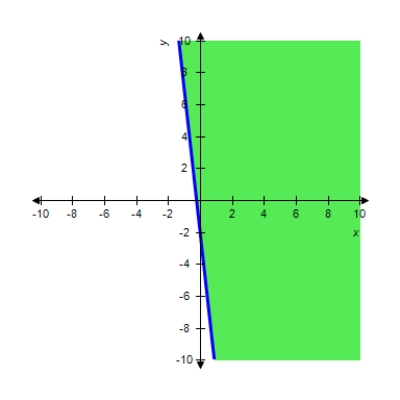
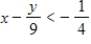
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
8
Graph the solution of the system of inequalities. 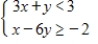
A) 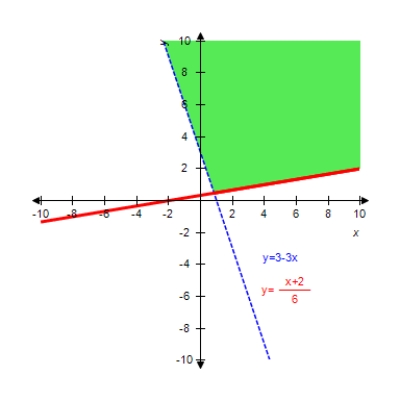
B) 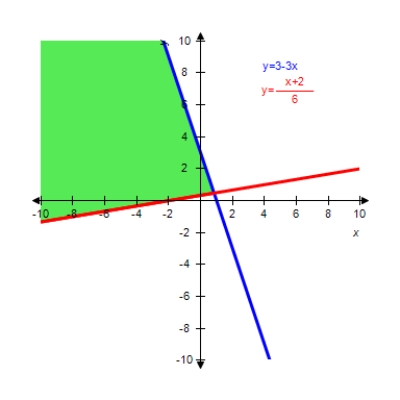
C) 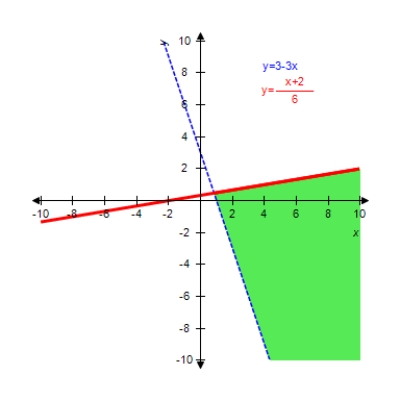
D) 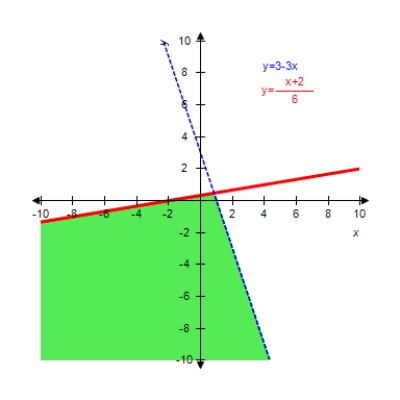
E) 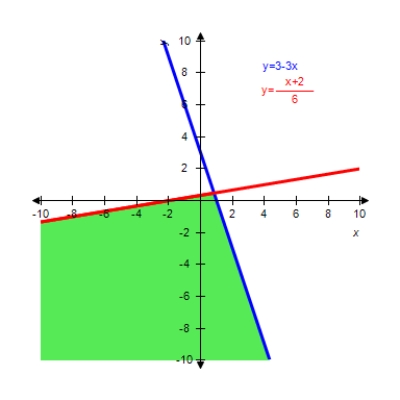
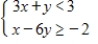
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
9
Graph the solution of the system of inequalities. 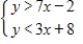
A) 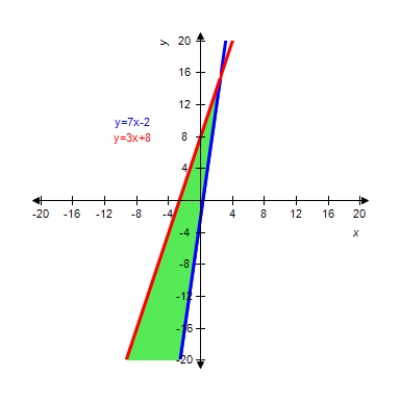
B) 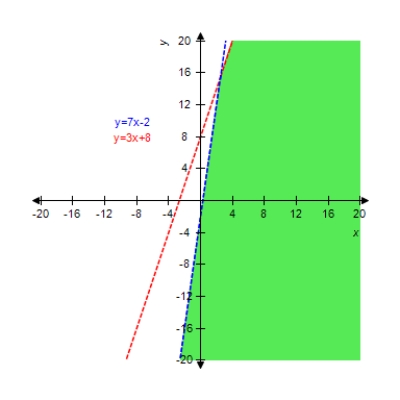
C) 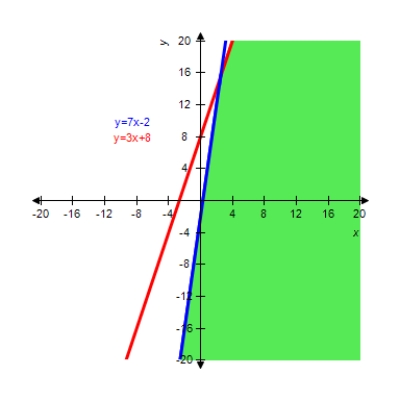
D) 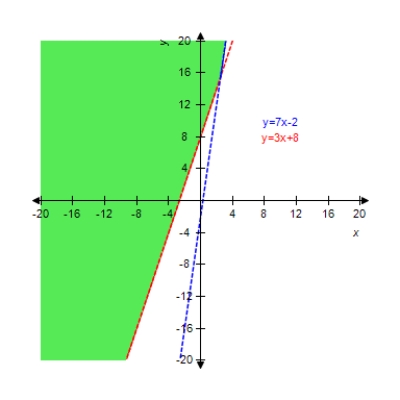
E) 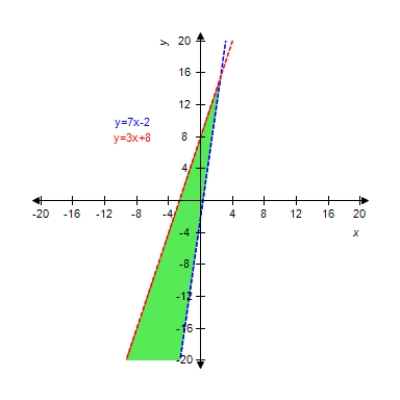
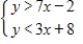
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
10
An experiment that involves learning in animals requires placing white mice and rabbits into separate, controlled environments, environment I and environment II. The maximum amount of time available in environment I 400 is minutes, and the maximum amount of time available in environment II is 500 minutes. The white mice must spend 11 minutes in environment I and 40 minutes in environment II, and the rabbits must spend 35 minutes in environment I and 50 minutes in environment II. Write a system of inequalities that describes the constraints on the number of each type of animal used in the experiment. Let x denote the number of white mice and y denote the number of rabbits.
A) 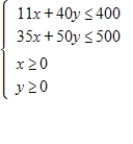
B) 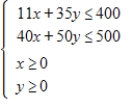
C) 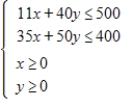
D) 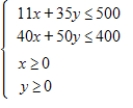
E) 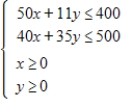
A)
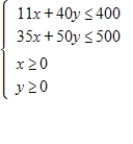
B)
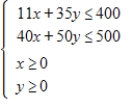
C)
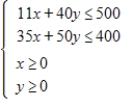
D)
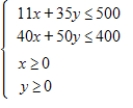
E)
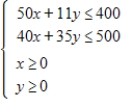

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
11
A company manufactures two types of electric hedge trimmers, one of which is cordless. The cord-type trimmer requires 2 hours to make, and the cordless model requires 7 hours. The company has only 800 work hours to use in manufacturing each day, and the packaging department can package only 300 trimmers per day. Let x represent the number of cord type models and y represent the number of cordless models. Write the inequalities that describe the constraints on the number of each type of hedge trimmer produced.
A)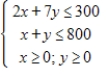
B)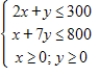
C)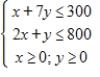
D)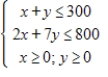
E)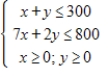
A)
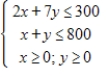
B)
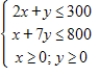
C)
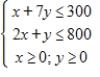
D)
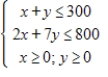
E)
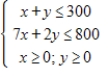

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
12
Graph the solution of the system of inequalities. 
A) 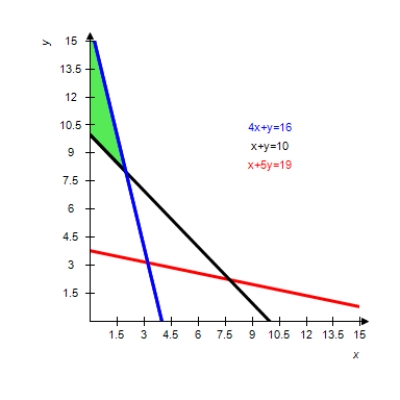
B) 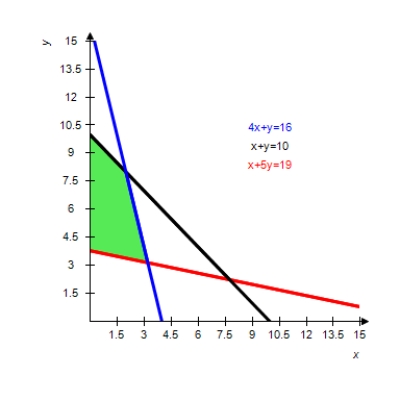
C) 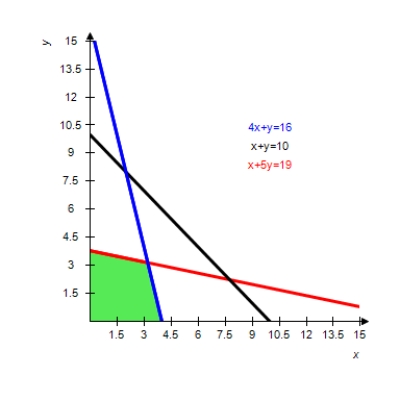
D) 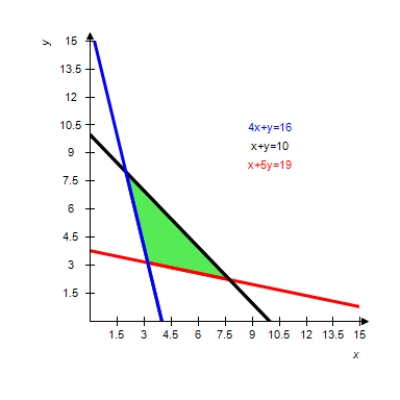
E) 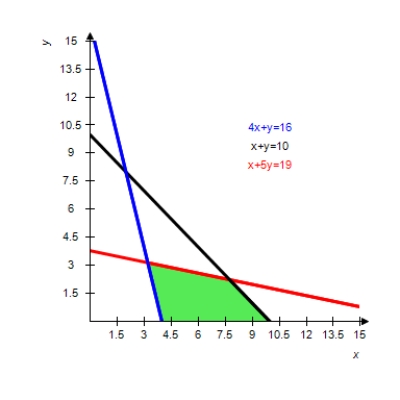
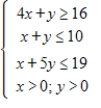
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
13
Apex Motors manufactures luxury cars and sport utility vehicles. The most likely customers are high-income men and women, and company managers want to initiate an advertising campaign targeting these groups. They plan to run 1-minute spots on business/investment programs, where they can reach 7 million women and 4 million men from their target groups. They also plan 1-minute spots during sporting events, where they can reach 2 million women and 12 million men from their target groups. Apex feels that the ads must reach at least 40 million women and at least 24 million men who are prospective customers. Let x represent the number of minutes of business/investment program commercials and y represent the number of minutes of sporting events commercials. Write the inequalities that describe the constraints on the number of each type of 1-minute spots needed to reach these target groups.
A)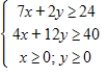
B)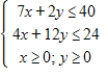
C)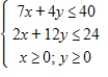
D)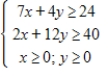
E)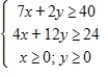
A)
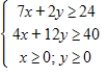
B)
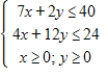
C)
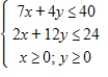
D)
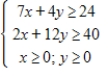
E)
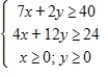

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
14
Apex Motors manufactures luxury cars and sport utility vehicles. The most likely customers are high-income men and women, and company managers want to initiate an advertising campaign targeting these groups. They plan to run 1-minute spots on business/investment programs, where they can reach 7 million women and 4 million men from their target groups. They also plan 1-minute spots during sporting events, where they can reach 2 million women and 12 million men from their target groups. Apex feels that the ads must reach at least 32 million women and at least 20 million men who are prospective customers. Let x represent the number of minutes of business/investment program commercials and y represent the number of minutes of sporting events commercials. Graph the region determined by the constraint inequalities.
A) 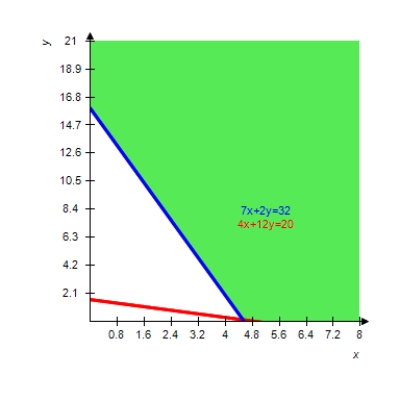
B) 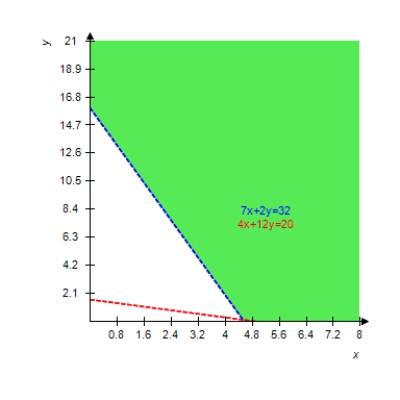
C) 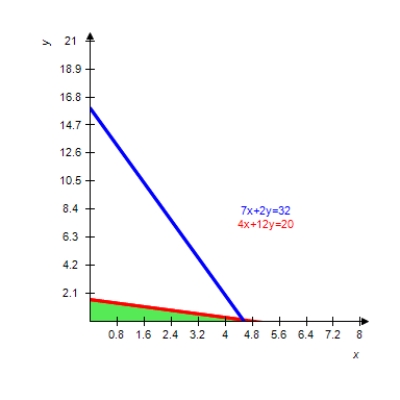
D) 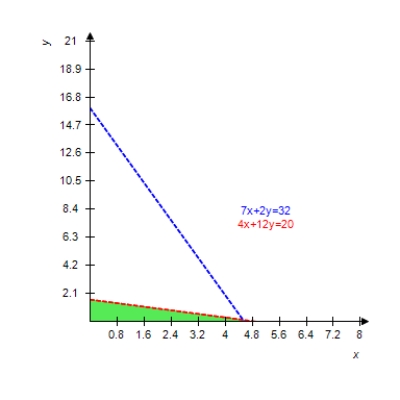
E) 
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
15
The Wellbuilt Company produces two types of wood chippers, economy and deluxe. The deluxe model requires 3 hours to assemble and 4 hour to paint, and the economy model requires 2 hours to assemble and 1 hour to paint. The maximum number of assembly hours available is 24 per day, and the maximum number of painting hours available is 17 per day. Let x represent the number of deluxe models and y represent the number of economy models. Graph the solution of the system of inequalities and find the corners of the solution region.
A) 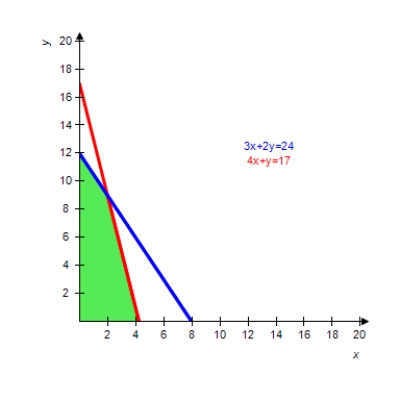
B) 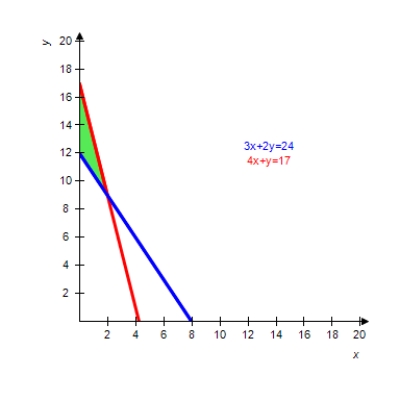
C) 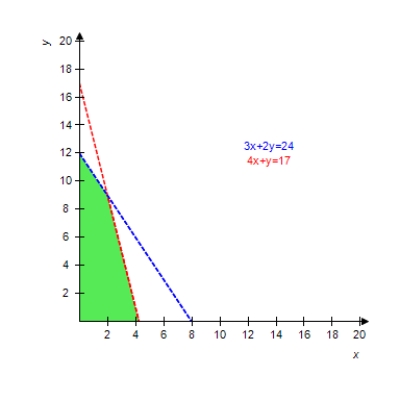
D) 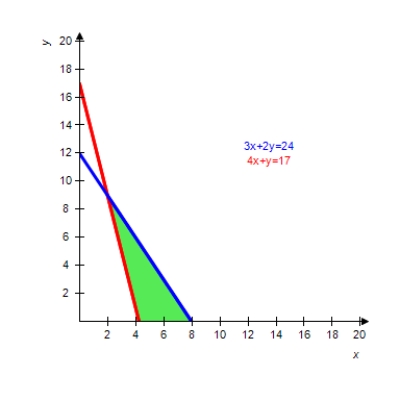
E) 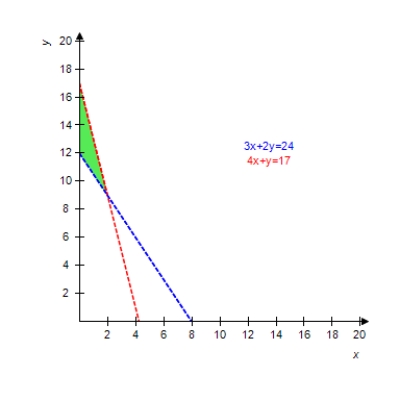
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
16
Graph the solution of the system of inequalities. 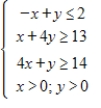
A) 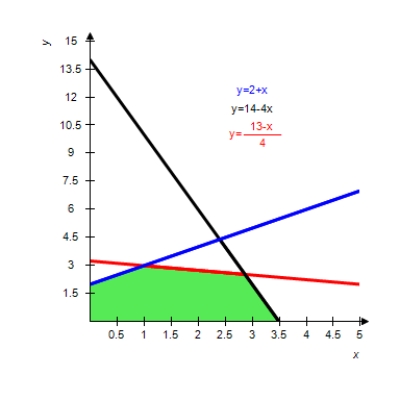
B) 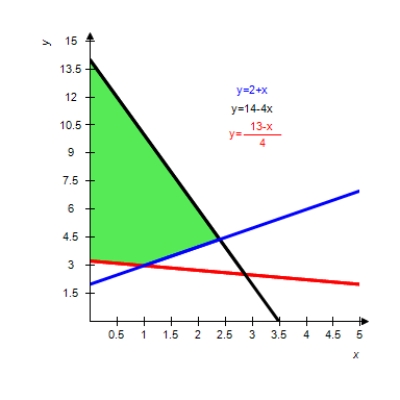
C) 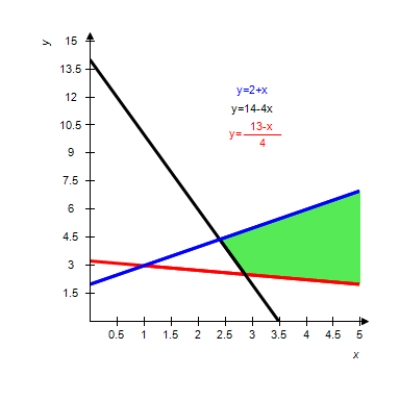
D) 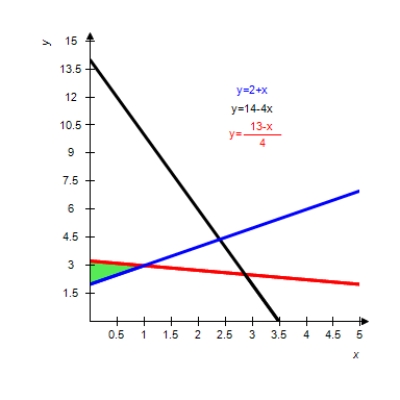
E) 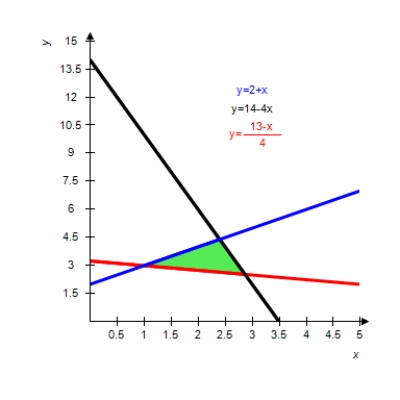
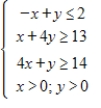
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
17
Graph the solution of the system of inequalities. 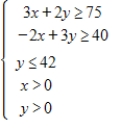
A) 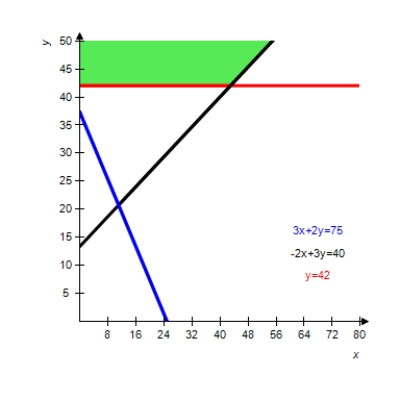
B) 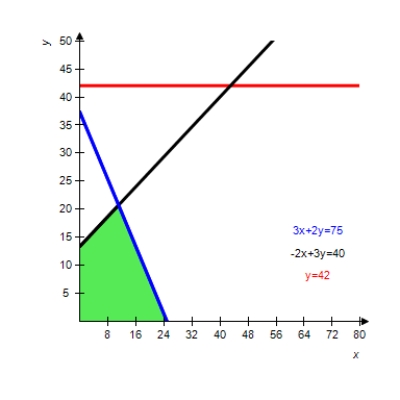
C) 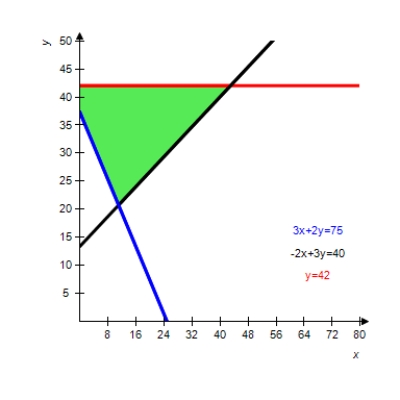
D) 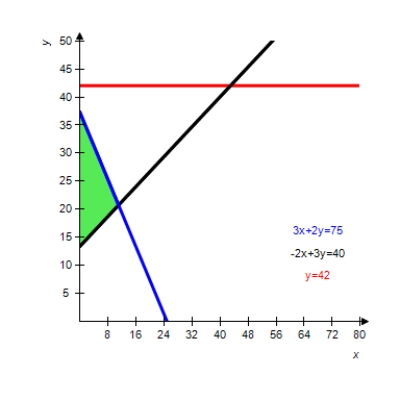
E) 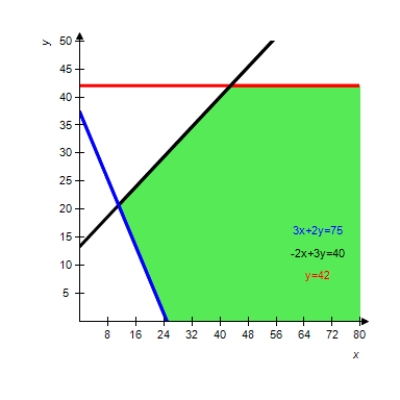
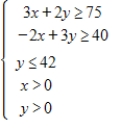
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
18
Suppose that an experiment that involves learning in animals requires placing white mice and rabbits into separate, controlled environments, environment I and environment II. The maximum amount of time available in environment I is 300 minutes, and the maximum amount of time available in environment II is 400 minutes. The white mice must spend 20 minutes in environment I and 35 minutes in environment II, and the rabbits must spend 45 minutes in environment I and 50 minutes in environment II. Let x represent number of white mice and y represent number of rabbits. Determine the system of inequalities that describes the constraints on the number of each type of animal used in the experiment. Then graph the solution of the system of inequalities.
A) 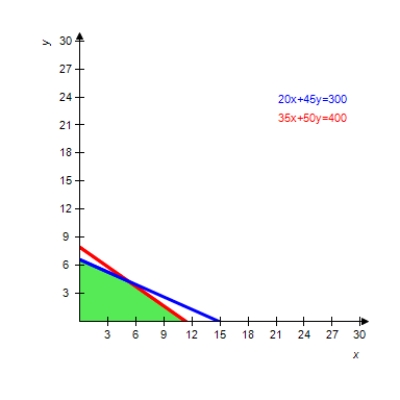
B) 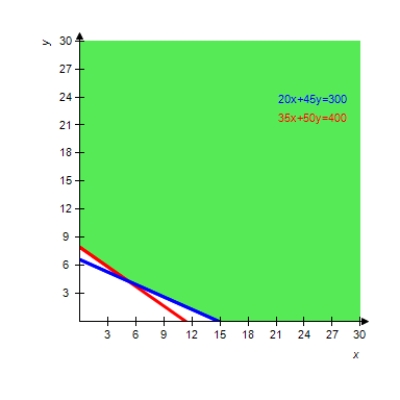
C) 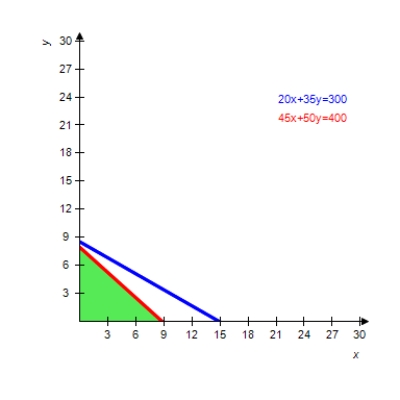
D) 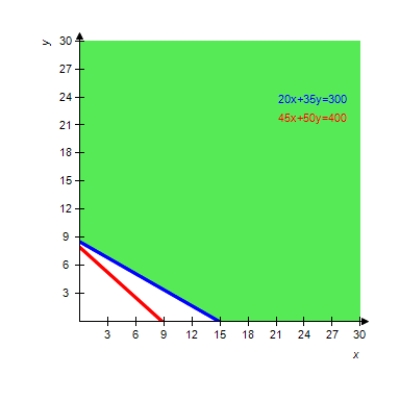
E) 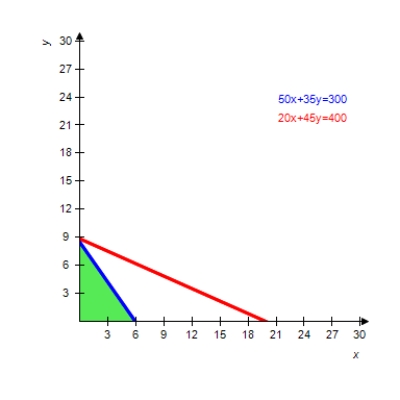
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
19
Graph the inequality. 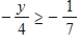
A) 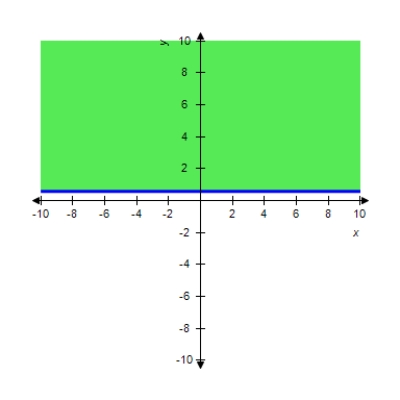
B) 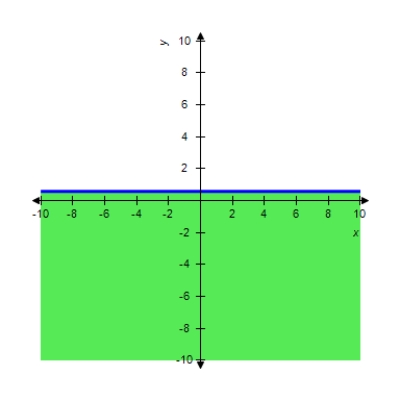
C) 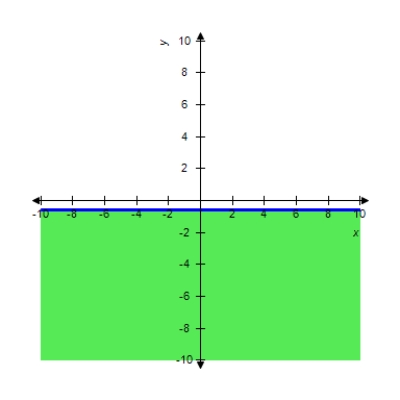
D) 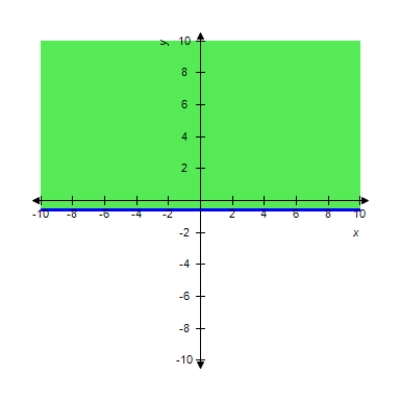
E) 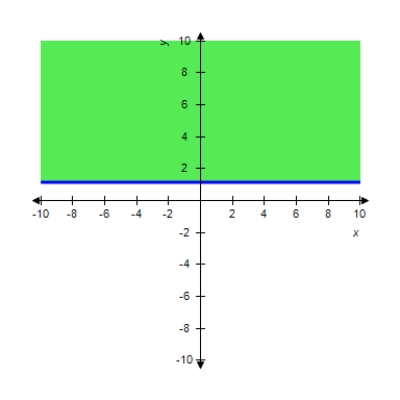
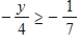
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
20
A company manufactures two types of electric hedge trimmers, one of which is cordless. The cord-type trimmer requires 7 hours to make, and the cordless model requires 1.5 hours. The company has only 800 work hours to use in manufacturing each day, and the packaging department can package only 300 trimmers per day. Let x represent the number of cord type models and y represent the number of cordless models. Graph the solution of the system of inequalities.
A) 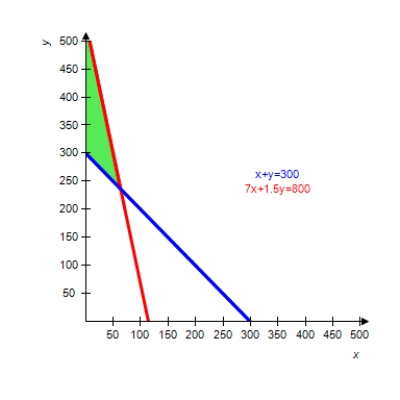
B) 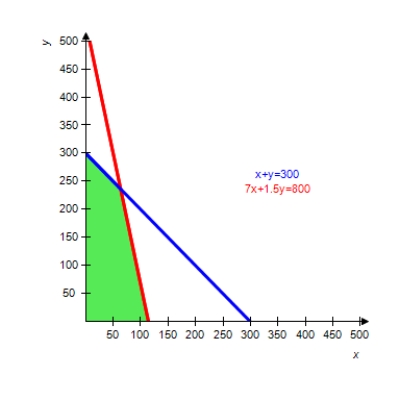
C) 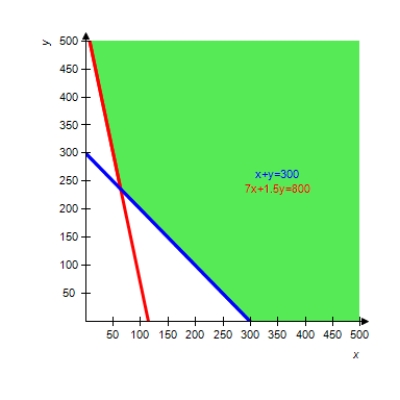
D) 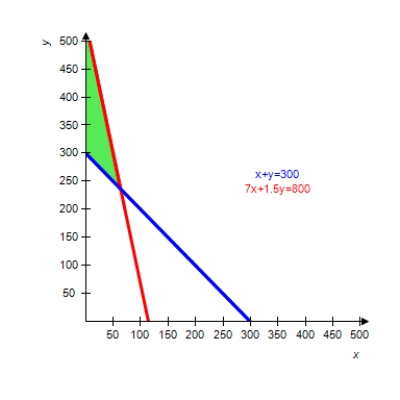
E) 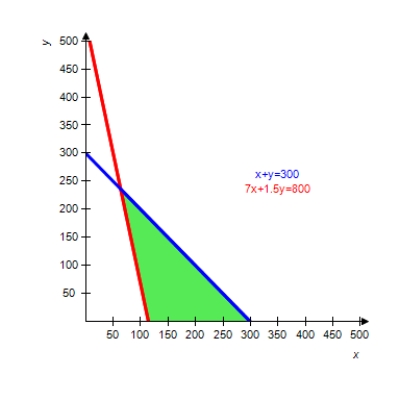
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
21
Use the given feasible region determined by the constraint inequalities to find the maximum and minimum of the given objective function (if they exist). 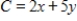
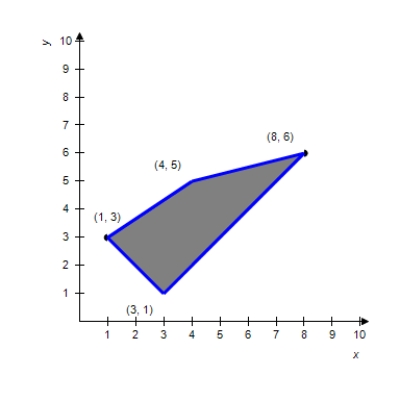
A) max: 110, min: 24
B) max: 52, min: 11
C) max: 11, min: 17
D) max: 46, min: 11
E) do not exist
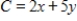

A) max: 110, min: 24
B) max: 52, min: 11
C) max: 11, min: 17
D) max: 46, min: 11
E) do not exist

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
22
A farm co-op has 6,001 acres available to plant with corn and soybeans. Each acre of corn requires 9 gallons of fertilizer/herbicide and 0.75 hour of labor to harvest. Each acre of soybeans requires 3 gallons of fertilizer/herbicide and 1 hour of labor to harvest. The co-op has available at most 40,500 gallons of fertilizer/herbicide and at most 5,250 hours of labor for harvesting. Find the maximum profit if the profits per acre are $75 for corn and $45 for soybeans. Round your answer to the nearest cent if necessary.
A) $236,250.00
B) $393,750.00
C) $202,500.00
D) $382,530.00
E) $335,472.97
A) $236,250.00
B) $393,750.00
C) $202,500.00
D) $382,530.00
E) $335,472.97

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
23
Use the given feasible region determined by the constraint inequalities to find the maximum and minimum of the objective function 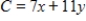 .
. 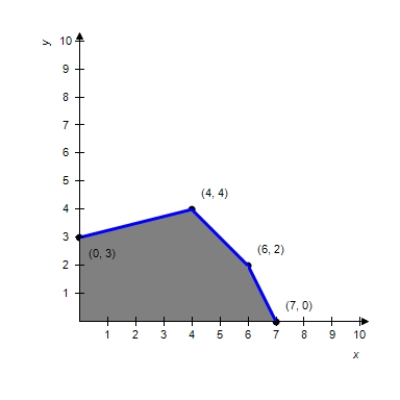
A) maximum value of C: 72 at (4,4); minimum value of C: 33 at (0,3)
B) maximum value of C: 72 at (4,4); minimum value of C: 49 at (7,0)
C) maximum value of C: 64 at (6,2); minimum value of C: 0 at (0,0)
D) maximum value of C: 72 at (4,4); minimum value of C: 0 at (0,0)
E) maximum value of C: 64 at (6,2); minimum value of C: 33 at (0,3)
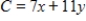 .
. 
A) maximum value of C: 72 at (4,4); minimum value of C: 33 at (0,3)
B) maximum value of C: 72 at (4,4); minimum value of C: 49 at (7,0)
C) maximum value of C: 64 at (6,2); minimum value of C: 0 at (0,0)
D) maximum value of C: 72 at (4,4); minimum value of C: 0 at (0,0)
E) maximum value of C: 64 at (6,2); minimum value of C: 33 at (0,3)

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
24
A sausage company makes two different kinds of hot dogs, regular and all beef. Each pound of all-beef hot dogs requires 0.79 lb of beef and 0.24 lb of spices, and each pound of regular hot dogs requires 0.17 lb of beef, 0.34 lb of pork, and 0.22 lb of spices. Suppliers can deliver at most 1044 lb of beef, at most 402 lb of pork, and at least 300 lb of spices. Let x represent the number of pounds of regular meat and y represent the number of pounds of all beef. Graph the region determined by the constraint inequalities.
A) 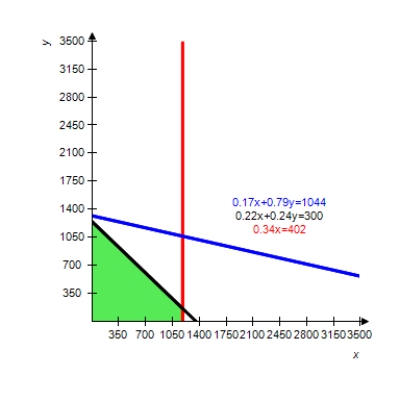
B) 
C) 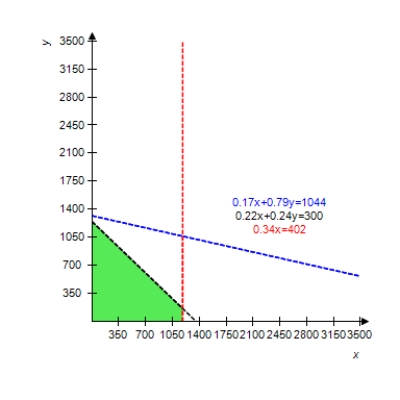
D) 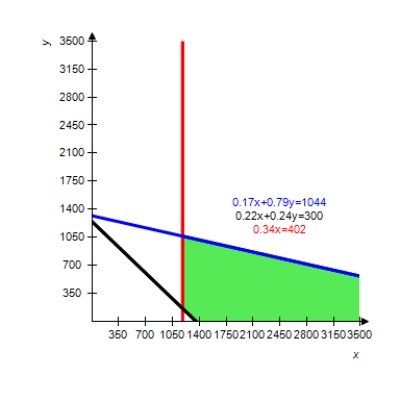
E) 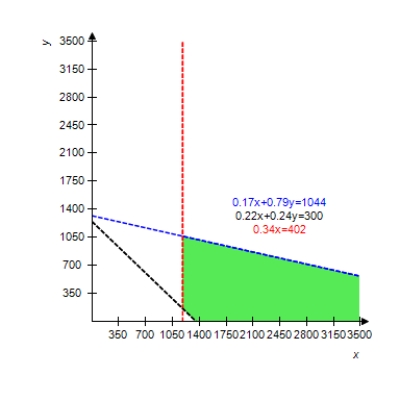
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the following linear programming problem.
Minimize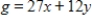 subject to
subject to
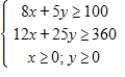
A) 810
B) 360
C) 240
D) 540
E) 324
Minimize
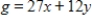 subject to
subject to
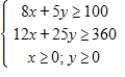
A) 810
B) 360
C) 240
D) 540
E) 324

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
26
Solve the following linear programming problem.
Maximize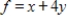 subject to
subject to

A) 36
B) 9
C) 2
D) 15
E) 12
Maximize
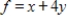 subject to
subject to

A) 36
B) 9
C) 2
D) 15
E) 12

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
27
The Wellbuilt Company produces two types of wood chippers, economy and deluxe. The deluxe model requires 3 hours to assemble and 0.5 hour to paint, and the economy model requires 2 hours to assemble and 1 hour to paint. The maximum number of assembly hours available is 24 per day, and the maximum number of painting hours available is 8 per day. If the profit on the deluxe model is $18 per unit and the profit on the economy model is $14 per unit, find the maximum profit. Round your answer to the nearest dollar if necessary.
A) $112
B) $164
C) $144
D) $256
E) $156
A) $112
B) $164
C) $144
D) $256
E) $156

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
28
At one of its factories, a jeans manufacturer makes two styles: #891 and #917. Each pair of style-891 takes 10 minutes to cut out and 20 minutes to assemble and finish. Each pair of style-917 takes 10 minutes to cut out and 30 minutes to assemble and finish. The plant has enough workers to provide at most 7,500 minutes per day for cutting and at most 19,500 minutes per day for assembly and finishing. The profit on each pair of style-891 is $6.00 and the profit on each pair of style-917 is $9.50. Find the maximum daily profit. Round your answer to the nearest cent if necessary.
A) $6175.00
B) $4500.00
C) $7125.00
D) $3900.00
E) $5600.00
A) $6175.00
B) $4500.00
C) $7125.00
D) $3900.00
E) $5600.00

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
29
Solve the following linear programming problem.
Maximize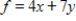 subject to
subject to
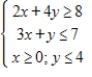
A) 16
B) 9
C) 14
D) 15
E) 49
Maximize
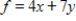 subject to
subject to
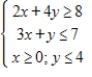
A) 16
B) 9
C) 14
D) 15
E) 49

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
30
Solve the following linear programming problem.
Minimize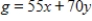 subject to
subject to

A) 1,485
B) 825
C) 1,890
D) 1,050
E) 630
Minimize
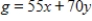 subject to
subject to

A) 1,485
B) 825
C) 1,890
D) 1,050
E) 630

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
31
Solve the following linear programming problem.
Maximize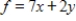 subject to
subject to
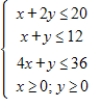
A) 20
B) 64
C) 24
D) 84
E) 70
Maximize
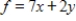 subject to
subject to
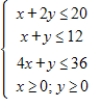
A) 20
B) 64
C) 24
D) 84
E) 70

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
32
The graph of the feasible region is shown. Locate the corners of the feasible region in order to find the minimum of the given objective function (if it exists). 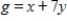
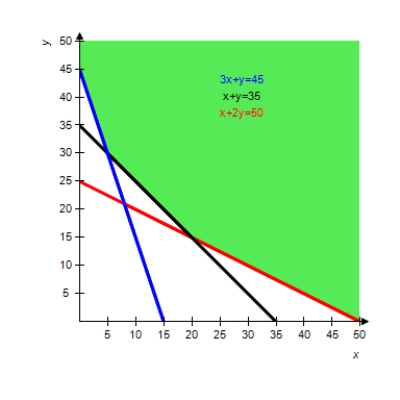
A) 50
B) 45
C) 215
D) 125
E) does not exist

A) 50
B) 45
C) 215
D) 125
E) does not exist

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
33
A cereal manufacturer makes two different kinds of cereal, Senior Citizen's Feast and Kids Go. Each pound of Senior Citizen's Feast requires 0.8 lb of wheat and 0.2 lb of vitamin-enriched syrup, and each pound of Kids Go requires 0.4 lb of wheat, 0.2 lb of sugar, and 0.2 lb of vitamin-enriched syrup. Suppliers can deliver at most 2880 lb of wheat, at most 850 lb of sugar, and at least 900 lb of the vitamin-enriched syrup. Let x represent the number of pounds of Senior Citizen's cereal and y represent the number of pounds of Kids Go cereal. Graph the region determined by the constraint inequalities.
A) 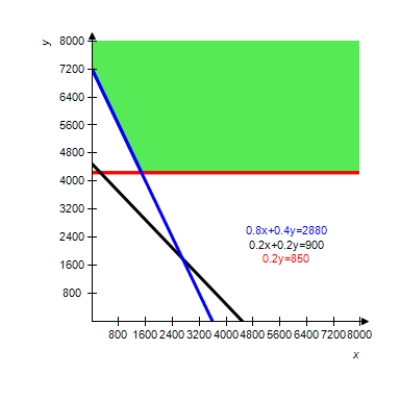
B) 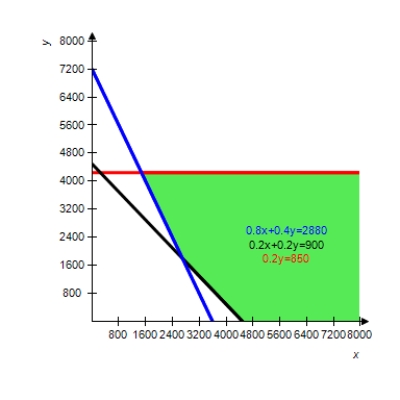
C) 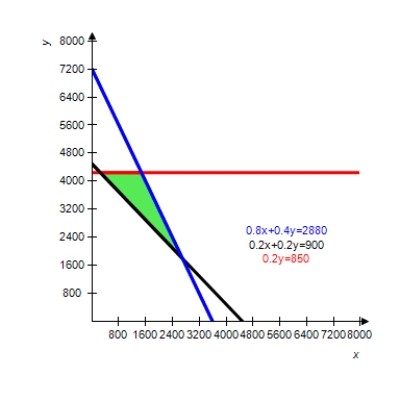
D) 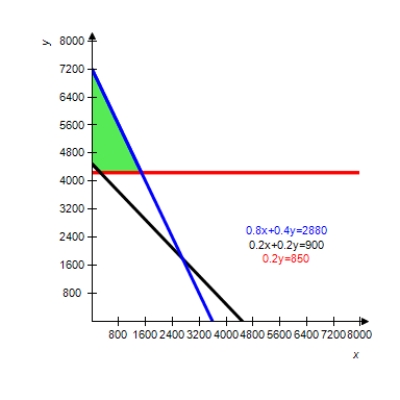
E) 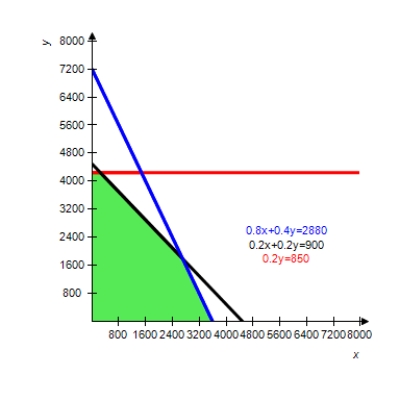
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
34
Solve the following linear programming problem.
Minimize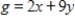 subject to
subject to
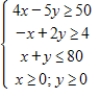
A) 720
B) 18
C) 370
D) 4
E) 278
Minimize
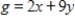 subject to
subject to
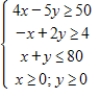
A) 720
B) 18
C) 370
D) 4
E) 278

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
35
The graph of the feasible region is shown. Locate the corners of the feasible region in order to find the maximum of the given objective function (if it exists). 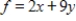
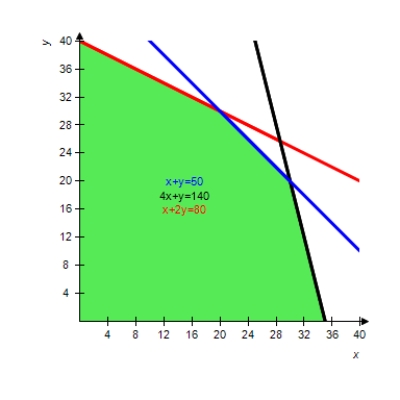
A) 240
B) 360
C) 70
D) 100
E) does not exist
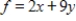

A) 240
B) 360
C) 70
D) 100
E) does not exist

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
36
A sausage company makes two different kinds of hot dogs, regular and beef. Each pound of beef hot dogs requires 0.75 lb of beef and 0.2 lb of spices, and each pound of regular hot dogs requires 0.17 lb of beef, 0.31 lb of pork, and 0.22 lb of spices. Suppliers can deliver at most 1020 lb of beef, at most 600 lb of pork, and at least 500 lb of spices. Let x represent the number of pounds of regular meat and y represent the number of pounds of beef. Write the inequalities that describe how many pounds of each type of hot dog can be produced.
A)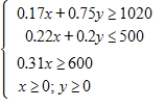
B)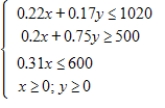
C)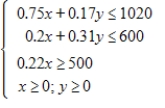
D)
E)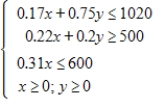
A)
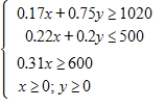
B)
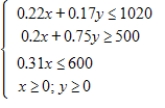
C)
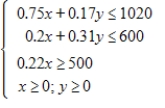
D)

E)
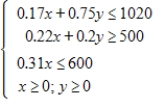

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
37
A cereal manufacturer makes two different kinds of cereal, Senior Citizen's Feast and Kids Go. Each pound of Senior Citizen's Feast requires 0.6 lb of wheat and 0.2 lb of vitamin-enriched syrup, and each pound of Kids Go requires 0.4 lb of wheat, 0.2 lb of sugar, and 0.2 lb of vitamin-enriched syrup. Suppliers can deliver at most 2800 lb of wheat, at most 800 lb of sugar, and at least 1000 lb of the vitamin-enriched syrup. Let x represent the number of pounds of Senior Citizen's cereal and y represent the number of pounds of Kids Go cereal. Write the inequalities that describe how many pounds of each type of cereal can be made.
A)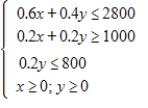
B)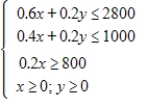
C)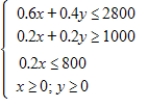
D)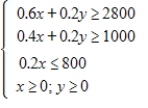
E)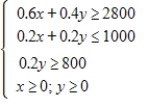
A)
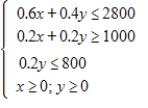
B)
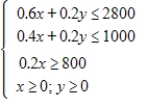
C)
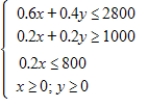
D)
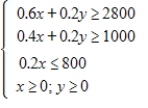
E)
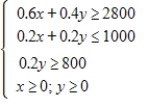

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
38
A candidate wishes to use a combination of radio and television advertisements in her campaign. Assume that each 1-minute spot on television reaches to 0.0945 million people and that each 1-minute spot on radio reaches to 0.003 million. The candidate feels she must reach at least 8.775 million people, and she must buy total of at least 85 minutes of advertisements. How many minutes of each medium should be used to minimize costs if television costs $450 per minute and radio costs $75 per minute? Round your answer to nearest integer if necessary.
A) Minimum cost is $41,786 with 0 minutes of TV time and 93 minutes of radio time.
B) Minimum cost is $41,786 with 93 minutes of TV time and 0 minutes of radio time.
C) Minimum cost is $8,839 with 5 minutes of TV time and 88 minutes of radio time.
D) Minimum cost is $8,839 with 88 minutes of TV time and 5 minutes of radio time.
E) Minimum cost is $7,339 with 0 minutes of TV time and 98 minutes of radio time.
A) Minimum cost is $41,786 with 0 minutes of TV time and 93 minutes of radio time.
B) Minimum cost is $41,786 with 93 minutes of TV time and 0 minutes of radio time.
C) Minimum cost is $8,839 with 5 minutes of TV time and 88 minutes of radio time.
D) Minimum cost is $8,839 with 88 minutes of TV time and 5 minutes of radio time.
E) Minimum cost is $7,339 with 0 minutes of TV time and 98 minutes of radio time.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
39
Suppose that in a hospital ward, the patients can be grouped into two general categories depending on their condition and the amount of solid foods they require in their diet. A combination of two diets is used for solid foods because they supply essential nutrients for recovery. The table below summarizes the patient groups and their minimum daily requirements. Let x represent the number of servings of A and y represent the number of servings of B. Determine the inequalities that describe how many servings of each diet are needed to provide the nutritional requirements and then graph the region determined by these constraint inequalities.
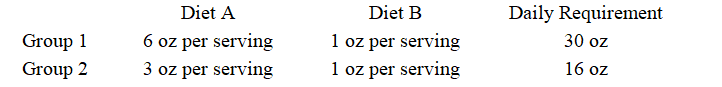
A) 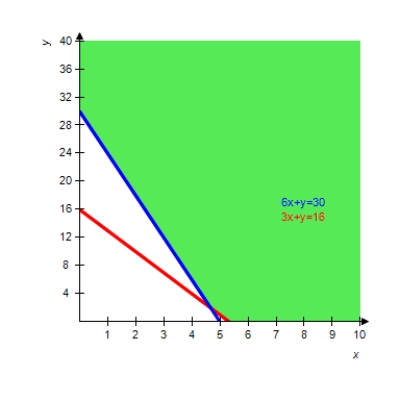
B) 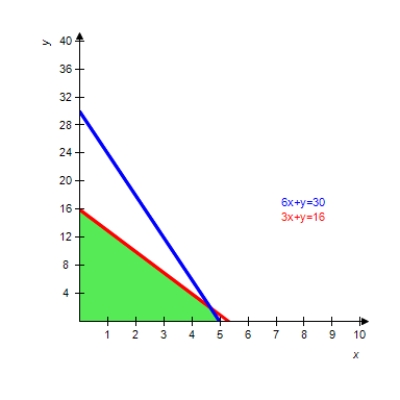
C) 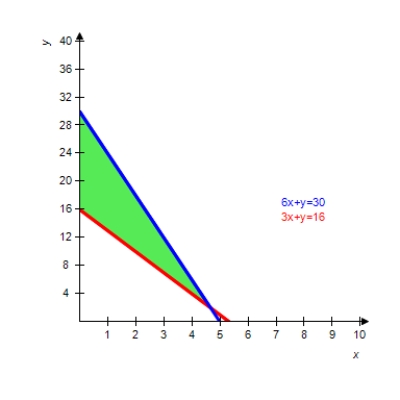
D) 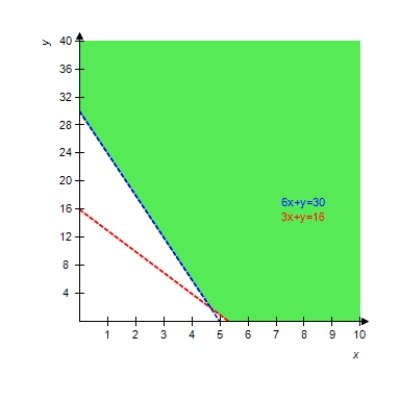
E) 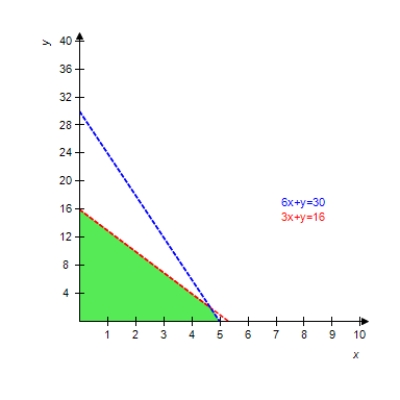
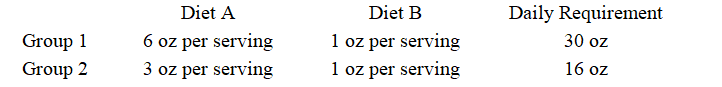
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
40
Suppose that in a hospital ward, the patients can be grouped into two general categories depending on their condition and the amount of solid foods they require in their diet. A combination of two diets is used for solid foods because they supply essential nutrients for recovery. The table below summarizes the patient groups and their minimum daily requirements. Let x represent number of servings of Diet A and y represent number of servings of Diet B. Write the inequalities that describe how many servings of each diet are needed to provide the nutritional requirements.
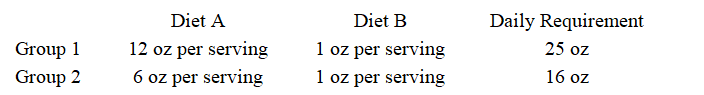
A) 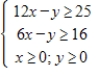
B) 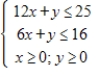
C) 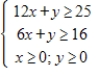
D) 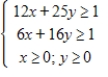
E) 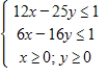
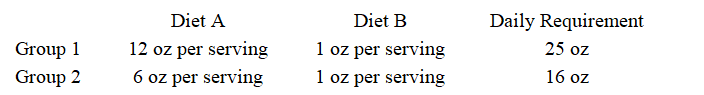
A)
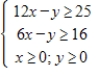
B)
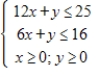
C)
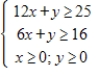
D)
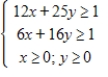
E)
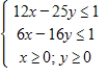

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
41
A contractor builds two types of homes. The Carolina requires one lot, $160,000 capital, and 160 worker-days of labor, whereas the Savannah requires one lot, $240,000 capital, and 160 worker-days of labor. The contractor owns 300 lots and has $48,000,000 available capital and 43,200 worker-days of labor. The profit on the Carolina is $40,000 and the profit on the Savannah is $52,000. Use the corner points of the feasible region to find how many of each type of home should be built to maximize profit. Find the maximum possible profit. Round your profit to the nearest dollar, another answers - to the nearest whole number.
A) Maximum profit of $13,320,000 obtained by building 60 Carolina homes and 210 Savannah homes.
B) Maximum profit of $12,000,000 obtained by building 300 Carolina homes and 0 Savannah homes.
C) Maximum profit of $11,520,000 obtained by building 210 Carolina homes and 60 Savannah homes.
D) Maximum profit of $21,200,000 obtained by building 270 Carolina homes and 200 Savannah homes.
E) Maximum profit of $18,400,000 obtained by building 200 Carolina homes and 200 Savannah homes.
A) Maximum profit of $13,320,000 obtained by building 60 Carolina homes and 210 Savannah homes.
B) Maximum profit of $12,000,000 obtained by building 300 Carolina homes and 0 Savannah homes.
C) Maximum profit of $11,520,000 obtained by building 210 Carolina homes and 60 Savannah homes.
D) Maximum profit of $21,200,000 obtained by building 270 Carolina homes and 200 Savannah homes.
E) Maximum profit of $18,400,000 obtained by building 200 Carolina homes and 200 Savannah homes.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
42
In a laboratory experiment, two separate foods are given to experimental animals. Each food contains essential ingredients, A and B, for which the animals have a minimum requirement, and each food also has an ingredient C, which can be harmful to the animals. The table below summarizes this information.

Determine how many grams of foods 1 and 2 should be given in order to satisfy the requirements for A and B while minimizing the amount of ingredient C ingested. Also determine the minimum amount of ingredient C ingested. Round your answer to one decimal place if necessary.
A) 11 grams of food 1 and 0 gram0 of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 11 grams.
B) 0 gram0 of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 11 grams.
C) 12 grams of food 1 and 0 gram0 of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 60 grams.
D) 1 gram0 of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 16 grams.
E) 2 grams of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 21 grams.

Determine how many grams of foods 1 and 2 should be given in order to satisfy the requirements for A and B while minimizing the amount of ingredient C ingested. Also determine the minimum amount of ingredient C ingested. Round your answer to one decimal place if necessary.
A) 11 grams of food 1 and 0 gram0 of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 11 grams.
B) 0 gram0 of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 11 grams.
C) 12 grams of food 1 and 0 gram0 of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 60 grams.
D) 1 gram0 of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 16 grams.
E) 2 grams of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 21 grams.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
43
Use the simplex method to maximize the given function. Assume all variables are nonnegative.
Maximize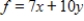 subject to
subject to
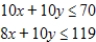
A) maximum; 49 at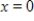 ,
, 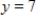
B) maximum; 119 at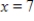 ,
, 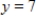
C) maximum; 70 at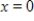 ,
, 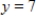
D) maximum; 300 at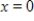 ,
, 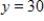
E) maximum; 10 at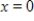 ,
, 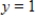
Maximize
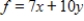 subject to
subject to
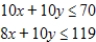
A) maximum; 49 at
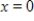 ,
, 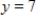
B) maximum; 119 at
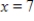 ,
, 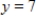
C) maximum; 70 at
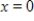 ,
, 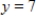
D) maximum; 300 at
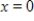 ,
, 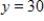
E) maximum; 10 at
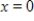 ,
, 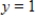

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
44
A farm co-op has over 6,000 acres available to plant with corn and soybeans. The farm co-op's maximum profit for planting 6,000 acres is $315,000 and the maximum profit for 6,008 acres is $315,320. What is the profit value of each additional acre of land? This value is called the shadow price of an acre of land. Round your answer to the nearest cent if necessary.
A) $320.00
B) $160.00
C) $35.56
D) $45.71
E) $40.00
A) $320.00
B) $160.00
C) $35.56
D) $45.71
E) $40.00

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
45
A farm co-op has over 40,500 gallons of fertilizer/herbicide available to use when planting corn and soybeans. The farm co-op's maximum profit for using 40,500 gallons of fertilizer/herbicide is $315,009 and the maximum profit for 40,508 gallons is $315,034. What is the profit value of each additional gallon of fertilizer/herbicide (that is, the shadow price of a gallon of fertilizer/herbicide)? Round your answer to the nearest cent if necessary.
A) $3.57
B) $3.13
C) $2.78
D) $25.00
E) $12.50
A) $3.57
B) $3.13
C) $2.78
D) $25.00
E) $12.50

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
46
The Janie Gioffre Drapery Company makes three types of draperies at two different locations. At location I, it can make 10 pairs of deluxe drapes, 20 pairs of better drapes, and 13 pairs of standard drapes per day. At location II, it can make 20 pairs of deluxe drapes, 50 pairs of better drapes, and 6 pairs of standard drapes per day. The company has orders for 2,000 pairs of deluxe drapes, 4,200 pairs of better drapes, and 1,200 pairs of standard drapes. If the daily costs are $700 per day at location I and $800 per day at location II, how many days should Janie schedule at each location in order to fill the orders at minimum cost? Find the minimum cost. Round your answers to the nearest whole number if necessary.
A) Minimum cost of $160,000 occurs with 0 days at location I and 200 days at location II.
B) Minimum cost of $140,000 occurs with 200 days at location I and 0 days at location II.
C) Minimum cost of $97,000 occurs with 70 days at location I and 60 days at location II.
D) Minimum cost of $128,000 occurs with 160 days at location I and 20 days at location II.
E) Minimum cost of $98,000 occurs with 60 days at location I and 70 days at location II.
A) Minimum cost of $160,000 occurs with 0 days at location I and 200 days at location II.
B) Minimum cost of $140,000 occurs with 200 days at location I and 0 days at location II.
C) Minimum cost of $97,000 occurs with 70 days at location I and 60 days at location II.
D) Minimum cost of $128,000 occurs with 160 days at location I and 20 days at location II.
E) Minimum cost of $98,000 occurs with 60 days at location I and 70 days at location II.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
47
A farm co-op has 6,000 acres available to plant with corn and soybeans. Each acre of corn requires 9 gallons of fertilizer/herbicide and 0.75 hour of labor to harvest. Each acre of soybeans requires 3 gallons of fertilizer/herbicide and 1hour of labor to harvest. The co-op has available at most 40,508 gallons of fertilizer/herbicide and at most 5,250 hours of labor for harvesting. Find the maximum profit if the profits per acre are $65 for corn and $50 for soybeans. Round your answer to the nearest cent if necessary.
A) $262,500.00
B) $356,270.00
C) $363,365.75
D) $333,730.00
E) $345,000.00
A) $262,500.00
B) $356,270.00
C) $363,365.75
D) $333,730.00
E) $345,000.00

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
48
A simplex matrix for a standard maximization problem is given. Indicate whether or not the solution shown is complete (optimal). If the solution is not complete, find the next pivot or indicate that no solution exists. 
A) next pivot: 1 in R4C3
B) next pivot: -2 in R4C5
C) next pivot: 7 in R2C5
D) optimal solution
E) no solution

A) next pivot: 1 in R4C3
B) next pivot: -2 in R4C5
C) next pivot: 7 in R2C5
D) optimal solution
E) no solution

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
49
A simplex matrix is given. In this case, the solution is complete, so identify the maximum value of f and a set of values for the variables that gives this maximum value. If multiple solutions may exist, indicate this and locate the next pivot. 
A) The maximum value of f is 82 when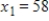 ,
, 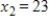 and
and 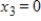 .
.
B) The maximum value of f is 82 when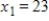 ,
, 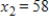 and
and 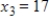 .
.
C) The maximum value of f is 82 when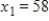 ,
, 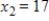 . Since multiple solutions are possible, pivot on 2 in R1C3.
. Since multiple solutions are possible, pivot on 2 in R1C3.
D) The maximum value of f is 82 when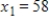 ,
, 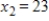 . Since multiple solutions are possible, pivot on 1 in R1C4.
. Since multiple solutions are possible, pivot on 1 in R1C4.
E) The maximum value of f is 82 when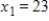 ,
, 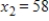 . Since multiple solutions are possible, pivot on 1 in R2C4.
. Since multiple solutions are possible, pivot on 1 in R2C4.

A) The maximum value of f is 82 when
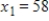 ,
, 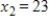 and
and 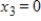 .
.B) The maximum value of f is 82 when
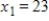 ,
, 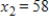 and
and 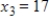 .
.C) The maximum value of f is 82 when
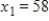 ,
, 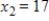 . Since multiple solutions are possible, pivot on 2 in R1C3.
. Since multiple solutions are possible, pivot on 2 in R1C3.D) The maximum value of f is 82 when
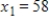 ,
, 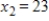 . Since multiple solutions are possible, pivot on 1 in R1C4.
. Since multiple solutions are possible, pivot on 1 in R1C4.E) The maximum value of f is 82 when
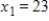 ,
, 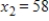 . Since multiple solutions are possible, pivot on 1 in R2C4.
. Since multiple solutions are possible, pivot on 1 in R2C4.
Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
50
A farm co-op has 6,000 acres available to plant with corn and soybeans. Each acre of corn requires 9 gallons of fertilizer/herbicide and 0.75 hour of labor to harvest. Each acre of soybeans requires 3 gallons of fertilizer/herbicide and 1hour of labor to harvest. The co-op has available at most 40,501 gallons of fertilizer/herbicide and at most 5,250 hours of labor for harvesting. Find the maximum profit if the profits per acre are $75 for corn and $40 for soybeans. Round your answer to the nearest cent if necessary.
A) $210,000.00
B) $393,750.00
C) $371,255.83
D) $180,004.44
E) $318,744.17
A) $210,000.00
B) $393,750.00
C) $371,255.83
D) $180,004.44
E) $318,744.17

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
51
Two foods contain only proteins, carbohydrates, and fats. Food A costs $1.30 per pound and contains 30% protein and 50% carbohydrates. Food B costs $1.95 per pound and contains 20% protein and 75% carbohydrates. What combination of these two foods provides at least 1 pound of protein, 2.5 pounds of carbohydrates, and 0.25 pounds of fat at the lowest cost? Round your answer to the nearest cent.
A) Minimum cost of $6.50 occurs on the line between (2,2) and (5,0).
B) Minimum cost of $9.75 occurs with 0 pounds of food A and 5 pounds of food B.
C) Minimum cost of $8.45 occurs on the line between (2,2) and (5,0).
D) Minimum cost of $5.20 occurs with 2 pounds of food A and 2 pounds of food B.
E) Minimum cost of $12.35 occurs with 5 pounds of food A and 3 pounds of food B.
A) Minimum cost of $6.50 occurs on the line between (2,2) and (5,0).
B) Minimum cost of $9.75 occurs with 0 pounds of food A and 5 pounds of food B.
C) Minimum cost of $8.45 occurs on the line between (2,2) and (5,0).
D) Minimum cost of $5.20 occurs with 2 pounds of food A and 2 pounds of food B.
E) Minimum cost of $12.35 occurs with 5 pounds of food A and 3 pounds of food B.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
52
A sausage company makes two different kinds of hot dogs, regular and all beef. Each pound of all-beef hot dogs requires 0.75 lb of beef and 0.2 lb of spices, and each pound of regular hot dogs requires 0.18 lb of beef, 0.3 lb of pork, and 0.2 lb of spices. Suppliers can deliver at most 1,020 lb of beef, at most 600 lb of pork, and at least 500 lb of spices. If the profit is $0.70 on each pound of all-beef hot dogs and $0.40 on each pound of regular hot dogs, how many pounds of each should be produced to obtain maximum profit? What is the maximum profit? Round your profit to the nearest cent, another answers - to the nearest whole number.
A) Maximum profit of $1150.00 with 2000 lbs of regular and 500 lbs of all beef.
B) Maximum profit of $1416.00 with 2000 lbs of regular and 880 lbs of all beef.
C) Maximum profit of $1752.00 with 880 lbs of regular and 2000 lbs of all beef.
D) Maximum profit of $1300.00 with 1500 lbs of regular and 1000 lbs of all beef.
E) Maximum profit of $1450.00 with 1000 lbs of regular and 1500 lbs of all beef.
A) Maximum profit of $1150.00 with 2000 lbs of regular and 500 lbs of all beef.
B) Maximum profit of $1416.00 with 2000 lbs of regular and 880 lbs of all beef.
C) Maximum profit of $1752.00 with 880 lbs of regular and 2000 lbs of all beef.
D) Maximum profit of $1300.00 with 1500 lbs of regular and 1000 lbs of all beef.
E) Maximum profit of $1450.00 with 1000 lbs of regular and 1500 lbs of all beef.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
53
A cereal manufacturer makes two different kinds of cereal, Senior Citizen's Feast and Kids Go. Each pound of Senior Citizen's Feast requires 0.6 lb of wheat and 0.2 lb of vitamin-enriched syrup, and each pound of Kids Go requires 0.4 lb of wheat, 0.2 lb of sugar, and 0.2 lb of vitamin-enriched syrup. Suppliers can deliver at most 2,800 lb of wheat, at most 800 lb of sugar, and at least 1,000 lb of the vitamin-enriched syrup. If the profit is $0.80 on each pound of Senior Citizen's Feast and $1.00 on each pound of Kids Go, find the number of pounds of each cereal that should be produced to obtain maximum profit. Find the maximum profit. Round your profit to the nearest cent, another answers - to the nearest whole number.
A) Maximum profit of $4200.00 when 4000 lbs of Senior Citizen's Feast and 1000 lbs of Kids Go are produced.
B) Maximum profit of $4800.00 when 1000 lbs of Senior Citizen's Feast and 4000 lbs of Kids Go are produced.
C) Maximum profit of $5200.00 when 4000 lbs of Senior Citizen's Feast and 2000 lbs of Kids Go are produced.
D) Maximum profit of $6000.00 when 2000 lbs of Senior Citizen's Feast and 4000 lbs of Kids Go are produced.
E) Maximum profit of $5600.00 when 2000 lbs of Senior Citizen's Feast and 4000 lbs of Kids Go are produced.
A) Maximum profit of $4200.00 when 4000 lbs of Senior Citizen's Feast and 1000 lbs of Kids Go are produced.
B) Maximum profit of $4800.00 when 1000 lbs of Senior Citizen's Feast and 4000 lbs of Kids Go are produced.
C) Maximum profit of $5200.00 when 4000 lbs of Senior Citizen's Feast and 2000 lbs of Kids Go are produced.
D) Maximum profit of $6000.00 when 2000 lbs of Senior Citizen's Feast and 4000 lbs of Kids Go are produced.
E) Maximum profit of $5600.00 when 2000 lbs of Senior Citizen's Feast and 4000 lbs of Kids Go are produced.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
54
Convert the constraint inequalities 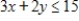 and
and 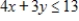 to equations containing slack variables.
to equations containing slack variables.
A)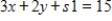 and
and 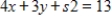
B)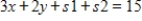 and
and 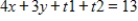
C)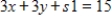 and
and 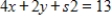
D)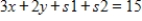 and
and 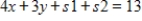
E)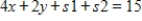 and
and 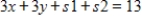
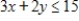 and
and 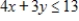 to equations containing slack variables.
to equations containing slack variables. A)
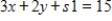 and
and 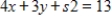
B)
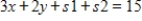 and
and 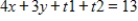
C)
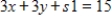 and
and 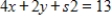
D)
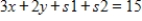 and
and 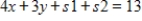
E)
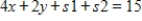 and
and 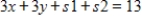

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
55
A simplex matrix for a standard maximization problem is given. Indicate whether or not the solution shown is complete (optimal). If the solution is not complete, find the next pivot or indicate that no solution exists. 
A) next pivot: -12 in R4C3
B) next pivot: -7 in R1C3
C) next pivot: 5 in R2C2
D) optimal solution
E) no solution

A) next pivot: -12 in R4C3
B) next pivot: -7 in R1C3
C) next pivot: 5 in R2C2
D) optimal solution
E) no solution

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
56
A simplex matrix is given. In this case the solution is complete, so identify the maximum value of f and a set of values of the variables that gives this maximum value. If multiple solutions may exist, indicate this and locate the next pivot. 
A) The maximum value of f is 160 when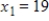 ,
, 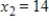 and
and 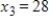 .
.
B) The maximum value of f is 160 when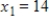 ,
, 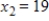 and
and 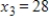 .
.
C) The maximum value of f is 160 when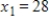 ,
, 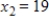 and
and 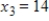 .
.
D) The maximum value of f is 160 when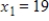 ,
, 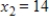 . Since multiple solutions are possible, pivot on 1 in R4C4.
. Since multiple solutions are possible, pivot on 1 in R4C4.
E) The maximum value of f is 160 when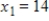 ,
, 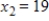 . Since multiple solutions are possible, pivot on 2 in R3C5.
. Since multiple solutions are possible, pivot on 2 in R3C5.

A) The maximum value of f is 160 when
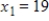 ,
, 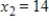 and
and 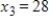 .
.B) The maximum value of f is 160 when
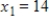 ,
, 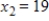 and
and 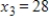 .
.C) The maximum value of f is 160 when
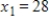 ,
, 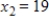 and
and 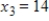 .
.D) The maximum value of f is 160 when
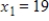 ,
, 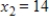 . Since multiple solutions are possible, pivot on 1 in R4C4.
. Since multiple solutions are possible, pivot on 1 in R4C4.E) The maximum value of f is 160 when
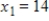 ,
, 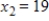 . Since multiple solutions are possible, pivot on 2 in R3C5.
. Since multiple solutions are possible, pivot on 2 in R3C5.
Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
57
A simplex matrix for a standard maximization problem is given. Indicate whether or not the solution shown is complete (optimal). If the solution is not complete, find the next pivot or indicate that no solution exists. 
A) next pivot: 4 in R3C1
B) next pivot: -3 in R4C1
C) next pivot: 2 in R1C1
D) optimal solution
E) no solution

A) next pivot: 4 in R3C1
B) next pivot: -3 in R4C1
C) next pivot: 2 in R1C1
D) optimal solution
E) no solution

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
58
Use the simplex method to maximize the given function. Assume all variables are nonnegative.
Maximize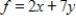 subject to
subject to
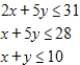
A) maximum: 31 at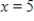 ,
, 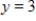
B) maximum: 41 at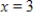 ,
, 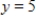
C) maximum: 27 at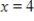 ,
, 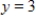
D) maximum: 32 at ,
, 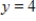
E) maximum: 59 at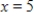 ,
, 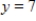
Maximize
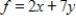 subject to
subject to
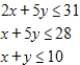
A) maximum: 31 at
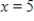 ,
, 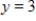
B) maximum: 41 at
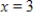 ,
, 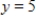
C) maximum: 27 at
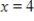 ,
, 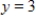
D) maximum: 32 at
 ,
, 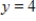
E) maximum: 59 at
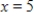 ,
, 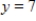

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
59
Set up the simplex matrix used to solve the linear programming problem. Assume all variables are nonnegative.
Maximize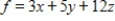 subject to
subject to
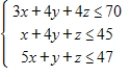
A) 

B) 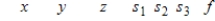

C) 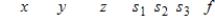

D) 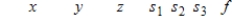

E) 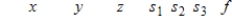

Maximize
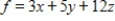 subject to
subject to
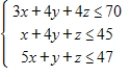
A)


B)

C)
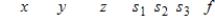

D)
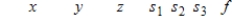

E)
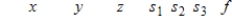


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
60
A farm co-op has 6,008 acres available to plant with corn and soybeans. Each acre of corn requires 9 gallons of fertilizer/herbicide and 0.75 hour of labor to harvest. Each acre of soybeans requires 3 gallons of fertilizer/herbicide and 1hour of labor to harvest. The co-op has available at most 40,500 gallons of fertilizer/herbicide and at most 5,250 hours of labor for harvesting. Find the maximum profit if the profits per acre are $70 for corn and $55 for soybeans. Round your answer to the nearest cent if necessary.
A) $386,630.00
B) $288,750.00
C) $375,080.00
D) $247,500.00
E) $394,169.00
A) $386,630.00
B) $288,750.00
C) $375,080.00
D) $247,500.00
E) $394,169.00

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
61
A woman has a building with 26 one-bedroom apartments, 40 two-bedroom apartments, and 60 three-bedroom apartments available to rent to students. She has set the rent at $550 per month for the one-bedroom units, $900 per month for the two-bedroom units, and $1,100 per month for the three-bedroom units. She must rent to one student per bedroom, and zoning laws limit her to at most 250 students in this building. There are enough students available to rent all the apartments. How many of each type of apartment should she rent to maximize her monthly revenue? Find the maximum possible monthly revenue. Round your profit to the nearest dollar.
A) Maximum revenue is $89,250 by renting 25 1 - BR, 35 2 - BR, and 40 3 - BR apartments.
B) Maximum revenue is $93,100 by renting 22 1 - BR, 35 2 - BR, and 45 3 - BR apartments.
C) Maximum revenue is $105,850 by renting 29 1 - BR, 40 2 - BR, and 49 3 - BR apartments.
D) Maximum revenue is $98,300 by renting 30 1 - BR, 42 2 - BR, and 40 3 - BR apartments.
E) Maximum revenue is $103,100 by renting 26 1 - BR, 40 2 - BR, and 48 3 - BR apartments.
A) Maximum revenue is $89,250 by renting 25 1 - BR, 35 2 - BR, and 40 3 - BR apartments.
B) Maximum revenue is $93,100 by renting 22 1 - BR, 35 2 - BR, and 45 3 - BR apartments.
C) Maximum revenue is $105,850 by renting 29 1 - BR, 40 2 - BR, and 49 3 - BR apartments.
D) Maximum revenue is $98,300 by renting 30 1 - BR, 42 2 - BR, and 40 3 - BR apartments.
E) Maximum revenue is $103,100 by renting 26 1 - BR, 40 2 - BR, and 48 3 - BR apartments.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
62
Patio Iron makes wrought iron outdoor dining tables, chairs, and stools. Each table uses 8 ft of a standard-width wrought iron, 2 hours of labor for cutting and assembly, and 2 hours of labor for detail and finishing work. Each chair uses 6 ft of the wrought iron, 2 hours of cutting and assembly labor, and 1.5 hours of detail and finishing labor. Each stool uses 1 ft of the wrought iron, 1.5 hours for cutting and assembly, and 0.5 hour for detail and finishing work, and the daily demand for stools is at most 16. Each day Patio Iron has available at most 108 ft of wrought iron, 50 hours for cutting and assembly, and 40 hours for detail and finishing. If the profits are $60 for each dining table, $49 for each chair, and $39 for each stool, how many of each item should be made each day to maximize profit? Find the maximum profit. Round your profit to the nearest dollar and another answers - to the nearest whole number.
A) 7 tables, 6 chairs, and 16 stools per day for a maximum daily profit of $1,338
B) 6 tables, 5 chairs, and 18 stools per day for a maximum daily profit of $1,307
C) 5 tables, 4 chairs, and 18 stools per day for a maximum daily profit of $1,198
D) 8 tables, 5 chairs, and 16 stools per day for a maximum daily profit of $1,349
E) 8 tables, 6 chairs, and 15 stools per day for a maximum daily profit of $1,359
A) 7 tables, 6 chairs, and 16 stools per day for a maximum daily profit of $1,338
B) 6 tables, 5 chairs, and 18 stools per day for a maximum daily profit of $1,307
C) 5 tables, 4 chairs, and 18 stools per day for a maximum daily profit of $1,198
D) 8 tables, 5 chairs, and 16 stools per day for a maximum daily profit of $1,349
E) 8 tables, 6 chairs, and 15 stools per day for a maximum daily profit of $1,359

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
63
A car rental agency has a budget of $1.8 million to purchase at most 100 new cars. The agency will purchase either compact cars at $15,000 each or luxury cars at $30,000 each. From past rental patterns, the agency decides to purchase at most 50 luxury cars and expects an annual profit of $8,000 per compact car and $13,500 per luxury car. How many of each type of car should be purchased in order to obtain the maximum profit while satisfying budgetary and other planning constraints? Find the maximum profit. Round your profit to the nearest dollar and another answers - to the nearest whole number.
A) 50 compact cars and 40 luxury cars for a maximum profit of $940,000
B) 70 compact cars and 50 luxury cars for a maximum profit of $1,235,000
C) 80 compact cars and 20 luxury cars for a maximum profit of $910,000
D) 75 compact cars and 30 luxury cars for a maximum profit of $1,005,000
E) 60 compact cars and 45 luxury cars for a maximum profit of $1,087,500
A) 50 compact cars and 40 luxury cars for a maximum profit of $940,000
B) 70 compact cars and 50 luxury cars for a maximum profit of $1,235,000
C) 80 compact cars and 20 luxury cars for a maximum profit of $910,000
D) 75 compact cars and 30 luxury cars for a maximum profit of $1,005,000
E) 60 compact cars and 45 luxury cars for a maximum profit of $1,087,500

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
64
A medical clinic performs three types of medical tests that use the same machines. Tests A, B, and C take 15 minutes, 30 minutes, and 1 hour, respectively, with respective profits of $90, $120 and $150. The clinic has 4 machines available. One person is qualified to do test A, two to do test B, and one to do test C. If the clinic has a rush of customers for these tests, how many of each type should it schedule in a 9 - hour day to maximize its profit? Round your profit to the nearest dollar and another answers - to the nearest whole number.
A) 36 A tests; 36 B tests; 36 C tests; maximum profit $12,960
B) 36 A tests; 36 B tests; 18 C tests; maximum profit $12,960
C) 36 A tests; 36 B tests; 9 C tests; maximum profit $8,910
D) 36 A tests; 18 B tests; 9 C tests; maximum profit $6,750
E) 18 A tests; 18 B tests; 9 C tests; maximum profit $5,130
A) 36 A tests; 36 B tests; 36 C tests; maximum profit $12,960
B) 36 A tests; 36 B tests; 18 C tests; maximum profit $12,960
C) 36 A tests; 36 B tests; 9 C tests; maximum profit $8,910
D) 36 A tests; 18 B tests; 9 C tests; maximum profit $6,750
E) 18 A tests; 18 B tests; 9 C tests; maximum profit $5,130

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
65
Suppose a primal minimization problem and its dual maximization problem were solved by using the simplex method on the dual problem. The final simplex matrix is given. Find the solution of the maximization problem. Use x1, x2, x3 as the variables and f as the function. 
A)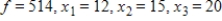
B)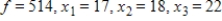
C)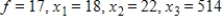
D)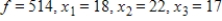
E)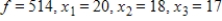

A)
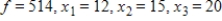
B)
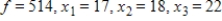
C)
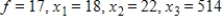
D)
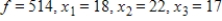
E)
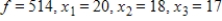

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
66
Find the transpose of the matrix associated with the given minimization problem. y
Minimize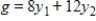 subject to
subject to
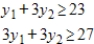
A)
B)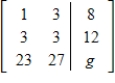
C)
D)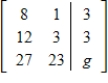
E)
Minimize
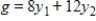 subject to
subject to
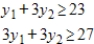
A)

B)
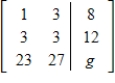
C)

D)
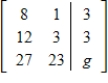
E)


Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
67
Write the dual maximization problem. Be sure to rename the variables.
Minimize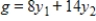 subject to
subject to
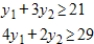
A) Maximize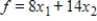
Constraints: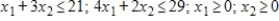
B) Maximize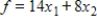
Constraints: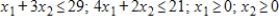
C) Maximize
Constraints:
D) Maximize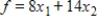
Constraints: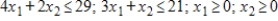
E) Maximize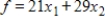
Constraints: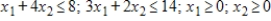
Minimize
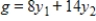 subject to
subject to
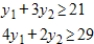
A) Maximize
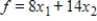
Constraints:
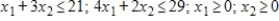
B) Maximize
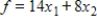
Constraints:
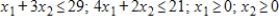
C) Maximize

Constraints:

D) Maximize
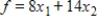
Constraints:
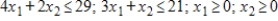
E) Maximize
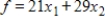
Constraints:
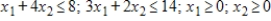

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
68
The simplex matrix shown below indicates that an optimal solution has been found ( 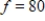 when
when 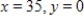 ) but that a second solution is possible. Find the second solution.
) but that a second solution is possible. Find the second solution. 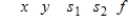

A) The maximum is 80 at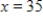 ,
, 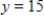
B) The maximum is 80 at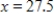 ,
, 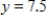
C) The maximum is 80 at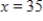 ,
, 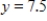
D) The maximum is 80 at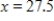 ,
, 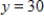
E) The maximum is 80 at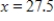 ,
, 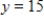
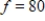 when
when 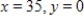 ) but that a second solution is possible. Find the second solution.
) but that a second solution is possible. Find the second solution. 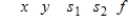

A) The maximum is 80 at
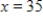 ,
, 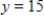
B) The maximum is 80 at
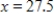 ,
, 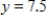
C) The maximum is 80 at
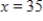 ,
, 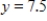
D) The maximum is 80 at
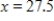 ,
, 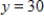
E) The maximum is 80 at
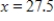 ,
, 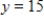

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
69
At one of its factories, a jeans manufacturer makes two styles: #891 and #917. Each pair of style-891 takes 10 minutes to cut out and 20 minutes to assemble and finish. Each pair of style-917 takes 10 minutes to cut out and 30 minutes to assemble and finish. The plant has enough workers to provide at most 7,500 minutes per day for cutting and at most 19,500 minutes per day for assembly and finishing. The profit on each pair of style-891 is $6.00 and the profit on each pair of style-917 is $8.50. How many pairs of each style should be produced per day to obtain maximum profit? Find the maximum daily profit. Round your profit to the nearest cent and anothet answers - to the nearest whole number.
A) 350 pairs of style #891 and 400 pairs of style #917 for maximum profit of $5,500.00
B) 300 pairs of style #891 and 450 pairs of style #917 for maximum profit of $5,625.00
C) 299 pairs of style #891 and 399 pairs of style #917 for maximum profit of $5,185.50
D) 225 pairs of style #891 and 375 pairs of style #917 for maximum profit of $4,537.50
E) 310 pairs of style #891 and 300 pairs of style #917 for maximum profit of $4,410.00
A) 350 pairs of style #891 and 400 pairs of style #917 for maximum profit of $5,500.00
B) 300 pairs of style #891 and 450 pairs of style #917 for maximum profit of $5,625.00
C) 299 pairs of style #891 and 399 pairs of style #917 for maximum profit of $5,185.50
D) 225 pairs of style #891 and 375 pairs of style #917 for maximum profit of $4,537.50
E) 310 pairs of style #891 and 300 pairs of style #917 for maximum profit of $4,410.00

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
70
Use the simplex method to maximize the function (if possible) subject to the given constraints. If there is no solution, indicate this; if multiple solutions exist, give one solution.
Maximize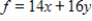 subject to
subject to
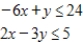
A) maximum: 384 at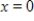 ,
, 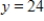
B) maximum: 336 at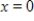 ,
, 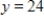
C) maximum: 224 at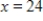 ,
, 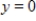
D) maximum: 336 at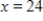 ,
, 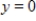
E) no solution
Maximize
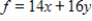 subject to
subject to
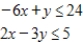
A) maximum: 384 at
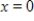 ,
, 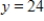
B) maximum: 336 at
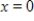 ,
, 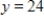
C) maximum: 224 at
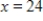 ,
, 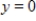
D) maximum: 336 at
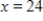 ,
, 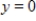
E) no solution

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
71
Use the simplex method to maximize the given function. Assume all variables are nonnegative.
Maximize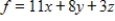 subject to
subject to
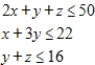
A) maximum: 132 at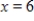 ,
, 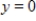 ,
, 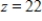
B) maximum: 252 at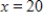 ,
, 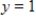 ,
, 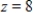
C) maximum: 292 at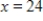 ,
, 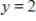 ,
, 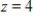
D) maximum: 92 at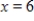 ,
, 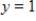 ,
, 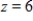
E) maximum: 260 at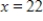 ,
, 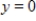 ,
, 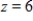
Maximize
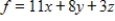 subject to
subject to
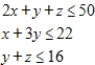
A) maximum: 132 at
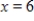 ,
, 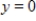 ,
, 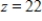
B) maximum: 252 at
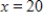 ,
, 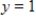 ,
, 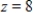
C) maximum: 292 at
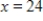 ,
, 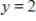 ,
, 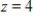
D) maximum: 92 at
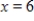 ,
, 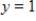 ,
, 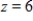
E) maximum: 260 at
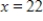 ,
, 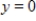 ,
, 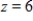

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
72
Tire Corral has $6,000 available per month for advertising. Newspaper ads cost $100 each and can occur a maximum of 21 times per month. Radio ads cost $300 each and can occur a maximum of 28 times per month at this price. Each newspaper ad reaches 6,750 men over 20 years of age, and each radio ad reaches 8,500 of these men. The company wants to maximize the number of ad exposures to this group. How many of each ad should it purchase? What is the maximum possible number of exposures? Round your answers to the nearest whole number if necessary.
A) 21 newspaper ads and 28 radio ads for maximum exposure of 379,750 people
B) 28 newspaper ads and 21 radio ads for maximum exposure of 367,500 people
C) 13 newspaper ads and 21 radio ads for maximum exposure of 266,250 people
D) 21 newspaper ads and 13 radio ads for maximum exposure of 252,250 people
E) 20 newspaper ads and 15 radio ads for maximum exposure of 262,500 people
A) 21 newspaper ads and 28 radio ads for maximum exposure of 379,750 people
B) 28 newspaper ads and 21 radio ads for maximum exposure of 367,500 people
C) 13 newspaper ads and 21 radio ads for maximum exposure of 266,250 people
D) 21 newspaper ads and 13 radio ads for maximum exposure of 252,250 people
E) 20 newspaper ads and 15 radio ads for maximum exposure of 262,500 people

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
73
Use the simplex method to maximize the function (if possible) subject to the given constraints. If there is no solution, indicate this; if multiple solutions exist, give one solution.
Maximize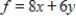 subject to
subject to
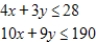
A) maximum: 56 at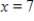 ,
, 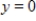
B) maximum: 100 at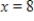 ,
, 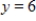
C) maximum: 84 at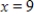 ,
, 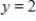
D) maximum: 70 at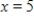 ,
, 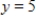
E) no solution
Maximize
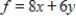 subject to
subject to
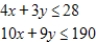
A) maximum: 56 at
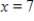 ,
, 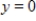
B) maximum: 100 at
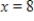 ,
, 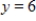
C) maximum: 84 at
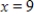 ,
, 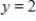
D) maximum: 70 at
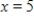 ,
, 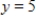
E) no solution

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
74
An ice cream company is planning its production for next week. Demand for premium and light ice creams continues to outpace the company's production capacities. Two resources used in ice cream production are in short supply for next week. The mixing machine will be available for only 150 hours, and only 30000 gallons of high grade milk will be available. One hundred gallons of premium ice-cream requires 0.6 hour of mixing and 180 gallons of milk. One hundred gallons of light ice cream requires 1 hour of mixing and 140 gallons of milk. If company earns a profit of $100 per hundred gallons on both of its ice creams, how many gallons of premium and of light ice cream should company produce next week to maximize profit? How much profit will result? Round your profit to the nearest cent and another answers - to the nearest whole number.
A) The company should produce 94 gallons of premium and 94 gallons of light ice cream to maximize the profit. The resultant maximum profit is $15,000.00 .
B) The company should produce 94 gallons of premium and 94 gallons of light ice cream to maximize the profit. The resultant maximum profit is $18,750.00.
C) The company should produce 94 gallons of premium and 94 gallons of light ice cream to maximize the profit. The resultant maximum profit is $16,666.67.
D) The company should produce 69 gallons of premium and 69 gallons of light ice cream to maximize the profit. The resultant maximum profit is $13,800.00.
E) The company should produce 119 gallons of premium and 119 gallons of light ice cream to maximize the profit. The resultant maximum profit is $23,800.00.
A) The company should produce 94 gallons of premium and 94 gallons of light ice cream to maximize the profit. The resultant maximum profit is $15,000.00 .
B) The company should produce 94 gallons of premium and 94 gallons of light ice cream to maximize the profit. The resultant maximum profit is $18,750.00.
C) The company should produce 94 gallons of premium and 94 gallons of light ice cream to maximize the profit. The resultant maximum profit is $16,666.67.
D) The company should produce 69 gallons of premium and 69 gallons of light ice cream to maximize the profit. The resultant maximum profit is $13,800.00.
E) The company should produce 119 gallons of premium and 119 gallons of light ice cream to maximize the profit. The resultant maximum profit is $23,800.00.

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
75
Use the simplex method to maximize the given function. Assume all variables are nonnegative.
Maximize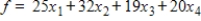 subject to
subject to
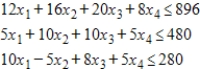
A) maximum: 1,496 at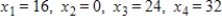
B) maximum: 985 at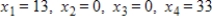
C) maximum: 1,888 at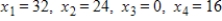
D) maximum: 2,435 at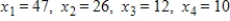
E) maximum: 2,045 at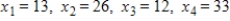
Maximize
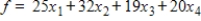 subject to
subject to
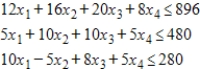
A) maximum: 1,496 at
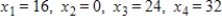
B) maximum: 985 at
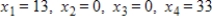
C) maximum: 1,888 at
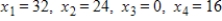
D) maximum: 2,435 at
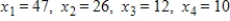
E) maximum: 2,045 at
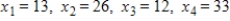

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
76
Solve both the primal and dual problems with the simplex method. Use f to represent the dual objective function.
Minimize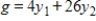 subject to
subject to
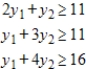
A) Primal: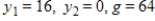
Dual: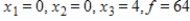
B) Primal: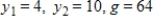
Dual: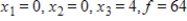
C) Primal: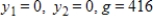
Dual: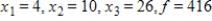
D) Primal: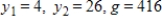
Dual: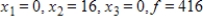
E) Primal: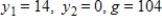
Dual: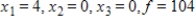
Minimize
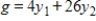 subject to
subject to
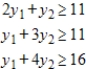
A) Primal:
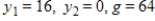
Dual:
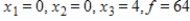
B) Primal:
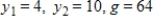
Dual:
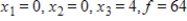
C) Primal:
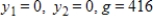
Dual:
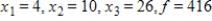
D) Primal:
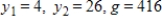
Dual:
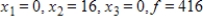
E) Primal:
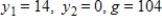
Dual:
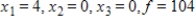

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
77
Suppose a primal minimization problem and its dual maximization problem were solved by using the simplex method on the dual problem. The final simplex matrix is given. Find the solution of the minimization problem. Use y1, y2, y3 as the variables and g as the function. 
A)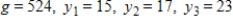
B)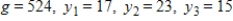
C)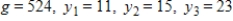
D)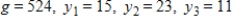
E)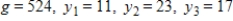

A)
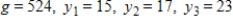
B)
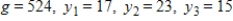
C)
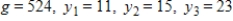
D)
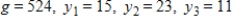
E)
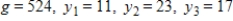

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
78
Form the matrix associated with the given minimization problem and find its transpose.
Minimize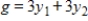 subject to
subject to
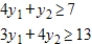
A)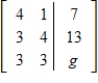
B)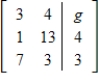
C)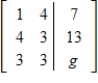
D)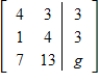
E)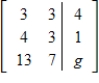
Minimize
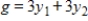 subject to
subject to
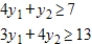
A)
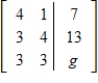
B)
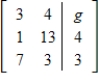
C)
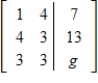
D)
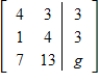
E)
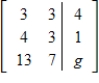

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
79
Write the dual maximization problem. Be sure to rename the variables.
Minimize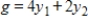 subject to
subject to
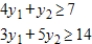
A) Maximize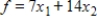
Constraints: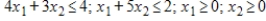
B) Maximize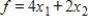
Constraints: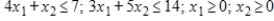
C) Maximize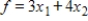
Constraints: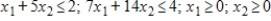
D) Maximize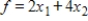
Constraints: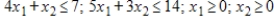
E) Maximize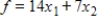
Constraints: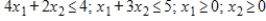
Minimize
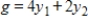 subject to
subject to
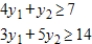
A) Maximize
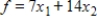
Constraints:
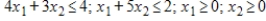
B) Maximize
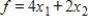
Constraints:
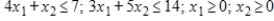
C) Maximize
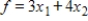
Constraints:
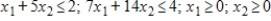
D) Maximize
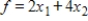
Constraints:
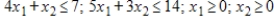
E) Maximize
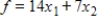
Constraints:
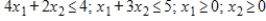

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck
80
Solve the following minimization problem by solving the primal problem with the simplex method.
Minimize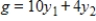 subject to
subject to
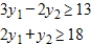
A) Minimum: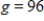 at
at 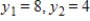
B) Minimum: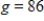 at
at 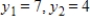
C) Minimum: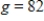 at
at 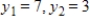
D) Minimum: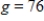 at
at 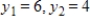
E) Minimum: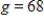 at
at 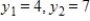
Minimize
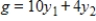 subject to
subject to
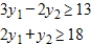
A) Minimum:
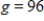 at
at 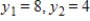
B) Minimum:
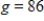 at
at 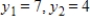
C) Minimum:
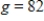 at
at 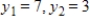
D) Minimum:
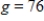 at
at 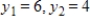
E) Minimum:
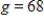 at
at 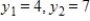

Unlock Deck
Unlock for access to all 119 flashcards in this deck.
Unlock Deck
k this deck



