Deck 5: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/24
Play
Full screen (f)
Deck 5: Exponential and Logarithmic Functions
1
Write the expression as a single logarithm with a coefficient of 1. 2log 10 x - 5log 10 y
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Simplify the expression.
A) 1
B) 2
C) - 2
D)
E)
A) 1
B) 2
C) - 2
D)
E)
2
3
Write in exponential form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Simplify the expression by using the definition and properties of logarithms. log 10 9,000 - log 10 9
A) 8,991
B) 1
C) 9
D) 3
E) 1,000
A) 8,991
B) 1
C) 9
D) 3
E) 1,000

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
5
Find all the real-number roots of the equation. Give an exact expression for the root and also(where appropriate) a calculator approximation rounded to three decimal places.
A)
B)
C)
D)
E) no solution
A)
B)
C)
D)
E) no solution

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
6
Find all the real-number roots of the equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
7
A bank offers an interest rate of 4% per annum compounded daily(assuming 365-day year). What is the effective rate?
A) 2.88%
B) 4.01%
C) 4.28%
D) 3.04%
E) 4.08%
A) 2.88%
B) 4.01%
C) 4.28%
D) 3.04%
E) 4.08%

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
8
The functions and are inverse functions. Find the
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
9
Find all the real-number roots of the equation. Give an exact expression for the root and also(where appropriate) a calculator approximation rounded to three decimal places.
A)
B)
C)
D)
E) no solution
A)
B)
C)
D)
E) no solution

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
10
Solve the equation.
A) x = 7
B) x = 5
C) x = 8
D) x = 20
E) no solution
A) x = 7
B) x = 5
C) x = 8
D) x = 20
E) no solution

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
11
Graph the pair of functions on the same set of axes. y = 2 x ; y = - 2 x 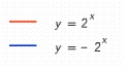
A)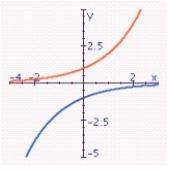
B)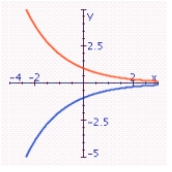
C)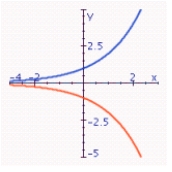
D)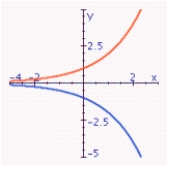
E)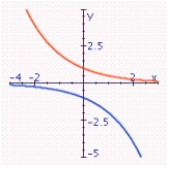
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
12
Is the following statement true? (No calculators allowed)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
13
Solve the equation. Express the answer in terms of natural logarithms.
5 = 3e 3x - 2
A)
B)
C)
D)
E)
5 = 3e 3x - 2
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
14
You invest $200 at 7% per annum compounded quarterly. How long will it take for your balance to exceed $300? (Round your answer up to the next quarter.)
A) 6 quarters
B) 18 quarters
C) 24 quarters
D) 23 quarters
E) 13 quarters
A) 6 quarters
B) 18 quarters
C) 24 quarters
D) 23 quarters
E) 13 quarters

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
15
Is the following statement true? (No calculators allowed) e<

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
16
Complete the table.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
17
Suppose that during the first hour and 15 minutes of a physics experiment, the surface temperature of a small iron block is modeled by the exponential function f(t) = 15e t , where f(t) is the Celsius temperature t hours after the experiment begins. Compute the average rate of change of temperature over the second half hour of the experiment (i.e., over the interval ). Round the answer to one decimal place.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
18
Use properties of exponents to simplify.
A) 49
B) 81
C) 16
D)
E) 343
A) 49
B) 81
C) 16
D)
E) 343

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
19
Estimate 4 40 in terms of powers of ten.
A) 10 22
B) 10 27
C) 10 24
D) 10 19
A) 10 22
B) 10 27
C) 10 24
D) 10 19

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
20
Solve the inequality.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
21
The radioactive isotope carbone-14 is used as a tracer in medical and biological research. Compute the half-life of carbone-14 given that the decay constant k is (The units for k here are such that your half-life answer will be in years.) Please round the answer to one decimal place.
A) 5,728.7years
B) 5,729.2years
C) 5,758.5years
D) 5,730.3years
E) 5,729.9years
A) 5,728.7years
B) 5,729.2years
C) 5,758.5years
D) 5,730.3years
E) 5,729.9years

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
22
You place a sum of $600 in a savings account at 4% per annum compounded continuously. When will the balance reach $800?
A) in 7.23 years
B) in 5.99 years
C) in 15.59 years
D) in 7.19 years
E) in 4.6 years
A) in 7.23 years
B) in 5.99 years
C) in 15.59 years
D) in 7.19 years
E) in 4.6 years

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
23
The half-life of radium-226 is 1620 years. How much of an initial 2-g sample remains after 500 years? Please round the answer to two decimal places.
A) 0.81 grams
B) 1.54 grams
C) 1.61 grams
D) 1.63 grams
E) 1.65 grams
A) 0.81 grams
B) 1.54 grams
C) 1.61 grams
D) 1.63 grams
E) 1.65 grams

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
24
In 2000, the nations of Niger and Portugal had similar size populations: Niger 10.1 million, Portugal 10.0 million. However, the relative growth rate for Niger was 3.0%/year, whereas that for Portugal was only 0.1%/year. Assuming exponential growth at the given rates, make projections for each population in the year 2055. Please round the answers to one decimal place.
A) Niger: 10.6 million; Portugal: 52.6 million
B) Niger: 53.8 million; Portugal: 11.0 million
C) Niger: 52.6 million; Portugal: 10.6 million
D) Niger: 54.4 million; Portugal: 11.6 million
E) Niger: 54.0 million; Portugal: 11.1 million
A) Niger: 10.6 million; Portugal: 52.6 million
B) Niger: 53.8 million; Portugal: 11.0 million
C) Niger: 52.6 million; Portugal: 10.6 million
D) Niger: 54.4 million; Portugal: 11.6 million
E) Niger: 54.0 million; Portugal: 11.1 million

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck



