Deck 7: Section 2: Systems of Linear Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/15
Play
Full screen (f)
Deck 7: Section 2: Systems of Linear Equations
1
Solve the system of equations.
3x - 2y + 4z = 12
-5x - y - z = -10
-3x - 5y - 3z = -4
3x - 2y + 4z = 12
-5x - y - z = -10
-3x - 5y - 3z = -4
{(2, -1, 1)}
2
Solve by graphing. Label each line and the solution point. 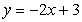
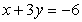
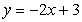
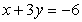
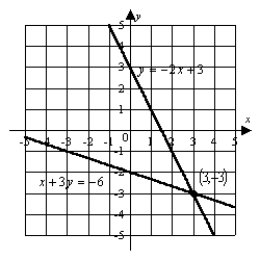
3
Solve the system of linear equations by the substitution method. 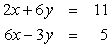
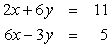
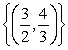
4
Fred, Tiger, and Nancy play a round of golf together. Their combined score is 226. Fred's score was 5 more than Tiger's, and Nancy's score was 9 more than Fred's. What was each person's score?

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
5
Solve the system of linear equations. 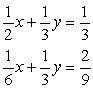

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
6
When rolling a pair of dice, the probability of getting a particular total is not very high. For example, the probability of failing to roll a total of 3 is 17 times the probability of successfully rolling a total of 3. The total probability (probability of failing to roll a 3 + probability of successfully rolling a 3) is 1. What is the probability of rolling a 3?

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
7
Solve the system of equations. 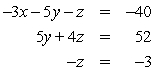

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
8
Cashews sell for $5 per pound and peanuts sell for $2 per pound. How many pounds of each would you use to make 15 pounds of a mixture that sells for $3.20 per pound?

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
9
Solve the system of linear equations by the substitution method.
-5x = -2y + 9
5x + 5y = -30
-5x = -2y + 9
5x + 5y = -30

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
10
Suzanne invested $14,300 in two mutual fund accounts: the Abernathy Fund and the Brice Fund. During the subsequent year, The Abernathy Fund earned 4% and the Brice account earned 5%. At the end of the year, the total interest earned was $672. How much did Suzanne invest in each fund?

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
11
Solve the system of equations.
-3.5x + 3y = -5.375
-0.875x - 0.5y = 0.53125
-3.5x + 3y = -5.375
-0.875x - 0.5y = 0.53125

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
12
For Monday morning's staff meeting, Jim bought 2 bags of bagels and 3 packages of cream cheese and paid $9.00 (excluding sales tax). For Friday's meeting, he bought 4 bags of bagels and 2 packages of cream cheese and paid $10.00 (again, excluding sales tax). How much do bags of bagels and packages of cream cheese cost?

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
13
For the system find the value of a so that the solution set to the system is {(4, 7)}. 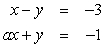
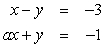

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
14
Solve the system of equations.
-x + 5y - 3z = 31
-4x - 5y + 4z = 6
3x - 15y + 9z = -91
-x + 5y - 3z = 31
-4x - 5y + 4z = 6
3x - 15y + 9z = -91

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
15
Solve the system of linear equations by the substitution method. Then, state whether the system is independent, dependent, or inconsistent.
4x - 3y = 4
12x - 9y = 12
4x - 3y = 4
12x - 9y = 12

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck



