Deck 8: Linear Programming
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/44
Play
Full screen (f)
Deck 8: Linear Programming
1
Sketch the region described by the inequalities. 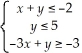
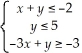

-3x + y = -3 
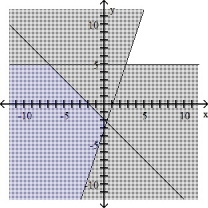




2
x - y > -3 
A)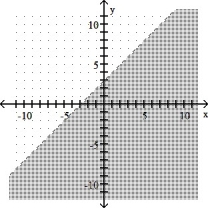
B)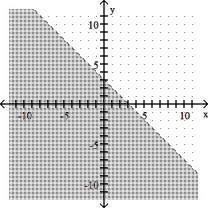
C)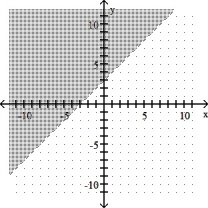
D)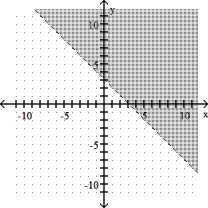

A)

B)

C)

D)


3
Maximize
Z = 5x - 3y
subject to
2x - y ≤ 8
2x - 5y ≥ 0
x - y = -2
x,y ≥ 0.
Z = 5x - 3y
subject to
2x - y ≤ 8
2x - 5y ≥ 0
x - y = -2
x,y ≥ 0.
no optimum solution (empty feasible region)
4
To make some extra money,you make two types of picture frames,type A and type B,for sale.You have an initial start-up expense of $75.The production cost for type A is $3.60 per frame,and the production cost for type B is $5.20 per frame.The price for type A is $6.00 per frame and the price for type B is $10.00 per frame.Let x be the number of type A and y be the number of type B produced and sold.Write an inequality describing revenue less than cost.Solve the inequality and describe the region.Also,describe what this means in terms of frames.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
5
A car rental company has $540,000 to purchase up to 25 new cars of two different models.One model costs $18,000 each and the other model costs $24,000 each.Write a system of linear inequalities to describe the situation.Let x represent the first model and y represent the second.Find the region described by the system of linear inequalities.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
6
Sketch the region described by the inequalities. 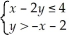
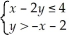


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
7
4x + y > -2 
A)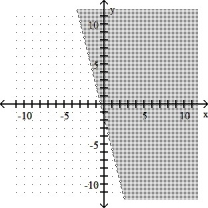
B)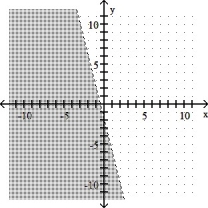
C)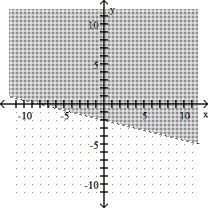
D)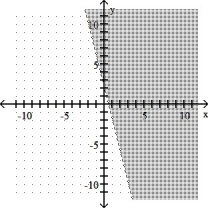

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
8
The XYZ Corporation produces two models of home computers,the Alpha model and the Beta model.Let x be the number of Alpha models and y the number of Beta models produced at the San Antonio factory per week.If the factory can produce at the most 100 Beta models in a week,write an inequality to describe this situation.Describe the region for the inequality.What additional inequalities can you add to the situation?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
9
The region indicated in the diagram 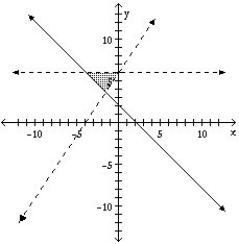 is described by
is described by
A)
B)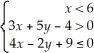
C)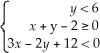
D)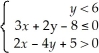
E)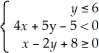
 is described by
is described byA)

B)
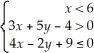
C)
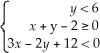
D)
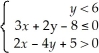
E)
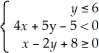

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
10
Sketch the region described by the inequalities 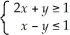


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
11
4x - 2y ≤ 4 
A)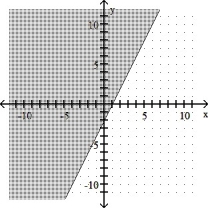
B)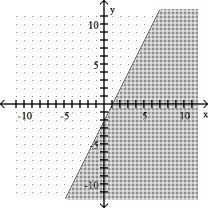
C)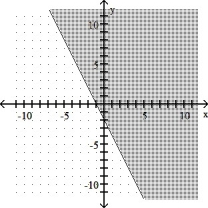
D)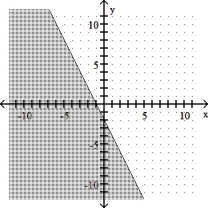

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
12
Maximize
Z = 4x + y
subject to
-x + y ≤ 2
3x + y ≤ 18
x,y ≥ 0.
Z = 4x + y
subject to
-x + y ≤ 2
3x + y ≤ 18
x,y ≥ 0.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
13
A chair company produces two models of chairs,the Sequoia and the Saratoga.The Sequoia model takes 3 hours to assemble and 
hour to paint.The Saratoga model takes 2 hours to assemble and 1 hour to paint.The maximum number of hours available to assemble is 24 per day and the maximum number of hours available to paint is 8 per day.
(a)If the company earns a profit of $20 per Sequoia model and $30 per Saratoga model,find the number of models produced per day in order to maximize profit.
(b)If the company earns a profit of $30 per Sequoia model and $15 per Saratoga model,find the number of models produced per day in order to maximize profit.
(c)Suppose the company decides to upgrade the two models so it takes an additional 2 hours to detail the Sequoia and 2 hours to detail the Saratoga.The maximum number of hours available to detail is 18 per day.If the company earns a profit of $45 per Sequoia model and $35 per Saratoga model,find the number of models produced per day in order to maximize profit.
(d)Suppose the company decides to upgrade the two models so it takes an additional 2 hours to detail the Sequoia and 2 hours to detail the Saratoga.The maximum number of hours available to detail is 18 per day.If the company earns a profit of $30 per Sequoia model and $40 per Saratoga model,find the number of models produced per day in order to maximize profit.

hour to paint.The Saratoga model takes 2 hours to assemble and 1 hour to paint.The maximum number of hours available to assemble is 24 per day and the maximum number of hours available to paint is 8 per day.
(a)If the company earns a profit of $20 per Sequoia model and $30 per Saratoga model,find the number of models produced per day in order to maximize profit.
(b)If the company earns a profit of $30 per Sequoia model and $15 per Saratoga model,find the number of models produced per day in order to maximize profit.
(c)Suppose the company decides to upgrade the two models so it takes an additional 2 hours to detail the Sequoia and 2 hours to detail the Saratoga.The maximum number of hours available to detail is 18 per day.If the company earns a profit of $45 per Sequoia model and $35 per Saratoga model,find the number of models produced per day in order to maximize profit.
(d)Suppose the company decides to upgrade the two models so it takes an additional 2 hours to detail the Sequoia and 2 hours to detail the Saratoga.The maximum number of hours available to detail is 18 per day.If the company earns a profit of $30 per Sequoia model and $40 per Saratoga model,find the number of models produced per day in order to maximize profit.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
14
The region indicated in the diagram 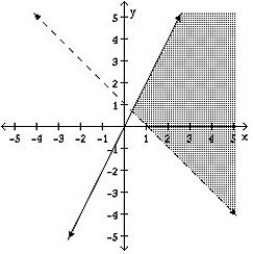 is described by
is described by
A)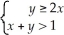
B)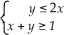
C)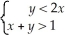
D)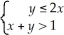
E)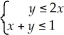
 is described by
is described byA)
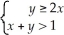
B)
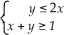
C)
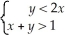
D)
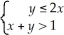
E)

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
15
Maximize
Z = 2x - 3y
subject to
2x + y ≥ 1
x - y ≤ 1
x,y ≥ 0.
Also find the corner point where the value of z is attained.
Z = 2x - 3y
subject to
2x + y ≥ 1
x - y ≤ 1
x,y ≥ 0.
Also find the corner point where the value of z is attained.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
16
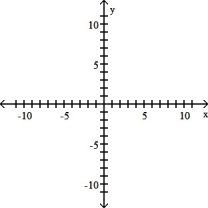
Sketch the region described by the following system of inequalities: 



Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
17
Sketch the region described by the inequalities. 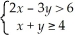


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
18
A store sells two types of calculators.In order to cover overhead,it must sell at least 40 calculators total per week,and in order to satisfy distribution requirements,it must sell at least twice as many of type II as type I.Write a system of inequalities to describe the situation.Let x be the number of type I that it sells in a week and y be the number of type II that it sells in a week.Find the region described by the system of linear inequalities.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
19
2x - 3y ≥ 6 
A)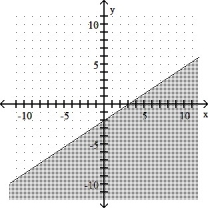
B)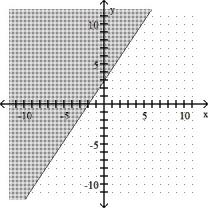
C)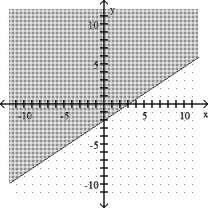
D)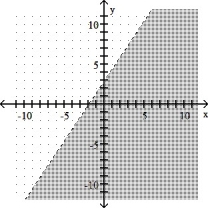

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
20
x + y ≤ -2 
A)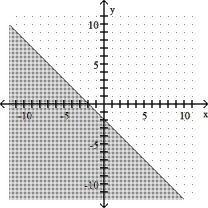
B)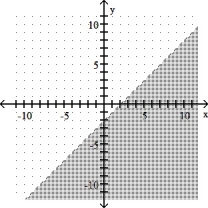
C)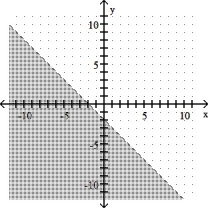
D)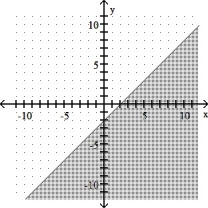

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
21
Maximize
Z =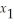
- 2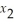
+ 3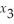
Subject to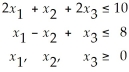
A) 0
B) 5
C) 10
D) 15
E) 20
Z =
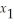
- 2
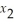
+ 3
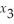
Subject to
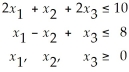
A) 0
B) 5
C) 10
D) 15
E) 20

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
22
When no quotients exist in a simplex table,
A) a degenerate BFS will occur.
B) the problem has an unbounded solution.
C) there is a possibility for multiple optimum solutions.
A) a degenerate BFS will occur.
B) the problem has an unbounded solution.
C) there is a possibility for multiple optimum solutions.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
23
Use the simplex method to minimize
Z = 3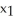
+ 2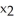
Subject to
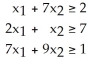
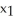
,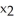
≥ 0
A) Z =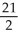 when
when  =
=  ,
,  = 0
= 0
B) Z = 21 when = 7,
= 7, 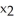 = 0
= 0
C) Z = 14 when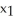 = 0,
= 0, 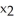 = 7
= 7
D) Z = 14 when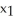 = 7,
= 7,  = 0
= 0
Z = 3
+ 2
Subject to

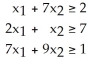
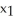
,
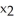
≥ 0
A) Z =
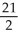 when
when 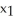 =
=  ,
, 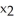 = 0
= 0B) Z = 21 when
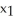 = 7,
= 7, 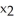 = 0
= 0C) Z = 14 when
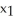 = 0,
= 0, 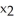 = 7
= 7D) Z = 14 when
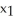 = 7,
= 7, 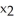 = 0
= 0
Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
24
Maximize
Z = 10x + 15y
subject to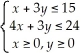
Z = 10x + 15y
subject to
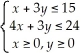

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
25
A manufacturer produces two products,product A and product B.Both products require processing on Machines I and II.The number of hours needed to produce one unit is given by the following chart: 
Machine I is available for at most 1150 hours and Machine II is available for at most 1100 hours.If the profit made on product A is $15 / unit and the profit made on product B is $30 / unit.Find the production level that will maximize profit and find the Maximum profit.

Machine I is available for at most 1150 hours and Machine II is available for at most 1100 hours.If the profit made on product A is $15 / unit and the profit made on product B is $30 / unit.Find the production level that will maximize profit and find the Maximum profit.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
26
A person decides to take two different dietary supplements.Each supplement contains two essential ingredients,A and B,for which there are minimum daily requirements,and each contains a third ingredient,C,which needs to be minimized.Find the amount of each supplement that the person should take each day in order to satisfy the requirements for A and B while minimizing C.Also find the amount of C the person takes each day. 


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
27
Use the simplex method to
maximize
Z =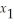
+ 4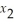
+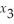
subject to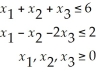
.
maximize
Z =
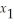
+ 4
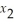
+
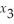
subject to
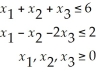
.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
28
A summer camp wants to hire counselors and aides to fill its staffing needs at minimum cost.The average monthly salary of a counselor is $2400 and the average monthly salary of an aide is $1100.The camp can accommodate up to 45 staff members and needs at least 30 to run properly.They must have at least 10 aides,and may have up to 3 aides for every 2 counselors.How many counselors and how many aides should the camp hire to minimize cost?
A) 12 counselors and 18 aides
B) 27 counselors and 18 aides
C) 35 counselors and 10 aides
D) 18 counselors and 12 aides
A) 12 counselors and 18 aides
B) 27 counselors and 18 aides
C) 35 counselors and 10 aides
D) 18 counselors and 12 aides

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
29
A company has two different locations to assemble three different models of PCs.The table below summarizes the daily production capacity,the minimum number of each type needed,and the daily operating costs for each location.Find the number of days that each location needs to operate in order to fill the orders at minimum cost. 


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
30
Give the dual of:
Maximize
Z =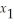
+ 3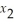
+ 4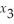
subject to
3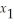
+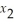
-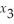
≤ 4
4
-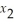
+ 2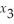
≥ 1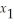
,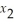
,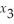
≥ 0.
Maximize
Z =
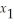
+ 3
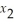
+ 4
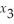
subject to
3
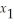
+
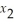
-
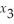
≤ 4
4

-
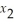
+ 2
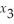
≥ 1
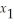
,
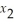
,
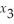
≥ 0.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
31
In the initial simplex table below,find the pivot entry. 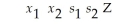
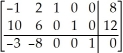
A) -1
B) 0
C) 10
D) 2
E) 6

A) -1
B) 0
C) 10
D) 2
E) 6

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
32
In the initial simplex table below,find the departing variable. 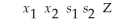
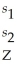

A)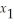
B)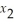
C)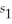
D)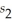
E) Z
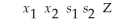
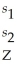

A)
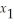
B)
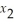
C)
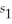
D)
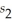
E) Z

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
33
A company manufactures two models of inline skates,Alpha and Beta,at two different manufacturing plants.The maximum output at plant I is 1000 per month,while the maximum output at plant II is 1200 per month.Due to contractual obligations,the number of Alpha models produced at plant I must exceed the number of Beta models produced at plant I by at least 100.The profit per Alpha and Beta model manufactured at plant I is $50 and $80,respectively,while the profit per Alpha and Beta model manufactured at plant II is $60 and $70,respectively.This month,the company received an order for 900 Alpha and 1000 Beta models.Find how many of each model should be produced at each plant in order to satisfy the order and maximize the profit.(Hint: Let 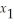
represent the number of Alpha models and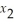
represent the number of Beta models manufactured at plant I.)
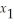
represent the number of Alpha models and
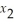
represent the number of Beta models manufactured at plant I.)

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
34
A manufacturer produces two products,product A and product B.Both products require processing on Machines I and II.The number of hours needed to produce one unit is given by the following chart: 
Machine I is available for at most 1000 hours and Machine II is available for at most 2500 hours.If the profit made on product A is $20 / unit and the profit made on product B is $25 / unit.Find the production level that will maximize profit and find the Maximum profit.

Machine I is available for at most 1000 hours and Machine II is available for at most 2500 hours.If the profit made on product A is $20 / unit and the profit made on product B is $25 / unit.Find the production level that will maximize profit and find the Maximum profit.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
35
Use the simplex method to minimize
Z = 4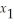
+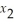
subject to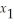
+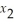
≥ 5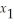
+ 2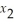
≥ 8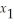
,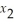
≥ 0.
Z = 4
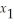
+
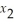
subject to
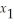
+
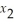
≥ 5
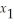
+ 2
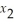
≥ 8
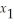
,
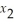
≥ 0.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
36
Maximize
Z = 4x + 6y
subject to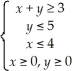
Z = 4x + 6y
subject to
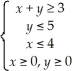

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
37
Use the simplex method to
maximize
Z = 2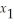
- 3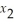
subject to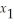
+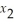
≥ 5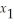
+ 2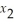
≤ 8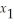
,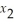
≥ 0
maximize
Z = 2
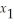
- 3
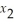
subject to
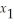
+
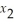
≥ 5
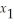
+ 2
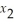
≤ 8
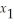
,
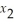
≥ 0

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
38
Use the simplex method to solve the following problem: United Blimpo Co.produces two types of exercise devices,regular and heavy-duty,each of which requires in its manufacture the use of two machines,A and B.A regular model requires the use of machine A for 2 hours and machine B for 3 hours.A heavy-duty model requires the use of machine A for 3 hours and machine B for 3 hours.Machine A can be used at most 18 hours a day and machine B can be used at most 21 hours a day.If the profits on the regular and heavy-duty models are $20 and $15,respectively,and United Blimpo Co.can sell all it produces,how many of each model should be produced per day in order to realize maximum profit? What is the maximum profit per day?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
39
Use the simplex method to maximize
Z = 30x + 50y
subject to
2x + y ≤ 16
x + 2y ≤ 11
x + 3y ≤ 15
x,y ≥ 0
Z = 30x + 50y
subject to
2x + y ≤ 16
x + 2y ≤ 11
x + 3y ≤ 15
x,y ≥ 0

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
40
Use the simplex method to minimize
Z = -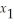
+ 2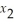
subject to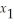
+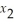
≤ 4
5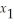
+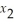
≥ -12
2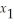
+ 5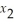
≥ -14
3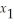
- 2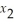
≤ 17
Z = -
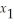
+ 2
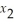
subject to
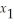
+
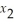
≤ 4
5
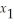
+
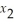
≥ -12
2
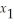
+ 5
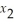
≥ -14
3
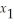
- 2
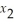
≤ 17

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
41
Use the dual and the simplex method to minimize
Z = 4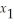
+ 5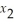
subject to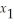
-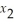
≥ 4
2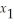
-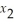
≥ 1
5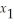
+ 3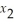
≥ 3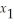
,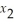
≥ 0.
Z = 4
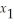
+ 5
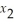
subject to
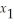
-
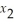
≥ 4
2
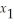
-
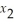
≥ 1
5
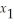
+ 3
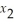
≥ 3
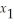
,
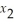
≥ 0.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
42
Find the dual problem to the following: A company has two different locations to assemble three different models of PCs.The table below summarizes the daily production capacity,the minimum number of each type needed,and the daily operating costs for each location.What is the number of days that each location needs to operate in order to fill the orders at minimum cost. 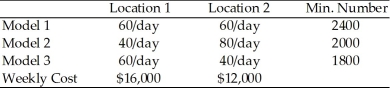


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
43
The dual of
Minimize
Z =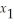
+ 3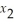
Subject to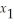
- 2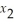
≥ 4
3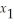
+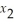
≥ 1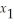
,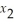
≥ 0
Is:
A) Maximize W =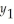 + 3
+ 3 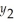 subject to
subject to 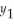 - 2
- 2 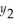 ≤ 4; 3
≤ 4; 3 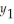 +
+  ≤ 1;
≤ 1; 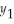 ,
, 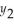 ≥ 0.
≥ 0.
B) Maximize W =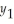 + 3
+ 3 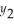 subject to
subject to 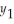 + 3
+ 3 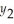 ≥ 4; - 2
≥ 4; - 2 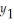 +
+ 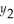 ≥ 1;
≥ 1; 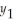 ,
, 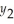 ≥ 0.
≥ 0.
C) Maximize W = 4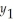 +
+ 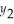 subject to
subject to 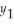 - 2
- 2 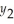 ≥ 1; 3
≥ 1; 3 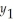 +
+ 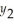 ≥ 3;
≥ 3; 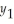 ,
, 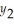 , ≥ 0.
, ≥ 0.
D) Maximize W = 4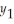 +
+ 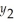 subject to
subject to 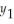 + 3
+ 3 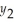 ≤ 1; - 2
≤ 1; - 2 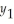 +
+ 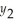 ≤ 3;
≤ 3; 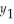 ,
, 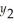 ≥ 0.
≥ 0.
E) Maximize W = 4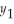 +
+ 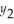 subject to
subject to 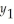 + 3
+ 3 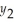 ≥ 1; - 2
≥ 1; - 2 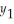 +
+ 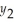 ≥ 3;
≥ 3; 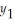 ,
, 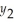 ≥ 0.
≥ 0.
Minimize
Z =
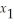
+ 3
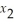
Subject to
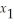
- 2
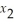
≥ 4
3
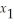
+
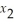
≥ 1
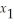
,
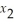
≥ 0
Is:
A) Maximize W =
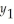 + 3
+ 3 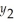 subject to
subject to 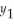 - 2
- 2 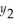 ≤ 4; 3
≤ 4; 3 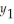 +
+  ≤ 1;
≤ 1; 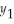 ,
, 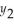 ≥ 0.
≥ 0.B) Maximize W =
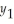 + 3
+ 3 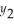 subject to
subject to 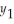 + 3
+ 3 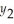 ≥ 4; - 2
≥ 4; - 2 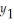 +
+ 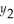 ≥ 1;
≥ 1; 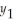 ,
, 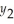 ≥ 0.
≥ 0.C) Maximize W = 4
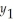 +
+ 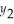 subject to
subject to 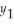 - 2
- 2 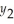 ≥ 1; 3
≥ 1; 3 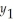 +
+ 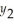 ≥ 3;
≥ 3; 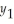 ,
, 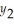 , ≥ 0.
, ≥ 0.D) Maximize W = 4
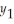 +
+ 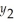 subject to
subject to 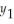 + 3
+ 3 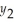 ≤ 1; - 2
≤ 1; - 2 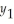 +
+ 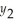 ≤ 3;
≤ 3; 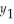 ,
, 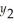 ≥ 0.
≥ 0.E) Maximize W = 4
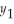 +
+ 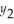 subject to
subject to 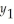 + 3
+ 3 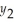 ≥ 1; - 2
≥ 1; - 2 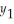 +
+ 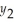 ≥ 3;
≥ 3; 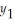 ,
,  ≥ 0.
≥ 0.
Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
44
Find the dual problem to the following: The What If Company has $30,000 for the purchase of material to make three types of gadgets.The company has allocated a total of 1200 hours of assembly time and 180 hours of packaging time for the gadgets.The following table gives the cost per gadget,the number of hours per gadget,and the profit per gadget for each type. 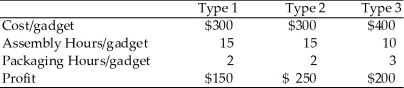
What is the number of gadgets of each type the company should produce to maximize profit?

What is the number of gadgets of each type the company should produce to maximize profit?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck



