Deck 3: Polynomial and Rational Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/122
Play
Full screen (f)
Deck 3: Polynomial and Rational Functions
1
Complete the square to write the function in shifted form. f(x) = x2 + 7x + 1
A) f(x) =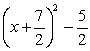
B) f(x) =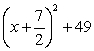
C) f(x) =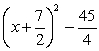
D) f(x) =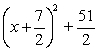
A) f(x) =
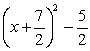
B) f(x) =
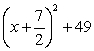
C) f(x) =
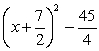
D) f(x) =
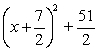
f(x) = 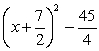
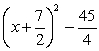
2
Use the following to answer questions :
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function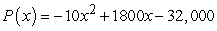 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.
Find the y-intercept and explain what it means in this context.
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function
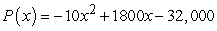 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.Find the y-intercept and explain what it means in this context.
(0, -22,000); when no cars are produced, there is a loss of $22,000
3
Use synthetic division and the remainder theorem to evaluate P(-3) if P(x) = 2x3 - 3x2 + 5x - 13.
A) -110
B) -109
C) -108
D) -107
A) -110
B) -109
C) -108
D) -107
-109
4
Use the following to answer questions :
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function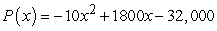 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.
What is the maximum profit?
A) $98,000
B) $99,000
C) $100,000
D) $101,000
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function
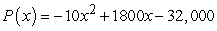 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.What is the maximum profit?
A) $98,000
B) $99,000
C) $100,000
D) $101,000

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
5
Use synthetic division and the remainder theorem to show that x = -2 is a zero of P(x) = x3 - 4x2 - 4x + 16.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
6
Find the vertex.
f(x) = x2 + 10x - 8
f(x) = x2 + 10x - 8

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
7
Use the following to answer questions :
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function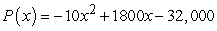 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.
How many cars should be made and sold to maximize profit?
A) 25
B) 75
C) 150
D) 1500
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function
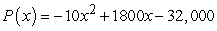 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.How many cars should be made and sold to maximize profit?
A) 25
B) 75
C) 150
D) 1500

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
8
Graph the function using end behavior, intercepts and by completing the square to write the function in shifted form. Clearly state the transformations used to obtain the graph, and label the vertex and all intercepts (if they exist). Use the quadratic formula to find the x-intercepts.
f(x) = 2x2 - 9x + 4
f(x) = 2x2 - 9x + 4

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
9
Find the intercepts. Round to the nearest tenth if necessary.
f(x) = 3x2 + 8x - 6
f(x) = 3x2 + 8x - 6

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
10
Find the intercepts.
f(x) = x2 - 10x + 24
f(x) = x2 - 10x + 24

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
11
Use the vertex/intercept formula, 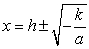 , to find zeroes, real or complex of the formula. f(x) = 3(x + 1)2 - 2
, to find zeroes, real or complex of the formula. f(x) = 3(x + 1)2 - 2
A)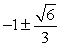
B)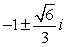
C)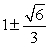
D)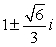
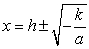 , to find zeroes, real or complex of the formula. f(x) = 3(x + 1)2 - 2
, to find zeroes, real or complex of the formula. f(x) = 3(x + 1)2 - 2A)
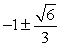
B)
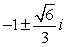
C)
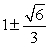
D)
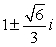

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
12
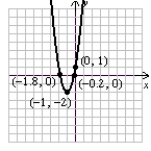
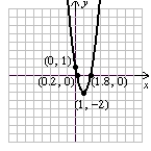
Graph the function using end behavior, intercepts and by completing the square to write the function in shifted form. Clearly state the transformations used to obtain the graph, and label the vertex and all intercepts (if they exist). Use the quadratic formula to find the x-intercepts. f(x) = 3x2 + 5x + 1
A) left 1, down 2, stretched vertically
B) right 1, down 2, stretched vertically
C) left .8, down 1.1, stretched vertically
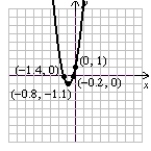
D) right .8, down 1.1, stretched vertically
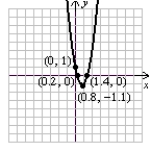
A) left 1, down 2, stretched vertically

B) right 1, down 2, stretched vertically

C) left .8, down 1.1, stretched vertically

D) right .8, down 1.1, stretched vertically


Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
13
Complete the square to write the function in shifted form.
f(x) = x2 + 6x + 1
f(x) = x2 + 6x + 1

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
14
Determine the equation of the function shown. 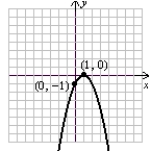
A) f(x) = -x2 + 1
B) f(x) = -x2 - 1
C) f(x) = -(x + 1)2
D) f(x) = -(x - 1)2

A) f(x) = -x2 + 1
B) f(x) = -x2 - 1
C) f(x) = -(x + 1)2
D) f(x) = -(x - 1)2

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
15
Graph the function using end behavior, intercepts and by completing the square to write the function in shifted form. Clearly state the transformations used to obtain the graph, and label the vertex and all intercepts (if they exist). Use the quadratic formula to find the x-intercepts.
f(x) = -x2 + 4x - 3
f(x) = -x2 + 4x - 3

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
16
Graph the function using the concavity, y-intercept, x-intercept(s), vertex, and symmetry. f(x) = 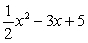
A)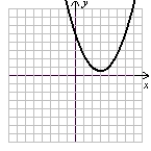
(Gridlines are spaced one unit apart.)
B)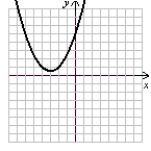
(Gridlines are spaced one unit apart.)
C)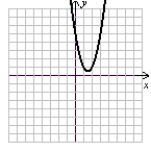
(Gridlines are spaced one unit apart.)
D)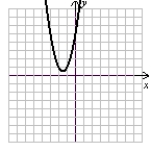
(Gridlines are spaced one unit apart.)
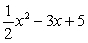
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
17
Use synthetic division and the remainder theorem to evaluate P(4) if P(x) = x3 - 5x2 - 4x + 20. Verify using a second method.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
18
Use the following to answer questions :
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function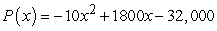 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.
Find the x-intercepts and explain what they mean in this context.
An automobile manufacturer can produce up to 300 cars per day. The profit made from the sale of these vehicles can be modeled by the function
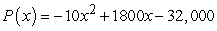 , where P(x) is the profit in dollars and x is the number of automobiles made and sold.
, where P(x) is the profit in dollars and x is the number of automobiles made and sold.Find the x-intercepts and explain what they mean in this context.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
19
Graph the function using the end behavior, y-intercept, x-intercept(s), vertex, and symmetry. Label the vertex and all intercepts (if they exist). (Round to tenths as necessary).
f(x) = -x2 + 2x + 5
f(x) = -x2 + 2x + 5

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
20
Graph the function using end behavior, intercepts and by completing the square to write the function in shifted form. Clearly state the transformations used to obtain the graph, and label the vertex and all intercepts (if they exist). Use the quadratic formula to find the x-intercepts.
f(x) = 2x2 + 9x + 4
f(x) = 2x2 + 9x + 4

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
21
A polynomial P of degree 3 with integer coefficients has zeroes x = 5, 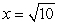 , and
, and 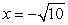 . Use the factor theorem to write the polynomial in factored form and standard form.
. Use the factor theorem to write the polynomial in factored form and standard form.
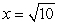 , and
, and 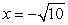 . Use the factor theorem to write the polynomial in factored form and standard form.
. Use the factor theorem to write the polynomial in factored form and standard form.
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
22
Use the factor theorem to find the polynomial of degree 4 having zeroes 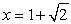 ,
, 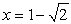 , x = 3 + i, x = 3 - i. Assume a lead coefficient of 1.
, x = 3 + i, x = 3 - i. Assume a lead coefficient of 1.
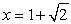 ,
, 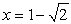 , x = 3 + i, x = 3 - i. Assume a lead coefficient of 1.
, x = 3 + i, x = 3 - i. Assume a lead coefficient of 1.
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
23
Find a cubic polynomial with real coefficients having roots x = -2 and x = 1 + 4i. Assume a lead coefficient of 1.
A) P(x) = x3 + 13x + 34
B) P(x) = x3 + 13x - 34
C) P(x) = x3 + 4x2 + 21x + 34
D) P(x) = x3 - 4x2 + 21x - 34
A) P(x) = x3 + 13x + 34
B) P(x) = x3 + 13x - 34
C) P(x) = x3 + 4x2 + 21x + 34
D) P(x) = x3 - 4x2 + 21x - 34

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
24
Use the rational roots theorem to write the function in factored form and find all zeroes. Note a = 1. p(x) = x4 + 6x3 - 13x2 - 66x + 72
A) p(x) = (x - 1)(x - 3)(x + 4)(x + 6); zeroes: 1, 3, -4, -6
B) p(x) = (x + 1)(x + 3)(x - 4)(x - 6); zeroes: -1, -3, 4, 6
C) p(x) = (x - 1)(x + 3)(x - 4)(x + 6); zeroes: 1, -3, 4, -6
D) p(x) = (x + 1)(x - 3)(x + 4)(x - 6); zeroes: -1, 3, -4, 6
A) p(x) = (x - 1)(x - 3)(x + 4)(x + 6); zeroes: 1, 3, -4, -6
B) p(x) = (x + 1)(x + 3)(x - 4)(x - 6); zeroes: -1, -3, 4, 6
C) p(x) = (x - 1)(x + 3)(x - 4)(x + 6); zeroes: 1, -3, 4, -6
D) p(x) = (x + 1)(x - 3)(x + 4)(x - 6); zeroes: -1, 3, -4, 6

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
25
List all possible rational roots for the polynomial but do not solve.
f(x) = 21x3 - 5x2 + x - 175
f(x) = 21x3 - 5x2 + x - 175

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
26
Use the following to answer questions :
p(x) = x4 - 5x3 + 5x2 + 5x - 6
Use the rational roots theorem to list all possible rational roots.
A)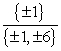
B)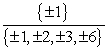
C)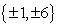
D)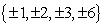
p(x) = x4 - 5x3 + 5x2 + 5x - 6
Use the rational roots theorem to list all possible rational roots.
A)
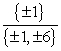
B)
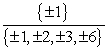
C)
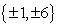
D)
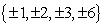

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
27
Use the rational roots theorem to write the function in factored form and find all zeroes. Note a = 1.
p(x) = x3 + 4x2 - 9x - 36
p(x) = x3 + 4x2 - 9x - 36

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
28
Find a quartic polynomial (degree 4) with real coefficients having roots x = -5i and x = 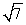 i. Assume a lead coefficient of 1.
i. Assume a lead coefficient of 1.
A) P(x) = x4 + 32x3 + 25x2 + 7x + 175
B) P(x) = x4 + 25x3 + 32x2 + 7x + 175
C) P(x) = x4 + 32x2 + 175
D) P(x) = x4 + 25x2 + 175
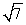 i. Assume a lead coefficient of 1.
i. Assume a lead coefficient of 1.A) P(x) = x4 + 32x3 + 25x2 + 7x + 175
B) P(x) = x4 + 25x3 + 32x2 + 7x + 175
C) P(x) = x4 + 32x2 + 175
D) P(x) = x4 + 25x2 + 175

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
29
Use synthetic division and the remainder theorem to show x = 1 - 3i is a zero of P(x) = -x3 - x2 - 4x - 30.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
30
Find a polynomial P with real coefficients having degree 5, only one real, rational root, and zeroes x = 2 and x = 1 + 5i. Assume a lead coefficient of 1. Leave your answer in factored form.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
31
Find the zeroes of the polynomial using any combination of the rational roots theorem, synthetic division, testing for 1 and -1, and/or the remainder and factor theorems.
p(x) = 4x4 - 3x3 - 30x2 - x + 30
p(x) = 4x4 - 3x3 - 30x2 - x + 30

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
32
Find all rational zeroes of the function given and use them to write the function in factored form. Use the factored form to state all zeroes of the function. Begin by applying the tests for 1 and -1. p(x) = 4x4 + x3 + 33x2 +9x - 27
A) p(x) = (x - 1)(4x - 3)(x - 3i)(x + 3i); zeroes: 1,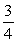 , 3i, -3i
, 3i, -3i
B) p(x) = (x - 1)(4x + 3)(x - 3i)(x + 3i); zeroes: 1, -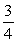 , 3i, -3i
, 3i, -3i
C) p(x) = (x + 1)(4x - 3)(x - 3i)(x + 3i); zeroes: -1,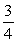 , 3i, -3i
, 3i, -3i
D) p(x) = (x + 1)(4x + 3)(x - 3i)(x + 3i); zeroes: -1, -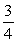 , 3i, -3i
, 3i, -3i
A) p(x) = (x - 1)(4x - 3)(x - 3i)(x + 3i); zeroes: 1,
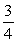 , 3i, -3i
, 3i, -3iB) p(x) = (x - 1)(4x + 3)(x - 3i)(x + 3i); zeroes: 1, -
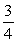 , 3i, -3i
, 3i, -3iC) p(x) = (x + 1)(4x - 3)(x - 3i)(x + 3i); zeroes: -1,
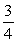 , 3i, -3i
, 3i, -3iD) p(x) = (x + 1)(4x + 3)(x - 3i)(x + 3i); zeroes: -1, -
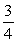 , 3i, -3i
, 3i, -3i
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
33
Find a cubic polynomial with real coefficients having roots x = -3 and x = -i. Assume a lead coefficient of 1.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
34
Use the remainder theorem to show x = 2i is a zero of P(x) = x3 + 2x2 + 4x + 8.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
35
Factor completely. Then state the multiplicity of the roots and the degree of P.
P(x) = x3 - 2x2 - 15x + 36.
P(x) = x3 - 2x2 - 15x + 36.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
36
A polynomial P of degree 3 with integer coefficients has zeroes x = 3, x = -9, and x = 1. Use the factor theorem to write the polynomial in factored form.
A) P(x) = (x + 3)(x - 9)(x + 1)
B) P(x) = (x - 3)(x + 9)(x - 1)
C) P(x) = (3x + 1)(-9x + 1)(x + 1)
D) P(x) = (-3x + 1)(9x + 1)(-x + 1)
A) P(x) = (x + 3)(x - 9)(x + 1)
B) P(x) = (x - 3)(x + 9)(x - 1)
C) P(x) = (3x + 1)(-9x + 1)(x + 1)
D) P(x) = (-3x + 1)(9x + 1)(-x + 1)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
37
Find a quartic polynomial (degree 4) with real coefficients having roots x = 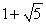 and x = 1 +
and x = 1 + 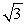 i. Assume a lead coefficient of 1.
i. Assume a lead coefficient of 1.
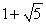 and x = 1 +
and x = 1 + 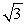 i. Assume a lead coefficient of 1.
i. Assume a lead coefficient of 1.
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
38
Find the zeroes of the polynomial using any combination of the rational roots theorem, synthetic division, testing for 1 and -1, and/or the remainder and factor theorems. p(x) = x4 + 3x3 + 3x2 - 17x - 18
A) -1, 2,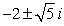
B) 1, -2,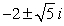
C) -1, 2,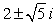
D) 1, -2,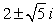
A) -1, 2,
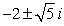
B) 1, -2,
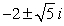
C) -1, 2,
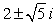
D) 1, -2,
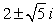

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
39
Use the factor theorem to find the polynomial of degree 3 having zeroes x = 1, x = 1 - 4i, and x = 1 + 4i. Assume a lead coefficient of 1.
A) P(x) = x3 - 3x2 + 20x - 16
B) P(x) = x3 - 3x2 + 20x - 17
C) P(x) = x3 - 3x2 + 19x - 16
D) P(x) = x3 - 3x2 + 19x - 17
A) P(x) = x3 - 3x2 + 20x - 16
B) P(x) = x3 - 3x2 + 20x - 17
C) P(x) = x3 - 3x2 + 19x - 16
D) P(x) = x3 - 3x2 + 19x - 17

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
40
Use the intermediate value theorem to verify that the polynomial f(x) = -2x3 - 4x2 + 3x - 4 has at least one zero "ci" in the interval [-3, -1]. Do not find the zeroes.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
41
Find all zeroes (real and complex) of the polynomial.
p(x) = 4x3 - 5x2 - 23x + 6
p(x) = 4x3 - 5x2 - 23x + 6

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
42
Gather information on the polynomial using the rational roots theorem, testing for 1 and -1, Descartes's rule of signs, and the upper and lower bounds property. Respond explicitly to each.
p(x) = x4 - 3x3 + 9x - 27
p(x) = x4 - 3x3 + 9x - 27

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
43
State the degree and end behavior of the function. f(x) = (x - 1)6(x + 3)(x - 3)3
A) degree 9, up/up
B) degree 9, down/up
C) degree 10, up/up
D) degree 10, down/up
A) degree 9, up/up
B) degree 9, down/up
C) degree 10, up/up
D) degree 10, down/up

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
44
State the end behavior and y-intercept of the function. Do not graph.
f(x) = x5 + 5x4 - 5x2 - 3x + 5
f(x) = x5 + 5x4 - 5x2 - 3x + 5

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
45
Use the following to answer questions : 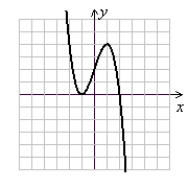 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Find the minimum possible degree of the function and write it in factored form. Assume all zeroes are real.
A) f(x) = -(x + 1)(x - 2)
B) f(x) = -(x + 1)2(x - 2)
C) f(x) = (x + 1)(x - 2)2
D) f(x) = (x + 1)2(x - 2)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)Find the minimum possible degree of the function and write it in factored form. Assume all zeroes are real.
A) f(x) = -(x + 1)(x - 2)
B) f(x) = -(x + 1)2(x - 2)
C) f(x) = (x + 1)(x - 2)2
D) f(x) = (x + 1)2(x - 2)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
46
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
State whether the degree of the function is even or odd.
A) even
B) odd
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)State whether the degree of the function is even or odd.
A) even
B) odd

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
47
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Use the graph to estimate the zeroes of the function, then state whether their multiplicity is even or odd.
A) -1, even; 2 odd
B) -1, odd; 2 even
C) -1, even; 2 even
D) -1, odd; 2 odd
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)Use the graph to estimate the zeroes of the function, then state whether their multiplicity is even or odd.
A) -1, even; 2 odd
B) -1, odd; 2 even
C) -1, even; 2 even
D) -1, odd; 2 odd

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
48
Use the Guidelines for Graphing Polynomial Functions to graph the polynomial.
f(x) = x3 - 3x - 2
f(x) = x3 - 3x - 2

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
49
For the complex polynomial below, one of its zeroes is z = 5i. Use the given zero to help find all zeroes of the polynomial, then write the polynomial in completely factored form. Hint: synthetic division and the quadratic formula can be applied to all polynomials, even those with complex coefficients.
C(z) = z3 + (1 - 5i)z2 + (-6 - 5i)z + 30i.
C(z) = z3 + (1 - 5i)z2 + (-6 - 5i)z + 30i.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
50
State the degree, end behavior, and y-intercept of the function.
f(x) = -(x - 5)(x - 1)8(x - 2)2
f(x) = -(x - 5)(x - 1)8(x - 2)2

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
51
State the end behavior of the function. f(x) = 5x4 + 2x3 - 5x2 - 5x - 3
A) down/up
B) up/down
C) down/down
D) up/up
A) down/up
B) up/down
C) down/down
D) up/up

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
52
Use the following to answer questions :
p(x) = x4 - 5x3 + 5x2 + 5x - 6
Use the information gathered, testing for 1 and -1, synthetic division, and the factor theorem to factor p completely.
A) p(x) = (x + 1)(x - 1)(x + 2)(x + 3)
B) p(x) = (x + 1)(x - 1)(x - 2)(x + 3)
C) p(x) = (x + 1)(x - 1)(x + 2)(x - 3)
D) p(x) = (x + 1)(x - 1)(x - 2)(x - 3)
p(x) = x4 - 5x3 + 5x2 + 5x - 6
Use the information gathered, testing for 1 and -1, synthetic division, and the factor theorem to factor p completely.
A) p(x) = (x + 1)(x - 1)(x + 2)(x + 3)
B) p(x) = (x + 1)(x - 1)(x - 2)(x + 3)
C) p(x) = (x + 1)(x - 1)(x + 2)(x - 3)
D) p(x) = (x + 1)(x - 1)(x - 2)(x - 3)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
53
Find all zeroes (real and complex) of the polynomial.
p(x) = 6x3 + 29x2 - 149x + 24
p(x) = 6x3 + 29x2 - 149x + 24

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
54
Use the Guidelines for Graphing Polynomial Functions to graph the polynomial. f(x) = x3 - 4x2 + x + 6
A)
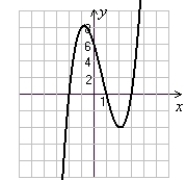
B)

C)
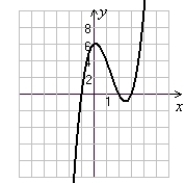
D)
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
55
State the end behavior of the function. f(x) = -3x5 + 5x4 + x2 - 2x + 2
A) down/up
B) up/down
C) down/down
D) up/up
A) down/up
B) up/down
C) down/down
D) up/up

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
56
State the end behavior and y-intercept of the function. Do not graph.
f(x) = -2x4 - 2x3 + x2 - 2x + 5
f(x) = -2x4 - 2x3 + x2 - 2x + 5

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
57
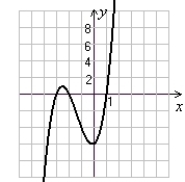
Use the following to answer questions :
p(x) = x4 - 5x3 + 5x2 + 5x - 6
Apply Descartes's rule of signs to count the possible number of positive, negative, and complex roots (organize a table).
A)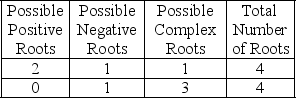
B)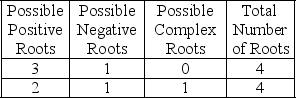
C)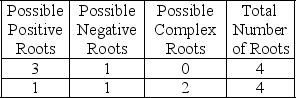
D)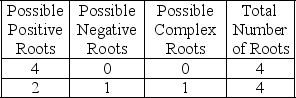
p(x) = x4 - 5x3 + 5x2 + 5x - 6
Apply Descartes's rule of signs to count the possible number of positive, negative, and complex roots (organize a table).
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
58
Sketch the graph of the function using the degree, end behavior, x- and y-intercepts, zeroes of multiplicity, and a few midinterval points to round out the graph. Connect all points with a smooth, continuous curve.
f(x) = (x - 1)3(x + 2)(x + 3)
f(x) = (x - 1)3(x + 2)(x + 3)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
59
Use Descartes's rule of signs to determine the possible combinations of real and complex roots for the polynomial. Then graph the function on the standard window of a graphing calculator and adjust it as needed until you're certain all real roots are in clear view. Use this screen and a list of the possible rational zeroes (rational roots theorem) to factor the polynomial and find all zeroes (real and complex). p(x) = 20x3 - 153x2 - 62x + 48
A) p(x) = (x - 8)(2x + 1)(10x + 6)
B) p(x) = (x + 8)(10x - 1)(2x + 6)
C) p(x) = (x - 8)(5x - 2)(4x + 3)
D) p(x) = (x + 8)(4x + 1)(5x + 6)
A) p(x) = (x - 8)(2x + 1)(10x + 6)
B) p(x) = (x + 8)(10x - 1)(2x + 6)
C) p(x) = (x - 8)(5x - 2)(4x + 3)
D) p(x) = (x + 8)(4x + 1)(5x + 6)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
60
For the complex polynomial below, one of its zeroes is x = 3 - i. Use the given zero to help find all zeroes of the polynomial, then write the polynomial in completely factored form. Hint: synthetic division and the quadratic formula can be applied to all polynomials, even those with complex coefficients. C(x) = x3 - 3x2 + (3 + 3i)x + (-6 + 2i)
A) C(x) = (x - 3 - i)(x + 2i)(x + i); x = -2i, x = -i
B) C(x) = (x - 3 + i)(x - 2i)(x - i); x = 2i, x = i
C) C(x) = (x - 3 + i)(x + 2i)(x - i); x = -2i, x = i
D) C(x) = (x - 3 + i)(x - 2i)(x + i); x = 2i, x = -i
A) C(x) = (x - 3 - i)(x + 2i)(x + i); x = -2i, x = -i
B) C(x) = (x - 3 + i)(x - 2i)(x - i); x = 2i, x = i
C) C(x) = (x - 3 + i)(x + 2i)(x - i); x = -2i, x = i
D) C(x) = (x - 3 + i)(x - 2i)(x + i); x = 2i, x = -i

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
61
Graph the polynomial function.
f(x) = x3 + 2x2 - 5x - 6
f(x) = x3 + 2x2 - 5x - 6

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
62
Use the following to answer questions : 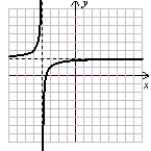 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Give the equation related to the graph. Assume |a| = 1.
A)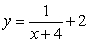
B)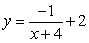
C)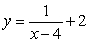
D)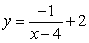
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)Give the equation related to the graph. Assume |a| = 1.
A)
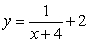
B)
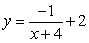
C)
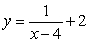
D)
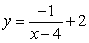

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
63
Determine the location of the horizontal asymptote if it exists. Then determine whether the graph will cross the asymptote, and if so, where it crosses. 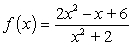
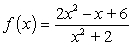

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
64
Use the following to answer questions : 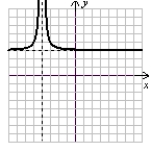 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
State the equations of the horizontal and vertical asymptotes.
A) y = 4, x = -3
B) y = -4, x = 3
C) y = -3, x = 4
D) y = 3, x = -4
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)State the equations of the horizontal and vertical asymptotes.
A) y = 4, x = -3
B) y = -4, x = 3
C) y = -3, x = 4
D) y = 3, x = -4

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
65
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
State the equations of the horizontal and vertical asymptotes.
A) y = -4, x = 2
B) y = 4, x = -2
C) y = 2, x = -4
D) y = -2, x = 4
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)State the equations of the horizontal and vertical asymptotes.
A) y = -4, x = 2
B) y = 4, x = -2
C) y = 2, x = -4
D) y = -2, x = 4

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
66
Give the location of the vertical asymptote(s) if they exist, and state whether function values will change sign (positive to negative or negative to positive) from one side of the asymptote to the other. 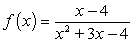
A) x = 1, no
B) x = 1, yes
C) x = -4, no; x = 1, no
D) x = -4, yes; x = 1, yes
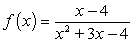
A) x = 1, no
B) x = 1, yes
C) x = -4, no; x = 1, no
D) x = -4, yes; x = 1, yes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
67
Use the following to answer questions : 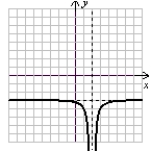 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
-State the range of the function.
A) y (-?, -2) (-2, ?)
B) y (-?, 2) (2, ?)
C) y (-?, -3)
D) y (-?, 3)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)-State the range of the function.
A) y (-?, -2) (-2, ?)
B) y (-?, 2) (2, ?)
C) y (-?, -3)
D) y (-?, 3)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
68
Use polynomial division or synthetic division to rewrite the function, then sketch the graph using transformations of a parent function and the x- and y-intercepts (if they exist). 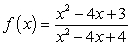
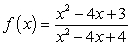

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
69
Graph the polynomial function.
f(x) = x4 + 3x3 - 3x2 - 11x - 6
f(x) = x4 + 3x3 - 3x2 - 11x - 6

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
70
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Give the equation related to the graph. Assume |a| = 1.
A)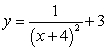
B)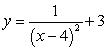
C)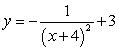
D)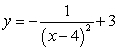
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)Give the equation related to the graph. Assume |a| = 1.
A)
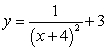
B)
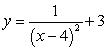
C)
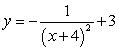
D)
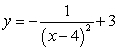

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
71
Use the following to answer questions : 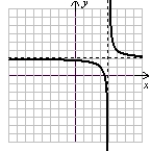 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Use the direction/approach notation to describe the end behavior of the graph. (This should produce four statements.)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)Use the direction/approach notation to describe the end behavior of the graph. (This should produce four statements.)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
72
Determine the location of the horizontal asymptote if it exists. Then determine whether the graph will cross the asymptote, and if so, where it crosses. 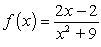
A) y = 0; crosses at (1, 0)
B) y = 0; does not cross
C) y = 2; does not cross
D) No horizontal asymptote
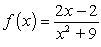
A) y = 0; crosses at (1, 0)
B) y = 0; does not cross
C) y = 2; does not cross
D) No horizontal asymptote

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
73
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
-State the domain of the function.
A) x (-?, -2) (-2, ?)
B) x (-?, 2) (2, ?)
C) x (-?, -3) (-3, ?)
D) x (-?, 3) (3, ?)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)-State the domain of the function.
A) x (-?, -2) (-2, ?)
B) x (-?, 2) (2, ?)
C) x (-?, -3) (-3, ?)
D) x (-?, 3) (3, ?)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
74
Give the location of the vertical asymptote(s) if they exist, and state the function's domain in set notation. 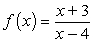
A) x = 1; {x | x R, x ? 1}
B) x = -3; {x | x R, x ? -3}
C) x = 4; {x | x R, x ? 4}
D) No vertical asymptote; {x | x R}
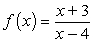
A) x = 1; {x | x R, x ? 1}
B) x = -3; {x | x R, x ? -3}
C) x = 4; {x | x R, x ? 4}
D) No vertical asymptote; {x | x R}

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
75
Give the location of the vertical asymptote(s) if they exist, and state whether function values will change sign (positive to negative or negative to positive) from one side of the asymptote to the other. 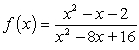
A) x = 4, no
B) x = 4, yes
C) x = -1, no; x = 2, no
D) x = -1, yes; x = 2, yes
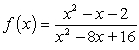
A) x = 4, no
B) x = 4, yes
C) x = -1, no; x = 2, no
D) x = -1, yes; x = 2, yes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
76
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Use the direction/approach notation to describe the end behavior of the graph. (This should produce four statements.)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)Use the direction/approach notation to describe the end behavior of the graph. (This should produce four statements.)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
77
Determine the location of the x- and y-intercepts (if they exist), and state the behavior of the function (bounce or cut) at each x-intercept. 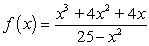
A) (0, 0), bounce; (-2, 0), cut
B) (0, 0), cut; (-2, 0), bounce
C) (0, 0), bounce; (-2, 0), cut; (5, 0), bounce
D) (0, 0), cut; (-2, 0), bounce; (5, 0), cut
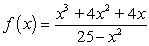
A) (0, 0), bounce; (-2, 0), cut
B) (0, 0), cut; (-2, 0), bounce
C) (0, 0), bounce; (-2, 0), cut; (5, 0), bounce
D) (0, 0), cut; (-2, 0), bounce; (5, 0), cut

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
78
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
-State the domain of the function.
A) x (-?, -4) (-4, ?)
B) x (-?, 4) (4, ?)
C) x (-?, -2) (-2, ?)
D) x (-?, 2) (2, ?)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)-State the domain of the function.
A) x (-?, -4) (-4, ?)
B) x (-?, 4) (4, ?)
C) x (-?, -2) (-2, ?)
D) x (-?, 2) (2, ?)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
79
Give the location of the vertical asymptote(s) if they exist, and state the function's domain in set notation. 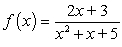
A) x = 2; {x | x R, x ? 2}
B) x =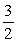 ; {x | x R, x ?
; {x | x R, x ?
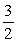 }
}
C) x = -5, x = -1; {x | x R, x ? -5, x ? -1}
D) No vertical asymptote; {x | x R}
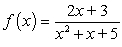
A) x = 2; {x | x R, x ? 2}
B) x =
 ; {x | x R, x ?
; {x | x R, x ?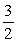 }
}C) x = -5, x = -1; {x | x R, x ? -5, x ? -1}
D) No vertical asymptote; {x | x R}

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
80
Use the following to answer questions :  (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
-State the range of the function.
A) y (-?, -4) (-4, ?)
B) y (-?, 4) (4, ?)
C) y (-?, -2) (-2, ?)
D) y (-?, 2) (2, ?)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)-State the range of the function.
A) y (-?, -4) (-4, ?)
B) y (-?, 4) (4, ?)
C) y (-?, -2) (-2, ?)
D) y (-?, 2) (2, ?)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck



