Deck 1: Real Numbers and Algebraic Expressions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/314
Play
Full screen (f)
Deck 1: Real Numbers and Algebraic Expressions
1
Write each set in roster form.
-{x|x is an odd whole number less than 16}
-{x|x is an odd whole number less than 16}
{1, 3, 5, 7, 9, 11, 13, 15}
2
Write each set in roster form.
-{x|x is an integer greater than 20}
-{x|x is an integer greater than 20}
{21, 22, 23, …}
3
Write each set in roster form.
-{x|x is a natural number less than 9}
-{x|x is a natural number less than 9}
{1, 2, 3, 4, 5, 6, 7, 8}
4
List the elements of the set that are also elements of the given set.
-Natural numbers
-Natural numbers

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
5
List the elements of the set that are also elements of the given set.
-Rational numbers
-Rational numbers

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
6
List the elements of the set that are also elements of the given set.
-Integers
-Integers

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
7
List the elements of the set that are also elements of the given set.
-Irrational numbers
-Irrational numbers

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
8
Determine whether each statement is true or false.
-Some integers are natural numbers.
-Some integers are natural numbers.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
9
Determine whether each statement is true or false.
--3 {x|x is an integer}
--3 {x|x is an integer}

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
10
Determine whether each statement is true or false.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
11
Determine whether each statement is true or false.
-{x|x is a day of the week starting with the letter L} is
-{x|x is a day of the week starting with the letter L} is

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
12
Write each phrase as an algebraic expression.
-Eight more than five times a number.
-Eight more than five times a number.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
13
Write each phrase as an algebraic expression.
-Three times a number, divided by ten.
-Three times a number, divided by ten.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
14
Write each phrase as an algebraic expression.
-The difference of fourteen and a number.
-The difference of fourteen and a number.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
15
Write each phrase as an algebraic expression.
-Nine less than twice a number.
-Nine less than twice a number.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
16
Write each phrase as an algebraic expression.
-Twelve minus seven times a number.
-Twelve minus seven times a number.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
17
Write each phrase as an algebraic expression.
-The quotient of three and the sum of a number and five.
-The quotient of three and the sum of a number and five.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
18
Write each phrase as an algebraic expression.
-Six times the difference of a number and eleven.
-Six times the difference of a number and eleven.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
19
Write each phrase as an algebraic expression.
-Name a negative rational number that does not simplify to an integer.
-Name a negative rational number that does not simplify to an integer.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
20
Write each sentence as an equation.
-The sum of 8 and twice x is -14.
-The sum of 8 and twice x is -14.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
21
Write each sentence as an equation.
-The difference of y and 5 is the same as the product of y and 8.
-The difference of y and 5 is the same as the product of y and 8.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
22
Write each sentence as an equation.
-The quotient of z and 7 is 5 more than three times z.
-The quotient of z and 7 is 5 more than three times z.

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
23
Insert <, >, or = between each pair of numbers to form a true statement.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
24
Insert <, >, or = between each pair of numbers to form a true statement.
-3.9 3.90
-3.9 3.90

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
25
Insert <, >, or = between each pair of numbers to form a true statement.
--4.1 -4.01
--4.1 -4.01

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
26
Insert <, >, or = between each pair of numbers to form a true statement.
--11.6 2.8
--11.6 2.8

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
27
Write the opposite (or additive inverse) of each number.
--5.6
--5.6

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
28
Write the opposite (or additive inverse) of each number.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
29
Write the opposite (or additive inverse) of each number.
-0
-0

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
30
Write the reciprocal (or multiplicative inverse) of each number if one exists.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
31
Write the reciprocal (or multiplicative inverse) of each number if one exists.
--10
--10

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
32
Write the reciprocal (or multiplicative inverse) of each number if one exists.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
33
Use a commutative property to write an equivalent expression.
-m + n
-m + n

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
34
Use a commutative property to write an equivalent expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
35
Use an associative property to write an equivalent expression.
-3x + (y + 2z)
-3x + (y + 2z)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
36
Use an associative property to write an equivalent expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
37
Use the distributive property to multiply.
-6(x + 9)
-6(x + 9)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
38
Use the distributive property to multiply.
-7(2a - 3b)
-7(2a - 3b)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
39
Use the distributive property to multiply.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
40
Find the absolute value.
-|-9|
-|-9|

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
41
Find the absolute value.
-|-12|
-|-12|

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
42
Add or subtract as indicated
--23 + 10
--23 + 10

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
43
Add or subtract as indicated
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
44
Add or subtract as indicated
-1.2 - 3.4
-1.2 - 3.4

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
45
Add or subtract as indicated
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
46
Add or subtract as indicated
--2 - 8 + 3
--2 - 8 + 3

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
47
Add or subtract as indicated
--11 + (-9) - 12
--11 + (-9) - 12

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
48
Multiply or divide as indicated
--3(-12)
--3(-12)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
49
Multiply or divide as indicated
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
50
Multiply or divide as indicated
-0 ÷ (-10)
-0 ÷ (-10)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
51
Multiply or divide as indicated
--5(-6)(-1)
--5(-6)(-1)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
52
Multiply or divide as indicated
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
53
Multiply or divide as indicated
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
54
Evaluate.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
55
Evaluate.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
56
Evaluate.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
57
Find each root.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
58
Find each root.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
59
Find each root.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
60
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
61
Simplify each expression.
--4[9 - (-2)]
--4[9 - (-2)]

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
62
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
63
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
64
Simplify each expression.
-3 - [(17 - 16) + (4 - 12)]
-3 - [(17 - 16) + (4 - 12)]

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
65
Simplify each expression.
-15 - 2(-5) +9
-15 - 2(-5) +9

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
66
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
67
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
68
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
69
Simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
70
For 11 and 12, evaluate each expression when x = 2 and y = -5.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
71
For 11 and 12, evaluate each expression when x = 2 and y = -5.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
72
For 11 and 12, evaluate each expression when x = 2 and y = -5.
-The algebraic expression represents the cost per compact disc (in dollars) of producing x compact discs (CD's).
Complete the table.
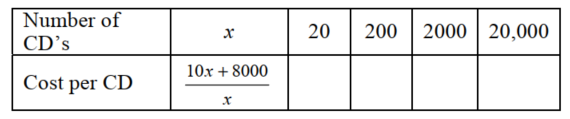
-The algebraic expression represents the cost per compact disc (in dollars) of producing x compact discs (CD's).
Complete the table.


Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
73
For 11 and 12, evaluate each expression when x = 2 and y = -5.
-As the number of CD's produced increases, does the cost per CD increase or decrease?
-As the number of CD's produced increases, does the cost per CD increase or decrease?

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
74
Simplify.
--7 +5x +15 - 9x
--7 +5x +15 - 9x

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
75
Simplify.
-9a - b + 8a - 7b
-9a - b + 8a - 7b

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
76
Simplify.
-(3 - 2y) - (10 - 11y)
-(3 - 2y) - (10 - 11y)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
77
Simplify.
-5(4n - 3) - 2(12n - 5)
-5(4n - 3) - 2(12n - 5)

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
78
Simplify.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
79
Simplify.
--1.3(4.6x - 2.4) + 3.75x
--1.3(4.6x - 2.4) + 3.75x

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck
80
Use the product rule to simplify each expression.
-
-

Unlock Deck
Unlock for access to all 314 flashcards in this deck.
Unlock Deck
k this deck



