Deck 10: Relational Predicate Logic
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/38
Play
Full screen (f)
Deck 10: Relational Predicate Logic
1
Symbolizing
-If something is heavy, then Art won't lift it. (Hx = "x is heavy"; a = "Art"; Lxy = "x will lift y".)
-If something is heavy, then Art won't lift it. (Hx = "x is heavy"; a = "Art"; Lxy = "x will lift y".)
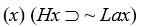
2
Symbolizing
-If something is heavy, then anyone who is weak will not lift it. (Px = "x is a person"; Wx = "x is weak".)
-If something is heavy, then anyone who is weak will not lift it. (Px = "x is a person"; Wx = "x is weak".)
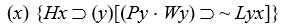
3
Symbolizing
-Everyone who loves himself hates someone (or other). (Px = "x is a person"; Lxy = "x loves y"; Hxy = "x hates y".)
-Everyone who loves himself hates someone (or other). (Px = "x is a person"; Lxy = "x loves y"; Hxy = "x hates y".)
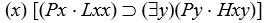
4
Symbolizing
-All who have sinned have come short of the glory of God. (Px = "x is a person"; Sx = "x has sinned"; Cxy = "x has come short of y"; g = "the glory of God.")
-All who have sinned have come short of the glory of God. (Px = "x is a person"; Sx = "x has sinned"; Cxy = "x has come short of y"; g = "the glory of God.")

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
5
Symbolizing
-If anything is heavy, then there is something heavier than this piece of chalk, which happens not to be heavy. (Hx = "x is heavy"; Hxy = "x is heavier than y"; c = "this piece of chalk".)
-If anything is heavy, then there is something heavier than this piece of chalk, which happens not to be heavy. (Hx = "x is heavy"; Hxy = "x is heavier than y"; c = "this piece of chalk".)

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
6
Symbolizing
-All freshmen date only seniors. (Fx = "x is a freshman"; Dxy = "x dates y"; Sx = "x is a senior".)
-All freshmen date only seniors. (Fx = "x is a freshman"; Dxy = "x dates y"; Sx = "x is a senior".)

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
7
Symbolizing
-Some freshmen date only seniors.
-Some freshmen date only seniors.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
8
Symbolizing
-Some freshmen date every senior.
-Some freshmen date every senior.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
9
Symbolizing
-Some freshmen date no seniors.
-Some freshmen date no seniors.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
10
Symbolizing
-No freshmen date all seniors.
-No freshmen date all seniors.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
11
Symbolizing
-No freshmen date any seniors.
-No freshmen date any seniors.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
12
Symbolizing
-Not all freshmen date seniors.
-Not all freshmen date seniors.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
13
Symbolizing
-Not all freshmen date every senior.
-Not all freshmen date every senior.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
14
Symbolizing
-Jones will listen to Smith, even though Smith is boring. (j = "Jones"; s = "Smith"; Lxy = "x will listen to y"; Bx = "x is boring".)
-Jones will listen to Smith, even though Smith is boring. (j = "Jones"; s = "Smith"; Lxy = "x will listen to y"; Bx = "x is boring".)

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
15
Symbolizing
-All sinners who sin against someone who is a sinner are sinned against by someone who is a sinner. (Sx = "x is a sinner"; Sxy = "x sins against y".)
-All sinners who sin against someone who is a sinner are sinned against by someone who is a sinner. (Sx = "x is a sinner"; Sxy = "x sins against y".)

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
16
Symbolizing
-If every sinner is sinned against by everyone who is a sinner, then everyone who sins against someone (or other) sins against himself.
-If every sinner is sinned against by everyone who is a sinner, then everyone who sins against someone (or other) sins against himself.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
17
Symbolizing
-Someone who is a sinner and sins against someone (or other) who is a sinner sins against himself.
-Someone who is a sinner and sins against someone (or other) who is a sinner sins against himself.

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
18
Symbolizing
-All heads of horses are heads of animals, provided that all horses are animals. (Hx = "x is a horse"; Ax = "x is an animal"; Hxy = "x is the head of y".)
-All heads of horses are heads of animals, provided that all horses are animals. (Hx = "x is a horse"; Ax = "x is an animal"; Hxy = "x is the head of y".)

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
19
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-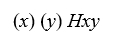
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
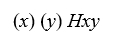

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
20
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-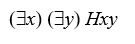
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
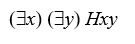

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
21
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-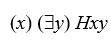
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
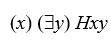

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
22
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-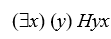
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
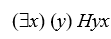

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
23
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-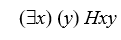
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
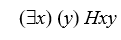

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
24
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-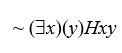
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
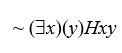

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
25
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-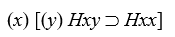
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
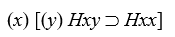

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
26
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-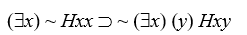
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
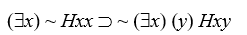

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
27
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-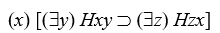
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
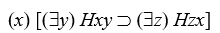

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
28
Translations.
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-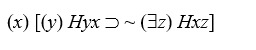
Letting Hxy = "x is heavier than y", translate the following into English (coming as close as possible to colloquial usage):
-
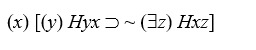

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
29
Proving invalidity.
Show that the following arguments are invalid:
-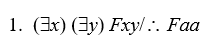
Show that the following arguments are invalid:
-
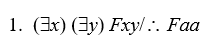

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
30
Proving invalidity.
Show that the following arguments are invalid:
-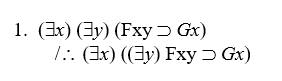
Show that the following arguments are invalid:
-
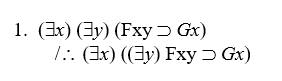

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
31
Prove valid
-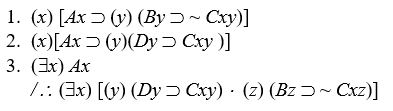
-
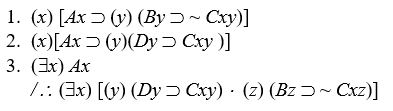

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
32
Prove valid
-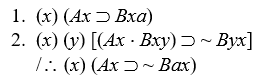
-
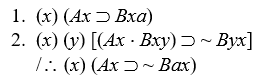

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
33
Prove valid
-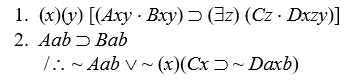
-
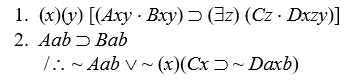

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
34
Prove valid
-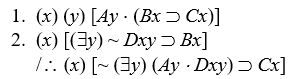
-
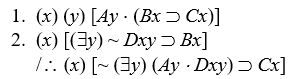

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
35
Theorems of logic.
Prove that the following are theorems of logic:
-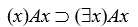
Prove that the following are theorems of logic:
-
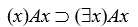

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
36
Theorems of logic.
Prove that the following are theorems of logic:
-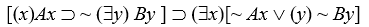
Prove that the following are theorems of logic:
-
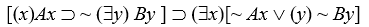

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
37
Theorems of logic.
Prove that the following are theorems of logic:
-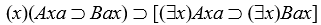
Prove that the following are theorems of logic:
-
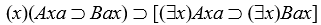

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck
38
Theorems of logic.
Prove that the following are theorems of logic:
-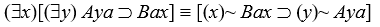
Prove that the following are theorems of logic:
-
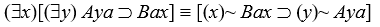

Unlock Deck
Unlock for access to all 38 flashcards in this deck.
Unlock Deck
k this deck



