Deck 11: Random Sampling and Sampling Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/24
Play
Full screen (f)
Deck 11: Random Sampling and Sampling Distributions
1
A sociologist engaged in research on religious attitudes tells her assistant to select a random sample of ten members for interviews from a large suburban church. The sample selected consists of nine women and one man. Upon seeing the uneven distribution of sexes in the sample, the sociologist complains, "This sample can't be random: it's almost all women." Any comments?
Whether or not a sample is random depends on how it was selected, not on its composition; the chance factors involved in random sampling occasionally lead to very atypical samples.
2
Explain on an intuitive basis why the sampling distribution of means for
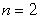
selected from a rectangular distribution has more cases in the middle than at the extremes.
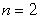
selected from a rectangular distribution has more cases in the middle than at the extremes.
In order to obtain an extremely low or extremely high mean, both scores would have to be extremely low or extremely high, events that would occur with relatively low frequency; on the other hand, there are many ways sample means toward the center can be obtained (a combination of a very high and a very low score, two moderate scores, etc.), and thus they occur with greater frequency.
3
For a normally distributed population of observations, 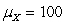
and
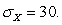
For a randomly selected sample of size 36, what is the probability that the sample mean
(a) will fall above 91
(b)will fall more than 10 points above the population mean of 100
(c)will differ (in either direction) from the population mean by 10 points or more
(d)What sample mean has such a high value that the probability is .01 of obtaining one as high or higher
(e)Within what limits would the central 95% of all possible sample means fall
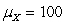
and
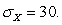
For a randomly selected sample of size 36, what is the probability that the sample mean
(a) will fall above 91
(b)will fall more than 10 points above the population mean of 100
(c)will differ (in either direction) from the population mean by 10 points or more
(d)What sample mean has such a high value that the probability is .01 of obtaining one as high or higher
(e)Within what limits would the central 95% of all possible sample means fall
(a).96
(b).02
(c).05
(d)111.6
(e)90.2-109.8.
(b).02
(c).05
(d)111.6
(e)90.2-109.8.
4
A large population of peer ratings of physical attractiveness is approximately normal with
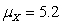
and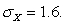
We plan to select a random sample of four ratings from this population. What is the probability of obtaining a sample mean
(a) above 6.0
(b)below 4.0
(c)between the population mean and .5 above the mean
(d)no more than .5 away from the population mean (in either direction)
(e)What sample mean has such a low value that the probability is .05 of obtaining one as low or lower?
(f)What are the centrally placed limits such that the probability is .95 that the sample mean will fall within those limits?
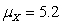
and
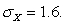
We plan to select a random sample of four ratings from this population. What is the probability of obtaining a sample mean
(a) above 6.0
(b)below 4.0
(c)between the population mean and .5 above the mean
(d)no more than .5 away from the population mean (in either direction)
(e)What sample mean has such a low value that the probability is .05 of obtaining one as low or lower?
(f)What are the centrally placed limits such that the probability is .95 that the sample mean will fall within those limits?

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
5
A widely used IQ test for children is constructed to have a normal distribution with a mean of 100 and a standard deviation of 15 for children in a very, very large national sample. If a child is selected at random from the national sample, what is the probability that his or her IQ score will be
(a) above 90
(b)between 90 and 110
(c)above 150
(d)The probability is .50 that the child's IQ score will be within (how many) IQ points of the mean (on either side)
(e)The probability is .10 that the child's IQ score will fall above what IQ score
(a) above 90
(b)between 90 and 110
(c)above 150
(d)The probability is .50 that the child's IQ score will be within (how many) IQ points of the mean (on either side)
(e)The probability is .10 that the child's IQ score will fall above what IQ score

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
6
In statistical work, it is preferable to define a population as
A) a set of objects
B) a set of people
C) a set of observations
D) any of the above
A) a set of objects
B) a set of people
C) a set of observations
D) any of the above

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
7
A basic aim of statistical inference is to form a conclusion about a
A) sample
B) random sample
C) population
D) random population
A) sample
B) random sample
C) population
D) random population

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
8
In the following statement, which alternative is false? The characteristics of a random sample
A) vary from sample to sample
B) vary less with larger samples
C) make statistical inference possible
D) duplicate those of the population if sampling is truly random
A) vary from sample to sample
B) vary less with larger samples
C) make statistical inference possible
D) duplicate those of the population if sampling is truly random

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
9
A random sample is defined by
A) the method of selection
B) how closely it resembles the population
C) the outcome of selection
D) all of the above
A) the method of selection
B) how closely it resembles the population
C) the outcome of selection
D) all of the above

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
10
Which, if any, is not a characteristic of random sampling?
A) whether a sample is random or not cannot be told from inspection of the sample
B) characteristics of a random sample may differ widely from characteristics of its population
C) a sample must be reasonably large to be considered a random sample
D) every element in the population must be given an equal chance for inclusion in the sample
A) whether a sample is random or not cannot be told from inspection of the sample
B) characteristics of a random sample may differ widely from characteristics of its population
C) a sample must be reasonably large to be considered a random sample
D) every element in the population must be given an equal chance for inclusion in the sample

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
11
The fundamental condition that permits proper statistical inference is
A) random sampling
B) normal distribution of scores
C) knowledge of the values of the parameters of the population
D) having a large sample
A) random sampling
B) normal distribution of scores
C) knowledge of the values of the parameters of the population
D) having a large sample

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
12
If the population consists of four scores: 5, 6, 7, and 8, and we are told that a sample of three scores from this population is: 7, 8, 8, we know that
A) sampling was done with replacement
B) sampling was done without replacement
C) sampling was random
D) sampling was not random
A) sampling was done with replacement
B) sampling was done without replacement
C) sampling was random
D) sampling was not random

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
13
A sampling distribution is a distribution of
A) scores obtained from samples
B) values of a statistic obtained from samples
C) values of a parameter obtained from samples
D) any of the above
A) scores obtained from samples
B) values of a statistic obtained from samples
C) values of a parameter obtained from samples
D) any of the above

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
14
Each score in a random sampling distribution of the mean represents
A) a single individual
B) a random data point
C) a standard score
D) a sample mean
A) a single individual
B) a random data point
C) a standard score
D) a sample mean

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
15
A particular sampling distribution of the mean is based on means of
A) all possible samples of the same size
B) n samples of the same size
C) all possible samples of all possible sizes
D) n samples of all possible sizes
A) all possible samples of the same size
B) n samples of the same size
C) all possible samples of all possible sizes
D) n samples of all possible sizes

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
16
A sampling distribution is
A) a relative frequency distribution
B) a probability distribution
C) both of the above
D) neither of the above
A) a relative frequency distribution
B) a probability distribution
C) both of the above
D) neither of the above

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
17
The difference between a distribution of sample scores and a sampling distribution is that the latter
A) has characteristics specified by statistical theory
B) is a distribution of sample statistics
C) has a mean and standard deviation which can be known in advance
D) is characterized by all of the above, while the former is not
A) has characteristics specified by statistical theory
B) is a distribution of sample statistics
C) has a mean and standard deviation which can be known in advance
D) is characterized by all of the above, while the former is not

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
18
Which is not a characteristic of the random sampling distribution of means?
A) its mean is the same as the mean of the population of scores
B) its standard deviation is greater than that of the population of scores
C) it tends to resemble the normal curve irrespective of the shape of the population of scores
D) its standard deviation changes with variation in sample size
A) its mean is the same as the mean of the population of scores
B) its standard deviation is greater than that of the population of scores
C) it tends to resemble the normal curve irrespective of the shape of the population of scores
D) its standard deviation changes with variation in sample size

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
19
The mean of the sampling distribution of means
A) increases as sample size increased
B) changes as the standard deviation of the population of scores is increased
C) changes from
if the population is not normally distributed
D) is unaffected by any of the above
A) increases as sample size increased
B) changes as the standard deviation of the population of scores is increased
C) changes from
if the population is not normally distributed
D) is unaffected by any of the above

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
20
The standard deviation of the sampling distribution of means
A) increases as sample size increases
B) increases as the mean of the population increases
C) increases as the standard deviation of the population decreases
D) none of the above is true
A) increases as sample size increases
B) increases as the mean of the population increases
C) increases as the standard deviation of the population decreases
D) none of the above is true

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
21
The standard error of the mean
A) is given in terms of standard units
B) is a standard deviation
C) is larger for larger populations
D) is the average amount by which sample values are in error
A) is given in terms of standard units
B) is a standard deviation
C) is larger for larger populations
D) is the average amount by which sample values are in error

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
22
The correct formula for the standard error of the mean is
A)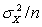
B)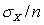
C)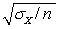
D)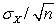
A)
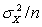
B)
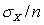
C)
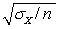
D)
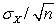

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
23
From the formula for the standard error of the mean, it is apparent that variation among sample means will be decreased when
A) variation among scores in the population is less
B) sample size is larger
C) either (or both) of the above occurs
D) sampling is done with replacement
A) variation among scores in the population is less
B) sample size is larger
C) either (or both) of the above occurs
D) sampling is done with replacement

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck
24
The Central Limit Theorem
A) says that approaches as sample size increases
B) says that S approaches as sample size increases
C) says that both (a) and (b) will occur
D) refers to a matter other than those stated above
A) says that approaches as sample size increases
B) says that S approaches as sample size increases
C) says that both (a) and (b) will occur
D) refers to a matter other than those stated above

Unlock Deck
Unlock for access to all 24 flashcards in this deck.
Unlock Deck
k this deck



