Deck 13: Tests of Hypotheses: Standard Deviations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/39
Play
Full screen (f)
Deck 13: Tests of Hypotheses: Standard Deviations
1
The symbol has which of the following properties?
A) It equals .
B) The area to its right equals .
C) It has degrees of freedom.
D) The area to its left equals .
A) It equals .
B) The area to its right equals .
C) It has degrees of freedom.
D) The area to its left equals .
It has degrees of freedom.
2
To find a confidence interval for the standard deviation from a small sample, we use the values
A) and .
B) and .
C) and .
D) only .
A) and .
B) and .
C) and .
D) only .
and .
3
If a significance level of 0.01 is used in testing the null hypothesis that against the alternative that , based on a random sample of size , then the relevant tabled value is
A) .
B) .
C) .
D) .
A) .
B) .
C) .
D) .
.
4
To test the hypothesis that two population standard deviations are equal, we use which of the following distributions?
A)
B) chi-square
C) normal
D)
A)
B) chi-square
C) normal
D)

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
5
If the hypothesis is tested against the hypothesis that , with , based on a random sample of size , then the appropriate tabled value(s) is(are)
A) only.
B) and .
C) only.
D) only.
A) only.
B) and .
C) only.
D) only.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
6
A computed value that is larger than the value allows us to reject the null hypothesis that __________ is based on a __________.
A) , small sample
B) , large sample
C) , small sample
D) , large sample
A) , small sample
B) , large sample
C) , small sample
D) , large sample

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
7
The hypothesis that two population standard deviations are equal can be rejected at the level of significance if
A)
B) .
C) .
D)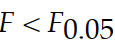
A)
B) .
C) .
D)
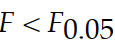

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
8
The more that the sample standard deviation differs from the hypothesized value , the more likely that the null hypothesis
A) can be rejected.
B) cannot be rejected.
C) can be rejected.
D) cannot be rejected.
A) can be rejected.
B) cannot be rejected.
C) can be rejected.
D) cannot be rejected.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
9
In an effort to evaluate the hypothesis that , the sample variances and were obtained, based on sample sizes of and , respectively. The numerator and denominator degrees of freedom are, respectively,
A) 14 and 9 .
B) 15 and 10 .
C) 10 and 15 .
D) 9 and 15
A) 14 and 9 .
B) 15 and 10 .
C) 10 and 15 .
D) 9 and 15

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
10
If , the hypothesis that , the sample variances and were obtained, based on sample sizes of and respectively, the null hypothesis should be
A) rejected since .
B) not rejected since .
C) rejected since .
D) not rejected since .
A) rejected since .
B) not rejected since .
C) rejected since .
D) not rejected since .

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
11
Find the critical value needed to test the null hypothesis that against the alternative hypothesis that 40 at using a sample of size .

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
12
A random sample of 11 copies of a particular mechanical component has a mean length of 5 inches with a standard deviation of .
-Find a confidence interval for the population variance.
-Find a confidence interval for the population variance.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
13
A random sample of 11 copies of a particular mechanical component has a mean length of 5 inches with a standard deviation of .
-Find a confidence interval for the population variance.
-Find a confidence interval for the population variance.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
14
A random sample of 11 copies of a particular mechanical component has a mean length of 5 inches with a standard deviation of .
-Find a confidence interval for the population standard deviation.
-Find a confidence interval for the population standard deviation.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
15
A vending machine company wants to limit the variation in the number of ounces of soda that their machine dispenses into each cup. Use the 0.01 level of significance to test the null hypothesis ounces against the alternative hypothesis ounces based on a random sample of size cups for which .

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
16
A man who needs an operation would like to evaluate the variation in survival rates in different hospitals for his type of operation. He hypothesizes that the standard deviation of survival rates at all hospitals is 0.08 . Test this hypothesis against the alternative hypothesis if a random sample of 12 hospitals produces a standard deviation of 0.11 . Use .

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
17
Calculate the ratio for the set of data and compare it to the appropriate tabled value. Make a decision concerning the null hypothesis of equal population standard deviations.
-
-

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
18
Calculate the ratio for the set of data and compare it to the appropriate tabled value. Make a decision concerning the null hypothesis of equal population standard deviations.
-
-

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
19
An owner of a chain of supermarkets claims that there is greater price competition among supermarkets than among small "convenience" food stores. A sample of ground beef prices in 13 supermarkets and 10 convenience stores gives standard deviations of and , respectively. Evaluate the claim at the significance level.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
20
In a random sample of nine gasoline stations in New York City, the prices per gallon of unleaded gas have a standard deviation of per gallon. In a random sample of 14 gasoline stations in Chicago, the prices per gallon have a standard deviation of per gallon. Use the significance level to test the null hypothesis that the price per gallon of gasoline is equally variable in the two cities.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
21
Finding a large-sample confidence interval for requires the use of the normal distribution, whereas finding a small-sample confidence interval for requires the use of distribution.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
22
In testing the null hypothesis that a population standard deviation equals a specified constant, the value is the hypothesized standard deviation.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
23
The procedure used to evaluate the null hypothesis is the same as the procedure used to evaluate the null hypothesis where is a constant.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
24
The ratio can be used to evaluate the null hypothesis that the population standard deviation equals a given constant.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
25
It is always true that the higher the value of the more likely the null hypothesis that will be rejected.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
26
A hypothesis test involving one population standard deviation sometimes involves the calculation of .

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
27
The value of the standard deviation is never obtained from a sample.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
28
If has a value very close to 0 , then the null hypothesis of equal population standard deviations cannot be rejected.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
29
An ratio is the ratio of two population standard deviations.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
30
In order to test the hypothesis that a population is a specified constant, it is never necessary to know the mean of the sample.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
31
A sample estimate of is symbolized by __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
32
The mean of chi-square distribution is equal to __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
33
The possible values that a chi-square distribution can assume are __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
34
If and , then the ratio we use is __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
35
In testing the null hypothesis that a population standard deviation equals a specified constant based on a small sample, the test statistic that is used is __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
36
In tests concerning the equality of two population variances, the test statistic is __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
37
In order to convert a small-sample confidence interval for the variance to a confidence interval for the standard deviation we must __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
38
When finding a confidence interval for , based on small sample, the number of degrees of freedom for the chi-square distribution is __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck
39
In testing for equality of two standard deviations using the statistic of , the table value that must be exceeded to reject the null hypothesis is given by the symbol __________.

Unlock Deck
Unlock for access to all 39 flashcards in this deck.
Unlock Deck
k this deck



