Deck 7: Probability

Question 1

Use the following information for questions:
For each situation, decide if the probability described is a subjective (personal) probability or a relative frequency probability.

-In a sample of 1000 students majoring in the humanities, 660 were female. The 66% (660/1000) chance of a humanities major being female is a

A) subjective probability.
B) relative frequency probability.

Answer 1

relative frequency probability.

Question 2

Use the following information for questions:
For each situation, decide if the probability described is a subjective (personal) probability or a relative frequency probability.

-A quarter is flipped 2000 times and results in 500 heads. The 25% (500/2000) chance of heads is a

A) subjective probability.
B) relative frequency probability.

Answer 2

relative frequency probability.

Question 3

Use the following information for questions:
For each situation, decide if the probability described is a subjective (personal) probability or a relative frequency probability.

-Among 5000 new tires sold by a tire company, 20% (1000/5000) lasted more than 100,000 miles. The 20% chance that a new tire will last more than 100,000 miles is a

A) subjective probability.
B) relative frequency probability.

Answer 3

relative frequency probability.

Question 4

Use the following information for questions:
For each situation, decide if the probability described is a subjective (personal) probability or a relative frequency probability.

-A football fan believes that the Oakland Raiders have a 50% chance of winning the next Super bowl. The 50% is a

A) subjective probability
B) relative frequency probability

Question 5

Use the following information for questions:
For each situation, decide if the probability described is a subjective (personal) probability or a relative frequency probability.

-A college basketball player has made 53% of his shots from 3-point range. The probability that he will make a 3-point shot, 53%, is a

A) subjective probability.
B) relative frequency probability.

Question 6

Use the following information for questions:
For each situation, decide if the probability described is a subjective (personal) probability or a relative frequency probability.

-A recent college graduate claims he only has a 30% chance of finding a job in the next two months. The chance of 30% is a

A) subjective probability.
B) relative frequency probability.

Question 7

Use the following information for questions:
In the past five years, only 5% of pre-school children did not improve their swimming skills after taking a Beginner Swimmer Class at a certain Recreation Center.

-The probability of 5% that a pre-school child who is taking this swim class will not improve his/her swimming skills is a

A) subjective probability.
B) relative frequency probability.

Question 8

Use the following information for questions:
In the past five years, only 5% of pre-school children did not improve their swimming skills after taking a Beginner Swimmer Class at a certain Recreation Center.

-What is the probability that a pre-school child who is taking this swim class will improve his/her swimming skills?

A) 5%
B) 10%
C) 95%
D) None of the above

Question 9

A survey was given to sophomores at a university and one of the questions asked was "What is the probability that you will leave school before you graduate?"The answer to this question for an individual student is an example of

A) a relative frequency probability based on long-run observation.
B) a relative frequency probability based on physical assumptions.
C) a personal probability.
D) a probability based on measuring a representative sample and observing relative frequencies that fall into various categories.

Question 10

A thumbtack is tossed repeatedly and observed to see if the point lands resting on the floor or sticking up in the air. The goal is to estimate the probability that a thumbtack would land with the point up. That probability is an example of

A) a relative frequency probability based on long-run observation.
B) a relative frequency probability based on physical assumptions.
C) a personal probability.
D) a probability based on measuring a representative sample and observing relative frequencies that fall into various categories.

Question 11

Which of the following is an example of a relative frequency probability based on measuring a representative sample and observing relative frequencies of possible outcomes?

A) According to the late Carl Sagan, the probability that the earth will be hit by a civilization-threatening asteroid in the next century is about 0.001.
B) If you flip a fair coin, the probability that it lands with heads up is ½.
C) Based on a recent Newsweek poll, the probability that a randomly selected adult in the US would say they oppose federal funding for stem cell research is about 0.37.
D) A new airline boasts that the probability that its flights will be on time is 0.92, because 92% of all flights it has ever flown did arrive on time.

Question 12

A survey was given to sophomores at a university and one of the questions asked was "What is the probability that you will leave school before you graduate?"The answer to this question for an individual student is an example of

A) a relative frequency probability based on long-run observation.
B) a relative frequency probability based on physical assumptions.
C) a probability based on measuring a representative sample and observing relative frequencies that fall into various categories.
D) a personal probability.

Question 13

Students at a university who apply for campus housing can be assigned to live in a dormitory room, a suite in a dormitory or an apartment. If Alice has a 0.30 chance of being assigned to a dormitory room and a 0.50 chance of being assigned to a suite in a dormitory, what is the probability that she will be assigned to an apartment?

Question 14

When a fair coin is tossed two times, there is a 25% chance of getting 0 heads and a 50% chance of getting 1 head. What is the chance of getting 2 heads?

Question 15

When 2 cards are drawn at random from a standard 52-card deck, there is a 0.8507 chance of drawing 0 Aces, and a 0.1448 chance of drawing 1 Ace. What is the chance of drawing 2 Aces?

Question 16

A plane flies over the ocean in search of schools of fish. On any given day, there is a 0.07 chance of finding 2 or more schools of fish, and a 0.28 chance of finding only one school of fish. What is the chance the day of searching results in not finding any schools of fish at all?

Question 17

When two dice are rolled, there are 36 possible outcomes. Six of these outcomes have two the same numbers (two ones, two twos, etc.). The chance of getting two of the same numbers when rolling two dice is 6/36 or 0.1667. What is the chance of getting 2 numbers that are different from one another?

Question 18

Every year, on the first day of school, the sixth grade teacher at Greenville Middle School asks his students to pick their favorite primary color: red, yellow, or blue. From years of experience, the teacher knows that only 1 in 7 students choose yellow and that about half of the students pick blue. What is the chance that a student picks red?

Question 19

The lifetime risk for a woman to develop breast cancer is estimated to be about 1 in 8. Express this probability as a fraction, as a proportion, and as a percentage.

Question 20

Use the following information for questions:
A statistics class has 4 teaching assistants (TAs): three female assistants (Lauren, Rona, and Leila) and one male assistant (Josh). Each TA teaches one discussion section.

-A student picks a discussion section. The two events W = {the TA is a woman} and J = {the TA is Josh} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 21

Use the following information for questions:
A statistics class has 4 teaching assistants (TAs): three female assistants (Lauren, Rona, and Leila) and one male assistant (Josh). Each TA teaches one discussion section.

-A student picks a discussion section. The events L = {the TA is Lauren} and R = {the TA is Rona} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 22

Use the following information for questions:
A statistics class has 4 teaching assistants (TAs): three female assistants (Lauren, Rona, and Leila) and one male assistant (Josh). Each TA teaches one discussion section.

-A student picks a discussion section. The two events W = {the TA is a woman} and M = {the TA is a man} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 23

Use the following information for questions:
A statistics class has 4 teaching assistants (TAs): three female assistants (Lauren, Rona, and Leila) and one male assistant (Josh). Each TA teaches one discussion section.

-Two students, Bill and Tom, who don't know each other, each pick a discussion section. The two events B = {Bill's TA is Lauren} and T = {Tom's TA is a woman} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 24

Use the following information for questions:
Students who live in the dorms at a college get free T.V. service in their rooms, but only receive 6 stations. On a certain evening, a student wants to watch T.V. and the six stations are broadcasting separate shows on baseball, football, basketball, local news, national news, and international news. The student is too tired to check which channels the shows are playing on, so the student picks a channel at random.

-The two events A = {the student watches an athletic event} and N = {the student watches a news broadcast} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 25

Use the following information for questions:
Students who live in the dorms at a college get free T.V. service in their rooms, but only receive 6 stations. On a certain evening, a student wants to watch T.V. and the six stations are broadcasting separate shows on baseball, football, basketball, local news, national news, and international news. The student is too tired to check which channels the shows are playing on, so the student picks a channel at random.

-The events F = {the student watches football} and B = {the student watches baseball} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 26

Use the following information for questions:
Students who live in the dorms at a college get free T.V. service in their rooms, but only receive 6 stations. On a certain evening, a student wants to watch T.V. and the six stations are broadcasting separate shows on baseball, football, basketball, local news, national news, and international news. The student is too tired to check which channels the shows are playing on, so the student picks a channel at random.

-The two events F = {the student watches football} and A = {the student watches an athletic event} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 27

Use the following information for questions:
Students who live in the dorms at a college get free T.V. service in their rooms, but only receive 6 stations. On a certain evening, a student wants to watch T.V. and the six stations are broadcasting separate shows on baseball, football, basketball, local news, national news, and international news. The student is too tired to check which channels the shows are playing on, so the student picks a channel at random.

-On a different night, two students who don't know each other each choose a channel this way. The two events, N = {the first student watches a news broadcast } and F = {the second student watches football} are

A) independent events.
B) disjoint (mutually exclusive) events.
C) each simple events.
D) None of the above.

Question 28

Lauren wants to wear something warm when she leaves for class. She reaches into her coat closet without looking and grabs a hanger. Based on what she has in her coat closet, she has a 30% chance of picking a sweater, a 50% chance of picking a coat, and a 20% chance of picking a jacket. What is the probability that she will pick a sweater or a coat?

A) 15%
B) 30%
C) 50%
D) 80%

Question 29

A birth is selected at random. Define events B = {the baby is a boy} and F = {the mother had the flu during her pregnancy}. The events B and F are

A) disjoint but not independent.
B) independent but not disjoint.
C) disjoint and independent.
D) neither disjoint nor independent.

Question 30

Use the following information for questions:
A student is randomly selected from a large college campus. Define the eventsH = {the student has blond hair} and E = {the student has blue eyes}.

-The events H and E are

A) disjoint but not independent.
B) independent but not disjoint.
C) disjoint and independent.
D) neither disjoint nor independent.

Question 31

Use the following information for questions:
A student is randomly selected from a large college campus. Define the eventsH = {the student has blond hair} and E = {the student has blue eyes}.

-The chance that a blond haired student has blue eyes equals 75%. How do we write this probability?

A) P(H) = 0.75
B) P(E) = 0.75
C) P(E|H) = 0.75
D) P(H|E) = 0.75

Question 32

Use the following information for questions:
A student is randomly selected from a large college. Define the events C = {the student owns a cell phone} and I = {the student owns an iPod}.

-Which of the following is the correct interpretation of the probability P(I|C)?

A) The chance that a randomly selected student owns an iPod.
B) The percentage of students who own both a cell phone and an iPod.
C) The proportion of students who own a cell phone who also own an iPod.
D) The relative frequency of iPod owners who own a cell phone.

Question 33

Use the following information for questions:
A student is randomly selected from a large college. Define the events C = {the student owns a cell phone} and I = {the student owns an iPod}.

-Which of the following is the correct notation for the percentage of students who own a cell phone but not an iPod?

A) P(C|I)
B) P(C|I^C)
C) P(C^C and I)
D) P(C and I^C)

Question 34

Use the following information for questions:
A student is randomly selected from a large college. Define the events C = {the student owns a cell phone} and I = {the student owns an iPod}.

-Suppose you know that your friend's sister, who attends this college, owns a cell phone and you are wondering what would be the chance that she owns an iPod. What type of probability would this be?

A) A probability of independent events.
B) A probability of dependent events.
C) A conditional probability.
D) A probability of disjoint events.

Question 35

Use the following information for questions:
A football player is randomly selected from all NCAA Division I college teams. Define the events D = {the football player plays defense} and T = {the football player is over 6 feet tall}.
-How do we interpret the probability P(D|T)?

Question 36

Use the following information for questions:
A football player is randomly selected from all NCAA Division I college teams. Define the events D = {the football player plays defense} and T = {the football player is over 6 feet tall}.
-Suppose the percentage of football players who play defense that are shorter than 6 feet tall is only 8%. How do write this statement using events D and T?

Question 37

Use the following information for questions:
A football player is randomly selected from all NCAA Division I college teams. Define the events D = {the football player plays defense} and T = {the football player is over 6 feet tall}.

-Refer to question 36. Suppose that we also know that of all NCAA Division I football players, 15% are shorter than 6 feet. What do we know about events D and T?

A) They are independent events.
B) They are dependent events.
C) They are disjoint events.

Question 38

At a particular university, a study has shown that students who live on campus are more likely to have GPAs over 3.0 than students who do not. A freshman at this university is randomly selected at the beginning of the fall term. Define events A = {the student lives on campus}, and B = {the student has a GPA over 3.0 at the end of the fall term}. According to the housing office, P(A) = .80. Which statement is definitely true about P(B|A) based on this information?

A) P(B|A) = .80
B) P(B|A) is greater than P(B|not A)
C) P(B|A) is less than P(B|not A)
D) P(B|A) = P(B|not A)

Question 39

Michael wants to take French or Spanish, or both. But classes are closed, and he must apply and get accepted to be allowed to enroll in a language class. He has a 50% chance of being admitted to French, a 50% chance of being admitted to Spanish, and a 20% chance of being admitted to both French and Spanish. If he applies to both French and Spanish, the probability that he will be enrolled in either French or Spanish (or possibly both) is

A) 70%
B) 80%
C) 90%
D) 100%

Question 40

If one card is randomly picked from a standard deck of 52 cards, the probability that the card will be a number from 2 through 10, or a Heart, or both, is

A) 51.9% (27/52)
B) 69.2% (36/52)
C) 76.9% (40/52)
D) 94.2% (49.52)

Question 41

If one card is randomly picked from a standard deck of 52 cards, the probability that the card will be a red suit (Heart or Diamond), or a face card (Jack, Queen, or King), or both, is

A) 50.0% (26/52)
B) 61.5% (32/52)
C) 76.9% (40/52)
D) 88.5% (46/52)

Question 42

Use the following information for questions:
A standard 52-card deck is shuffled and 2 cards are picked from the top of the deck.

-The probability that the first card is a Heart and the second card is a Spade is

A) 5.9%
B) 6.3%
C) 6.4%
D) 25.0%

Question 43

Use the following information for questions:
A standard 52-card deck is shuffled and 2 cards are picked from the top of the deck.

-The probability that the both cards are Hearts is

A) 6.3%
B) 5.9%
C) 6.4%
D) 25.0%

Question 44

Use the following information for questions:
A standard 52-card deck is shuffled and 2 cards are picked from the top of the deck.

-The probability that the first card is a face card (Jack, Queen, King) and the second card is not a face card is

A) 5.3%
B) 17.8%
C) 18.1%
D) 59.2%

Question 45

Two standard 52-card decks are shuffled and two cards are picked at random - one card from each deck. The probability that two Hearts are drawn is

A) 5.9%
B) 6.3%
C) 25.0 %
D) 50.0%

Question 46

Use the following information for questions:
A card is drawn at random from a standard 52-card deck.

-The conditional probability that the card is a King given that a face card (Jack, Queen, or King) was drawn is

A) 20.0% (1/5)
B) 25.0% (1/4)
C) 33.3% (1/3)
D) 50.0% (1/2)

Question 47

Use the following information for questions:
A card is drawn at random from a standard 52-card deck.

-The conditional probability that the card is a 2 given that a 2 or a 3 was drawn is

A) 20.0% (1/5)
B) 25.0% (1/4)
C) 33.3% (1/3)
D) 50.0% (1/2)

Question 48

A soft drink company holds a contest in which a prize may be revealed on the inside of the bottle cap. The probability that each bottle cap reveals a prize is 0.2 and winning is independent from one bottle to the next. What is the probability that a customer must open three or more bottles before winning a prize?

A) (0.2)(0.2)(0.8) = 0.032
B) (0.8)(0.8)(0.2) = 0.128
C) (0.8)(0.8) = 0.64
D) 1 - (0.2)(0.2)(0.8) = .968

Question 49

Use the following information for questions:
Suppose two different states each pick a two-digit lottery number between 00 and 99 (for a 100 possible numbers).

-What is the probability that both states pick the number 13?

A) 2/100
B) 1/100
C) 1/200
D) 1/10,000

Question 50

Use the following information for questions:
Suppose two different states each pick a two-digit lottery number between 00 and 99 (for a 100 possible numbers).

-What is the probability that both states pick the same number?

A) 2/100
B) 1/100
C) 1/200
D) 1/10,000

Question 51

A six-sided die is made that has four Green sides and two Red sides, all equally likely to land face up when the die is tossed. The die is tossed three times. Which of these sequences (in the order shown) has the highest probability?

A) Green, Green, Green
B) Green, Green, Red
C) Green, Red, Red
D) They are all equally likely

Question 52

Use the following information for questions:
A student doing an internship at a large research firm collected the following data, representing all of the studies the firm had conducted over the past 3 years.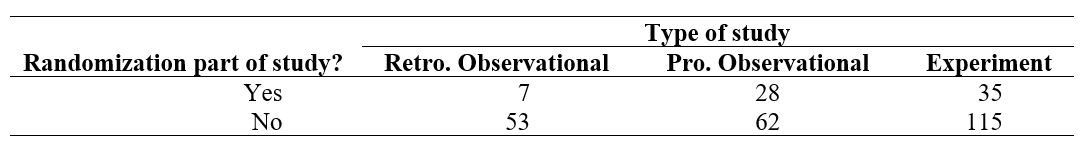

-Suppose one study is to be randomly selected from all studies conducted over the past 3 years by this large research firm. What is the probability that this study used randomization?

A) 0.1167
B) 0.2333
C) 0.26
D) 0.3111

Question 53

Use the following information for questions:
A student doing an internship at a large research firm collected the following data, representing all of the studies the firm had conducted over the past 3 years.

-What is the probability that the study used randomization, if we already know that the study was a retrospective observational study?

A) 0.1167
B) 0.2333
C) 0.26
D) 0.3111

Question 54

Use the following information for questions:
A student doing an internship at a large research firm collected the following data, representing all of the studies the firm had conducted over the past 3 years.

-Define the events E = {the study was an experiment}, U = {the study used randomization} and R = {the study was a retrospective observational study}. Which of the following sets of events are disjoint?

A) E and U
B) E and R
C) U and R
D) All three
E) None of the above

Question 55

Use the following information for questions:
A student doing an internship at a large research firm collected the following data, representing all of the studies the firm had conducted over the past 3 years.

-Define the events E = {the study was an experiment} and U = {the study used randomization}. Are the events E and U independent?

A) Yes
B) No
C) Can't tell
D) Need more information to determine this.

Question 56

Use the following information for questions:
For each of the following statements, determine if they are true or false.

-The probability of the intersection of two events A and B, and the probability of the union of A and B can never be equal.

Question 57

Use the following information for questions:
For each of the following statements, determine if they are true or false.

-If events A and B are known to be independent and P(A) = 0.2 and P(B) = 0.3, then P(A and B) = 0.5.

Question 58

Use the following information for questions:
For each of the following statements, determine if they are true or false.

-If events D and E are known to be mutually exclusive, then P(D|E) = 0.

Question 59

Which of the following is definitely true for mutually exclusive events A and B?

A) P(A) = 1 - P(B)
B) P(A) + P(B) = 1
C) P(A and B) = P(A)P(B)
D) P(A and B) = 0

Question 60

Suppose that the probability of event A is 0.2 and the probability of event B is 0.4. Also, suppose that the two events are independent. Then P(A|B) is:

A) P(A) = 0.2
B) P(A) / P(B) = 0.2/0.4 = ½
C) P(A)P(B) = (0.2)(0.4) = 0.08
D) None of the above.

Question 61

When two cards are drawn at random from a 52 card deck, the probability of drawing one Jack is 0.145. The probability of drawing one Ace is 0.145, and the probability of drawing a Jack and an Ace is 0.012. What is the probability of drawing a Jack or an Ace?

Question 62

Use the following information for questions:
When a fair coin is flipped 6 times, the probability of getting an odd number of heads(1, 3, or 5) is 0.5.
-What is the probability of getting an even number of heads (0, 2, 4, or 6)?

Question 63

Use the following information for questions:
When a fair coin is flipped 6 times, the probability of getting an odd number of heads(1, 3, or 5) is 0.5.
-The probability of getting more than 3 heads is 0.344, and the probability of getting 5 heads is 0.094. What is the probability of getting either an odd number of heads or more than 3 heads?

Question 64

Use the following information for questions:
A mail-order company classifies its customers by gender (male/female) and by location of residence (urban/suburban). The market research department has determined that 2/3 of their customers are female and that 75% of their customers live in the suburbs. They have also determined that gender and location of residence seem to be independent of one another.
-If the mail order company will randomly select one of their customers, what is the probability that it will be a suburban female?

Question 65

Use the following information for questions:
A mail-order company classifies its customers by gender (male/female) and by location of residence (urban/suburban). The market research department has determined that 2/3 of their customers are female and that 75% of their customers live in the suburbs. They have also determined that gender and location of residence seem to be independent of one another.
-The mail order company will randomly select one of their customers. Suppose we know they have selected a person in the suburbs. What is the probability that this person is female?

Question 66

Use the following information for questions:
A mail-order company classifies its customers by gender (male/female) and by location of residence (urban/suburban). The market research department has determined that 2/3 of their customers are female and that 75% of their customers live in the suburbs. They have also determined that gender and location of residence seem to be independent of one another.
-Making up the majority of their customers, the females and suburban customers are identified as the focus group for their new ad campaign. If the mail order company will randomly select one of their customers, what is the probability that it will be a suburban customer or a female?

Question 67

Use the following information for questions:
A study was conducted at a small college on first-year students living on campus. A number of variables were measured. The table below provides information regarding number of roommates and end of term health status for the first-year students at this college. Health status for individuals is measured as poor, average, and exceptional.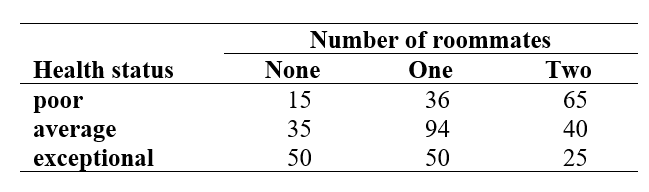
-What is the probability that a randomly selected first-year student with no roommates had poor end of term health status?

Question 68

Use the following information for questions:
A study was conducted at a small college on first-year students living on campus. A number of variables were measured. The table below provides information regarding number of roommates and end of term health status for the first-year students at this college. Health status for individuals is measured as poor, average, and exceptional.
-What is the probability that a randomly selected first-year student with 1 roommate had poor end of term health status?

Question 69

Use the following information for questions:
A study was conducted at a small college on first-year students living on campus. A number of variables were measured. The table below provides information regarding number of roommates and end of term health status for the first-year students at this college. Health status for individuals is measured as poor, average, and exceptional.
-Are the events H = {the student has poor health status} and N = {the student has no roommates} mutually exclusive?

Question 70

Use the following information for questions:
A study was conducted at a small college on first-year students living on campus. A number of variables were measured. The table below provides information regarding number of roommates and end of term health status for the first-year students at this college. Health status for individuals is measured as poor, average, and exceptional.
-Are the events H = {the student has poor health status} and N = {the student has no roommates} independent?

Question 71

Use the following information for questions:
The following table provides information regarding health status and smoking status of residents of a small community (health status for individuals was measured by the number of visits to the hospital during the year).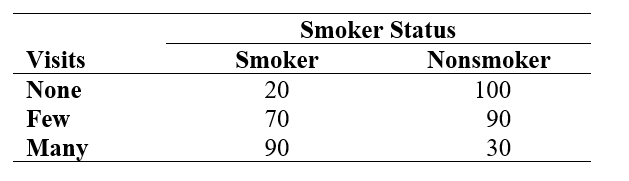
-What is the probability that a randomly selected resident made zero visits to the hospital?

Question 72

Use the following information for questions:
The following table provides information regarding health status and smoking status of residents of a small community (health status for individuals was measured by the number of visits to the hospital during the year).
-What is the probability that a randomly selected nonsmoker made zero visits to the hospital?

Question 73

Use the following information for questions:
The following table provides information regarding health status and smoking status of residents of a small community (health status for individuals was measured by the number of visits to the hospital during the year).
-Are the events N = {the resident does not smoke} and Z = {the resident made zero visits to the hospital} mutually exclusive?

Question 74

Use the following information for questions:
The following table provides information regarding health status and smoking status of residents of a small community (health status for individuals was measured by the number of visits to the hospital during the year).
-Are the events N = {the resident does not smoke} and Z = {the resident made zero visits to the hospital} independent?

Question 75

Use the following information for questions:
In a survey of 1000 adults, respondents were asked about the expense of a college education and the relative necessity of financial assistance. The correspondents were classified as to whether they currently had a child in college or not (college status), and whether they thought the loan obligation for most college students was too high, about right, or too little (loan obligation opinion). The table below summarizes some of the survey results. Use these results to answer the following questions.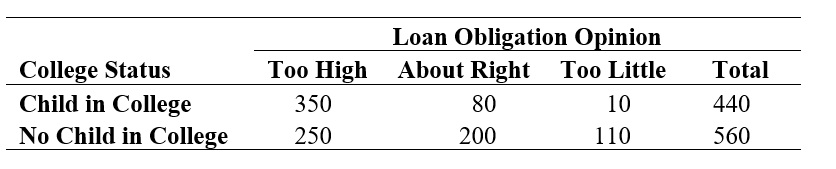
-What is the probability that a randomly selected adult will think loan obligations are too high?

Question 76

Use the following information for questions:
In a survey of 1000 adults, respondents were asked about the expense of a college education and the relative necessity of financial assistance. The correspondents were classified as to whether they currently had a child in college or not (college status), and whether they thought the loan obligation for most college students was too high, about right, or too little (loan obligation opinion). The table below summarizes some of the survey results. Use these results to answer the following questions.
-What is the probability that a randomly selected adult with a child in college will think loan obligations are too high?

Question 77

Use the following information for questions:
In a survey of 1000 adults, respondents were asked about the expense of a college education and the relative necessity of financial assistance. The correspondents were classified as to whether they currently had a child in college or not (college status), and whether they thought the loan obligation for most college students was too high, about right, or too little (loan obligation opinion). The table below summarizes some of the survey results. Use these results to answer the following questions.
-Are the events H = {the adult thinks loan obligations are too high} and C = {the adult has a child in college} mutually exclusive?

Question 78

Use the following information for questions:
In a survey of 1000 adults, respondents were asked about the expense of a college education and the relative necessity of financial assistance. The correspondents were classified as to whether they currently had a child in college or not (college status), and whether they thought the loan obligation for most college students was too high, about right, or too little (loan obligation opinion). The table below summarizes some of the survey results. Use these results to answer the following questions.
-Are the events H = {the adult thinks loan obligations are too high} and C = {the adult has a child in college} independent?

Question 79

Use the following information for questions:
A standard 52-card deck is shuffled and 5 cards are picked from the top of the deck.

-The probability that the first four cards are a red suit (Heart or Diamond) and the last card is a black suit (Spade or Club) is

A) 2.53%
B) 2.99%
C) 3.13%
D) 50.0%

Question 80

Use the following information for questions:
A standard 52-card deck is shuffled and 5 cards are picked from the top of the deck.

-The probability that the first four cards are Aces and the last card is a 2 is

A) 3.1 ×10^-7
B) 3.7 × 10^-6
C) 2.7 × 10^-6
D) 0.077